The mean of the following frequency distribution is 62.8 and the sum of all the frequencies is 50. Compute the missing frequency f1 and f2 . Class: 0-20 20-40 40-60 60-80 80-100 100-120 Frequency: 5 f1 10 f2 7 8
To solve the problem, we need to find the missing frequencies f1 and f2 in the given frequency distribution. The mean of the distribution is provided as 62.8, and the total sum of frequencies is 50.
Step 1: Set up the frequency distribution table
We start by organizing the data into a table format:
| Class | Frequency (f_i) | Midpoint (x_i) | f_i * x_i |
|------------|------------------|----------------|-----------|
| 0 - 20 | 5 | 10 | 50 |
| 20 - 40 | f1 | 30 | 30f1 |
| 40 - 60 | 10 | 50 | 500 |
| 60 - 80 | f2 | 70 | 70f2 |
| 80 - 100 | 7 | 90 | 630 |
| 100 - 120 | 8 | 100 | 800 |
| Total | 50 | | |
Step 2: Calculate the total frequency
From the problem, we know that the total frequency n is:
n=5+f1+10+f2+7+8=50
This simplifies to:
f1+f2+30=50
Thus, we can derive our first equation:
f1+f2=20(Equation 1)
Step 3: Calculate the total of fi×xi
Next, we calculate the total of fi×xi:
Total fixi=50+30f1+500+70f2+630+800
This simplifies to:
fixi=1980+30f1+70f2
Step 4: Use the mean to set up the second equation
The mean is given by the formula:
Mean=∑fixi∑fi
Substituting the known values:
62.8=1980+30f1+70f250
Multiplying both sides by 50 gives:
3140=1980+30f1+70f2
Rearranging this gives us our second equation:
30f1+70f2=1160(Equation 2)
Step 5: Solve the system of equations
Now we have two equations:
1. f1+f2=20 (Equation 1)
2. 30f1+70f2=1160 (Equation 2)
From Equation 1, we can express f2 in terms of f1:
f2=20−f1
Substituting this into Equation 2:
30f1+70(20−f1)=1160
Expanding this gives:
30f1+1400−70f1=1160
Combining like terms results in:
−40f1+1400=1160
Subtracting 1400 from both sides:
−40f1=−240
Dividing by -40:
f1=6
Step 6: Find f2
Now substituting f1 back into Equation 1:
6+f2=20
Thus:
f2=20−6=14
Final Answer
The missing frequencies are:
f1=6,f2=14
We start by organizing the data into a table format:
|------------|------------------|----------------|-----------|
| 0 - 20 | 5 | 10 | 50 |
| 20 - 40 | f1 | 30 | 30f1 |
| 40 - 60 | 10 | 50 | 500 |
| 60 - 80 | f2 | 70 | 70f2 |
| 80 - 100 | 7 | 90 | 630 |
| 100 - 120 | 8 | 100 | 800 |
| Total | 50 | | |
From the problem, we know that the total frequency n is:
Next, we calculate the total of fi×xi:
The mean is given by the formula:
Now we have two equations:
2. 30f1+70f2=1160 (Equation 2)
Now substituting f1 back into Equation 1:
The missing frequencies are:
Topper's Solved these Questions
Similar Questions
The mean of the following frequency distribution is 2117. Find the value of f
 View Solution
View SolutionThe mean of the following distribution is 62.8 and the sum of all the frequencies is 50. Find the missing frequencies f1 and f2
 View Solution
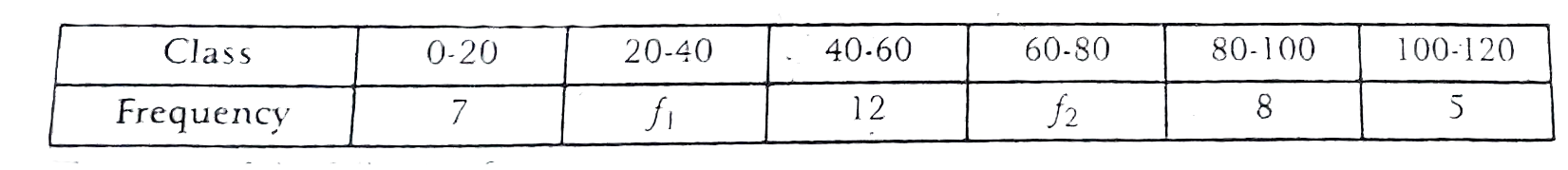
View SolutionThe mean of the following frequency distribution is 57.6 and the sum of the observations is 50. Find the missing frquencies f1andf2 :
 View Solution
View SolutionThe mean of the following frequency distribution is 50 Find f1 f2 f3 if f2 : f3 = 4 : 3:
 View Solution
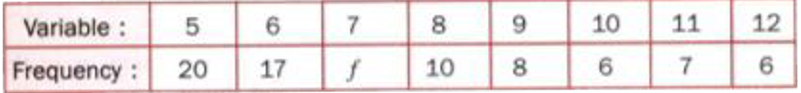
View SolutionIf the mean of the following distribution is 7.5, find the missing frequency f
 View Solution
View SolutionThe mean of the following distribution is 18. Find the frequency f of the class 19−21.
View SolutionThe mean of the following distribution is 52 and the frequency of class interval 30-40 is f. Find f.
View SolutionFind the mean of the following frequency distribution: Classes: 0-20 20-40 40-60 60-80 80-100 Frequency: 15 18 21 29 17
View SolutionFind the mean of the following frequency distribution: Classes: 0-20 20-40 40-60 60-80 80-100 Frequency: 15 18 21 29 17
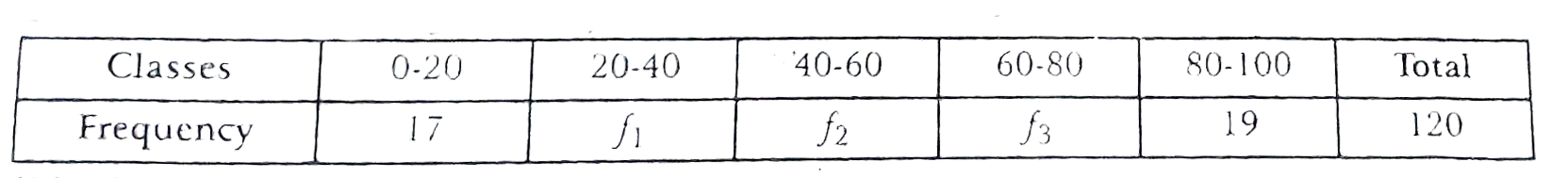
View SolutionThe mean of the following frequency table 50. But the frequencies f1andf2 in class 20−40and60−80 are missing. Find the missing frequencies. Class: 0-20 20-40 40-60 60-80 80-100 Total Frequency: 17 f1 32 f2 19 120
View Solution

