The median of 100 observations grouped in classes of equal width is 25. If the median class interval is 20-30 and the number of observations less than 20 is 45, then the frequency of median class is
20
12
10
15
The correct Answer is:C
To find the frequency of the median class, we can use the formula for the median in a grouped frequency distribution. Let's break down the solution step-by-step.
Step 1: Identify the given values
- Total number of observations (n) = 100
- Number of observations less than 20 (f) = 45
- Median class interval = 20-30
- Lower boundary of the median class (l) = 20
- Width of the class interval (h) = 10 (since the class is 20-30)
Step 2: Calculate the cumulative frequency less than the median class
The cumulative frequency less than the median class (20-30) is given as:
- Cumulative frequency less than 20 = 45
Let the frequency of the median class (20-30) be denoted as f. Therefore, the cumulative frequency less than 30 will be:
- Cumulative frequency less than 30 = Cumulative frequency less than 20 + Frequency of the median class
- Cumulative frequency less than 30 = 45 + f
Step 3: Use the median formula
The formula for the median in a grouped frequency distribution is given by:
Median=l+n2−Ff×h
Where:
- Median = 25
- l = 20 (lower boundary of the median class)
- n = 100 (total number of observations)
- F = cumulative frequency less than the median class = 45
- f = frequency of the median class
- h = width of the class = 10
Step 4: Substitute the known values into the median formula
Substituting the values into the formula:
25=20+1002−45f×10
25=20+50−45f×10
25=20+5f×10
25=20+50f
Step 5: Rearrange the equation to solve for f
Subtract 20 from both sides:
5=50f
Now, cross-multiply to solve for f:
5f=50
f=505
f=10
Conclusion
The frequency of the median class (20-30) is 10.
- Total number of observations (n) = 100
- Number of observations less than 20 (f) = 45
- Median class interval = 20-30
- Lower boundary of the median class (l) = 20
- Width of the class interval (h) = 10 (since the class is 20-30)
The cumulative frequency less than the median class (20-30) is given as:
- Cumulative frequency less than 20 = 45
- Cumulative frequency less than 30 = Cumulative frequency less than 20 + Frequency of the median class
- Cumulative frequency less than 30 = 45 + f
The formula for the median in a grouped frequency distribution is given by:
Median=l+n2−Ff×h
Where:
- Median = 25
- l = 20 (lower boundary of the median class)
- n = 100 (total number of observations)
- F = cumulative frequency less than the median class = 45
- f = frequency of the median class
- h = width of the class = 10
Substituting the values into the formula:
25=20+1002−45f×10
25=20+50−45f×10
25=20+5f×10
25=20+50f
Subtract 20 from both sides:
5=50f
Now, cross-multiply to solve for f:
5f=50
f=505
f=10
The frequency of the median class (20-30) is 10.
Topper's Solved these Questions
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Exercise
40 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Chapter Test
29 Videos
- MISCELLANEOUS EQUATIONS AND INEQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Chapter Test
29 Videos
Similar Questions
When the number of observations (n) is odd, then median is equal to
View SolutionIn the class intervales 10-20, 20-30, the number is 20 is included in
View SolutionIf the median of 25 observations is 45 and if the observations greater than the median are increased by 4, then the median of the new data is
View SolutionThe median of the 10 different observations is 46. If the observation 70 is replaced with 100 then the new median is _________
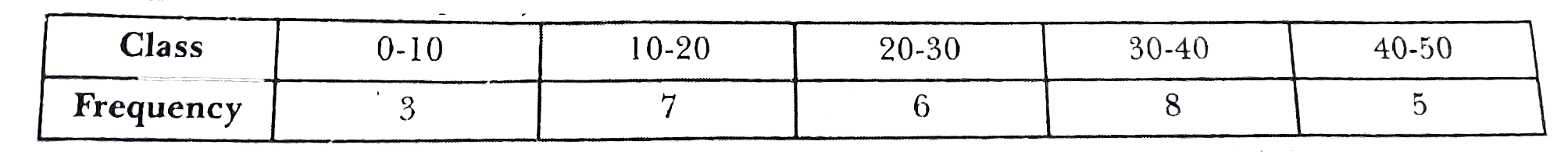
View SolutionWrite the comulative frequency of class interval 30-40
View SolutionThe median of 11 observations is 10. The number of possible observations in the data which are less than 10 is 5 (b) 6 3 (d) 10
View SolutionFor the following distribution
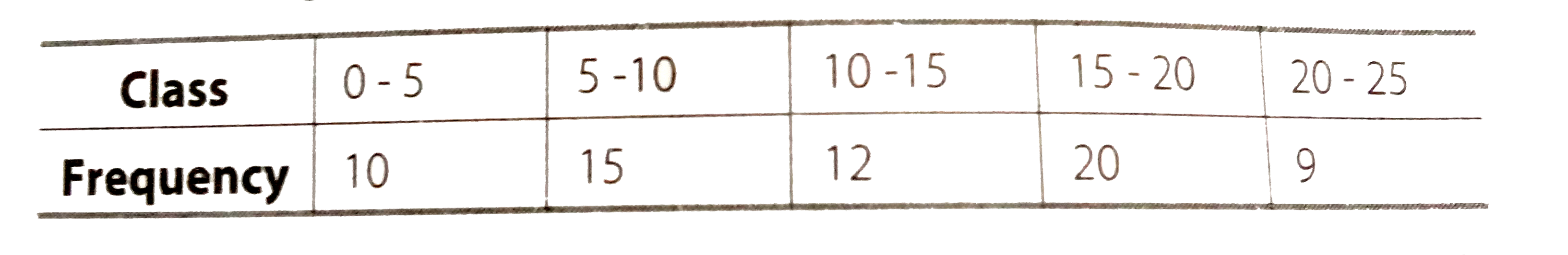
the sum of lower limits of the median class and modal class is
View SolutionIn the following data, find the values of a and b . Find the median class modal class :

View SolutionThe median of a series is 10. Two additional observations 7 and 20 are added to series. The median of new series is
View SolutionThe median of a set of nine distinct observations is 20.5. If each of the last four observations of the set is increased by 2, then the median of the new set is
View Solution
