The probability distribution of the discrete random variables XandY are given below: X:0123Y:0123 P(X):152/5151/5P(Y):15310251/(10)
E(y2)=2E(X)
E(X)=EXp(X)
0(15)+1(25)+2(15)+3(15)=75
2E(X)=145
E(Y2)=∑y2P(Y)
0(15)+1(310)+4(25)+9(110)
310+1610+910=2810=145
E(Y2)=145
from above equations, we get
E(Y2)=2E(X)
Similar Questions
Knowledge Check
- Question 1 - Select One
The probability distribution of a discrete random variable X is given below
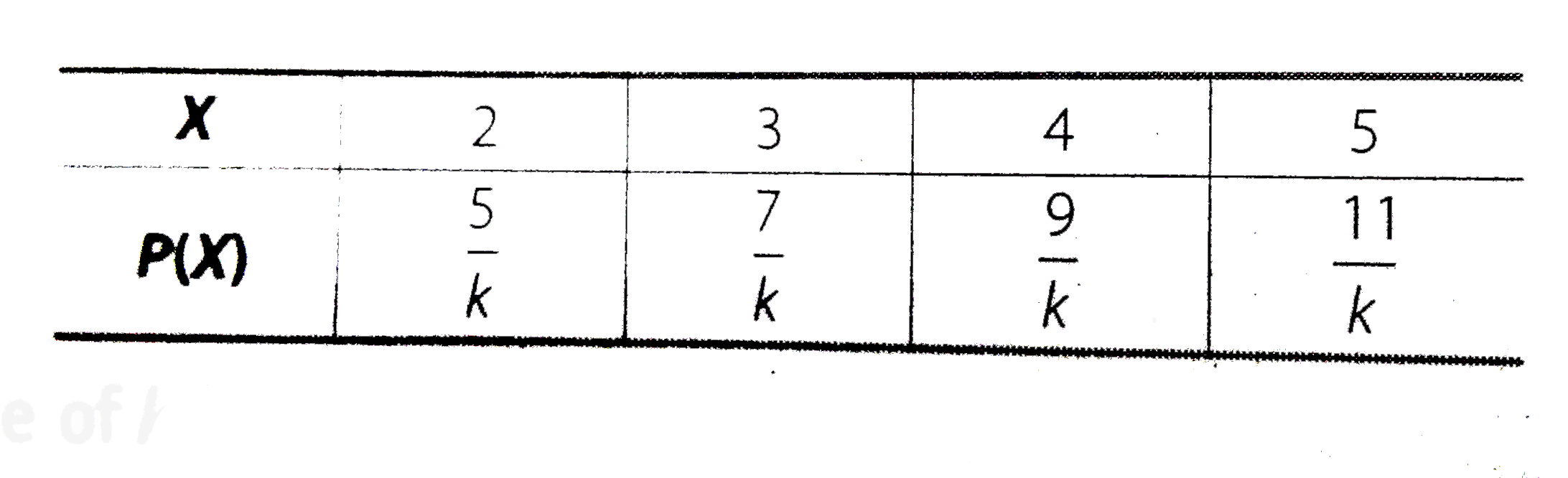
The value of k isA8B16C32D48
The probability distribution of a discrete random variable X is given below: X: 2 3 4 5 P(X):5k 7k 9k 11k The value of E(X) is
View SolutionThe probability distribution of a random variable X is given below: X: 0 1 2 3 P(X):k k2 k4 k8 (i) Determine the value of k (ii) Determine P(X≤2)and P(X>2) (iii) Find P(X≤2)+P(X>2)
View SolutionFind the variance and standard deviation of the random variable X whose probability distribution is given below :
x0123P(X=x)18383818View SolutionWhich of the following distributions of probability of random variable X are the probability distributions? X: 0 1 2 3 P(X):0.3 0.2 0.4 0.1
View SolutionIf mandσ2 are the mean and variance of the random variable X, whose distribution is given by:
X=x:0123P(X=x):1312016View SolutionA random variable X has the following probability distribution: [X=x0123P(X=x)181214k]
View SolutionThe mean and variance of a binomial distribution for a randonil distribution for a random variable are 10and53 respectively. Find P(X≤1).
View Solution

