The quadrilateral formed by angle bisectors of a cyclic quadrilateral is also cyclic.
Video Solution
|
Answer
Step by step video & image solution for The quadrilateral formed by angle bisectors of a cyclic quadrilateral is also cyclic. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - TARGET PUBLICATIONCHAPTER - CIRCLEEXERCISE - Chapter Assessment9videos
CIRCLE
- BOOK - TARGET PUBLICATIONCHAPTER - CIRCLEEXERCISE - Multiple Choice Question25videos
CIRCLE
- BOOK - TARGET PUBLICATIONCHAPTER - CHALLENGING QUESTIONS EXERCISE - Chapter 6: Statistics4videos
CHALLENGING QUESTIONS
- BOOK - TARGET PUBLICATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - CHAPTER ASSESSMENT19videos
CO-ORDINATE GEOMETRY
Similar Questions
Knowledge Check
- Question 1 - Select One
The quadrilateral formed by the bisectors of the angles of a parallelogram is a
 ArectangleBsquareCparallelogramDcyclic quadrilateral
ArectangleBsquareCparallelogramDcyclic quadrilateral - Question 2 - Select One
Which of the following statements is/are correct?
The intersections of the angle bisectors of the quadrilateral are the vertices of the cyclic quadrilateral
If diagonals of a cyclic quadrilateral perpendicularly intersect at point P, then the line through P, perpendicular to any side bisects the opposite side.
The product of the lengths of the two diagonals of a cyclic quadrilateral is equal to the sum of the products of opposite sides.A(i) and (ii)B(ii) and (iii)C(i) and (iii)Dall of these - Question 3 - Select One
The quadrilateral formed by the bisectors of the angles of a parallelogram is a
 ATrapeziumBKiteCRectangleDNone
ATrapeziumBKiteCRectangleDNone
Prove that, any rectangle is a cyclic quadrilateral.
 View Solution
View SolutionProve that, any rectangle is a cyclic quadrilateral.
 View Solution
View SolutionProve that the quadrilateral formed by the bisectors of the angles of a parallelogram is a rectangle.
View SolutionABCD is a cyclic quadrilateral (see Figure). Find the angles of the cyclic quadrilateral.
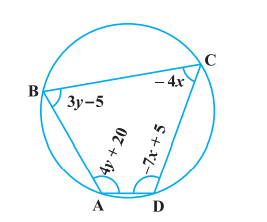 View Solution
View SolutionThe bisectors of the angles formed by producing the opposite sides of a cyclic quadrilateral (provided that they are not parallel) intersect at right angle.
View Solution

