Prove that the quadrilateral formed by the bisectors of the angles of a parallelogram is a rectangle.

Video Solution

Text Solution

Verified by Experts

Given : A parallelogram ABCD in which bisectors of angles A,B, C,D intersect at P,Q,S to from a quadrilaateral PQRS.
To prove : PQRS is a rectangle.
Proof: Since, ABCD is a parallelogram.
Therefore, $A B || D C$
Now, $A B || D C$ and transversal AD interects them at D and A respectively.
Therefore,
$∠ A + ∠ D = 180^{\circ} (∵ sum of consective interior angles is 180^{\circ})$
$\Rightarrow \frac{1}{2} ∠ A + \frac{1}{2} ∠ D = 90^{\circ}$
$\Rightarrow ∠ D A S + ∠ A D S = 90^{\circ} ... (1)$
$(∵ DS and AS are bisectors of ∠ A and ∠ D respectively)$
But , in $Δ D A S$ we have
$∠ D A S + ∠ A S D + ∠ A D S = 180^{\circ} (∵ sum of angle of a triangle is 180^{\circ})$
$\Rightarrow ∠ 90^{\circ} + ∠ A S D = 180^{\circ} [using (1)]$
$\Rightarrow ∠ A S D = 90^{\circ}$
$\Rightarrow ∠ P S R = 90^{\circ} (∵ ∠ S A D and ∠ P S R are vertically opposite angle ∴ ∠ P S R = ∠ A S D)$
Similarly, we can prove that
$∠ S E Q = 90^{\circ}, ∠ R Q P = 90^{\circ} and ∠ S P Q = 90^{\circ}$
Hence, PQRS is a rectangle.

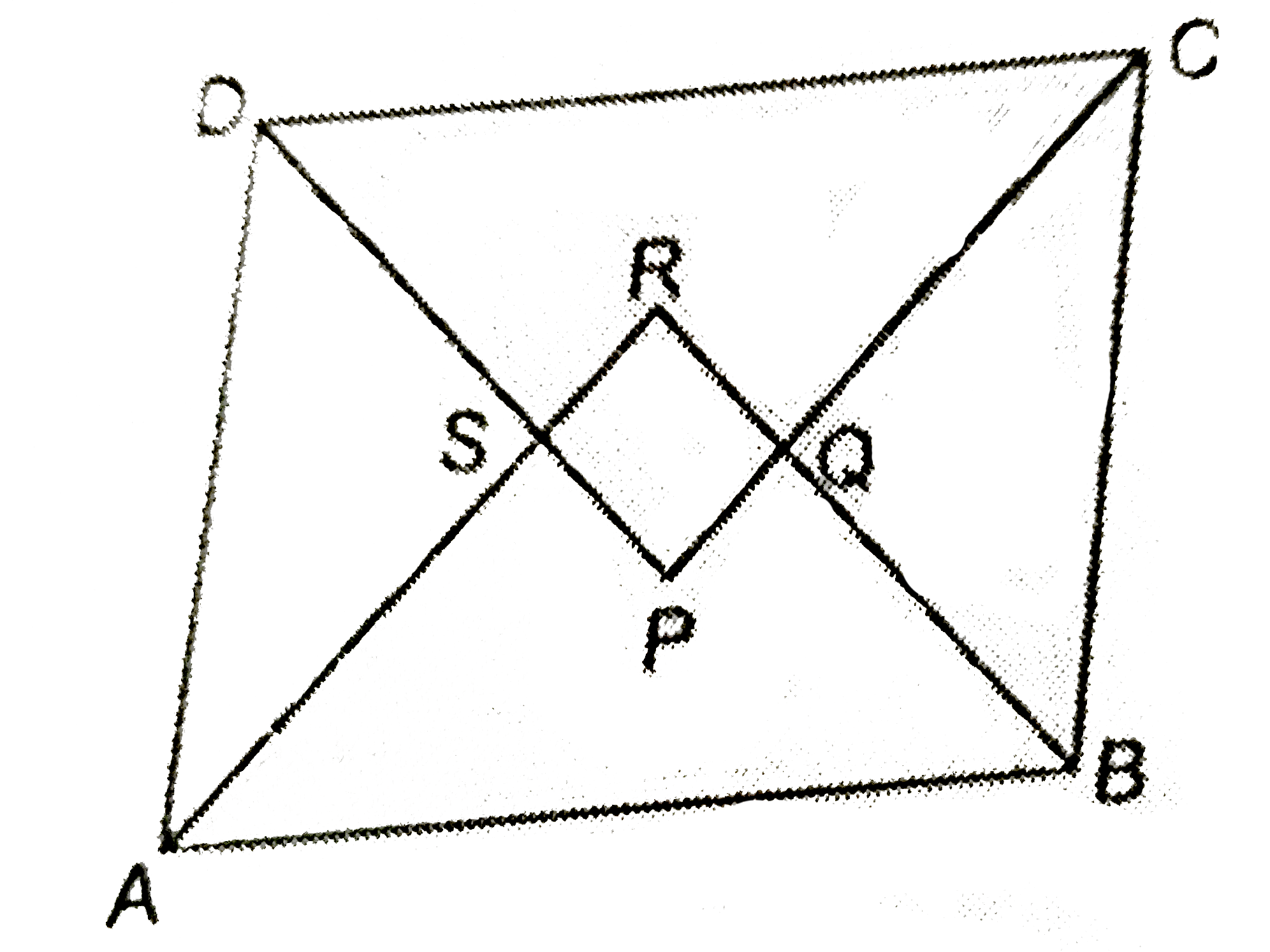
Updated on:21/07/2023

The bisector of the angles of a parallelogram enclose a
Arectangle
Brhombus
Csquare
Dtrapezium
Shape made by the bisectors of angles of a parallelogram is
ARectangle
BSquare
CCircle
DStraight line
The bisector of the angles of a parallelogram enclose a ..................
AParallelogram
Bsquare
CRhombus
DRectangle