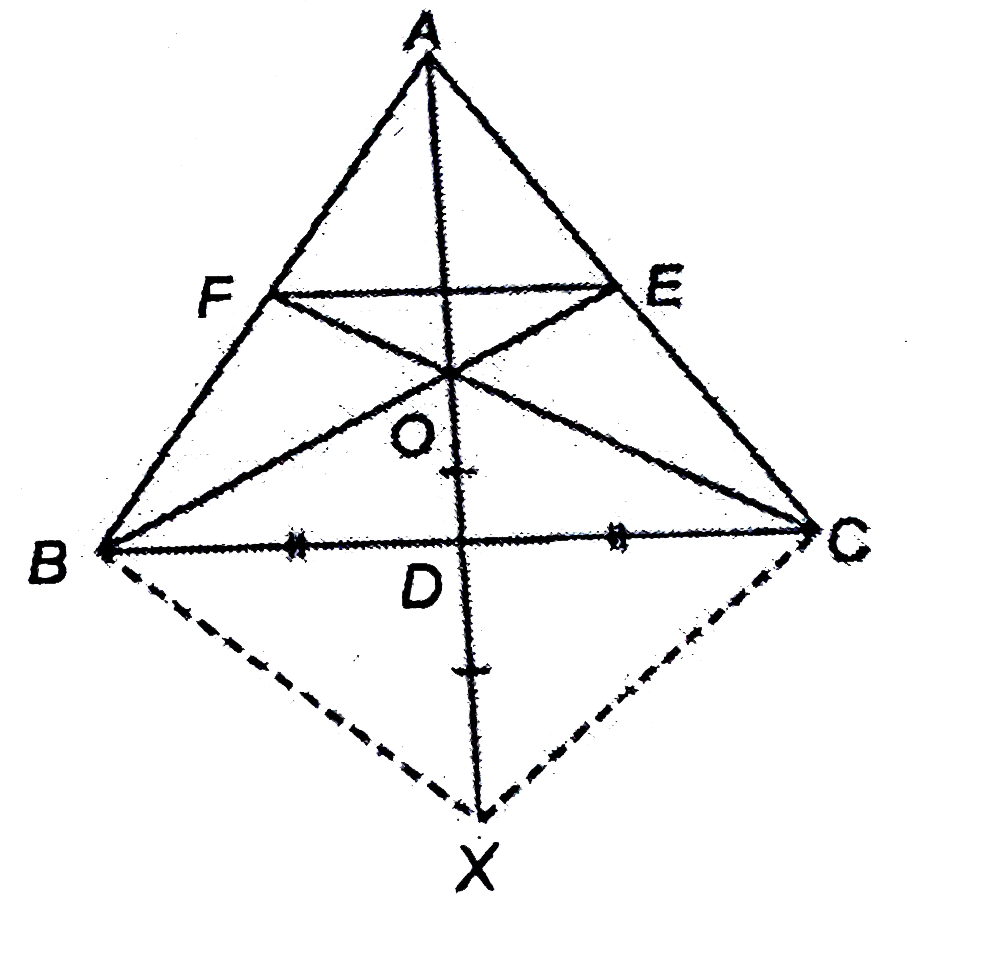
The side BC of a triangle ABC is bisected at D;O is any point in AD.BOandCO produced meet ACandAB in EandF respectively and AD is produced to X so that D is the mid-point of OX. Prove that AO:AX=AF:AB and show that FE∣∣BC.
Join BX and CX.
Since D is the mid -point of BC ( given )
BD= DC …(1)
Also D is the mid-point of OX (given )
OD= DX … (2)
From (1) and (2) we, have
□OBXC is a parallelogram ( diagonals bisect each other)
OC||BX ( opposite sides of a parallelogram)
⇒FC||BX⇒FO||BX
InΔABX,AOOX=AFFB ( by B.P theorem) ...(3)
Also , BO||XC ( opposite sides of a parallelogram)
⇒BE||XC⇒OE||XC
AOOX=AEEC (by B.P theorem) ...(4)
From (3) and (4) , we have
1AFFB=(AE)(EC)
In ΔABC,FE∣∣BC. (converse of B.P theorem) Hence Proved.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar14videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Exercise 6a24videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions4videos
STATISTICS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions5videos
VOLUME AND SURFACE AREA OF SOLIDS
Similar Questions
D is the mid-point of side BC of a triangle ABC.AD is bisected at the point E and BE produced cuts AC at the point X. Prove that BE:EX=3:1.
View SolutionAD is a median of ΔABC . The bisector of ∠ADB and ∠ADC meet AB and AC in E and F respectively. Prove that EF||BC
View SolutionIn Figure, O is the mid point of AB and CD . Prove that (i) AOC≅BOD (ii) AC=BD
View SolutionIn a triangle ABC median AD is produced to X such that AD=DX. Prove that ABXC is a parallelogram.
View SolutionIn a triangle ABC median AD is produced to X such that AD=DX. Prove that ABXC is a parallelogram.
View SolutionIn a triangle ABC median AD is produced to X such that AD=DX. Prove that ABXC is a parallelogram.
View SolutionIn ABC,AD is the median through A and E is the mid-point of AD . BE produced meets AC in F (Figure). Prove that AF=13AC.
View SolutionIn ABC,AD is the median through A and E is the mid-point of AD . BE produced meets AC in F (Figure). Prove that AF=13AC.
View SolutionIn ABC,AD is the median through A and E is the mid-point of AD . BE produced meets AC in F (Figure). Prove that AF=13AC.
View SolutionIn Figure, line segments AB is parallel to another line segment CD.O is the mid-point of AD. Show that: AOB≅ DOC.
View Solution

