The sides AB and AC of ABC are product to P and Q respectively. the bisectors of exterior angles at B and C of ABC meet at O (fig.19) prove that ∠BOC=900−12∠A
∠7+∠3=∠2+∠6 ....(1) (exterior antgle theorem)
Also ∠4∠8=∠2+∠5 ....(2) (exterior angle theorem)
Adding (1) and , we get
∠7+∠3+∠4∠8=∠2+∠6+∠5+∠2
⇒∠3+∠3+∠4∠4=180∘+∠2 (∴BOandCO∠ bisectors and by using angle sum property)
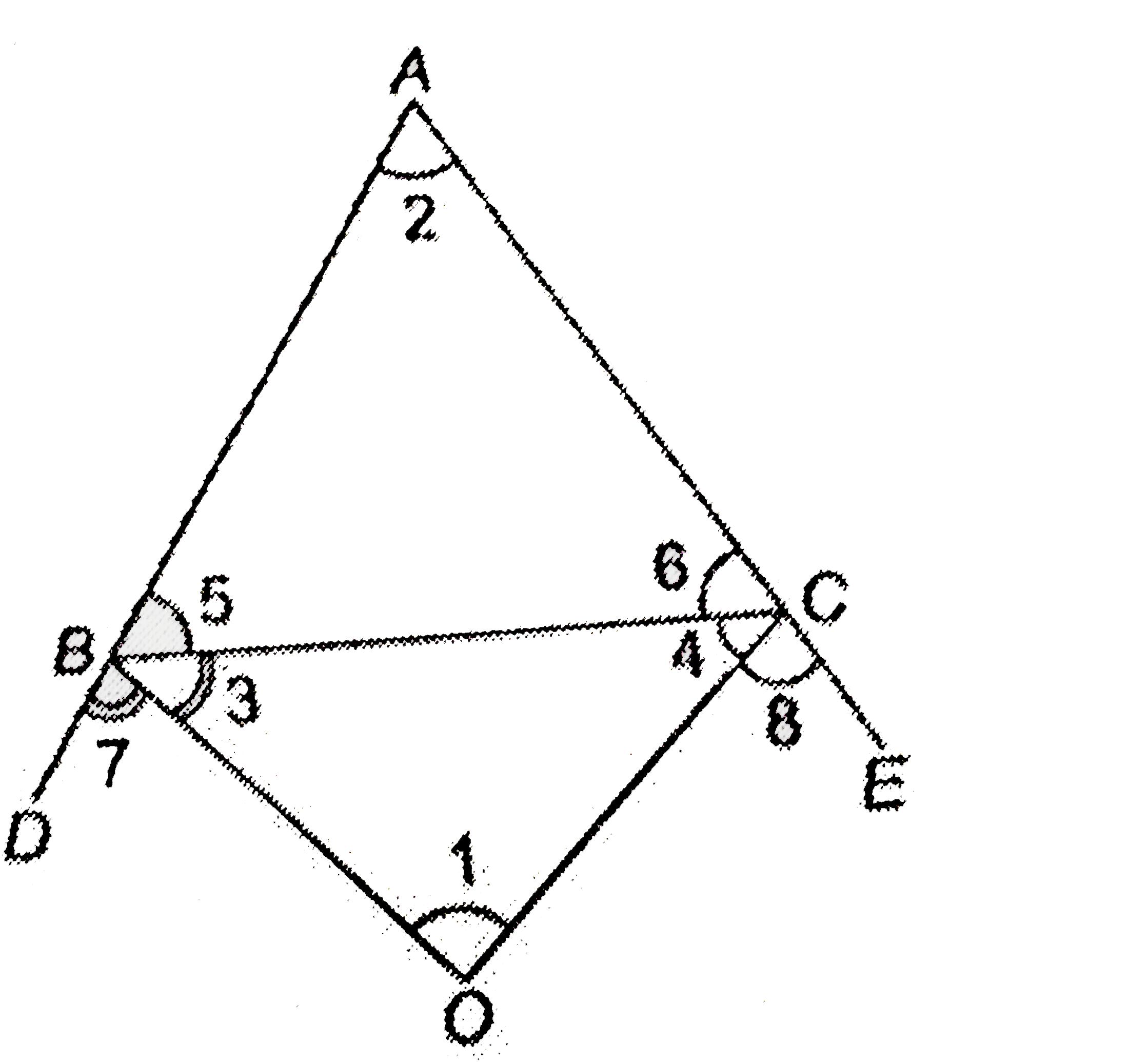
⇒2∠3+2∠4=180∘+∠2
Dividing both sides by
∴∠3+∠4=90∘+12
⇒180∘−∠1=90∘+12∠2 (by angle sum property ∠1+∠3+∠4=180∘)
⇒∠=180∘−90∘−12∠2
⇒∠1=90∘−12∠2
Topper's Solved these Questions
- LINES AND ANGLESBOOK - NAGEEN PRAKASHANCHAPTER - LINES AND ANGLESEXERCISE - Problems From NCERT/exemplar
6 Videos
- LINES AND ANGLESBOOK - NAGEEN PRAKASHANCHAPTER - LINES AND ANGLESEXERCISE - Exercise 6 A
19 Videos
- LINEAR EQUATIONS IN TWO VARIABLESBOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Revision Exercise
12 Videos
- NUMBER SYSTEMBOOK - NAGEEN PRAKASHANCHAPTER - NUMBER SYSTEMEXERCISE - Revision Exercise (short Answer Questions)
10 Videos
Similar Questions
The sides AB and AC of ABC are product to P and Q respectively. the bisectors of exterior angles at B and C of ABC meet at O (fig.19) prove that ∠BOC=900−12∠A
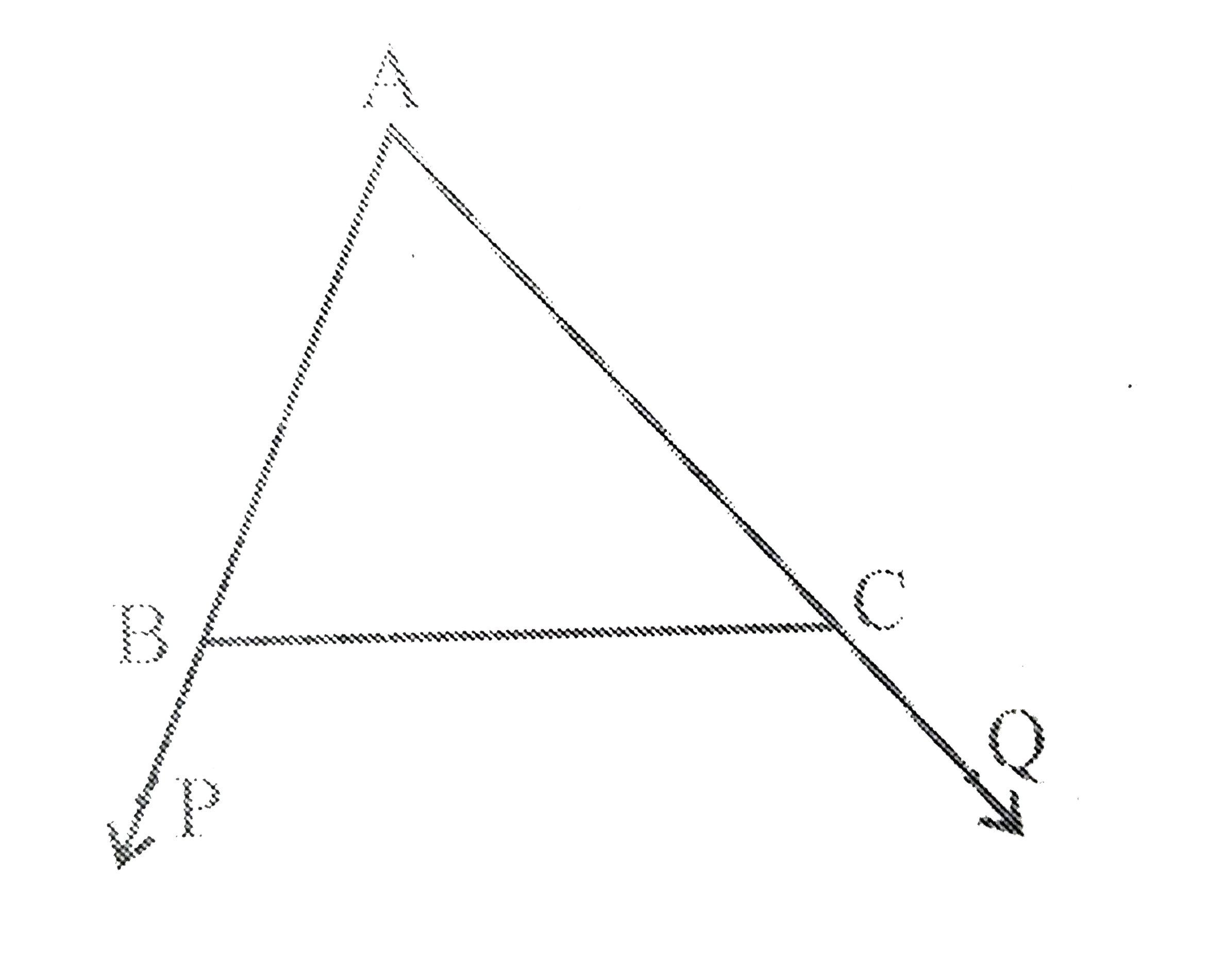
View SolutionThe sides AB and AC of a ABC are produced to P and Q respectively. If the bisectors of ∠PBC AND ∠QCB intersect at O, then ∠BOC=900−12∠A GIVEN : A ABC in which sides AB and AC are produced to P and Q respectively. The bisectors of ∠PBC and ∠QCB intersect at O.
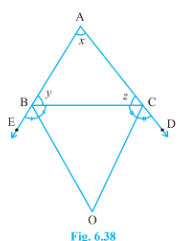
View SolutionIn Fig. 6.38, the sides AB and AC of ABC are produced to points E and D respectively. If bisectors BO and CO of CBE and BCD respectively meet at point O, then prove that ∠BOC=90∘−12∠BAC..
View SolutionABC is a triangle. The bisector of the exterior angle at B and the bisector of ∠C intersect each other at D. Prove that ∠D=12∠A
View SolutionThe bisectors of exterior angles at B and C of ∠ABC meet at O. If ∠A=x0, then ∠BOC= 900+x02 (b) 900−x02 1800+x02 (d) 1800−x02
View SolutionIn Fig. 7.48, sides ABandAC of ΔABC are extended to points PandQ respectively. Also, ∠PBC <∠QCB. Show that AC > AB.
View SolutionAn exterior angle of a triangle is 1100 , and one of the interior opposite angles is 300. Find the other two angles of the triangle.
View SolutionThe diagonals of a rectangle ABCD meet at O . If ∠BOC=44∘, find ∠OAD
View SolutionIn figure AB=AC and CP∣∣BA and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA (ii) ABCP is a parallelogram
View SolutionABC is a triangle in which ∠A=720, the internal bisectors of angles B and C meet in O. Find the magnitude of ∠BOC
View Solution
