The sum of squares of the deviation of the values of the variable is_____ when taken about their arithmetic mean
The correct Answer is:N/a
To solve the question, we need to understand the concept of the sum of squares of deviations from the arithmetic mean.
1. Understanding Deviation:
The deviation of a value xi from the mean ¯x is given by xi−¯x.
2. Sum of Squares of Deviations:
The sum of squares of deviations from the mean is calculated as:
S=∑(xi−¯x)2
where S is the sum of squares of deviations, and xi represents each value in the dataset.
3. Properties of the Mean:
One important property of the arithmetic mean is that it minimizes the sum of the squared deviations. This means that when we calculate the sum of squares of deviations about the mean, it will yield the smallest possible value compared to any other point.
4. Conclusion:
Therefore, the sum of squares of the deviations of the values of the variable when taken about their arithmetic mean is minimum.
Final Answer:
The sum of squares of the deviation of the values of the variable is minimum when taken about their arithmetic mean.
The deviation of a value xi from the mean ¯x is given by xi−¯x.
The sum of squares of deviations from the mean is calculated as:
S=∑(xi−¯x)2
where S is the sum of squares of deviations, and xi represents each value in the dataset.
One important property of the arithmetic mean is that it minimizes the sum of the squared deviations. This means that when we calculate the sum of squares of deviations about the mean, it will yield the smallest possible value compared to any other point.
Therefore, the sum of squares of the deviations of the values of the variable when taken about their arithmetic mean is minimum.
The sum of squares of the deviation of the values of the variable is minimum when taken about their arithmetic mean.
Topper's Solved these Questions
Similar Questions
If the mean of 10 observation is 50 and the sum of the square of the deviations of observation from the mean is 250, then the coefficient of variation of these observation is
View SolutionStatement 1: Sum of squares of deviations is minimum when it is taken about their mean.
Statement 2: Quadratic expression ax2+bx+c takes its minimum value at x=4ac−b24a
View SolutionThe standard deviation is ….. To the mean deviation taken from the arithmetic mean
View SolutionFind the mean deviation about the mean of the distributon .
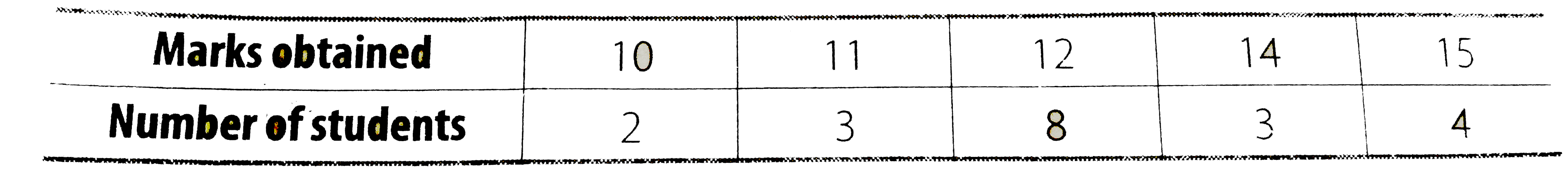
View SolutionFind the mean deviation about the mean of the distributon .
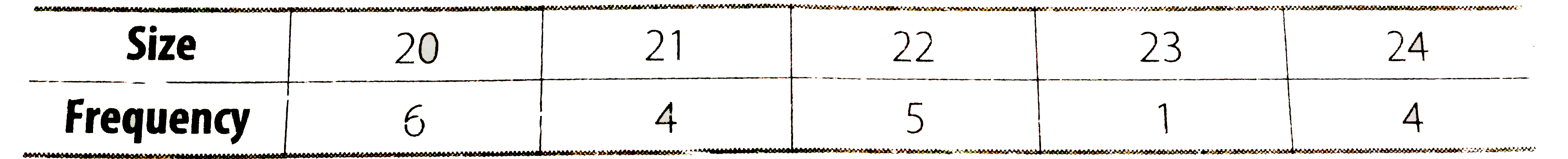
View SolutionFind the mean deviation about the mean for the data is Question:
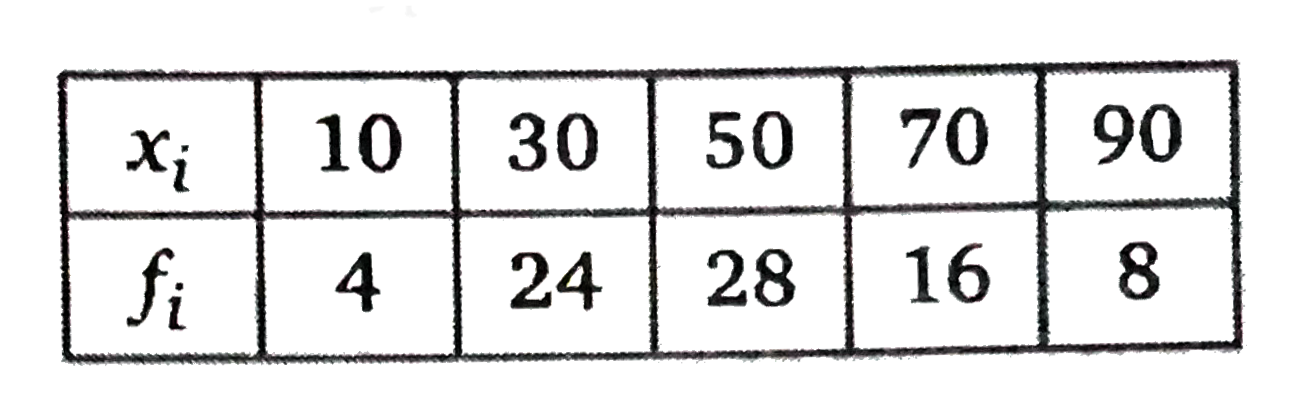
View SolutionThe sum of the squares of deviation of 10 observations from their mean 50 is 250,then coefficient of variation is
View SolutionFind the sum of the deviations of the variate values 3,4,6,8, 14 from their mean.
View SolutionIf the sum of the squares of deviations for 10 observations taken from their means is 2.5, then write the value of standard deviation.
View SolutionFind the mean deviation about the mean for the data in Question:

View Solution
