Through a fixed point (h,k), secant are drawn to the circle . Show that the locus of the midpoints of the secants by the circle is .
From the diagram,
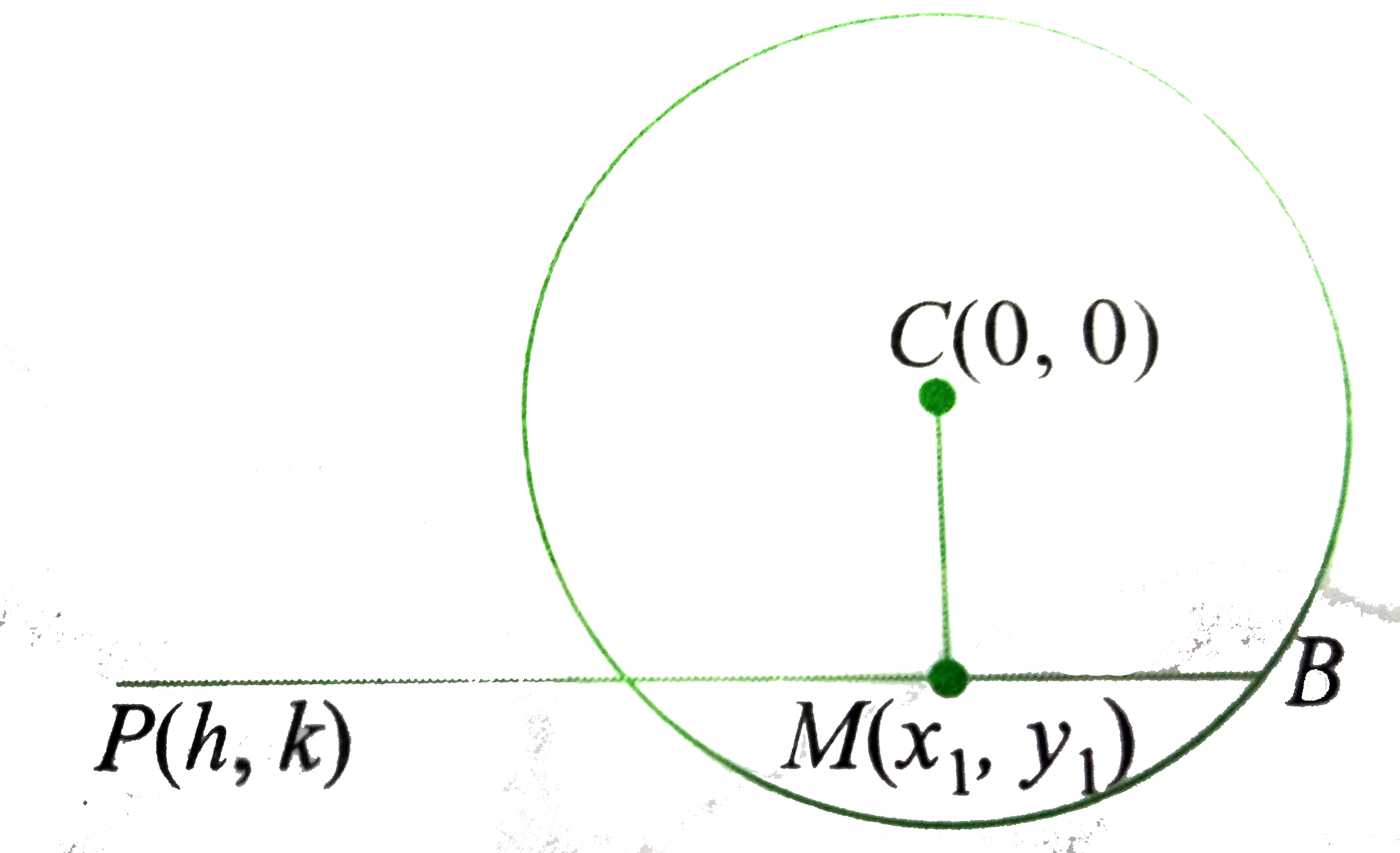
So, the locus of M is
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.4
14 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.5
12 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.2
5 Videos
- BINOMIAL THEORMBOOK - CENGAGECHAPTER - BINOMIAL THEORMEXERCISE - Question Bank
31 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
Similar Questions
Through a fixed point (h, k) secants are drawn to the circle x2+y2=r2. Then the locus of the mid-points of the secants by the circle is
View SolutionFrom the origin, chords are drawn to the circle (x−1)2+y2=1. The equation of the locus of the mid-points of these chords is circle with radius
View Solution
Knowledge Check
Through a fixed point (h, k) secants are drawn to the circle x2+y2=a2 . The locus of the mid points of the secants intercepted by the given circle is
A2(x2+y2)=hx+kyBx2+y2=hx+kyCx2+y2+hx+ky=0Dx2+y2−hx+ky+13=0If the line hx + ky = 1 touches x2+y2=a2, then the locus of the point (h, k) is a circle of radius
AaB1/aC√aD1/√aSTATEMENT-1 : The agnle between the tangents drawn from the point (6, 8) to the circle x2+y2=50 is 90∘.
and
STATEMENT-2 : The locus of point of intersection of perpendicular tangents to the circle x2+y2=r2 is x2+y2=2r2.AStatement-1 is true, statement-2 is true, Statement -2 is a correct explanation for Statement -1BStatement -1 is true, Statement-2 is true , Statement-2 is NOT a correct explanation for statement-1CStatement-1 is true, Statement-2 is FalseDStatement-1 is False, Statement-2 is true
The midpoint of the chord y=x−1 of the circle x2+y2−2x+2y−2=0 is
View SolutionFind the locus of the midpoint of the chords of circle x2+y2=a2 having fixed length l.
View SolutionThere are two perpendicular lines, one touches to the circle x2+y2=r21 and other touches to the circle x2+y2=r22 if the locus of the point of intersection of these tangents is x2+y2=9, then the value of r21+r22 is.
View SolutionIf a circle passes through the point (a,b) and cuts the circle x2+y2=k2 orthogonally, then the equation of the locus of its center is
View SolutionIf a circle passes through the point (a,b) and cuts the circle x2+y2=4 orthogonally, then the locus of its centre is
View Solution
