To find out the concentrations of in the air (in parts per million, i.e., ppm), the data was collected for 30 localities in a certain city and is presented below: Concentration of (in ppm) Frequency 0.00 - 0.04 4 0.04 - 0.08 9 0.08 - 0.12 9 0.12 - 0.16 2 0.16 - 0.20 4 0.20 - 0.24 2 Find the mean concentration of in the air.
To find the mean concentration of SO2 in the air based on the given frequency distribution, we will follow these steps:
Step 1: Identify the Classes and Frequencies
We have the following classes and their corresponding frequencies:
| Concentration (ppm) | Frequency (f) |
|----------------------|---------------|
| 0.00 - 0.04 | 4 |
| 0.04 - 0.08 | 9 |
| 0.08 - 0.12 | 9 |
| 0.12 - 0.16 | 2 |
| 0.16 - 0.20 | 4 |
| 0.20 - 0.24 | 2 |
Step 2: Calculate the Midpoint (xi) for Each Class
The midpoint for each class can be calculated using the formula:
\( x_i = \frac{\text{Lower limit} + \text{Upper limit}}{2} \)
Calculating the midpoints:
- For 0.00 - 0.04:
\( x_1 = \frac{0.00 + 0.04}{2} = 0.02 \)
- For 0.04 - 0.08:
\( x_2 = \frac{0.04 + 0.08}{2} = 0.06 \)
- For 0.08 - 0.12:
\( x_3 = \frac{0.08 + 0.12}{2} = 0.10 \)
- For 0.12 - 0.16:
\( x_4 = \frac{0.12 + 0.16}{2} = 0.14 \)
- For 0.16 - 0.20:
\( x_5 = \frac{0.16 + 0.20}{2} = 0.18 \)
- For 0.20 - 0.24:
\( x_6 = \frac{0.20 + 0.24}{2} = 0.22 \)
Step 3: Create a New Table with Midpoints and Frequencies
Now, we can create a new table that includes the midpoints and their corresponding frequencies:
| Concentration (ppm) | Frequency (f) | Midpoint (xi) |
|----------------------|---------------|---------------|
| 0.00 - 0.04 | 4 | 0.02 |
| 0.04 - 0.08 | 9 | 0.06 |
| 0.08 - 0.12 | 9 | 0.10 |
| 0.12 - 0.16 | 2 | 0.14 |
| 0.16 - 0.20 | 4 | 0.18 |
| 0.20 - 0.24 | 2 | 0.22 |
Step 4: Calculate \( f_i \cdot x_i \)
Now, we will calculate the product of frequency and midpoint for each class:
\(
f_i \cdot x_i
\)
| Frequency (f) | Midpoint (xi) | \( f_i \cdot x_i \) |
|---------------|---------------|---------------------|
| 4 | 0.02 | 0.08 |
| 9 | 0.06 | 0.54 |
| 9 | 0.10 | 0.90 |
| 2 | 0.14 | 0.28 |
| 4 | 0.18 | 0.72 |
| 2 | 0.22 | 0.44 |
Step 5: Calculate \( \Sigma f_i \) and \( \Sigma f_i \cdot x_i \)
Now, we will sum up the frequencies and the products:
\(
\Sigma f_i = 4 + 9 + 9 + 2 + 4 + 2 = 30
\)
\(
\Sigma (f_i \cdot x_i) = 0.08 + 0.54 + 0.90 + 0.28 + 0.72 + 0.44 = 2.96
\)
Step 6: Calculate the Mean Concentration
The mean concentration (\( \bar{x} \)) can be calculated using the formula:
\(
\bar{x} = \frac{\Sigma (f_i \cdot x_i)}{\Sigma f_i}
\)
Substituting the values:
\(
\bar{x} = \frac{2.96}{30} \approx 0.0987
\)
Conclusion
The mean concentration of SO2 in the air is approximately 0.0987 ppm.
---
We have the following classes and their corresponding frequencies:
|----------------------|---------------|
| 0.00 - 0.04 | 4 |
| 0.04 - 0.08 | 9 |
| 0.08 - 0.12 | 9 |
| 0.12 - 0.16 | 2 |
| 0.16 - 0.20 | 4 |
| 0.20 - 0.24 | 2 |
The midpoint for each class can be calculated using the formula:
\( x_i = \frac{\text{Lower limit} + \text{Upper limit}}{2} \)
\( x_1 = \frac{0.00 + 0.04}{2} = 0.02 \)
- For 0.04 - 0.08:
\( x_2 = \frac{0.04 + 0.08}{2} = 0.06 \)
- For 0.08 - 0.12:
\( x_3 = \frac{0.08 + 0.12}{2} = 0.10 \)
- For 0.12 - 0.16:
\( x_4 = \frac{0.12 + 0.16}{2} = 0.14 \)
- For 0.16 - 0.20:
\( x_5 = \frac{0.16 + 0.20}{2} = 0.18 \)
- For 0.20 - 0.24:
\( x_6 = \frac{0.20 + 0.24}{2} = 0.22 \)
Now, we can create a new table that includes the midpoints and their corresponding frequencies:
|----------------------|---------------|---------------|
| 0.00 - 0.04 | 4 | 0.02 |
| 0.04 - 0.08 | 9 | 0.06 |
| 0.08 - 0.12 | 9 | 0.10 |
| 0.12 - 0.16 | 2 | 0.14 |
| 0.16 - 0.20 | 4 | 0.18 |
| 0.20 - 0.24 | 2 | 0.22 |
Now, we will calculate the product of frequency and midpoint for each class:
f_i \cdot x_i
\)
|---------------|---------------|---------------------|
| 4 | 0.02 | 0.08 |
| 9 | 0.06 | 0.54 |
| 9 | 0.10 | 0.90 |
| 2 | 0.14 | 0.28 |
| 4 | 0.18 | 0.72 |
| 2 | 0.22 | 0.44 |
Now, we will sum up the frequencies and the products:
\Sigma f_i = 4 + 9 + 9 + 2 + 4 + 2 = 30
\)
\Sigma (f_i \cdot x_i) = 0.08 + 0.54 + 0.90 + 0.28 + 0.72 + 0.44 = 2.96
\)
The mean concentration (\( \bar{x} \)) can be calculated using the formula:
\bar{x} = \frac{\Sigma (f_i \cdot x_i)}{\Sigma f_i}
\)
\bar{x} = \frac{2.96}{30} \approx 0.0987
\)
The mean concentration of SO2 in the air is approximately 0.0987 ppm.
Topper's Solved these Questions
Similar Questions
To find out the concentration of SO2in the air (in parts per million, i.e., ppm), the data was collected for 30 localities in a certain city and is presented below:Find the mean concentration of SO2in the air.
View SolutionTo find out the concentration of SO2 in the air (in parts per million, i.e., ppm), the data was collected for 30 localities in a certain city Find the mean concentration of SO2 in the air.
 View Solution
View SolutionGiven below is the frequency distribution table regarding the concentration of sulphur dioxide in the air in parts per million of a certain city for 30 days.
Concentration0.00−0.040.04−0.080.08−0.120.12−0.160.16−0.200.20−0.24No. of days:489243
Find the probability of concentration of sulphur dioxide in the interval 0.12-0.16 on any of these days.View SolutionA study was conducted to find out the concentration of sulphur dioxide in the air in parts per million (ppm) of a certain city. The data obtained for 30 days is as follows: 0.03 0.08 0.08 0.09 0.04 0.170.16 0.05 0.02 0.06 0.18 0.200.11 0.08 0.12 0.13 0.22 0.070.08 0.01 0.10 0.06 0.09 0.180.11 0.07 0.05 0.07 0.01 0.04 (i) Make a grouped frequency distribution table for this data with class intervals as 0.00 - 0.04, 0.04 - 0.08, and so on.(ii) For how many days, was the concentration of sulphur dioxide more than 0.11 parts per million?
View SolutionA study was conducted to find out the concentration of sulphur dioxide in the air in parts per million (ppm) of a certain city. The data obtained for 30 days is a follows :
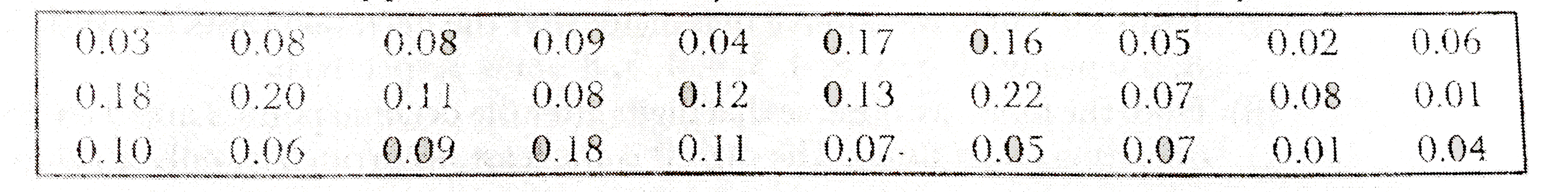
(i) Make a grouped frequency distribution table for this data with class-intervals as 0.00−0.04,0.04−0.08 and so on.
(ii) For how many day's was the concentration of sulphar dioxide more than 0.11 parts per million (ppm)?View SolutionA study was conduced to find out the concentration of sulphur dioxide in the air in parts per million (ppm) of a certain city. The data obtained for 30 days is as follow:
0.03 0.08 0.08 0.09 0.04 0.170.16 0.05 0.02 0.06 0.18 0.200.11 0.08 0.12 0.13 0.22 0.070.08 0.01 0.10 0.06 0.09 0.180.11 0.07 0.05 0.07 0.01 0.04
You were asked to prepare a frequency distribution table, regarding the concentration of sulphur dioxide in the air in parts per million of a certain city for 30 days. Using this table, find the probability of the concentration of sulphur dioxide in the interval 0.12-0.16 on any of these days.View SolutionEvaluate:
35.9−2.1×0.9+17.2÷0.4−0.4View Solution0.4x+0.3y=1.70.7x−0.2y=0.8
View SolutionEvaluate:
32.5+12.43×0.2+16.6÷0.02−4.07View SolutionA 500 g tooth paste sample has 0.2 g fluride concentration. What is the concentration of F in terms of ppm level?
View Solution

