To warn ships for underwater rocks, a lighthouse spreads a red coloured light over a sector of angle 80∘to a distance of 16.5 km. Find the area of the sea over which the ships are warned.
The correct Answer is:190.10 sq. km
To find the area of the sea over which the ships are warned by the lighthouse, we can follow these steps:
1. Identify the parameters:
- The angle of the sector (θ) = 80 degrees
- The radius (r) = 16.5 km
2. Formula for the area of a sector:
The area A of a sector of a circle can be calculated using the formula:
A=θ360∘×πr2
3. Substitute the values into the formula:
- Substitute θ=80∘ and r=16.5 km into the formula:
A=80360×π×(16.5)2
4. Simplify the fraction:
- Simplifying 80360:
80360=29
5. Calculate (16.5)2:
- Calculate 16.5×16.5:
(16.5)2=272.25
6. Substitute π:
- Use π≈227:
A=29×227×272.25
7. Calculate the area:
- First, calculate 2×229×7:
4463
- Now multiply by 272.25:
A=44×272.2563
8. Perform the multiplication:
- Calculate 44×272.25:
44×272.25=11979
9. Divide by 63:
- Now divide 11979 by 63:
A≈190.14 km2
10. Final Answer:
The area of the sea over which the ships are warned is approximately 190.14 km2.
- The angle of the sector (θ) = 80 degrees
- The radius (r) = 16.5 km
The area A of a sector of a circle can be calculated using the formula:
A=θ360∘×πr2
- Substitute θ=80∘ and r=16.5 km into the formula:
A=80360×π×(16.5)2
- Simplifying 80360:
80360=29
- Calculate 16.5×16.5:
(16.5)2=272.25
- Use π≈227:
A=29×227×272.25
- First, calculate 2×229×7:
4463
- Now multiply by 272.25:
A=44×272.2563
- Calculate 44×272.25:
44×272.25=11979
- Now divide 11979 by 63:
A≈190.14 km2
The area of the sea over which the ships are warned is approximately 190.14 km2.
Similar Questions
To warn ships for underwater rocks, a lighthouse spreads a red coloured light over a sector of angle 80oto a distance of 16.5 km. Find the area of the sea over which the ships are warned.
View SolutionTo warn ships for underwater rocks, a light house throws a red coloured light over a sector of 80o angle to a distance of 16.5 km. Find the area of the sea over which the ships are warned.
View Solution
Knowledge Check
A cargo ship is 4.2 miles from a lighthouse, and a fishing boat is 5.0 miles from the lighthouse, as shown below. The angle between the straight lines from the lighthouse to the 3 vessels is 5∘. The approximate distance in miles, from the cargo ship to the fishing boat is given by which of the following expressions?
(Note: The law of cosines states that for any triangle with vertices A, B and C adn the sides opposite those vertices with length a, b, and c, respectively. c2=a2+b2−2abcosC).
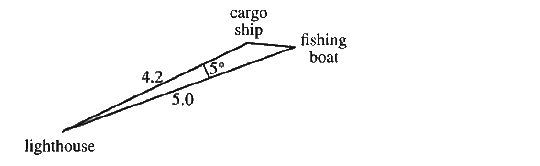 A√(5.0)2−(4.2)2B√(4.2)2+(5.0)2−2⋅4.2⋅5.0cos5∘C√(4.2)2+(5.0)2+2⋅4.2⋅5.0cos5∘D√(4.2)2+(5.0)2−2⋅4.2⋅5.0cos85∘
A√(5.0)2−(4.2)2B√(4.2)2+(5.0)2−2⋅4.2⋅5.0cos5∘C√(4.2)2+(5.0)2+2⋅4.2⋅5.0cos5∘D√(4.2)2+(5.0)2−2⋅4.2⋅5.0cos85∘
Two ships are there in the sea on either side of a light house in such a way that the ships and the light house are in the same straight line. The angles of depression of two ships are observed from the top of the light house are 60∘ and 45∘ respectively. If the height of the light house is 200 m, find the distance between the two ships. (Use √3=1.73)
View SolutionA driver having a definite reaction time is capable of stopping his car over a distance of 30 m on seeing a red traffic signal, when the speed of the car is 72 km/hr andover a distance of 10 m when the speed is 36 km/hr. Find the distance over which he can stop the car if it were running at a speed of 54 km/hr. Assume that his reaction time and the deceleration of the car remains same in all the three cases.
View SolutionAs observed from the top of a 80 m tall lighthouse, the angle of depression of two ships, on the same side of the light house in horizontal line with its base, are 30∘and40∘ respectively . Find the distance between the two ships. Given your answer correct to the nearest metre
View SolutionA ship is moving at a constant speed of 10km/hr in the direction of the unit vector ˆi. Initially. Its position vector, relative to a fixed origin is 10(−ˆi+ˆj) where ˆi & ˆj are perpendicular vectors of length 1 km. Find its position vector relative to the origin at time t hours later. A second ship is moving with constant speed ukm/hr parallele to the vector ˆi+2ˆj and is initially at the origin
(i) If u=10√5km/h. Find the minimum distance between the ships and the corresponding value of t
(ii) Find the value of u for which the shipd are on a collision course and determine the value of t at which the collision would occur if no avoiding action were taken.
View SolutionA field is in the form of a rectangle of length 18m and width 15m. A pit, 7.5m long, 6m broad and 0.8m deep, is dug in a corner of the field and the earth taken out is spread over the remaining area of the field. Find cut the extent to which the level of the field has been raised.
View SolutionAn agricultural field is in the form of a rectangle of length 20m and width 14m. A pit 6mlong,3mwideand2.5mdeep is dug in a corner of the field and the earth taken out of the pit is spread uniformly over the remaining area of the field. Find the extent to which the level of the field has been raised.
View SolutionAn agricultural field is in the form of a rectangle of length 20m and width 14 m. A pit 6m long, 3m wide and 2.5m deep is dug in a corner of the field and the earth taken out of the pit is spread uniformly over the remaining area of the field. Find the extent to which the level of the field has been raised.
View Solution
