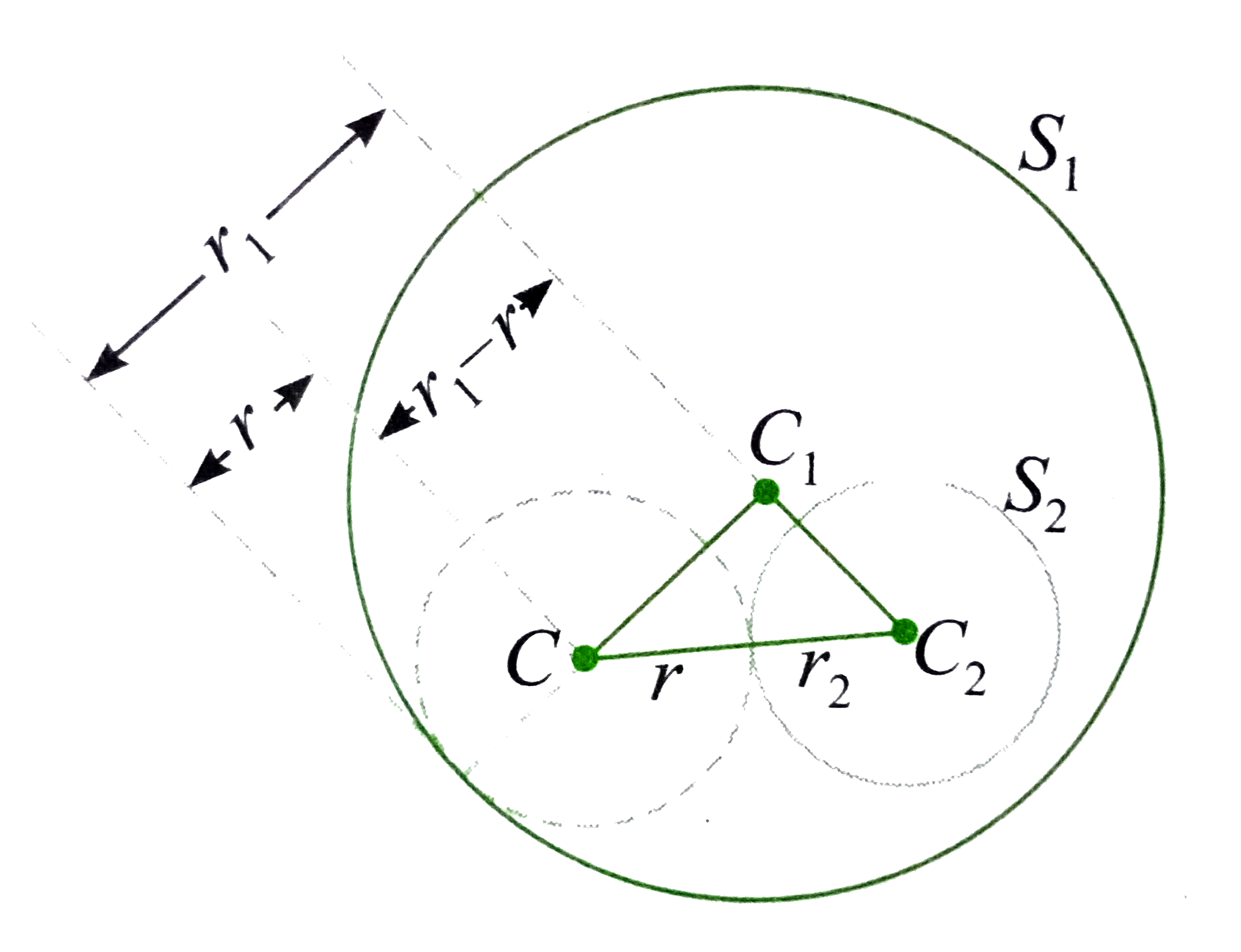
Two circles are given such that one is completely lying inside the other without touching. Prove that the locus of the center of variable circle which touches the smaller circle from outside and the bigger circle from inside is an ellipse.
Let the circle S2 having centre at C2 and redius r2 lie completely insid the circle S1 having centre at C1 and radius r1
Variable circle S touches S1 intrnally and S2 externally. Centre C of circle S is variable point and its radius 'r' is also variable. From the figure CC2=r+r2andCC1=r1−r So, CC1+CC2=r1+r2 (constant) Thus, locus of C is ellips whose foci are at C1andC2
Similar Questions
The locus of the centre of the circles which touches both the axes is given by
View SolutionProve that the locus of the center of the circle which touches the given circle externally and the given line is a parabola.
View SolutionThe locus of the centre of a circle which touches two given circles externally is a
View SolutionTwo circles are given such that they neither intersect nor touch. Then identify the locus of the center of variable circle which touches both the circles externally.
View SolutionThe locus of the centre of a circle the touches the given circle externally is a _______
View SolutionProve that the locus of centre of the circle which toches two given disjoint circles externally is hyperbola.
View SolutionThe locus of the center of a circle which touches the circles |z−z1|=a,|z−z2=b| externally will be
View SolutionFind the locus of centres of circles which touch two intersecting lines.
View SolutionProve that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.
View SolutionThe locus of centre of the circle touching x-axis nad the line y=x is
View Solution

