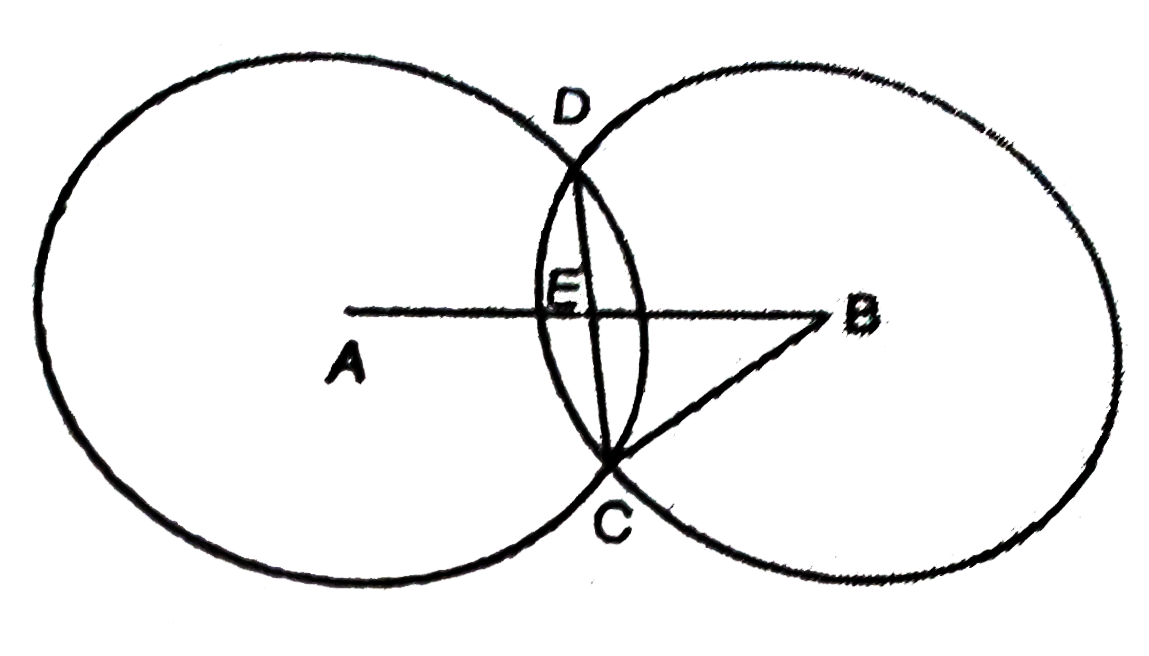
Two circles with centres $A and B$ of radii $3 c m and 4 c m$ respectively intersect at two points $C and D$ such that $A C and B C$ are tangents to the two circles. Find the length of the common chord $C D$ .

Video Solution

Text Solution

Verified by Experts

$∠ O P O^{'} = 90^{\circ}$ [Tangent at a point on the circle Is perpendicular to the radius through point of contact]

Therefore, $O P O$ is a right-angled triangle at $P$

Using Pythagoras, In triangle $O P O',$

${(O O^{'})}^{2} = {(O^{'} P)}^{2} + {(O P)}^{2}$

${(O O^{'})}^{2} = {(4)}^{2} + {(3)}^{2}$

${(O O^{'})}^{2} = 25$

$O O^{'} = 5 c m$

Assume $O N = x c m and N O^{'} = 5 - x c m$

In right angled triangle $O N P$

${(O N)}^{2} + {(P N)}^{2} = {(O P)}^{2}$

$x^{2} + P N^{2} = 3^{2}$

$P N^{2} = 9 - x^{2}$ …….(1)

In right angled triangle $O' N P$

${(O^{'} N)}^{2} + {(P N)}^{2} = {(O^{'} P)}^{2}$

${(5 - x)}^{2} + {(P N)}^{2} = {(4)}^{2}$ }

$25 - 10 x + x^{2} + {(P N)}^{2} = 16$

${(P N)}^{2} = - x^{2} + 10 x - 9$ ………(2)

From equation (1) and (2)

$9 - x^{2} = - x^{2} + 10 x - 9$

$10 x = 18$

$x = 1.8$

From equation (1)

$P N^{2} = 9 - {1.8}^{2}$

$= 9 - 3.24 = 5.76$

$P N = 2.4 c m$

$P Q = 2 P N = 2 (2 .4) = 4.8 c m$

Updated on:21/07/2023