Two flagstaffs stand on a horizontal plane. A and B are two points on the line joining their feet and between them. The angles of elevation of the tops of the flagstatts as seen from A are 30∘ and 60∘ and as seen from B are 60∘and45^(@). If AB=30 , then the distance between the flagstaffs in motres is:
30+15√3
30−15√3
60+15√3
60−15√3.
The correct Answer is:C
Topper's Solved these Questions
- HEIGHTS AND DISTANCES BOOK - MODERN PUBLICATIONCHAPTER - HEIGHTS AND DISTANCES EXERCISE - LATEST QUESTIONS FROM AIEEE/JEE EXAMINATION
2 Videos
- HEIGHTS AND DISTANCES BOOK - MODERN PUBLICATIONCHAPTER - HEIGHTS AND DISTANCES EXERCISE - QUESTIONS FROM KARNATAKA CET & COMED
1 Videos
- FUNCTIONSBOOK - MODERN PUBLICATIONCHAPTER - FUNCTIONSEXERCISE - QUESTION FROM KARNATAKA CET & COMED
14 Videos
- HYPERBOLA BOOK - MODERN PUBLICATIONCHAPTER - HYPERBOLA EXERCISE - Recent Competitive Questions
5 Videos
Similar Questions
Two hagstaffs stand on a horizontal plane. A and B are two points on the line joining their feet and between them. The angles of elevation of the tops of the flagstaffs as seen from A are 30∘ and60∘ and as seen from B are 60∘and 45∘. If AB is 30 m, then the distance between the flagstaffs is
View SolutionTwo towers stand on a horizontal plane. P and Q where PQ = 30 m, are two points on the line joining their feet. As seen from P the angle of elevation of the tops of the towers are 30 and 60 but as seen from Q are 60 and 45. The distance between the towers is equal to
View Solution
Knowledge Check
Two flagstaffs stand on a horizontal plane. A and B are two points on the line joining their feet and between them. The angle of elevation of the tops of the flagstaffs as seen from A are 30∘and60∘ and as seen from B are 60∘and45∘. If AB is 30 m, the distance between the flagstaffs in metre is
A30+15√3B45+15√3C60−15√3D60+15√3Two hagstaffs stand on a horizontal plane. A and B are two points on the line joining their feet and between them. The angles of elevation of the tops of the flagstaffs as seen from A are 30∘ and60∘ and as seen from B are 60∘and 45∘. If AB is 30 m, then the distance between the flagstaffs is
A30+15√3B45+15√3C60−15√3D60+15√3Two flagstaffs stand on a horizontal plane. A and B are two points on the line joining their feet and between them. The angles of elevation of the tops of the flagstaffs as seen from A are 30° and 60° and as seen from B are 60" and 45°. If AB is 30m, the distance between the flagstaffs in metres is
A3+15√3B45+15√3C60−15√3D60+15√3
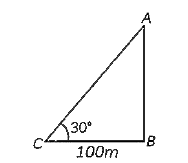
A vertical flagstaff stands on a horizontal plane. From a point 100 m from its foot, the angle of elevation the its top is 30°. Find the height of the flqgstaf.
View SolutionA flagstaff of length l is fixed on the top of a tower of height h. The angles of elevation of the top and bottom of the flagstaff at a point on the ground are 60∘ and 30∘ respectively. Then
View SolutionA vertical flagstaff stands on a horizontal plane. From a point 100 m from its foot, the angle of elevation of its top is found to be 45∘. Find the height of the flagstaff.
View SolutionTwo towers stand on a horizontal plane. P and Q where PQ = 30 m, are two points on the line joining their feet. As seen from P the angle of elevation of the tops of the towers are 30 and 60 but as seen from Q are 60 and 45. The distance between the towers is equal to
View SolutionA flagstaff of length l is fixed on the top of a tower of height h. The angles of elevation of the top and bottom of the flagstaff at a point on the ground are 60∘ and 30∘ respectively. Then
View Solution
