Two line segment AB and AC include an angle of , where AB=5 cm and AC= 7 cm. Locate points P and Q on AB and AC, respectively such that and . Join P and Q and measure the length PQ.
Given that ,AB= 5 cm and AC= 7 cm.
Also, and …..(i)
From Eq. (i),
Then, [ P is any point on the AB]
i.e, scale factor of line segment AB is .
Again from Eq. (i),
Then,
[ Q is any point on the AC]
i.e., scale factor of line segment AQ is .
Steps of construction
1. Draw a line segment AB=5 cm.
2. Now draw a ray AZ making an acute .
3. Whit A as centre and radius equal to 7 cm draw an arc cutting the line AZ at C.
4. Draw a ray AX, making an acute .
5. Along AX , mark 1+3=4 points .
Such that
6. Join ltbr> 7. From draw meeting AB at P. [ by making an angle equal to ]
Then, P is the point on AB which divided it in the ratio 3:1.
So, AP:PB=3:1
.
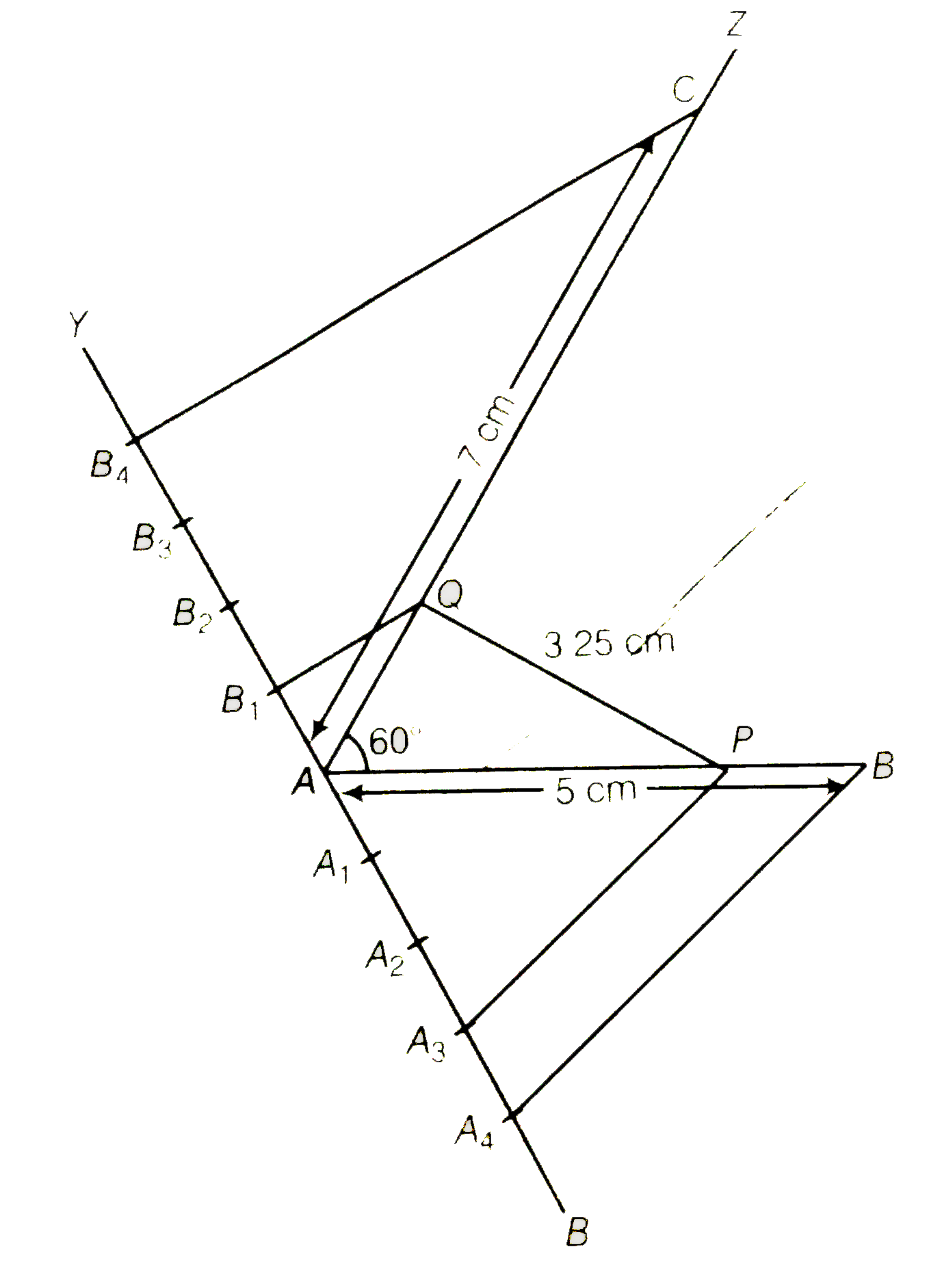
9. Along AY, mark 3+1=4 points
Such that
10. Join .
11. From draw meeting AC at Q. [by making an angle equal to ]
Then, Q is the point on AC which divides in the ratio 1:3.
So, AQ:QC=1:3 ltbtgt 12. Finally, join PQ and its measurement is 3.25 cm.
Topper's Solved these Questions
Similar Questions
Knowledge Check
For a triangle ABC,D and E are two points on AB and AC such that AD=14AB,AE=14AC. If BC=12cm, then DE is
A5 cmB4 cmC3 cmD6 cmIn a ΔABC,AB=AC,PandQ are points on AC and AB respectively such that CB=BP=PQ=QA. if ∠AQP=θ, then tan2θ is a root of the equation
Ay3+21y2−35y−12=0By3−21y2+35y−12=0Cy3−21y2+35y−7=0D12y3−35y2+35y−12=0
Consider a triangle ABC. The sides AB and AC are extended to points D and E respectively, such that AD=3ABandAE=3AC. Then one diagonal of BDEC divides the other diagonal in the ratio p:q then p+q=
View SolutionIn a ΔABC,AB=AC,PandQ are points on AC and AB respectively such that CB=BP=PQ=QA. if ∠AQP=θ, then tan2θ is a root of the equation
View SolutionIf D and E are points on the sides AB and AC respectively of DetlaABC such that AB=5.6cm,AD=1.4cm,AC=7.2cmandAE=1.8cm. Show that DE∣∣BC.
View SolutionIn a △ABC P and Q are points on AB and AC respectively and PQ||BC. Prove that the median AD bisects PQ.
View SolutionIf P and Q are points on the sides AB and AC respeactively of △ABC, if PQ||BC,l AP= 2 cm , AB = 6 cm and AC= 9 cm find AQ.
View SolutionIf P and Q are points on the sides AB and AC respeactively of △ABC, if PQ||BC,l AP= 2 cm , AB = 6 cm and AC= 9 cm find AQ.
View Solution
