Two lines through (2,3) from which the circle x2+y2=25 intercepts chords of length 8 units have equations
(A) 2x+3y=13, x+5y=17
(B) y=3, 12x+5y=39
(C) x=2, 9x−11y=51
(D) y=0, 12x+5y=39 Solution in Tamil
y=3
12x+5y=39
x=2
9x−11y=51
The correct Answer is:1,2
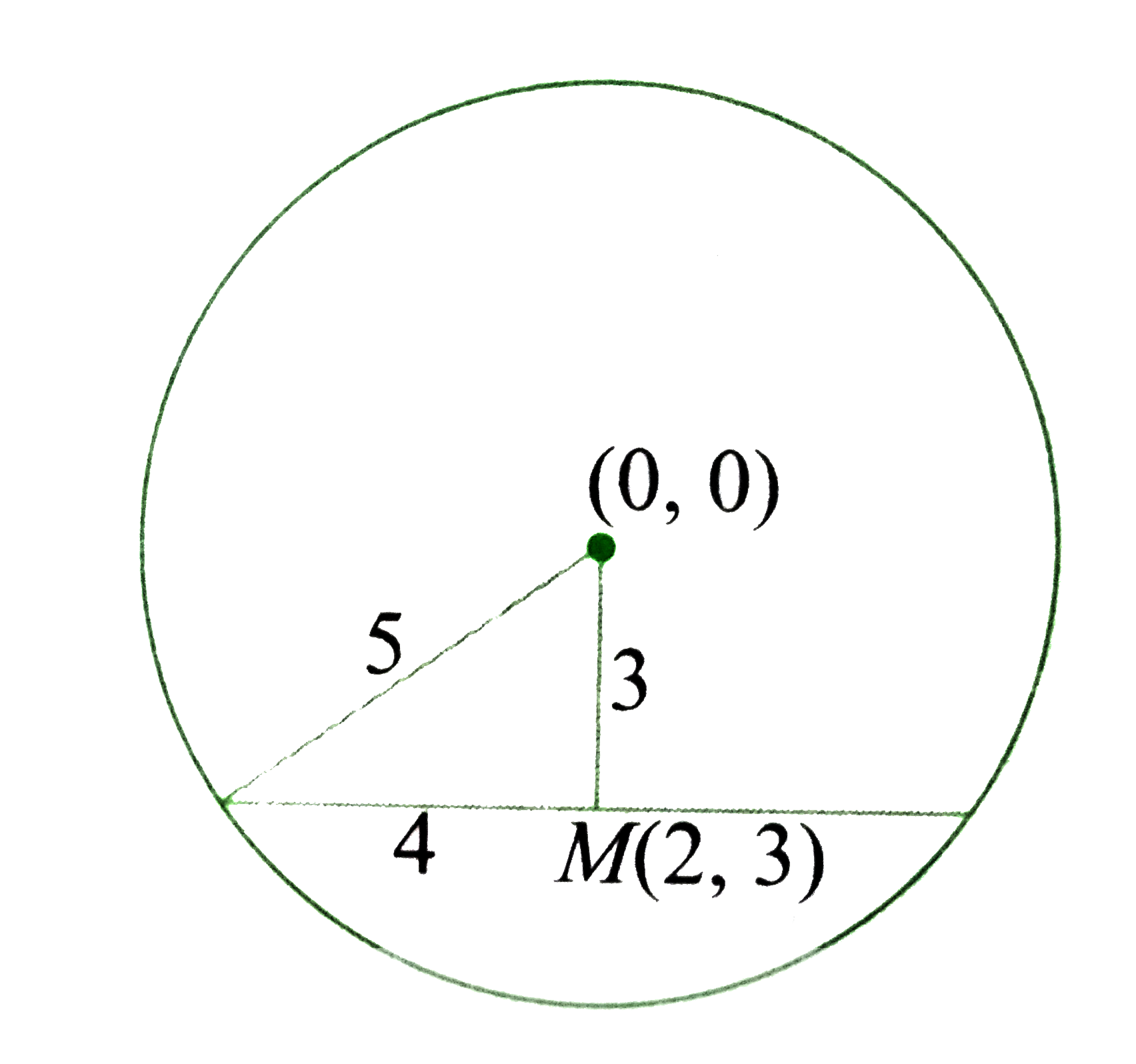
Let the slope the required line be m.
y−3=m(x−2)
⇒mx−y+(3−2m)=0
Length of perpendicular from origin =3
⇒9+4m2−12m=9+9m2
⇒5m2+12m=0
⇒m=0,−125
Hence, lines are
y−3=0 or y=3
and y−3=−125(x−2) or 12x+5y=39
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Comprehension)
46 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Matrix)
1 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Single)
109 Videos
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
16 Videos
Similar Questions
Each side of a square is of length 6 units and the centre of the square Is ( - 1, 2). One of its diagonals is parallel to x+y=0. Find the co-ordinates of the vertices of the square.
View SolutionLet C1 and C2 be two circles whose equations are x2+y2−2x=0 and x2+y2+2x=0 and P(λ,λ) is a variable point
View SolutionThe number of rational point(s) [a point (a, b) is called rational, if aandb both are rational numbers] on the circumference of a circle having center (π,e) is a)at most one b) at least two c)exactly two d) infinite
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionTwo lines through (2,3) from which the circle x^(2)+y^(2)=25 intercepts chords of length 8 units have equations (A) 2x+3y=13,x+5y=17 (B) y=3,12x+5y=39 (C) x=2,9x-11y=51 (D) y=0,12x+5y=39
View SolutionThe equation of the plane through the line x+y+z+3=0=2x−y+3z+1 and parallel to the line x1=y2=z3 is a.x−5y+3=7 b. x−5y+3z=7 c. x+5y=3z=7 d. x+5y+3z=−7
View SolutionProve that the line 12x−5y−3 is mid parallel to the lines 12x−5y+7=0and12x−5y−13=0
View SolutionFind all points on x - y + 2 = 0 that lie at a unit distance from the line 12x−5y+9=0
View SolutionProve that the line 12x-5y-3=0 is mid-parallel to the lines 12x-5y+7=0and 12x-5y-13=0
View Solutionसिद्ध कीजिए की रेखा 12x−5y−3=0 रेखाओं 12x−5y+7=0 तथा 12x−5y−13=0 के मध्यस्थ एक समांतर रेखा है|
View SolutionThe intercept made by the circle x2+y2−4x−6y−3=0 on the line x+y−3=0 is
View SolutionA line of fixed length 2 units moves so that its ends are on the positive x-axis and that part of the line x+y=0 which lies in the second quadrant. Then the locus of the midpoint of the line has equation. (a) x2+5y2+4xy−1=0 (b) x2+5y2+4xy+1=0 (c) x2+5y2−4xy−1=0 (d) 4x2+5y2+4xy+1=0
View SolutionTwo lines through (2,3) from which the circle x2+y2=25 intercepts chords of length 8 units have equations
(A) 2x+3y=13, x+5y=17
(B) y=3, 12x+5y=39
(C) x=2, 9x−11y=51
(D) y=0, 12x+5y=39
View SolutionThe equation of the plane through the line x+y+z+3=0=2x−y+3z+1 and parallel to the line x1=y2=z3 is a.x−5y+3=7 b. x−5y+3z=7 c. x+5y=3z=7 d. x+5y+3z=−7
View Solutionयदि x:y=2:3 हो, तो निम्नलिखित का मान ज्ञात कीजिए
(i) 3x + 2y : 9x + 5y, (ii) 6.x+7y: 12x+5y
View Solution
