Two points PandQ are given. R is a variable point on one side of the line PQ such that ∠RPQ−∠RQP is a positive constant 2α. Find the locus of the point R.
Let R≡(x1,y1).
Also , let, ∠RPM=thetand∠RQM=ϕ.
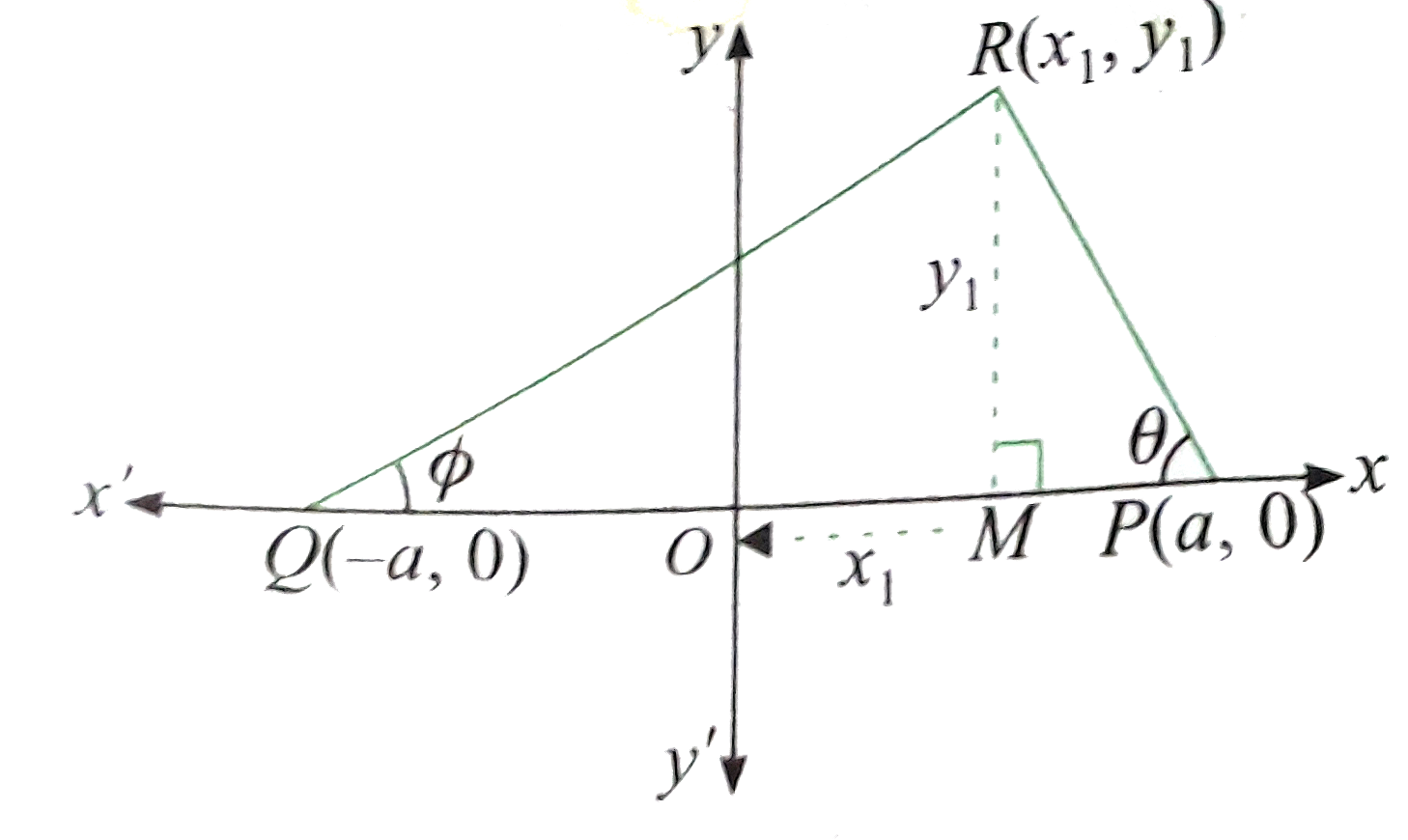
In ΔRMP,tanθ=RMMP=y1a−x1
In ΔRQM,tanθϕ=RMQM=y1a+x1
But given ∠RPQ−anl≥RQP=2α (constant).Therefore, θ−ϕ=2α
or tan(θ−ϕ)=tan2α
or tanθ−tanθ1+tanθtanθ=tan2α
or y1a−x1−y1a+x11+y1a−x1y1a+x1=tan2α
or 2x1y1a2=x21+y21=tanα
or a2−x21+y21=2x1y1cot2α
or x21−y21+2x1y1cot2α=a2
Hence, the locus of the point R(x1,y1) is x2−y2+2xycot2α=a2
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.1
6 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.2
8 Videos
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGECHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Two points P(a,0) and Q(-a,0) are given. R is a variable point on one side of the line PQ such that ∠RPQ−∠RQP is a positive constant 2α. Find the locus of the point R.
View SolutionTwo points P(a,0) and Q(-a,0) are given, R is a variable on one side of the line PQ such that ∠RPQ−∠RQP is a positive constant 2α. FInd the locus of point R.
View Solution
Knowledge Check
P and Q are two points on circle with centre O. The point R is on the minor arcPQ. The tangents from P and Q meet at S. If ∠PSQ=20∘ them ∠PRQ is-
A80∘B200∘C160∘D100∘The middle point of the segment of the straight line joining the points (p,q) and (q,-p) is (r/2 , s/2) . What is the length of the segment ?
A[(s2+r+2)1/2]/2B[(s2+r2)1/2]/4C(s2+r2)1/2Ds+r
Given P=(1,0) and Q=(−1,0) and R is a variable point on one side of the line PQ such that ∠RPQ−∠RQP=π4. The locus of the point R is
View SolutionIf P is any point on the plane lx+my+nz=pandQ is a point on the line OP such that OP.OQ=p2 , then find the locus of the point Q.
View SolutionP is a variable point on a circle C and R is a fixed point on PQ dividing it in the ratio p:q, where p>0andq>0 are fixed. Then the locus of R is
View SolutionP is a variable point on xy=1 in the first quadrant and R is a variable point on the positive x- axis . If rhombus OPQR ( in order ) is completed (where O is the origin ) , then find the locus of point Q.
View SolutionTwo different points P and Q are given on one side of line AB. Draw a circle passing through the points P and Q touching the line AB in point R.
View SolutionFrom a variable point R on the line y = 2x + 3 tangents are drawn to the parabola y2=4ax touch it at P and Q point. Find the locus of the centroid of the triangle PQR.
View Solution
