Two spheres that are tangent to each other have columes of 36π cubic centimeters and 972π cubic centimeters. What is the greatest possible distance, in centimeters, between a point on one sphere and a a second point on the other sphere?
The correct Answer is:24
To solve the problem of finding the greatest possible distance between a point on one sphere and a second point on the other sphere, we will follow these steps:
Step 1: Understand the relationship between volume and radius of a sphere.
The volume V of a sphere is given by the formula:
V=43πr3
where r is the radius of the sphere.
Step 2: Calculate the radius of the first sphere.
Given the volume of the first sphere is 36π cubic centimeters:
43πr31=36π
We can cancel π from both sides:
43r31=36
Multiplying both sides by 34:
r31=36×34=27
Taking the cube root:
r1=√(3)27=3 cm
Step 3: Calculate the radius of the second sphere.
Given the volume of the second sphere is 972π cubic centimeters:
43πr32=972π
Again, we can cancel π:
43r32=972
Multiplying both sides by 34:
r32=972×34=729
Taking the cube root:
r2=√(3)729=9 cm
Step 4: Calculate the diameters of both spheres.
The diameter d1 of the first sphere is:
d1=2r1=2×3=6 cm
The diameter d2 of the second sphere is:
d2=2r2=2×9=18 cm
Step 5: Calculate the greatest possible distance between points on the two spheres.
Since the spheres are tangent to each other, the greatest distance between a point on one sphere and a point on the other sphere is the sum of their diameters:
Greatest Distance=d1+d2=6+18=24 cm
Final Answer:
The greatest possible distance between a point on one sphere and a second point on the other sphere is 24 centimeters.
---
The volume V of a sphere is given by the formula:
V=43πr3
where r is the radius of the sphere.
Given the volume of the first sphere is 36π cubic centimeters:
43πr31=36π
We can cancel π from both sides:
43r31=36
Multiplying both sides by 34:
r31=36×34=27
Taking the cube root:
r1=√(3)27=3 cm
Given the volume of the second sphere is 972π cubic centimeters:
43πr32=972π
Again, we can cancel π:
43r32=972
Multiplying both sides by 34:
r32=972×34=729
Taking the cube root:
r2=√(3)729=9 cm
The diameter d1 of the first sphere is:
d1=2r1=2×3=6 cm
The diameter d2 of the second sphere is:
d2=2r2=2×9=18 cm
Since the spheres are tangent to each other, the greatest distance between a point on one sphere and a point on the other sphere is the sum of their diameters:
Greatest Distance=d1+d2=6+18=24 cm
The greatest possible distance between a point on one sphere and a second point on the other sphere is 24 centimeters.
Topper's Solved these Questions
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - EXERCISE45videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - Multiple Choice99videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATH EXERCISE - PRACTICE TEST24videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE6videos
COMPLEX NUMBERS
Similar Questions
Knowledge Check
- Question 1 - Select One
Jeff tests how the total volume occupied by a fluid contained in a graduated cylinder changes when round marbles of various size are added. He found that the total volume occupied by the fluid, V, in cubic centimeters, can be found using the equation below, where x equals the number of identical marbles Jeff added,one at a time, to the cylinder , and r is the radius of one of the marbles .
V=24π+x(43πr3)
If the volume of the graduated cylinder is 96π cubic centimeters ,then , what is the maximum number of marbles with a radius of 3 centimeters that Jeff can add without the volume of the fluid exceeding that of the graduated cylinder?A1B2C3D4 - Question 2 - Select One
A sphere with a diameter of 2 inches is resting on a table. If the distance from the center of the sphere to the edge of the table is 10 inches, what is the distance, in inches, between the point where the sphere contacts the table and the table edge ?
A4√6B3√11C10D√101
An oil tank has the shape of a right circular cylinder is 12 centimeters in height and has a volume of 108π cubic centimeters. What is the diameter of the base of the cylinder, in centimeters?
View SolutionThe volume of a sphere is increasing at the rate of 3 cubic centimeter per second. Find the rate of increase of its surface area, when the radius is 2 cm.
View SolutionThe volume of a sphere is increasing at the rate of 3 cubic centimeter per second. Find the rate of increase of its surface area, when the radius is 2 cm.
View SolutionTwo spheres of same mass and radius are in contact with each other. If the moment of inertia of a sphere about its diameter is I, then the moment of inertia of both the spheres about the tangent at their common point would be
View SolutionTwo identical spherical masses are kept at some distance. Potential energy when a mass m is taken from the surface of one sphere to the other
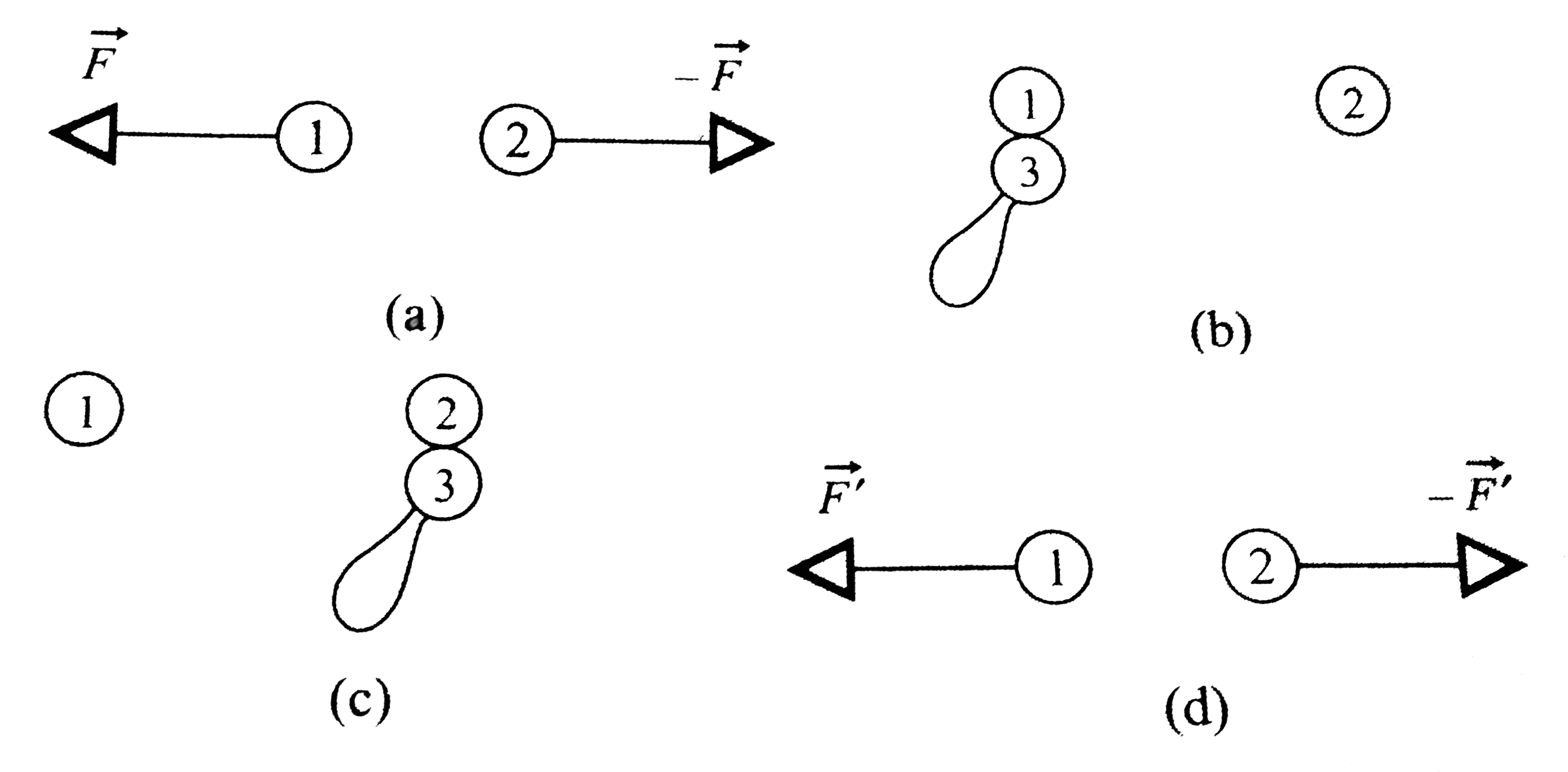
View SolutionTwo identical conducting spheres 1 and 2 carry equal amounts of charge and are ficed apart at a certain distance larger than their diameters. The spheres repel each other with an electrical force of 88mN. A thired identical sphere 3, having an insulating handle and initially uncharged, is touched first to sphere 1 and then to sphere 2 and finally removed. Find the force between spheres 1 and 2 as shown if Figure.
 ,View Solution
,View Solution