What is the equation of the circle which passes through the points (3,-2) and (-2, 0) and having its centre on the line 2x-y-3=0?

A

$x^{2} + y^{2} + 3 x + 2 = 0$

B

$x^{2} + y^{2} + 3 x + 12 y + 2 = 0$

C

$x^{2} + y^{2} + 2 x = 0$

D

$x^{2} + y^{2} = 5$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Given, centre of circle lies on line $2 x - y - 3 = 0$
Let $x = h$
$2 h - y - 3 = 0 \Rightarrow y = 2 n - 3$

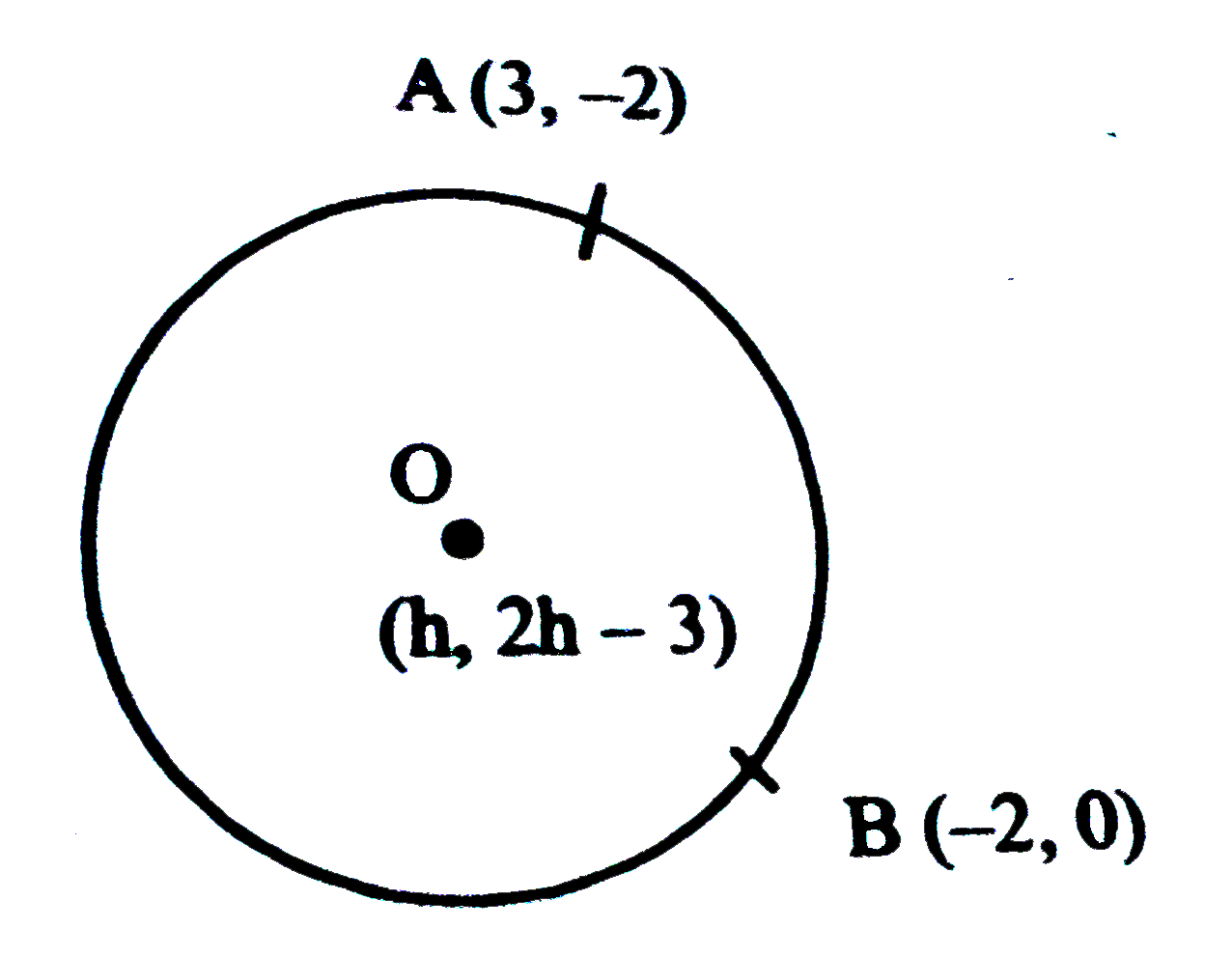
$∴$ centre of circle $= (h, 2 h - 3)$
We know, OA = OB
$\Rightarrow {(h - 3)}^{2} + {(2 h - 3 + 2)}^{2} = {(h + 2)}^{2} + {(2 h - 3)}^{2}$
$\Rightarrow h^{2} - 6 h + 9 + {(2 h - 1)}^{2} = h^{2} + 4 h + 4 + 4 h^{2} - 12 h + 9$
rArr cancel(h^(2)) - 6 h + 9 + cancel(4h^(2)) - 4h + 1
= cancel(h^(2)) + 4 h + 4 + cancel(4h^(2)) - 12h + 9
$\Rightarrow - 2 h = 3 \Rightarrow h = \frac{- 3}{2}$
$∴$ Centre $= (\frac{- 3}{2}, 2 (\frac{- 3}{2}) - 3) = (\frac{- 3}{2}, 6)$
$∴$ radius $= {(h + 2)}^{2} + {(2 h - 3)}^{2}$
$= {(\frac{- 3}{2} + 2)}^{2} + {[2 (\frac{- 3}{2}) - 3]}^{2} = \frac{1}{4} + 36$
$∴$ Equation of circle.
$\Rightarrow {(x + \frac{3}{2})}^{3} + {(y + 6)}^{2} = \frac{1}{4} + 36$
$\Rightarrow x^{2} + y^{2} + 3 x + 12 y + 2 = 0$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The equation of the circle which passes through the points $(2, 3) and (4, 5)$ and the centre lies on the straight line $y - 4 x + 3 = 0,$ is
A $x^{2} + y^{2} - 4 x - 10 y + 25 = 0$
B $x^{2} + y^{2} - 4 x - 10 y - 25 = 0$
C $x^{2} + y^{2} - 4 x + 10 y - 25 = 0$
DNone of the above

NDA PREVIOUS YEARS-CIRCLE-MCQs

Home
Ask Doubt
Profile