Which of the following cannot be valid assignment of probabilities for outcomes of sample Space S={ω1,ω2,ω3,ω4,ω5,ω6,ω7}
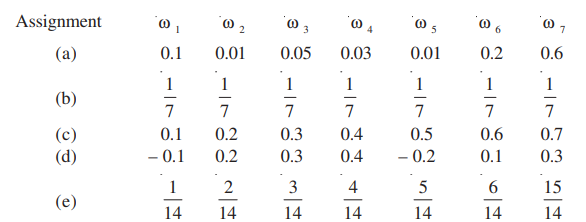
Concept : for valid assignment , sum of all possibilities of each assignment should be 1 .
(a) sum of all possibilities = 0.1+0.01+0.05+0.03+0.01+0.2+0.6=1
Hence, the assignment is valid .
(b) sum of all possibilities = 17+17+17+17+17+17+17=77=1
Hence, the assignment is valid.
(c) sum of possibilities = 0.1+0.2+0.3+0.4+0.5+0.6+0.7=2.8>1
Hence, the assignment is not valid .
(d) sum of possibilities =−0.1+0.2+0.3+0.4−0.2+0.1+0.3=1
Hence, the assignment is not valid because event can't be negative . .
(E) sum of possibilities = 114+214+314+414+514+614+1514=3614>1
Hence, the assignment is not valid .
Topper's Solved these Questions
- PROBABILITYBOOK - NCERTCHAPTER - PROBABILITYEXERCISE - EXERCISE 16.1
16 Videos
- PRINCIPLE OF MATHEMATICAL INDUCTIONBOOK - NCERTCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - EXERCISE 4.1
24 Videos
- RELATIONS AND FUNCTIONSBOOK - NCERTCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - EXERCISE 2.3
5 Videos
Similar Questions
Which of the following cannot be valid assignmentof probabilities for outcomes of sample space
S={ω1,ω2,ω3,ω4,ω5,ω6,ω7}?
Assignement ω1,ω2,ω3,ω4,ω5,ω6,ω7
a. 0.1,0.01,0.05,0.03,0.01,0.3,0.6
b. 17,17,17,17,17,17,17
c. 0.1,0.2,0.3,0.4,0.5,0.6,0.7
d. -0.1,0.2,0.3,0.4,-0.2,0.1,0.3
e. 114,214,314,414,514,614,1514
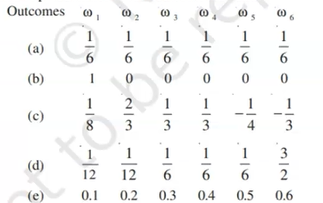
View SolutionLet a sample space be S={ω1,ω2,....,ω6}. Which of the following assignments of probabilities to each outcome are valid?
View Solution
Knowledge Check
∣∣ ∣ ∣∣1ωω2ωω21ω21ω∣∣ ∣ ∣∣
A1BωCω2D0
Let a sample space be:
S={ω1,ω2,…..ωn}. Which of the following assignments of probability to each outcome are valid?
Outcomes ω1,ω2,ω3,ω4,ω5,ω6
a. 16,16,16,16,16,16
b. 1,0,0,0,0,0
c. 18,23,13,13,−14,−13
d. 112,112,16,16,16,32
e. 0.1,0.2,0.3,0.4,0.5,0.6
View Solution∣∣ ∣ ∣∣1ωω2ωω21ω21ω∣∣ ∣ ∣∣=0
View Solution∣∣ ∣ ∣∣1ωω2ωω21ω21ω∣∣ ∣ ∣∣
View Solution∣∣ ∣ ∣∣1ωω2ωω21ω21ω∣∣ ∣ ∣∣=
View Solution∣∣ ∣ ∣∣ω+ω21ωω2+1ω211+ωωω2∣∣ ∣ ∣∣
View Solution(1−ω+ω2)(1+ω−ω2)=4
View SolutionIf ω is a complex cube root of unity. Show that Det⎡⎢⎣1ωω2ωω21ω21ω⎤⎥⎦=0
View Solution
