Which of the following functions stretches y=cos(x) vertically by a factor of 3?
A
y=cos(x+3)
B
y=cos(3x)
C
y=cos(13x)
D
y=3cosx
Video Solution
Text Solution
Vertical stretching is accomplished by multiplying the function by the stretching factor after the function is applied.
|
Topper's Solved these Questions
- TRANSFORMATION AND SYMMETRYBOOK - ENGLISH SATCHAPTER - TRANSFORMATION AND SYMMETRYEXERCISE - EXERCISES
4 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - ENGLISH SATCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - EXERCISE
6 Videos
- TRIANGLE TRIGONOMETRY BOOK - ENGLISH SATCHAPTER - TRIANGLE TRIGONOMETRY EXERCISE - EXERCISES
13 Videos
Similar Questions
Knowledge Check
The graph of the function f(x)=x2−x−3x−1 is shown in the standard (x,y) coordinate plane below . Which of the following , if any is a list of each of the vertical asymptotes of f(x) ?
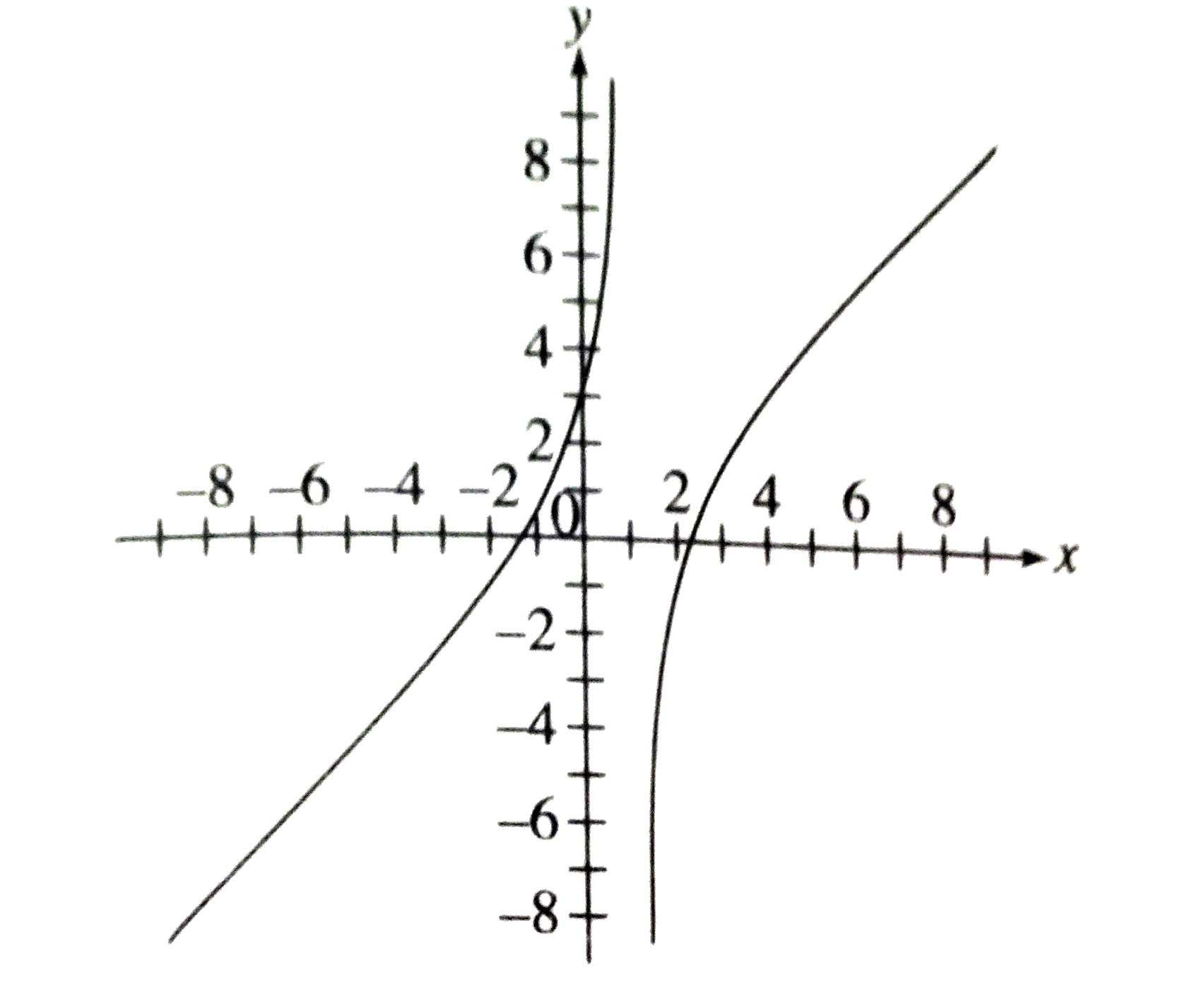 AThis function has no vertical asymptote .By=−12x+1Cy=2x-1Dx=1
AThis function has no vertical asymptote .By=−12x+1Cy=2x-1Dx=1
Plot the following functions.
y=x3−1
View SolutionFind the range of the following functions:
y=12−cos3x
View SolutionWhich of the following relations are functions ? y=3x+2.
View SolutionIn which of the following cases, the function f(x) has vertical tangent at x = 0 ?
f(x)=x2/3
View SolutionIn which of the following cases, the function f(x) has vertical tangent at x = 0 ?
(i) f(x)=x1/3
View SolutionIn which of the following cases, the function f(x) has vertical tangent at x = 0 ?
f(x)=√|x|
View SolutionIn which of the following cases, the function f(x) has vertical tangent at x = 0 ?
(ii) f(x)=sgnx
View Solution
