Which of the following functions transforms y=f(x) moving it 5 units to the right?

y=f(x+5)

y=f(x-5)

y=f(x)+5

y=f(x)-5

Video Solution

Text Solution

Generated By DoubtnutGPT

To determine which function transforms $y = f (x)$ by moving it 5 units to the right, we need to understand how horizontal transformations work in function notation.
1. Understanding Horizontal Shifts:
- A horizontal shift to the right by $a$ units means that we need to replace $x$ in the function with $x - a$ . This is because we want to shift the graph to the right, which effectively means we are evaluating the function at a smaller $x$ value.
2. Applying the Shift:
- For a shift of 5 units to the right, we replace $x$ in $f (x)$ with $x - 5$ . Therefore, the new function becomes:
$y = f (x - 5)$
3. Identifying the Correct Option:
- From the options provided, we need to identify which one corresponds to $y = f (x - 5)$ .
4. Conclusion:
- The correct transformation that moves the function $y = f (x)$ 5 units to the right is:
$y = f (x - 5)$

Updated on:7/8/2024

For which of the following functions does $f (x) f (2 - x)$ ?
A $f (x) = x + 2$
B $f (x) = 2 x - x^{2}$
C $f (x) = 2 - x$
D $f (x) = {(2 - x)}^{2}$
Which of the following is a transformation of y=f(x) that translates this function down 3, shrinks it horizontally by a factor of 2, and reflects it about the x-axis?
A $y = - 2 f (x - 3)$
B $y = f (- 2 x) - 3$
C $y = - f (\frac{1}{2} x) - 3$
D $y = - f (2 x) - 3$
The graph of f(x) is shown below :

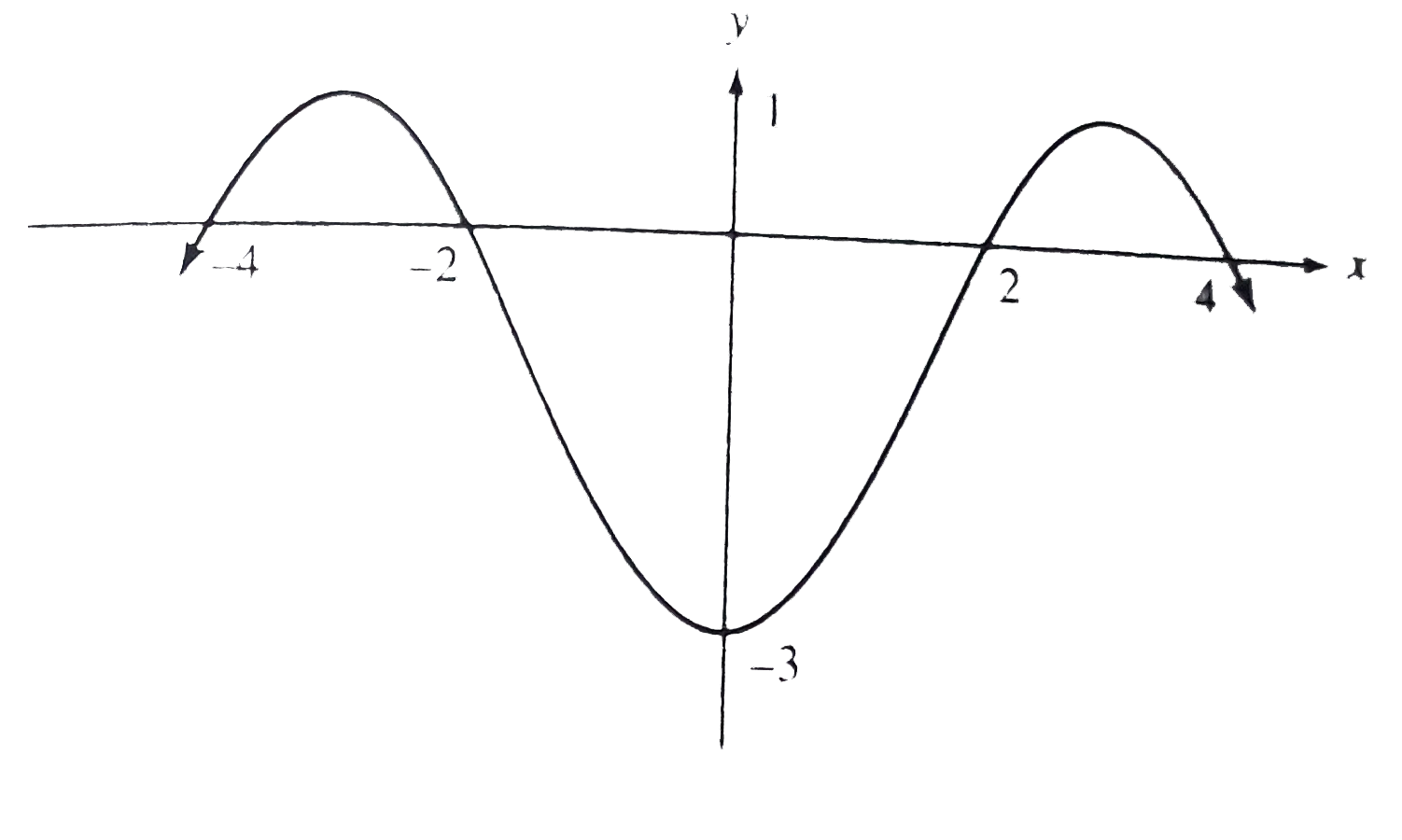
A transformation is applied that results in the following graph :

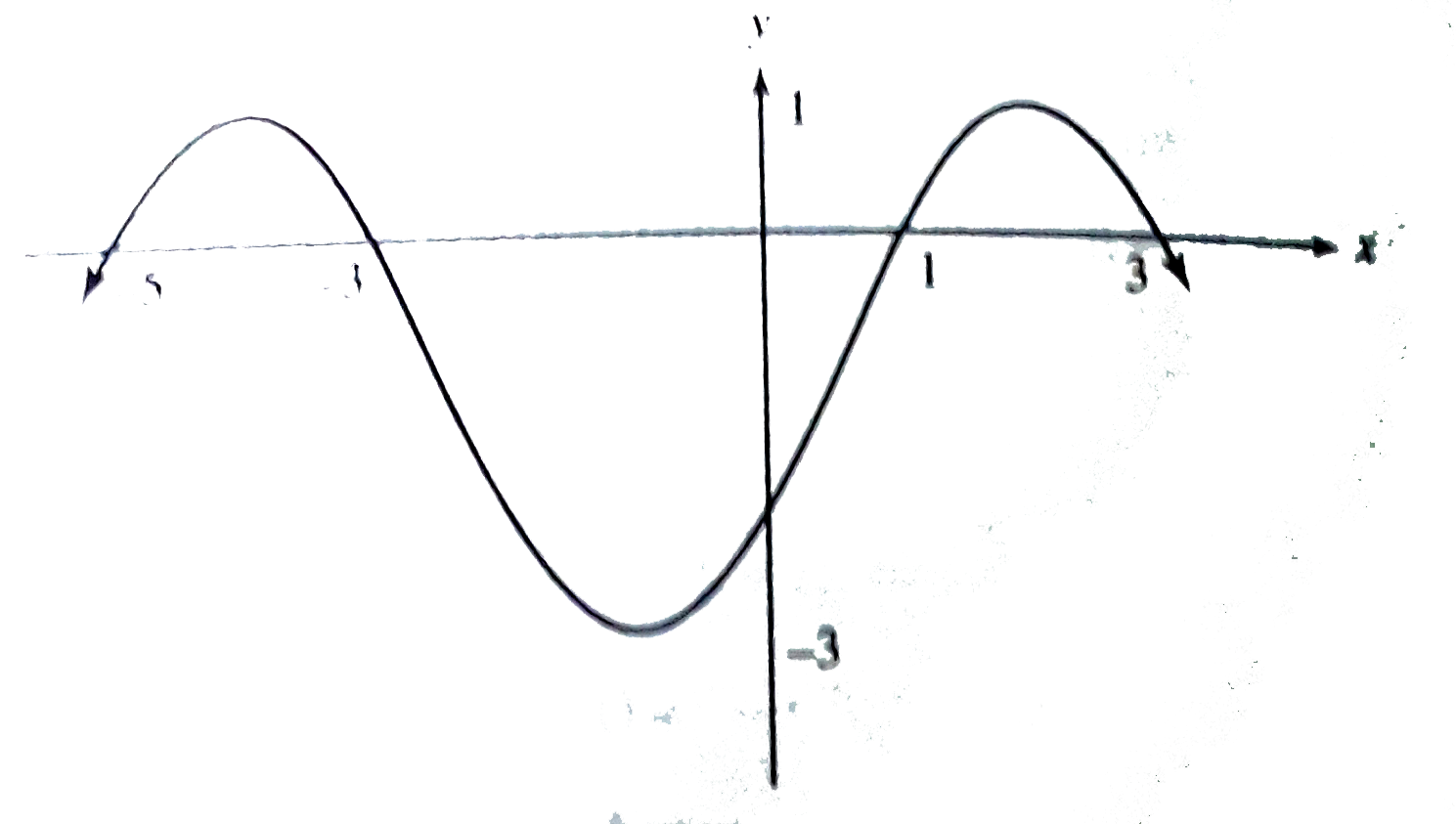
Which of the following functions describes this graph ?
Af(x-1)
Bf(x+1)
Cf(x)-1
Df(x)+1