Which of the following trigonometric functions has an amplitude of 3 ?
f(x)=3 cos x
The correct Answer is:D
To determine which of the given trigonometric functions has an amplitude of 3, we will analyze each option step by step.
Step 1: Understand the concept of amplitude
The amplitude of a trigonometric function is defined as half the distance between its maximum and minimum values. For sine and cosine functions, the general form is Asin(Bx) or Acos(Bx), where A represents the amplitude.
Step 2: Analyze each option
Option 1: f(x)=13sinx
- The amplitude is 13 (since A=13).
- Therefore, the range of this function is from −13 to 13.
- Conclusion: This option does not have an amplitude of 3.
Option 2: f(x)=cos(3x)
- The amplitude is 1 (since A=1).
- The range of this function is from −1 to 1.
- Conclusion: This option does not have an amplitude of 3.
Option 3: f(x)=sin(13x)
- The amplitude is 1 (since A=1).
- The range of this function is from −1 to 1.
- Conclusion: This option does not have an amplitude of 3.
Option 4: f(x)=3cosx
- The amplitude is 3 (since A=3).
- The range of this function is from −3 to 3.
- Conclusion: This option has an amplitude of 3.
Final Conclusion
The function that has an amplitude of 3 is Option 4: f(x)=3cosx.
---
The amplitude of a trigonometric function is defined as half the distance between its maximum and minimum values. For sine and cosine functions, the general form is Asin(Bx) or Acos(Bx), where A represents the amplitude.
- The amplitude is 13 (since A=13).
- Therefore, the range of this function is from −13 to 13.
- Conclusion: This option does not have an amplitude of 3.
- The amplitude is 1 (since A=1).
- The range of this function is from −1 to 1.
- Conclusion: This option does not have an amplitude of 3.
- The amplitude is 1 (since A=1).
- The range of this function is from −1 to 1.
- Conclusion: This option does not have an amplitude of 3.
- The amplitude is 3 (since A=3).
- The range of this function is from −3 to 3.
- Conclusion: This option has an amplitude of 3.
The function that has an amplitude of 3 is Option 4: f(x)=3cosx.
Topper's Solved these Questions
Similar Questions
Knowledge Check
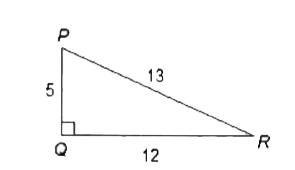
In ΔPQR above, which of the following trigonometric expressions have value 1213?Atan PBcos PCsin RDcos R
Which of the following relations are functions ? y<x+3.
View SolutionWhich of the following functions is an odd functions ?
View SolutionWhich of the following function is an even function ?
View SolutionWhich of the following functions has period 2π ?
View SolutionWhich of the following functions has period π ?
View SolutionEvaluate each of the following trigonometric functions:
(a) cos.4π3 (b) tan(−210∘) (c ) cosec.11π4
View SolutionFind the values of the following trigonometric ratios :
View Solution
