While computing mean of grouped data, we assume that the frequecies are
evenly distributed over all the classes
centred at the class marks of the classes
centred at the upper limits of the classes
centred at the lower limits of the classes
The correct Answer is:B
To solve the question regarding the assumption made while computing the mean of grouped data, we can follow these steps:
1. Understanding Grouped Data:
Grouped data is data that has been organized into classes or intervals. Each class has a frequency that indicates how many data points fall within that interval.
2. Class Marks:
The class mark is the midpoint of a class interval. It is calculated by taking the average of the upper and lower limits of the class. For example, for the class interval 40-50, the class mark would be (40 + 50) / 2 = 45.
3. Frequency Assumption:
When calculating the mean of grouped data, we assume that the frequencies are evenly distributed across the class intervals. This means we consider that all the data points within a class interval are centered around the class mark.
4. Options Analysis:
The question provides four options regarding where the frequencies are centered:
- A) Evenly distributed over all classes centered at class marks
- B) Centered at upper limits of the class
- C) Centered at lower limits of the class
- D) None of the above
5. Correct Answer:
Based on our understanding, the correct answer is that the frequencies are centered at the class marks of the classes. This aligns with option A.
Final Answer:
The correct option is A) Evenly distributed over all classes centered at class marks.
Grouped data is data that has been organized into classes or intervals. Each class has a frequency that indicates how many data points fall within that interval.
The class mark is the midpoint of a class interval. It is calculated by taking the average of the upper and lower limits of the class. For example, for the class interval 40-50, the class mark would be (40 + 50) / 2 = 45.
When calculating the mean of grouped data, we assume that the frequencies are evenly distributed across the class intervals. This means we consider that all the data points within a class interval are centered around the class mark.
The question provides four options regarding where the frequencies are centered:
- A) Evenly distributed over all classes centered at class marks
- B) Centered at upper limits of the class
- C) Centered at lower limits of the class
- D) None of the above
Based on our understanding, the correct answer is that the frequencies are centered at the class marks of the classes. This aligns with option A.
The correct option is A) Evenly distributed over all classes centered at class marks.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - VERY SHORT ANSWERS QUESTIONS14videos
STATISTICS AND PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - SHORT ANSWERS QUESTIONS42videos
STATISTICS AND PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - REAL NUMBERSEXERCISE - LONG ANSWER TYPE QUESTIONS5videos
REAL NUMBERS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - LONG ANSWER TYPE QUESTIONS20videos
SURFACE AREAS AND VOLUMES
Similar Questions
Why do we group data?
View SolutionIn Calculating the mean of grouped data, grouped in classes of equal width, we may use the formula
¯x=a+∑fidi∑fi
Where, a is the assumed mean, a must be one of the mid point of the classes. Is the last statement correct? Justify your answer.View SolutionIt is true to say that the mean, mode and median of grouped data always be different? Justify your answer.
View SolutionFind the mean of following data :
 View Solution
View SolutionMean deviation from mean of the data is
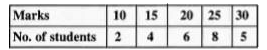 View Solution
View SolutionIn the formula ¯x=a+∑fidi∑fi
for finding the mean of grouped data di'S and deviation from a ofView SolutionFind the mean of the following data :
 View Solution
View SolutionFind the mean of the following data :
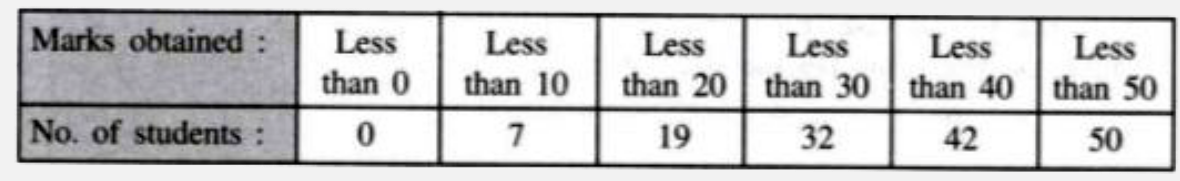 View Solution
View SolutionFind the mean of the following data:
View SolutionThe arithmetic mean and mode of a data are 24 and 12 respectively, then find the median of the data.
View Solution

