Complete Solutions to EXERCISE of chapter SAMPLE PAPER 8 of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | SAMPLE PAPER 8 |
| AGRAWAL PUBLICATION | EXERCISE |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
What is the HCF of 40 and 54 ?
View SolutionFind the value of k for which the polynomial `21 x ^(2) - 3 kx + 7` has real roots.
View SolutionIf the value of 'x' in the equation `2x + 3y = 13` is 2, then find the corresponding value of y.
View SolutionFind the ratio in which x-axis divides the join of points (2, -3) and (5,6) internally.
View SolutionThe coordinates of the point P dividing the line segment joining the points A(1, 3) and B(4, 6) in the ratio 2:1 is
View SolutionIf `Delta ABC ~ Delta DEF and AB = DE, BC = 8 cm,` then the value of EF ?
View SolutionABC is a right triangle, right angled at C and `AB = sqrt2 BC.` Then, find `angle ABC.`
View SolutionThe ratio fo the height of a tower and the length of its shadow is `sqrt3 : 1.` Find the angle of elevation of the Sun.
View SolutionFind the `10^(t h)`term of the AP : 2, 7, 12, . . .
View SolutionFind the sum of the first 10 multiples of 2.
View SolutionWrite a quadratic polynomial whose zero are 2 and -5.
View SolutionThe sum of the digits of a 2-digit number is 10. A number is selected at random. Find the probability of the chosen number to be divisible by 3.
View SolutionAn unbiased dice is rolled once. What is the probability of getting an even prime number?
View SolutionIf `theta` is the angle (in degrees) of a sector of a circle of radius r, then area of sector is
View SolutionA cylindrical pencil sharpend at one edge is the combination of
View SolutionIf one zero of `p (y) = 4y ^(2) - 8 ky - 9` is negative of other, then find the value of K.
View SolutionFind the value of k if the given system of equations 5x + ky = - 7 and x + 2y = 3 is inconsistent :
View SolutionIf `Delta ABC ~ Delta PQR ` , perimeter of `Delta ABC = 32 cm, `perimeter of `Delta `PQR = 48 cm and PR = 6 cm, then find the length of AC.
View SolutionJawaharlal Nehru Stadium in New Delhi is conducting the annual sports competition soon. The curator of the stadium is tasked with preparing the grounds for various sports as per the technical requirements of sports invigilator.
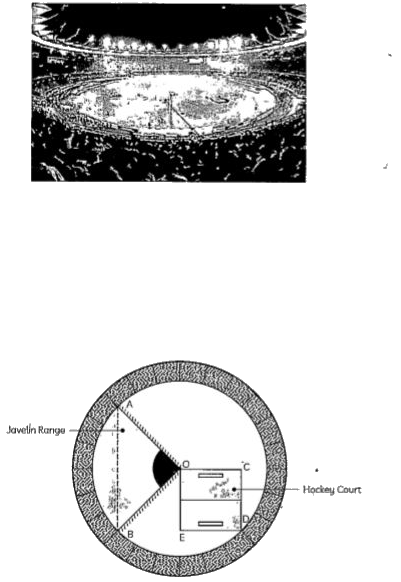
The engineer assigned to assist the curator is tasked with figuring out the dimensions for carving out some areas allotted for a 'hockey court' and a 'javelin range', as shown in the figure below.
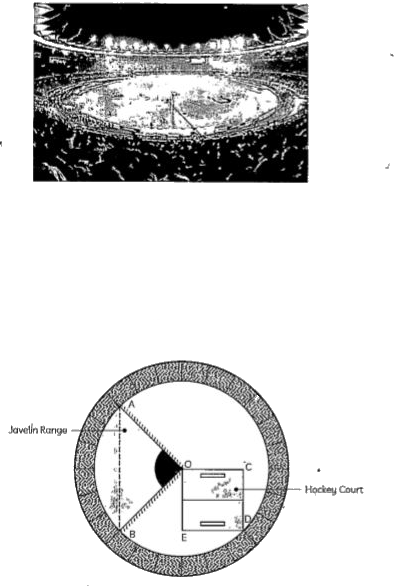
The shapes of the 'hockey court' and the 'javelin range' are square and triangle respectively. Both of the courts have a common edge that touches the centre of stadium. The construction of the javelin range is such that the angle to centre is `90^(@).` The radius of the stadium is 200 metres.
The area (in sq m) allotted to 'javelin" range' is
View SolutionJawaharlal Nehru Stadium in New Delhi is conducting the annual sports competition soon. The curator of the stadium is tasked with preparing the grounds for various sports as per the technical requirements of sports invigilator.
The engineer assigned to assist the curator is tasked with figuring out the dimensions for carving out some areas allotted for a 'hockey court' and a 'javelin range', as shown in the figure below.
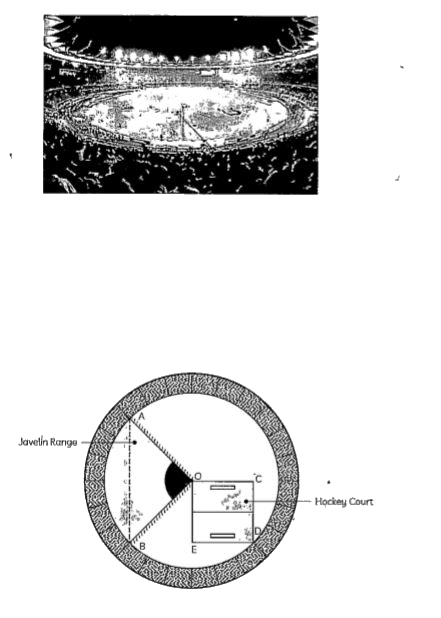
The shapes of the 'hockey court' and the 'javelin range' are square and triangle respectively. Both of the courts have a common edge that touches the centre of stadium. The construction of the javelin range is such that the angle to centre is `90^(@).` The radius of the stadium is 200 metres.
On the basis of the above information, answer any four of the following question:
The area (in sqm) allotted to 'Hockey court' is
View SolutionJawaharlal Nehru Stadium in New Delhi is conducting the annual sports competition soon. The curator of the stadium is tasked with preparing the grounds for various sports as per the technical requirements of sports invigilator.
The engineer assigned to assist the curator is tasked with figuring out the dimensions for carving out some areas allotted for a 'hockey court' and a 'javelin range', as shown in the figure below.
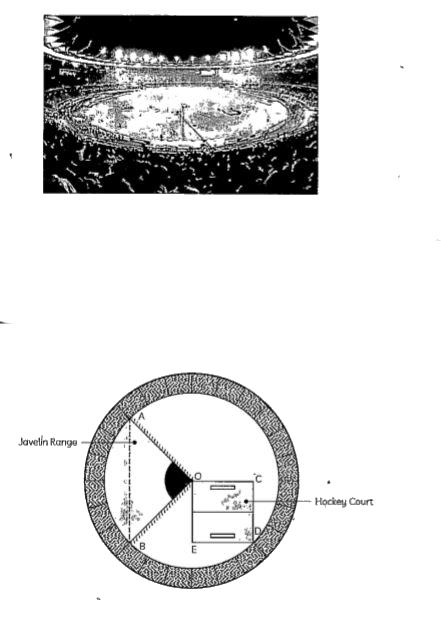
The shapes of the 'hockey court' and the 'javelin range' are square and triangle respectively. Both of the courts have a common edge that touches the centre of stadium. The construction of the javelin range is such that the angle to centre is `90^(@).` The radius of the stadium is 200 metres.
If the team of the curators managing the stadium, likes to allot space for some more sports, how much area (in sq m) is available to them?
View SolutionJawaharlal Nehru Stadium in New Delhi is conducting the annual sports competition soon. The curator of the stadium is tasked with preparing the grounds for various sports as per the technical requirements of sports invigilator.
The engineer assigned to assist the curator is tasked with figuring out the dimensions for carving out some areas allotted for a 'hockey court' and a 'javelin range', as shown in the figure below.
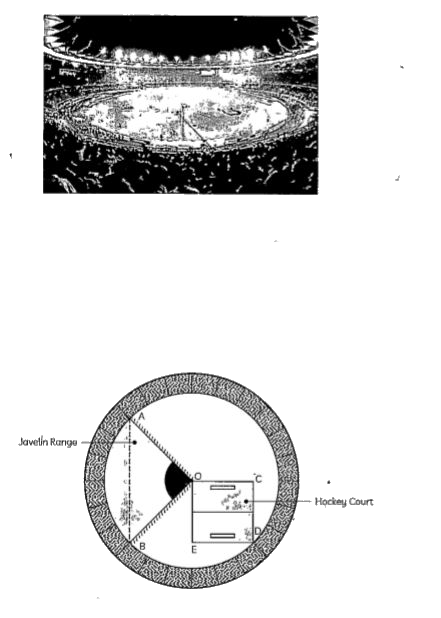
The shapes of the 'hockey court' and the 'javelin range' are square and triangle respectively. Both of the courts have a common edge that touches the centre of stadium. The construction of the javelin range is such that the angle to centre is `90^(@).` The radius of the stadium is 200 metres.
If the boundaries of the hockey court and javelin range are to be fenced, then the total length (in m) of the fence required is
View SolutionJawaharlal Nehru Stadium in New Delhi is conducting the annual sports competition soon. The curator of the stadium is tasked with preparing the grounds for various sports as per the technical requirements of sports invigilator.
The engineer assigned to assist the curator is tasked with figuring out the dimensions for carving out some areas allotted for a 'hockey court' and a 'javelin range', as shown in the figure below.
The shapes of the 'hockey court' and the 'javelin range' are square and triangle respectively. Both of the courts have a common edge that touches the centre of stadium. The construction of the javelin range is such that the angle to centre is `90^(@).` The radius of the stadium is 200 metres.
If the cost of fencing is Rs 12 per metre, then the total cost of fencing is
View SolutionA game at a stall in Diwali fare involves using a spinner first as a pre-cursor to complete the game with certain rules. If the spinner stops at a particular number, then the player is allowed to roll a 6. faced unbiased die,
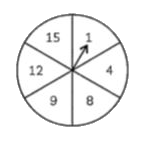
What is the probability of getting an odd number on the spinner?
View SolutionA game at a stall in Diwali fare involves using a spinner first as a pre-cursor to complete the game with certain rules. If the spinner stops at a particular number, then the player is allowed to roll a 6. faced unbiased die,
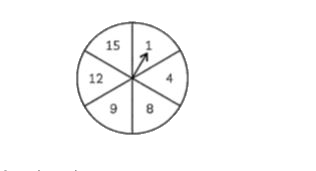
If getting an even number on the spinner allows a player to roll the die, then the probability of his rolling the die is
View SolutionA game at a stall in Diwali fare involves using a spinner first as a pre-cursor to complete the game with certain rules. If the spinner stops at a particular number, then the player is allowed to roll a 6. faced unbiased die,
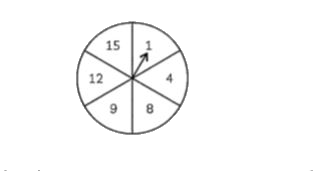
If the player is allowed to roll the die and getting a prime number entitles him to get prize, then the probability of his winning the prize is
View SolutionA game at a stall in Diwali fare involves using a spinner first as a pre-cursor to complete the game with certain rules. If the spinner stops at a particular number, then the player is allowed to roll a 6. faced unbiased die,
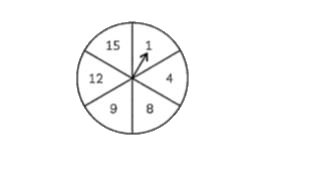
If getting a square number on the spinner allows a player to'roll the die, then the probability of his rolling the die is
View SolutionA game at a stall in Diwali fare involves using a spinner first as a pre-cursor to complete the game with certain rules. If the spinner stops at a particular number, then the player is allowed to roll a 6. faced unbiased die,
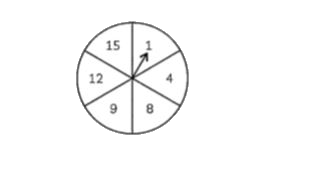
If the player is allowed to roll the die and getting a number greater than 5 erititles him to get prize, then the probability of his winning the prize'is
View SolutionRadio towers are typically tall structures designed to support antennas for tele communications and broadcasting, including television. There are 2 main types: guyed and self-supporting structures.
They are among the tallest human-made structures. Masts are often named after the broadcasting organizations that originally built them or currently use them.

On a similar concept, a radio - station tower was built in two sections A and B. From a point 24 m from the base of the tower, the angle of elevation of the top of section A is `30^(@)` and the angle of elevation of the top of section B is `45^@).`
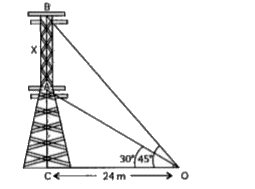
The height of the section A is
View SolutionRadio towers are typically tall structures designed to support antennas for tele communications and broadcasting, including television. There are 2 main types: guyed and self-supporting structures.
They are among the tallest human-made structures. Masts are often named after the broadcasting organizations that originally built them or currently use them.
On a similar concept, a radio - station tower was built in two sections A and B. From a point 24 m from the base of the tower, the angle of elevation of the top of section A is `30^(@)` and the angle of elevation of the top of section B is `45^@).`
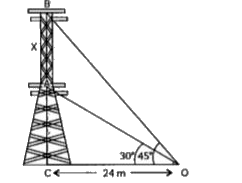
The height of the section B is
View SolutionRadio towers are typically tall structures designed to support antennas for tele communications and broadcasting, including television. There are 2 main types: guyed and self-supporting structures.
They are among the tallest human-made structures. Masts are often named after the broadcasting organizations that originally built them or currently use them.

On a similar concept, a radio - station tower was built in two sections A and B. From a point 24 m from the base of the tower, the angle of elevation of the top of section A is `30^(@)` and the angle of elevation of the top of section B is `45^@).`
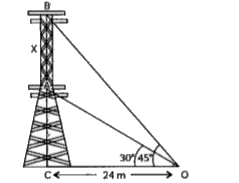
The height of the tower is
View SolutionRadio towers are typically tall structures designed to support antennas for tele communications and broadcasting, including television. There are 2 main types: guyed and self-supporting structures.
They are among the tallest human-made structures. Mosts are often named after the broadcasting organizations that originally built them or currently use them.
On a similar concept, a radio - station tower was built in two sections A and B. From a point 24 m from the base of the tower, the angle of elevation of the top of section A is `30^(@)` and the angle of elevation of the top of section B is `45^@).`
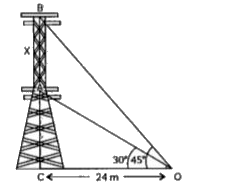
On the basis of the above information, answer any four of the following question:
The length of the wire structure from the point to the top of section A is
View SolutionRadio towers are typically tall structures designed to support antennas for tele communications and broadcasting, including television. There are 2 main types: guyed and self-supporting structures.
They are among the tallest human-made structures. Masts are often named after the broadcasting organizations that originally built them or currently use them.

On a similar concept, a radio - station tower was built in two sections A and B. From a point 24 m from the base of the tower, the angle of elevation of the top of section A is `30^(@)` and the angle of elevation of the top of section B is `45^@).`
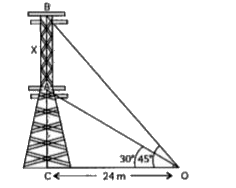
On the basis of the above information, answer any four of the following question:
The length of the wire structure from the point o to the top of section B is
View SolutionExpress 0.3528 in the form `(p)/( 2 ^(m) 5 ^(n))` and write the values of p, m and n.
View SolutionIf HCF (150, 210) = 30, then find LCM (150, 210).
View SolutionFind the value of x for which 2x, (x + 10) and (3x + 2) are three consecutive terms of an A.P.
View SolutionIf the first term of an A.P. is p and its common difference is q. then find its 6th term.
View SolutionFind a relation between x and y such that the point (x, y) is equidistant from the point `(3,\ 6)` and `(-3,\ 4)`.
View SolutionThe shadow of a 5-m-long stick is 2m long. At the same time, the length of the shadow of a `12.5m` high tree is
View SolutionThe area of a circle is 154 sq. cm. Find its circumference.
View SolutionA bag contains 3 red and 5 blue balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is:
red?
View SolutionA bag contains 3 red and 5 blue balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is yellow ?
View SolutionFind the greatest 4-digit number which is divisible by 15, 24 and 36.
View SolutionDetermine the AP whose 3rd term is 5 and the `7^(th)` term is 9 .
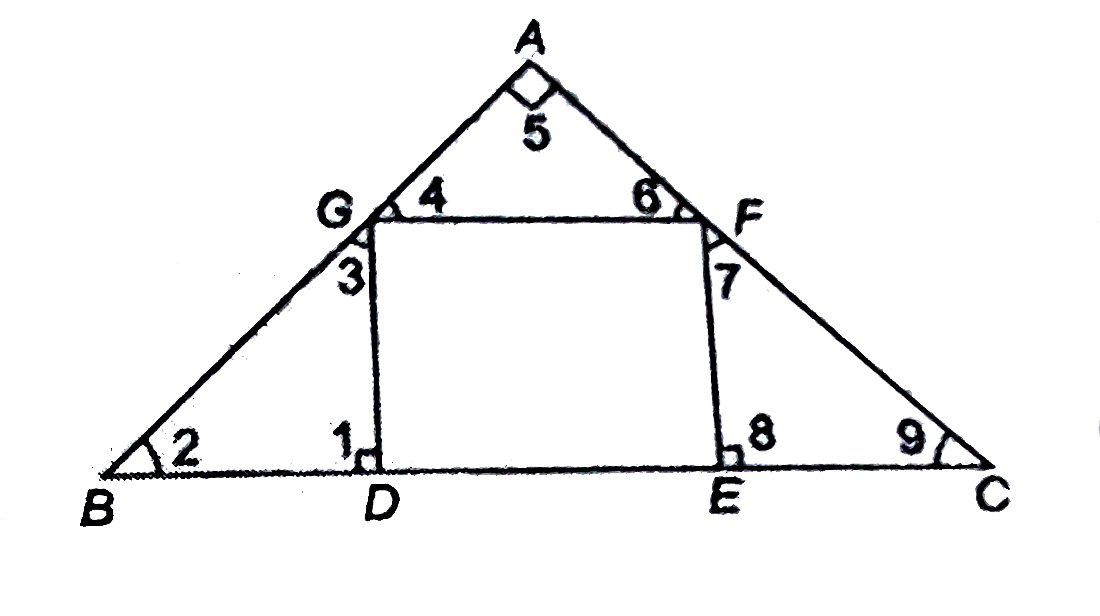
View SolutionIn the given figure , DEFG is a square and `angleBAC= 90^(@)`
prove that
(i) `triangleAGF~ triangleDBG`
`(ii) triangleAGF~triangleEFC`
(iii) `triangleDBG ~ triangleEFC`
(iv) ` DE^(2)= BD xx EC`
View SolutionDraw a line segment of length 5.6 cm and divide it in the ratio 4:3. Measure the two parts.
View SolutionIn Figure, a quadrilateral ABCD is drawn to circumscribe a circle .
Prove that
`AB+CD=BC+AD`

View SolutionA cubical block of side 7 cm is surmounted by a hemisphere. What is the greatest diameter of the hemisphere can have? Find the total surface area of the solid.
View SolutionSolve for x : `(1)/(x + 4) - (1)/(x-7) = (11)/(30), x ne 4, 7`
View SolutionUsing the quadratic formula,solve for `x`: `3x^(2)+2sqrt(5)x-5=0`
View SolutionState and prove the Pythagoras theorem.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is `30o` . Find the height of the tower.
View SolutionAfter how many places will the decimal expansion of `(189)/(125)` terminate?
View SolutionInsert a rational and irrational number between 2 and 3
View SolutionFind the zeros of the polynomial `4x^(2) -12x+9`.
View SolutionIf `x=a,y=b` is the solution of the equations `x-y=2 and x+y=4,` then find the values of a abd b.
View SolutionFind discriminant of the quadratic equation `2x^2+4x-7=0`
View SolutionFind the roots of quadratic equation `x^2 - 4x + 2`.
View SolutionIf `S_n = 5n^2 + 3n`, then find its `n^(th)` term.
View SolutionIn an A.P., a=-6 and d = 2. Find the sum of its first 20 terms.
View SolutionWrite the relationship between the coefficients, if the following pair of equations is inconsistent.
ax + by + c = 0,
a'x + b'y + c' = 0
View SolutionIn a `triangleABC`, right-angled at B, if AB : AC = 1:2, then find the value of
`(2tanA)/(1+tan^2A)`.
View SolutionIf tan `theta + cot theta = 2` then the value of `tan^2 theta + cot^2 theta` is
View SolutionIf tan A = 1 `(0^@ lt A lt 90^@`) and cos B =`(1)/(sqrt2)(0^@ lt B lt 90^@)`, then find the value of cos (A + B).
View SolutionEvaluate : `sin^2 60^@+ 2tan 45^@-cos^2 30^@`
View SolutionWhat would be the area of a circle whose circumference is 22 cms. ?
View SolutionThe volume of two sphere are in the ratio 8:27. The ratio of their surface area is :
View SolutionFind the class-marks of the class interval 10-25.
View SolutionOne card is drawn at from a pack of 52 cards. Find the probability that the card drawn is:
either red or a queen.
View SolutionHow many face cards are there in a pack of 52 cards?
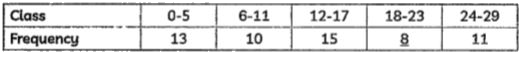
View SolutionDetermine the upper limit of the modal class of the following frequency distribution:
View SolutionEmpiricla relationship between the three measures of central tendency is
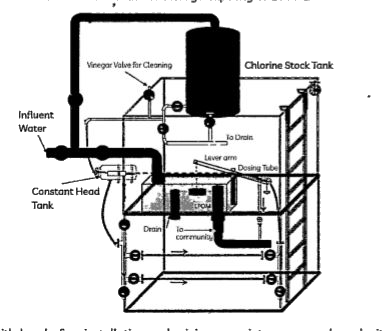
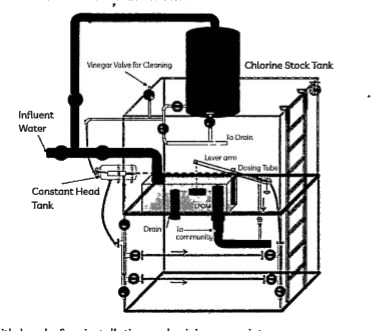
View SolutionSelvi is setting up a water purifier system in her house which includes setting up an overhead tank in the shape of a right circular cylinder. This is filled by pumping water from a sump (underground tank) which is in the shape of a cuboid.
The underground water tank (sump) is a sturdy single moulded piece built to with stand underground pressure and is available in the storage capacity of 2000 L.
These, along with hassle-free installation and minimum maintenance needs make it the ideal water storage solution.
Dimensions (sump): 1.57 m `xx` 1.44 m `xx` 95 cm.
Dimensions (overhead tank):
Radius 60 cm and Height 95 cm
Water flow conditions at the required overload capacity should be checked for critical pressure drop to ensure that valves are adequately sized.
On the basis of the above information, answer the following qeuestions:
The ratio of the capacity of the sump to the capacity of the overhead tank is
View SolutionSelvi is setting up a water purifier system in her house which includes setting up an overhead tank in the shape of a right circular cylinder. This is filled by pumping water from a sump (underground tank) which is in the shape of a cuboid.
The underground water tank (sump) is a sturdy single moulded piece built to with stand underground pressure and is available in the storage capacity of 2000 L.
These, along with hassle-free installation and minimum maintenance needs make it the ideal water storage solution.
Dimensions (sump): 1.57 m `xx` 1.44 m `xx` 95 cm.
Dimensions (overhead tank):
Radius 60 cm and Height 95 cm
Water flow conditions at the required overload capacity should be checked for critical pressure drop to ensure that valves are adequately sized.
On the basis of the above information, answer the following qeuestions:
If overhead tank need to be painted to save it from corrosion, how much area need to be painted?
View SolutionSelvi is setting up a water purifier system in her house which includes setting up an overhead tank in the shape of a right circular cylinder. This is filled by pumping water from a sump (underground tank) which is in the shape of a cuboid.
The underground water tank (sump) is a sturdy single moulded piece built to with stand underground pressure and is available in the storage capacity of 2000 L.
These, along with hassle-free installation and minimum maintenance needs make it the ideal water storage solution.
Dimensions (sump): 1.57 m `xx` 1.44 m `xx` 95 cm.
Dimensions (overhead tank):
Radius 60 cm and Height 95 cm
Water flow conditions at the required overload capacity should be checked for critical pressure drop to ensure that valves are adequately sized.
The capacity (in litres) of the overhead tank is
View SolutionSelvi is setting up a water purifier system in her house which includes setting up an overhead tank in the shape of a right circular cylinder. This is filled by pumping water from a sump (underground tank) which is in the shape of a cuboid.
The underground water tank (sump) is a sturdy single moulded piece built to with stand underground pressure and is available in the storage capacity of 2000 L.
These, along with hassle-free installation and minimum maintenance needs make it the ideal water storage solution.
Dimensions (sump): 1.57 m `xx` 1.44 m `xx` 95 cm.
Dimensions (overhead tank):
Radius 60 cm and Height 95 cm
Water flow conditions at the required overload capacity should be checked for critical pressure drop to ensure that valves are adequately sized.
On the basis of the above information, answer the following qeuestions:
If water is filled in the overhead tank at the rate of 20 litre per minute the tank will be completely filled in how many time?
View SolutionSelvi is setting up a water purifier system in her house which includes setting up an overhead tank in the shape of a right circular cylinder. This is filled by pumping water from a sump (underground tank) which is in the shape of a cuboid.
The underground water tank (sump) is a sturdy single moulded piece built to with stand underground pressure and is available in the storage capacity of 2000 L.
These, along with hassle-free installation and minimum maintenance needs make it the ideal water storage solution.
Dimensions (sump): 1.57 m `xx` 1.44 m `xx` 95 cm.
Dimensions (overhead tank):
Radius 60 cm and Height 95 cm
Water flow conditions at the required overload capacity should be checked for critical pressure drop to ensure that valves are adequately sized.
On the basis of the above information, answer the following qeuestions:
If the amount of water in the sump, at an instant, is 1500 litres, then the water level in the sump at that instant is
View SolutionRishu is riding in a hot air balloon. After reaching a point P, he spots a car parked at Bon the ground at an angle of depression of `30^@`. The balloon rises further by 50 metres and now he spots the same car at an angle of depression of 45o and a lorry parked at B' at an angle of depression of `30^@`. (Use `sqrt3` = 1.73)
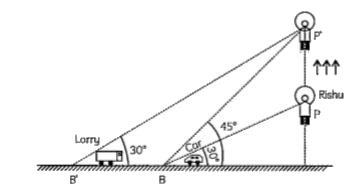
The measurement of Rishu facing vertically is the height. Distance is defined as the measurement of car/lorry from a point in a horizontal direction. If an imaginary line is drawn from the observation point to the top edge of the car/lorry, a triangle is formed by the vertical, horizontal and imaginary line.
If the height of the balloon at point P is 'h' m and distance AB is 'x' m, then 'x' and 'h' are related as:
View SolutionRishu is riding in a hot air balloon. After reaching a point P, he spots a car parked at Bon the ground at an angle of depression of `30^@`. The balloon rises further by 50 metres and now he spots the same car at an angle of depression of `45^@` and a lorry parked at B' at an angle of depression of `30^@`. (Use `sqrt3` = 1.73)
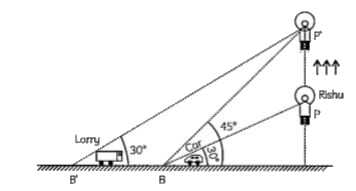
The measurement of Rishu facing vertically is the height. Distance is defined as the measurement of car/lorry from a point in a horizontal direction. If an imaginary line is drawn from the observation point to the top edge of the car/lorry, a triangle is formed by the vertical, horizontal and imaginary line.
The height of the balloon at point P, then
View SolutionRishu is riding in a hot air balloon. After reaching a point P, he spots a car parked at Bon the ground at an angle of depression of `30^@`. The balloon rises further by 50 metres and now he spots the same car at an angle of depression of `45^@` and a lorry parked at B' at an angle of depression of `30^@`. (Use `sqrt3` = 1.73)

The measurement of Rishu facing vertically is the height. Distance is defined as the measurement of car/lorry from a point in a horizontal direction. If an imaginary line is drawn from the observation point to the top edge of the car/lorry, a triangle is formed by the vertical, horizontal and imaginary line.
The height of the balloon at point P, then
View SolutionRishu is riding in a hot air balloon. After reaching a point P, he spots a car parked at Bon the ground at an angle of depression of `30^@`. The balloon rises further by 50 metres and now he spots the same car at an angle of depression of 45o and a lorry parked at B' at an angle of depression of `30^@`. (Use `sqrt3` = 1.73)
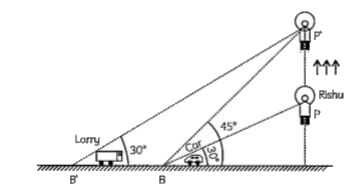
The distance AB on the ground is
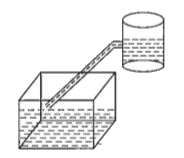
View SolutionA factory is using an inclined conveyor belt to transport its product from level 1 to 2 which is 3 m above level - 1 as shown in the figure below. The inclined conveyor is supported from one end to level 1 and from the other end to a post located 8 m away from level 1 supporting point.

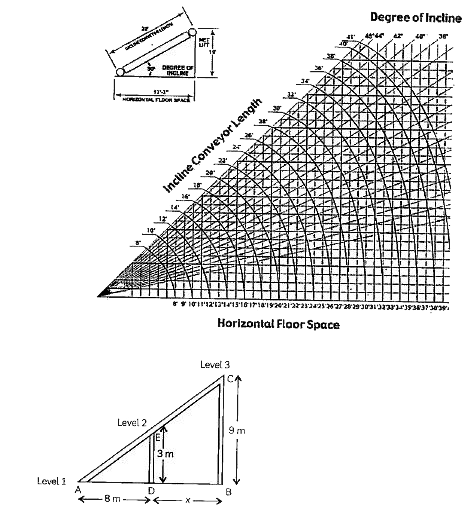
The factory wants to extend the conveyor belt to reach at a new level 3 which is 9 m above level 1 while maintaining the inclination angle.
How much distance is extended from D to B?
View SolutionA factory is using an inclined conveyor belt to transport its product from level 1 to 2 which is 3 m above level - 1 as shown in the figure below The inclined conveyor is supported from one end to level 1 and from the other end to a post located 8 m away from level 1 supporting point.

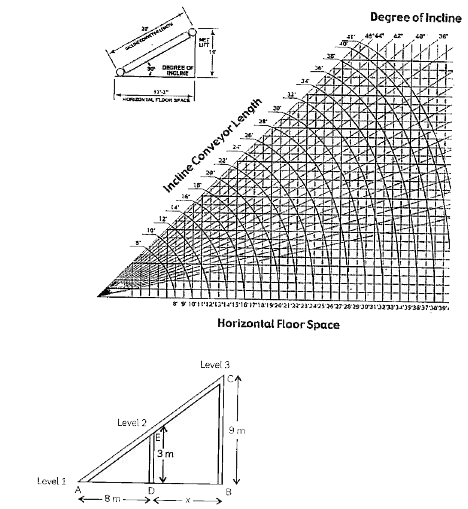
The factory wants to extend the conveyor belt to reach at a new level 3 which is 9 m above level 1 while maintaining the inclination angle.
The length of the conveyor belt up to level 3 is
View SolutionA factory is using an inclined conveyor belt to transport its product from level 1 to 2 which is 3 m above level - 1 as shown in the figure below The inclined conveyor is supported from one end to level 1 and from the other end to a post located 8 m away from level 1 supporting point.

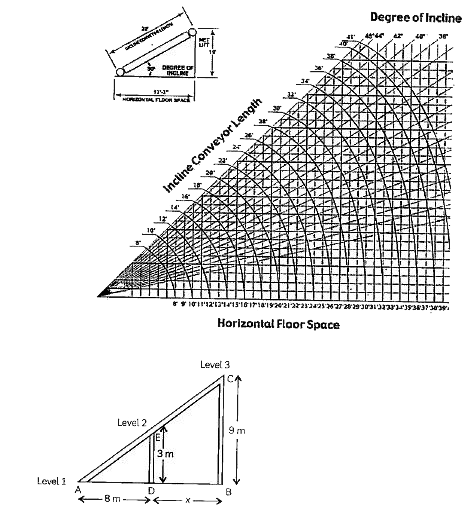
The factory wants to extend the conveyor belt to reach at a new level 3 which is 9 m above level 1 while maintaining the inclination angle.
The length of the conveyor belt up to level 2 is
View SolutionIn order to conduct Sports Day activities in your School, lines have been drawn with chalk powder at a distance of 1 m each, in a rectangular shaped ground ABCD, 100 flowerpots have been placed at a distance of 1 m from each other along AD, as shown in given figure below. Niharika runs 1/4 th the distance AD on the 2nd line and posts a green flag. Preet runs 1/5 th distance AD on the eighth line and posts a red flag.
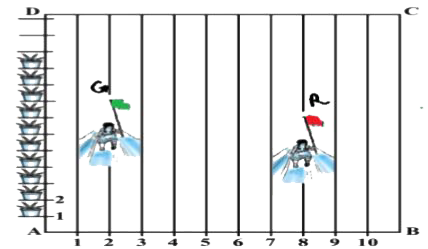
Find the position of green flag
View SolutionIn order to conduct Sports Day activities in your School, lines have been drawn with chalk powder at a distance of 1 m each, in a rectangular shaped ground ABCD, 100 flowerpots have been placed at a distance of 1 m from each other along AD, as shown in given figure below. Niharika runs 1/4 th the distance AD on the 2nd line and posts a green flag. Preet runs 1/5 th distance AD on the eighth line and posts a red flag.
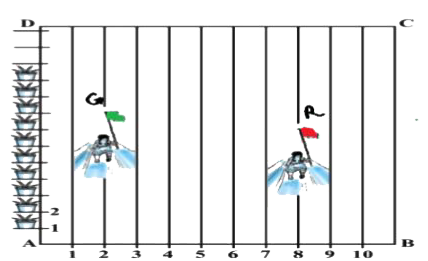
Find the position of red flag
View SolutionA line intersects the y-axis and x-axis at the points P and Q respectively. If (2,-5) is the mid-point of PQ then find the coordinates of P and Q.
View SolutionFind the third vertex of a triangle, if two of its vertices are at `(-3, 1)` and `(0, -2)` and the centroid is at the origin.
View SolutionExplain why `3 xx 5 xx 7 xx 9 xx 11 +11` is a composite number.
View SolutionIf `n=2^3xx3^4xx5^4xx7`, where n is a natural number, then find the number of consecutive zeros in n
View SolutionSolve for x and y :
`7x - 4y = 49, 5x - 6y=57`
View SolutionMetallic spheres of radii 6cm, 8cm and 10cm respectively are melted to form a solid sphere. Find the radius of the resulting sphere.
View SolutionIf a number `x` is chosen at random from the numbers `-2,\ -1,\ 0,\ 1,\ 2` . What is the probability that `x^2<2` ?
View SolutionProve that for any prime positive integer `p ,sqrt(p)` is an irrational number.
View SolutionFind the zeros of the polynomial `2x^2-(1+2sqrt2)x+sqrt2`
View SolutionSolve for x:
`(1)/(x-2)+(2)/(x-1)=(6)/(x)`,x `ne`0,1,2
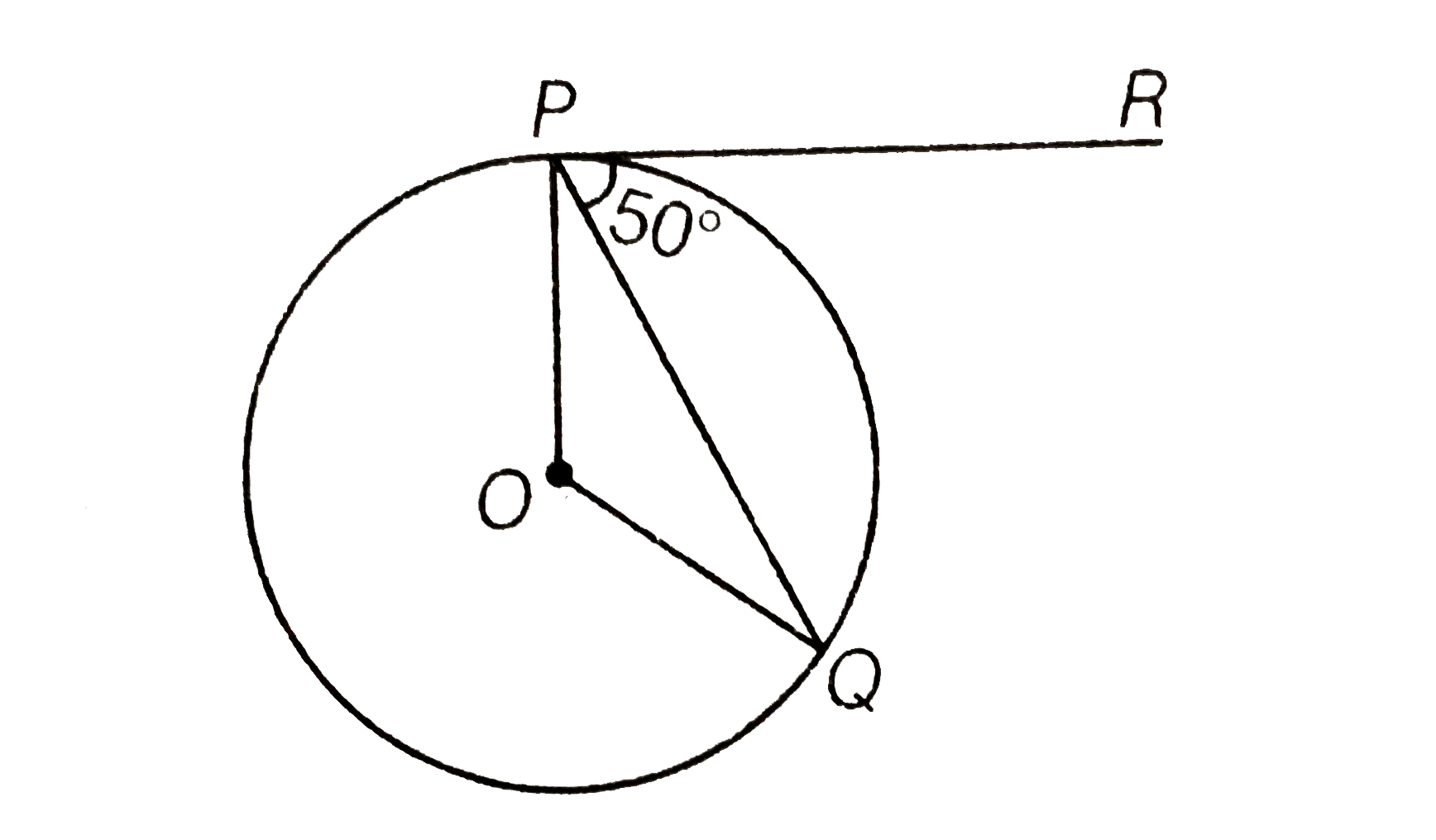
View SolutionIn figure, if 0 is the centre of a circle, PQ is a chord and the tangent PR at P makes an angle of `50^(@)` with PQ, then `anglePOQ` is equal to
View Solution`O` is any point inside a triangle `A B C` . The bisector of `/_A O B ,/_B O C` and `/_C O A` meet the sides `A B ,B C` and `C A` in point `D ,Ea n dF` respectively. Show that `A D x B E x C F=D B x E C x F A`
View SolutionThe central Park is in the form of a circle with centre O and radius 21 m.
Find the total lengths of the curbs,
View SolutionState and prove Basic Proportionality Theoram (Thales Theoram)
View Solution
EXERCISE questions and complete solutions for chapter SAMPLE PAPER 8 of AGRAWAL PUBLICATION of Class 10