Complete Solutions to EXAMPLE of chapter TRIANGLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | TRIANGLES |
| AGRAWAL PUBLICATION | EXAMPLE |
| Number Of Questions | 76 |
| Academic Year | 2023-24 |
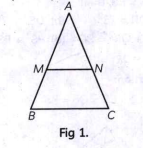
In the given figure, MN|| BC and AM : MB = 1 3, then `[ar(Delta AMN)]/[ar(triangle ABC)]`=………..
View SolutionIn a triangle ABC, AB=6 `sqrt 3` cm, AC = 12 cm and BC =6cm . Then measure of `angle B` is equal to :
एक त्रिभुज ABC में, AB=6 `sqrt 3` cm, AC = 12 cm और BC = 6cm| `angle B` का माप ज्ञात करे
View SolutionTwo triangles are similar if their corresponding sides are…………..
View SolutionA ladder 10 m long reaches a window 8 m above the ground. The distance of the foot of the ladder from the base of the wall is…………m.
View SolutionIf `triangle ABC` is an equilateral triangle of side 2a, then length of one of its altitude is………….
View SolutionThe perimeter of two similar triangles `triangle ABC` and `triangle PQR` are 35 cm and 45 cm respectively, then the ratio of the areas of the two triangles is…………..
View SolutionThe length of an altitude in an equilateral triangle of side 'a' cm is……………
View SolutionIf areas of two similar triangles are equal, then these triangles are………….
View SolutionDiagonals of a parallelogram separate it into two triangles of………
View SolutionIf S is a point on side PQ of a `trianglePQR` such that PS= QS= RS, then: `PR^2 + QR^2`=……...
View SolutionBC and BDE are two equilateral triangles such that D is the mid point of BC. Find the ratio of the areas of triangle ABC and BDE…………..
View SolutionIt is given that `triangle DEF ~ triangle RPQ`. Is it true to say that `angle D = angle R` and `angle F = angle P`?Why?
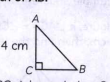
View SolutionIn the givne figure `triangle ABC` is an isosceles triangle right angled at C with AC = 4 cm. find the length of AB.
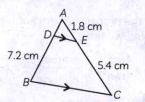
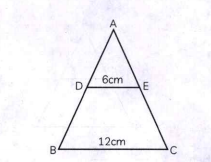
View SolutionIn the given figure, DE||BC. Find the length of side AD, given that AE = 1.8 cm, BD = 7.2 cm and CE = 5.4 cm.
View SolutionIs the following statement true?Why?
"Two quadrilaterals are similar, if their corrresponding angles are equal".
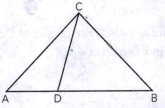
View SolutionIn the figure, if `angle ACB` = `angle CDA`, AC = 6 cm and AD = 3 cm, then find the length of AB.
View SolutionThe ratio of the corresponding altitudes of two similar triangles is `3/5`. Is it correct to say that the ratio of their areas is `6/5`?Why?
View SolutionFor a rhombus ABCD prove the following:
`4AB^2 = AC^2 + BD^2`.
View SolutionThe area of two similar triangles are 25 sq. m and 121 sq. cm. find the ratio of their corresponding sides.
View SolutionIn the given figure, if `angle D = angle C`, then it is true that `triangle ADE ~ triangle ACB`?Why?
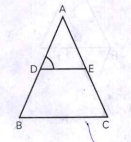
View SolutionA and B are respectivley the points on the sides PQ and PR of a `triangle PQR` such that PQ = 12.5 cm, PA = 5 cm, BR = 6 cm and PB = 4 cm is AB||QR? Give reasons for your answer.
View Solution`DeltaABC and DeltaDEF` are similar and their areas be respectively `64cm^(2)` and `121cm^(2)`. If `EF=15.4cm`, BC is.
View SolutionIn the given figure `DE||BC`, AD = 1 cm and BD = 2 cm. what is the ratio of the area `(triangle ABC)` to the area `(triangle ADE)`?
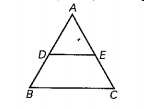
View SolutionIs the triangle with sides 25 cm, 5 cm and 24 cm a right triangle? Give reasons for your answer.
View SolutionThe perimeter of two similar triangles are 30cm and 20cm respectively. If one side of the first triangle is 9 cm. Determine the corresponding side of the second triangle.
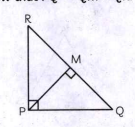
View SolutionIn the figure, `triangle PQR` is right angled at P. M is point on QR such that PM is perpendicular to QR. Show that `PQ^2= QM xx QR`.
View SolutionIn triangles `triangle PQR` and `triangle MST`, `angle P = 55^@`, `angle Q = 25^@`, `angle M = 100^@` and `angle S = 25^@`. Is `triangle QPR ~ triangle TSM`?Why?
View SolutionIn the figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that:
`[area(Delta ABC)]/[area(Delta DBC)]` = `(AO) /( DO)`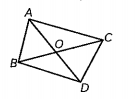
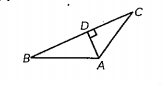
View SolutionIf `AD bot BC`, then prove that `AB^2 + CD^2 = BD^2 + AC^2`.
View SolutionTwo sides and the perimeter of one triangle are respectivley three times the corresponding sides and the perimeter of the other triangle. Are the two triangles similar?Why?
View SolutionIf in two right triangles, one of the acute angles of one triangle is equal to an acute angle of the other triangles, can you say that two triangles will be similar?Why?
View SolutionD is a point on side QR of `triangle PQR` such that `PD bot QR`. Will it be correct to say that `triangle PQD ~ triangle RPD`?Why?
View SolutionIn the figure, `DE||AC` and `DC||AP`. Prove that `(BE)/(EC)= (BC)/(CP)`
View SolutionIs it true to say that if in two triangles, an angle of one triangle is equal to an angle of another triangle and two sides of one triangle are proportional to the two sides of the other triangle, then the triangles are similar?Give reason for your answer.
View SolutionIn an equilateral triangle, prove that three times the square of one side is equal to four times the square of one of its altitudes.
View SolutionKitchen garden of Ms. Sanjana is in the form of a triangle as shown. She wants to divide it in two parts, one triangle and one trapezium.
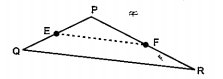
She takes PE = 4 m, QE = 4.5m PF = 8 m and RF = 9 m.
Is `EF||QR`?Justify your answer.
View SolutionTwo spotlights, P and Q are mounted on a verticl pole AB as shown.
Light beams from P and Q shine to two points on the ground,
H and K respectively, given that PQ = 16 m, KB = 16 m
PH = 35 m and QK = 20m find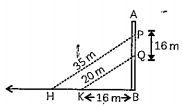
BQ, the height above the ground at which the spotlight Q is mounted.
View SolutionTwo spotlights, P and Q are mounted on a verticl pole AB as shown.
Light beams from P and Q shine to two points on the ground,
H and K respectively, given that PQ = 16 m, KB = 16 m
PH = 35 m and QK = 20m find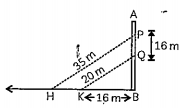
HK, the distance between the projections of the light beams.
View Solution`triangle ABC ~ triangle DEF` such that DE =3 cm, EF = 2 cm, DF = 2.5 cm and BC = 4 cm, find the perimeter of `triangle ABC`
View SolutionP and Q are the points on the sides DE and DF of a triangle DEF such that DP = 5 cm, DE = 15 cm, DQ = 6 cm and QF = 18 cm. Is `PQ||EF`?Give reasons for your answer.
View SolutionGiven that `triangle PQR` is similar to `triangle BAR`, find:
the value of y,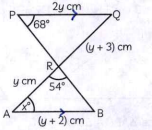
View SolutionIn the given figure, find the value of x in terms of a,b and c.
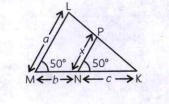
View SolutionR and S are points on the sides DE and EF respectively of a `triangle DEF` such that ER = 5 cm, RD = 2.5 cm, SE = 1.5 cm and FS = 3.5 cm. Find whether `RS||DF` or not.
View SolutionProve that the sum of the squares of the sides of a rhombus is equal to the sum of the squares of its diagonals.
View SolutionAreas of two similar triangles are `36 cm^2` and `100 cm^2`. If the length of a side of the larger triangle is 20cm. Find the length of the corresponding side of the smaller triangle.
View SolutionIn `triangle ABC` ~ `triangle DEF`, AB = 4 cm, DE =6cm , EF = 9 cm and FD = 12 cm, then find the perimeter of `triangle ABC`.
View SolutionIn the figure if `triangle ABC ~ triangle DEF` and their sides of lengths (in cm) are marked along them, them find the lengths of sides of each triangle.
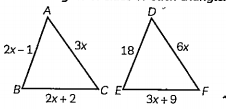
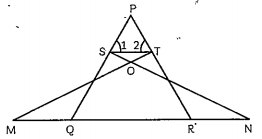
View SolutionIn the figure, if `angle 1` = `angle 2` and `triangle NSQ` = `triangle MTR`, then prove that `triangle PTS ~ triangle PRQ`.
View SolutionThree `30^@ - 60^@ - 90^@` set squares are together as shown in the diagram.
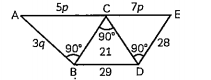
Find the value of P.
View SolutionThree `30^@ - 60^@ - 90^@` set squares are together as shown in the diagram.
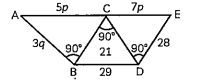
Find the value of length AB.
View SolutionIn the figure, `angle D` = `angle E` and `(AD)/(DB)=(AE)/(EC)`, prove that BAC is an isosceles triangle.
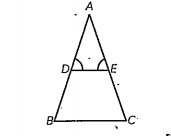
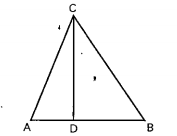
View SolutionIn `triangle ABC`, `angle B` = `90^@` and D is the mid point of BC. Prove that `AC^2` = `AD^2 + 3CD^2`.
View SolutionDiagonals of a trapezium PQRS intersect each other at the point O, `PQ||RS` and PQ = 3 Rs. Find the ratio of the areas of `triangle POQ` and `triangle ROS`.
View SolutionIn the figure, if `AB||DC` and AC, PQ intersect each other at the point O, prove that OA.CQ = OC.AP
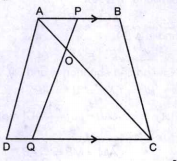
View SolutionIn the given figure, if `DE||BC`, then find the ratio of `ar(Delta ADE)` and `ar(DECB)`.
View SolutionABCD is a trapezium in which `AB||DC` and P, Q are points on AD and BC respectively, such that `PQ||DC`, if PD = 18 cm, BQ = 35 cm and QC = 15 cm, find AD.
View SolutionTwo right triangles ABC and DBC are drawn on the same hypotenuse BC and on the same side of BC. If AC and BD intersect at P, prove that `AP xx PC` = `BP xx DP`.
View SolutionDiagonals of a trapezium PQRS intersect each other at the point O, `PQ||RS` and PQ = 3 Rs. Find the ratio of the areas of `triangle POQ` and `triangle ROS`.
View SolutionIn the given figure, if `angle ACB` = `angle CDA`, AC = 8 cm and AD = 3 cm, then find BD.
View SolutionA 15 metres high tower coasts a shadow 24 metres long at a certain time and at the same time, a telephone pole casts a shadow 16 metres long. Find the height of the telephone pole.
View SolutionIf P and Q are the points on side CA and CB, respectively of `triangle ABC`, right angled at C, prove that `(AQ^2 + BP^2)` = `(AB^2 + PQ^2)`.
View SolutionIf the area of two similar triangles are equal, prove that they are congrent.
View SolutionThere is a circular park of radius 24 m and there is a pole at a distance of 26 m from the centre of the park as shown in the figure. It is planned to enclose the park by planting trees along line segments PQ and PR tangential to the park.
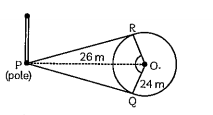
Find the length of PQ and PR,
View SolutionThere is a circular park of radius 24 m and there is a pole at a distance of 26 m from the centre of the park as shown in the figure. It is planned to enclose the park by planting trees along line segments PQ and PR tangential to the park.
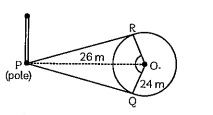
If six trees are to be planted along each tangential line segments at equal distances, find the distance between any two consecutive trees.
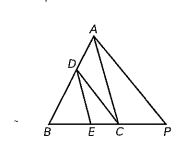
View SolutionD, E and F are respectively the mid points of the sides AB, AC and BC of triangle ABC respectively. Find the ratio of areas of triangle DEF and triangle ABC.
View SolutionA flag pole 18 m high casts a shadow 9.6m long. Find the distance of the top of the pole from the far end of the shadow.
View SolutionIf a line is drawn parallel to one side of a triangle to intersect other two sides in distinct points, then prove that the other two sides are divided in the same ratio.
View SolutionIn the given figure, if PQRS is a parallelogram and `AB||PS`, then prove that `OC||SR`.
View Solution`triangle ABC` figure, `AD bot BC`. Prove that `AC^2` = `AB^2 + BC^2 - 2BC xx BD`
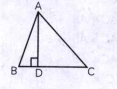
View SolutionProve that in a right angle triangle, the square of the hypotenuse is equal to the sum of squares of the other two sides.
View SolutionFor going to city B from city A, there is a route via city C such that `AC bot CB`, `AC = 2x km` and CB = 2(x + 7) km. It is proposed to construct a 26 km highway which directly connects the two cities A and B.Find how much distance will be saved in reaching city B from city A aftr the construction of the highway.
View SolutionIn `triangle PQR`, `PD bot QR` such that D lies on QR. If PQ = a, PR = b, QD = c and DR = d, prove that (a + b)(a - b)= (c + d)(c - d).
View SolutionIn an equilateral `triangle ABC`, D is a point on side BC such that BD = `1/3 BC`. Prove that `9(AD)^2` = `7(AB)^2`.
View SolutionIn the given figure,`l||m` and line segments AB, CD and EF are concurrent at point P.
Prove that `(AE)/(BF)=(AC)/(BD)=(CF)/(FD)`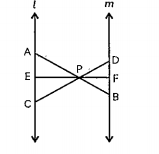
View SolutionIn the given figure, PA, QB, RC and SD are all perpendiculars to a line 'l'.AB = 6 cm, BC = 9 cm, CD = 12 cm and SP = 36 cm. Find the PQ, QR and RS.
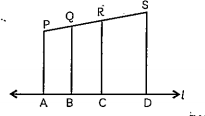
View SolutionProve that the area of the semicircle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the semicircles drawn on the other two sides of the triangle.
View Solution
EXAMPLE questions and complete solutions for chapter TRIANGLES of AGRAWAL PUBLICATION of Class 10