Complete Solutions to EXERCISE 20 (A) of chapter CYLINDER, CONE AND SPHERE of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | CYLINDER, CONE AND SPHERE |
| SELINA PUBLICATION | EXERCISE 20 (A) |
| Number Of Questions | 36 |
| Academic Year | 2023-24 |
The height of a circular cylinder is 20 cm and the radius of its base is 7 cm. Find :
the volume
View SolutionThe height of a circular cylinder is 20 cm and the radius of its base is 7 cm. Find :
the total surface area.
View SolutionThe inner radius of a pipe is 2.1 cm. How much water can 12 m of this pipe hold ?
View SolutionA cylinder of circumference 8 cm and length 21 cm rolls without sliding for `4 (1)/(2) ` seconds at the rate of 9 complete rounds per second. Find :
the distance travelled by the cylinder in `4 (1)/(2)` seconds ?
View SolutionA cylinder of circumference 8 cm and length 21 cm rolls without sliding for `4 (1)/(2) ` seconds at the rate of 9 complete rounds per second. Find :
the area covered by the cylinder in `4 (1)/(2)` seconds.
View SolutionHow many cubic metres of earth must be dug out to make a well 28 m deep and 2.8 m in diameter ? Also, find the cost of plastering its inner surface at Rs 4.50 per sq. metre.
View SolutionWhat length of solid cylinder 2 cm in diameter must be taken to recast into a hollow cylinder of external diameter 20 cm, 0-25 cm thick and 15 cm long?
View SolutionA cylinder has a diameter of 20 cm. The area of the curved surface is 100 sq cm. Find the height of the cylinder correct to one decimal place.
View SolutionA cylinder has a diameter of 20 cm. The area of the curved surface is 100 `cm^( 2)`. Find the volume of the cylinder (in. `cm^( 3)`).
View SolutionA metal pipe has a bore (inner diameter) of 5 cm. The pipe is 5 mm thick all round. Find the weight, in kilogram, of 2 metres of the pipe if `1 cm ^(3)` of the metal weighs 7.7 g.
View SolutionA cylindrical container with diameter of base 42 cm contains sufficient water to submerge a rectangular solid of iron with dimensions `22 cm xx 14 cm xx 10.5 cm.` Find the rise in level of the water when the solid is submerged.
View SolutionA cylindrical container with internal radius of its base 10 cm, contains water up to a height of 7 cm. Find the area of the wet surface of the cylinder.
View SolutionFind the total surface area of an open pipe of length 50 cm, external diameter 20 cm and internal diameter 6 cm.
View SolutionThe height and the radius of the base of a cylinder are in the ratio 3: 1. If its volume is 1029 `pi cm^(3),` find its total surface area.
View SolutionThe radius of a solid right circular cylinder increases by 20% and its height decreases by 20%. Find the percentage change in its volume.
View SolutionThe radius of a solid right circular cylinder decreases by 20% and its height increases by 10%. Find the percentage change in its :
volume
View SolutionThe radius of a solid right circular cylinder decreases by 20% and its height increases by 10%. Find the percentage change in its :
curved surface area
View SolutionFind the minimum length in cm and correct to nearest whole number of the thin metal sheet required to make a hollow and closed cylindrical box of diameter 20 cm and height 35 cm. Given that the width of the metal sheet is 1 m. Also, find the cost of the sheet at the rate of Rs 56 per sq m.
Find the area of metal sheet required, if 10% of it is wasted in cutting, overlapping, etc.
View Solution3080 `cm^(3)` of water is required to fill a cylindrical vessel completely and 2310 `cm ^(3)` of water is required to fill it upto 5 cm below the top. Find :
radius of the vessel.
View Solution3080 `cm^(3)` of water is required to fill a cylindrical vessel completely and 2310 `cm ^(3)` of water is required to fill it upto 5 cm below the top. Find :
height of the vessel.
View Solution3080 `cm^(3)` of water is required to fill a cylindrical vessel completely and 2310 `cm ^(3)` of water is required to fill it upto 5 cm below the top. Find :
wetted surface area of the vessel when it is half-filled with water.
View SolutionFind the volume of the largest cylinder formed when a rectangular piece of paper 44 cm by 33 cm is rolled along its shorter side.
View SolutionFind the volume of the largest cylinder formed when a rectangular piece of paper 44 cm by 33 cm is rolled along its longer side.
View SolutionA metal cube of side 11 cm is completely submerged in water contained in a cylindrical vessel with diameter 28 cm. Find the rise in the level of water.
View SolutionA circular tank of diameter 2 m is dug and the earth removed is spread uniformly all around the tank to form an embankment 2 m in width and 1.6 m in height. Find the depth of the circular tank.
View SolutionThe sum of the inner and the outer curved surfaces of a hollow metallic cylinder is `1056 cm ^(2)` and the volume of material in it is `1056 cm^(3).` Find its internal and external radii. Given that the height of the cylinder is 21 cm.
View SolutionThe difference between the outer curved surface area and the inner curved surface area of a hollow cylinder is `352 cm^(2).` If its height is 28 cm and the volume of material in it is `704 cm^(3),` find its external curved surface area.
View SolutionThe sum of the height and the radius of a solid cylinder is 35 cm and its total surface area is `3080 cm^(2),` find the volume of the cylinder.
View SolutionThe total surface area of a solid cylinder is `616 cm^(2).` If the ratio between its curved surface area and total surface area is 1 : 2, find the volume of the cylinder.
View SolutionA cylindrical vessel of height 24 cm and diamater 40 cm is full of water. Find the exact number of small cylindrical bottles, each of height 10 cm and diameter 8 cm, which can be filled with this water.
View SolutionTwo solid cylinders, one with diameter 60 cm and height 30 cm and the other with radius 30 cm and height 60 cm, are melted and recasted into a third solid cylinder of height 10 cm. Find the diameter of the cylinder formed.
View SolutionThe total surface area of a hollow cylinder, which is open from both the sides, is 3575 cm", area of its base ring is `357.5 cm^(2)` and its height is 14 cm. Find the thickness of the cylinder.
View SolutionThe given figure shows a solid formed of a solid cube of side 40 cm and a solid cylinder of radius 20 cm and height 50 cm attached to the cube as shown. Find the volume and the total surface area of the whole solid [Take `pi = 3.14]`
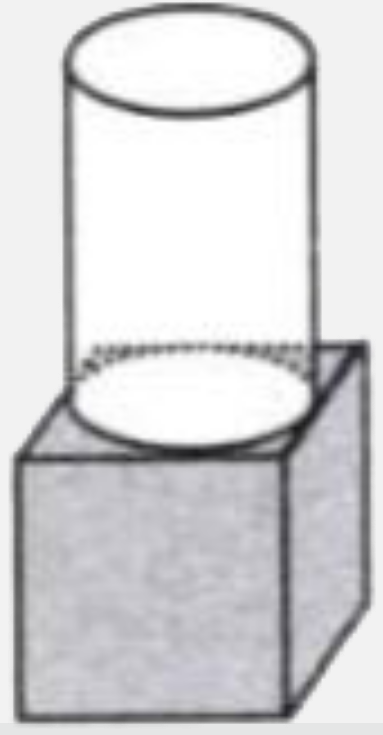
View SolutionTwo right circular solid cylinders have radii in the ratio 3 : 5 and heights in the ratio 2:3. Find the ratio between their curved surface areas.
View SolutionTwo right circular solid cylinders have radii in the ratio 3 : 5 and heights in the ratio 2:3. Find the ratio between their volumes.
View SolutionA closed cylindrical tank, made of thin iron sheet, has diameter = 8.4 m and height 5.4 m. How much metal sheet, to the nearest `m^(2),` is used in making this tank, if `(1)/(15)` of the sheet actually used was wasted in making the tank ?
View Solution
EXERCISE 20 (A) questions and complete solutions for chapter CYLINDER, CONE AND SPHERE of SELINA MATHS (ENGLISH) of Class 10