Complete Solutions to Exercise (Subjective Problems) (Long Answer Type) of chapter TRIANGLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | TRIANGLES |
| MTG IIT JEE FOUNDATION | Exercise (Subjective Problems) (Long Answer Type) |
| Number Of Questions | 7 |
| Academic Year | 2023-24 |
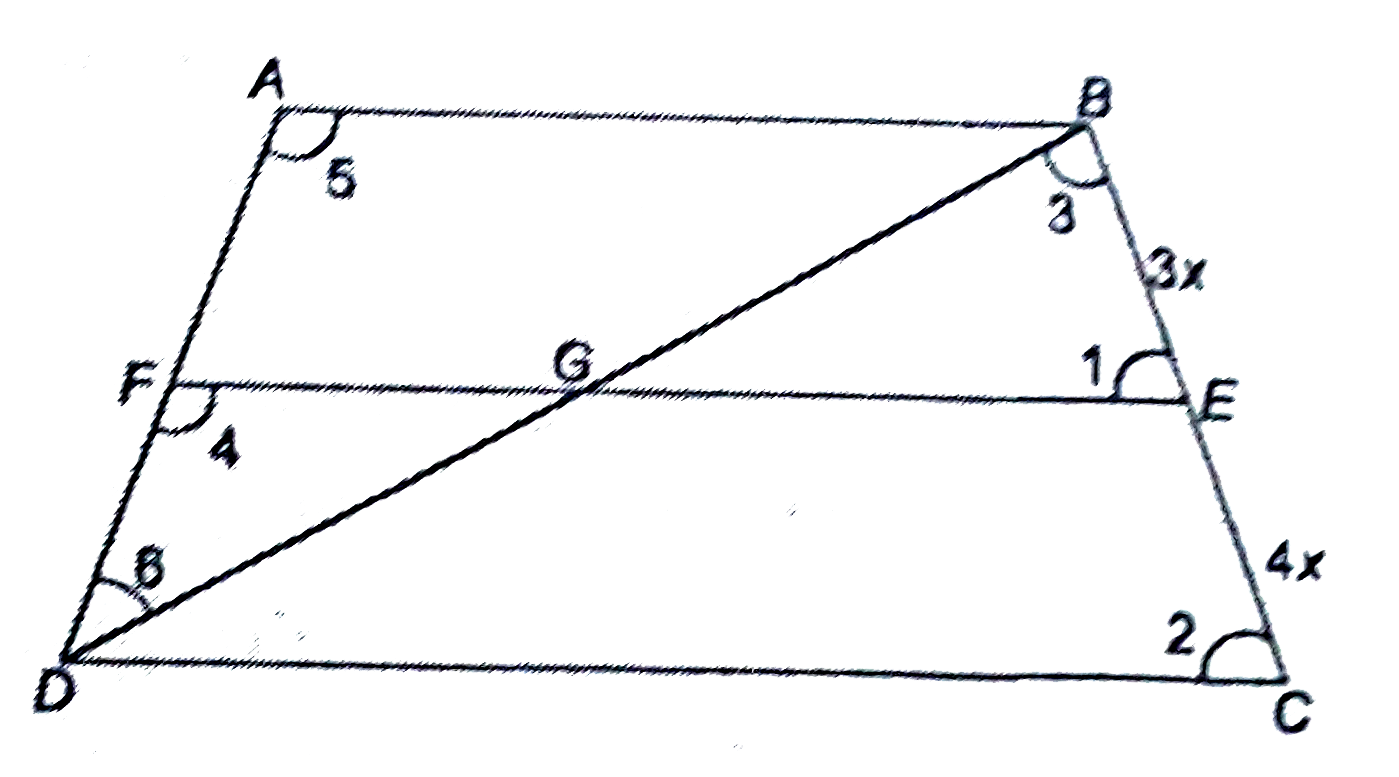
In trapezium ABCD. AB||DC and DC = 2AB. A line segment EF drawn parallel to AB cuts AD in F and BC in E such that `(BE)/(EC)=3/4` .
Diagonal DB intersects EF at G. prove that &EF= 10AB.
View SolutionIn a right triangle ABC , right angled at C, P and Q are the points of the sides CA and CB respectively , which divide these sides in the ratio `2 : 1` Prove that
`9AQ^(2)=9AC^(2)+4BC^(2)`
View SolutionIn a right triangle ABC , right angled at C, P and Q are the points of the sides CA and CB respectively , which divide these sides in the ratio `2 : 1` Prove that
`9BP^(2)=9BC^(2)+4AC^(2)`
View SolutionIn a right triangle ABC , right angled at C, P and Q are the points of the sides CA and CB respectively , which divide these sides in the ratio `2 : 1` Prove that
`9(AQ^(2)+BP^(2))=13AB^(2)`
View SolutionTwo poles of height a metres and `b` metres are `p` metres apart. Prove that the height of the point of intersection of the lines joining the top of each pole to the foot of the opposite pole is given by `(a b)/(a+b)` metres.
View SolutionProve that the sum of the squares of the diagonals of parallelogram is equal to the sum of the squares of its sides.
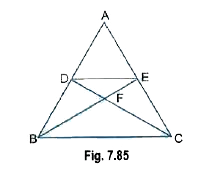
View SolutionIn Fig. 7 .85, `DEabs()BC` and AD : DB = 5 : 4. Find `("Area"(DeltaDEF))/("Area"(DeltaCFB))`
View Solution
Exercise (Subjective Problems) (Long Answer Type) questions and complete solutions for chapter TRIANGLES of MTG IIT JEE FOUNDATION of Class 10
All Exercises from MTG IIT JEE FOUNDATION Class 10 MATHS TRIANGLES
- Exercise (Assertion & Reason Type)
- Exercise (Comprehension Type)
- Exercise (Integer Numerical Value Type)
- Exercise (Match the following )
- Exercise (Multiple Choice Questions) (LEVEL -1 )
- Exercise (Multiple Choice Questions) (LEVEL -2)
- Exercise (Subjective Problems) (Long Answer Type)
- Exercise (Subjective Problems) (Short Answer Type)
- Exercise (Subjective Problems) (Very Short Answer Type)
- ILLUSTRATIONS
- NCERT Section Exercise 6.1
- NCERT Section Exercise 6.2
- NCERT Section Exercise 6.3
- NCERT Section Exercise 6.4
- NCERT Section Exercise 6.5
- NCERT Section Exercise 6 .6
- Olympiad /HOTS Corner
- SOLVED EXAMPLES