Complete Solutions to Exercise (Multiple Choice Questions) (LEVEL -1 ) of chapter TRIANGLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | TRIANGLES |
| MTG IIT JEE FOUNDATION | Exercise (Multiple Choice Questions) (LEVEL -1 ) |
| Number Of Questions | 35 |
| Academic Year | 2023-24 |
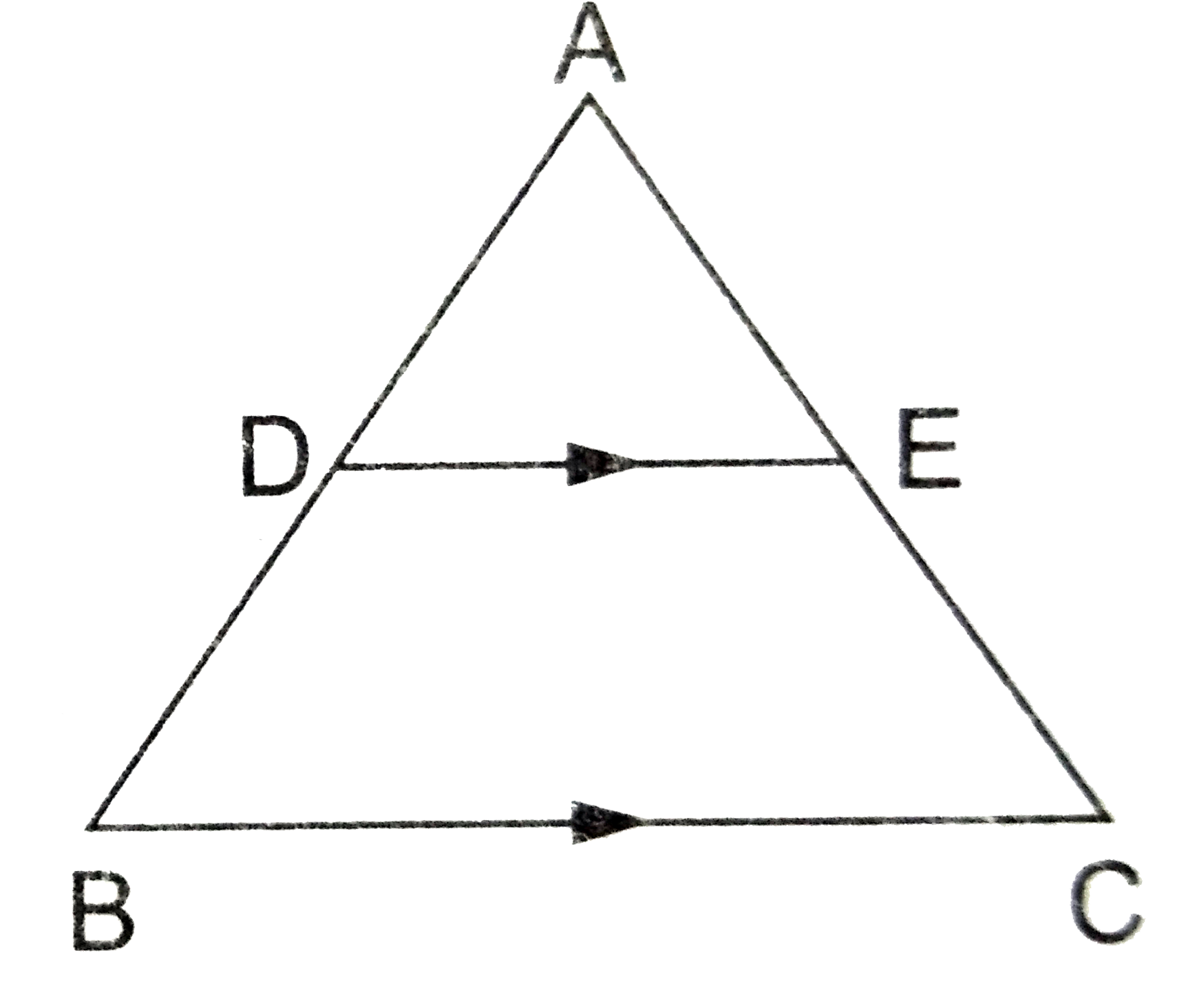
In `Delta ABC,DE||BC` so that `AD==2.4 cm, AE= 3.2 cm and EC=4.8 cm`, then , AB= ?
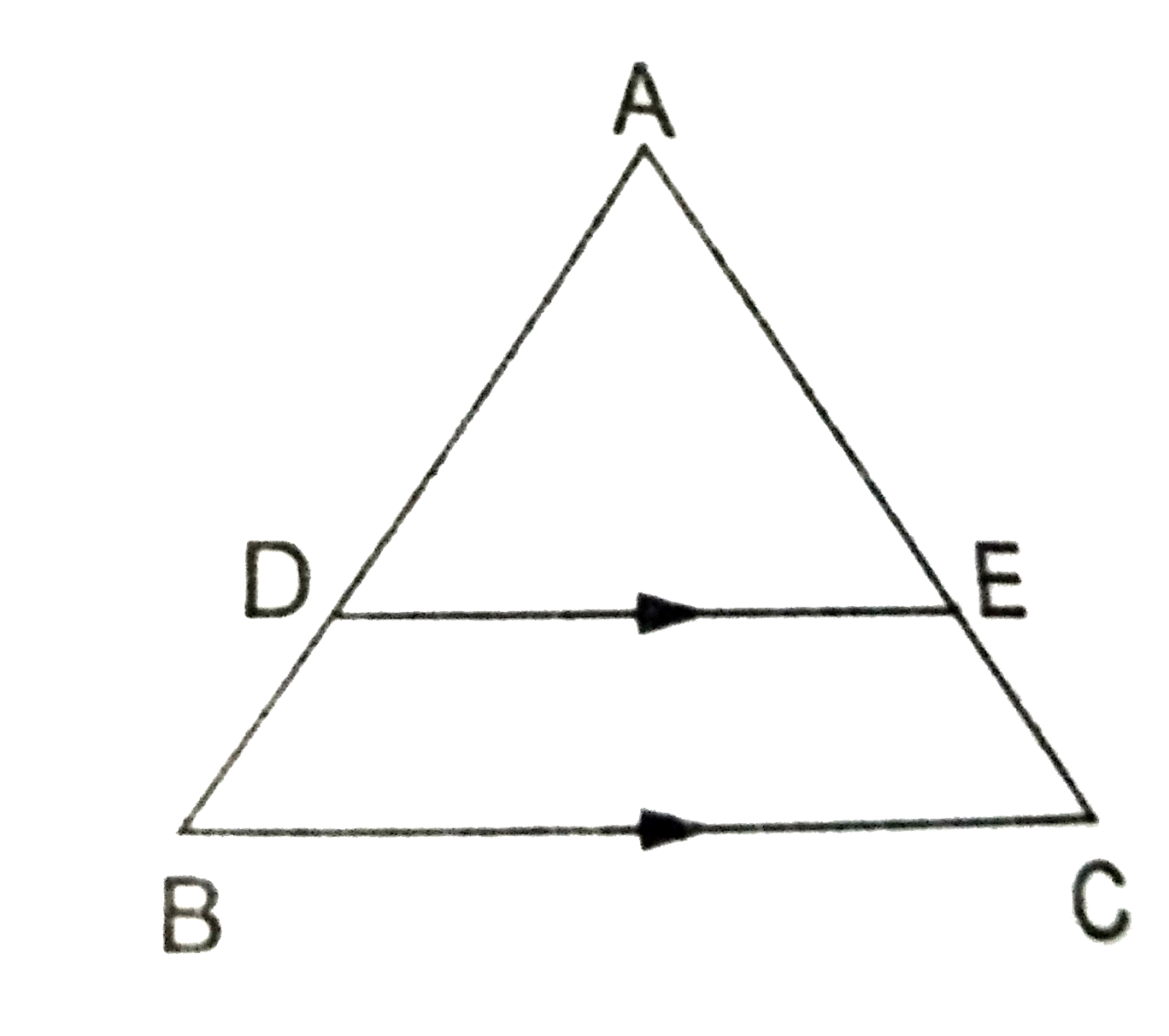
View SolutionIn `Delta ABC, DE||BC` so that `AD=(7x-4) cm, AE=(5x-2) cm, DB=(3x+4) cm and EC=3x`. Then, we have
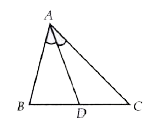
View SolutionIn `DeltaABC,AD` is the inernal besector of `angleA`. If BD = 5cm , BC=7.5 cm , then AB:AC=
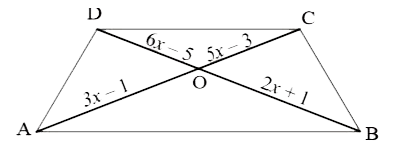
View SolutionIn the given fig., AB || DC and diagonals AC and BD intersects at O. If OA =3x-1 and OB =2x + 1, OC = 5x-3 and OD =6x-5, find the value of x.
View SolutionIn `Delta ABC` it is given that `(AB)/(AC)=(BD)/(DC)` . If `angle B=70^(@) and angle C=50^(@) "then" angle BAD=?`
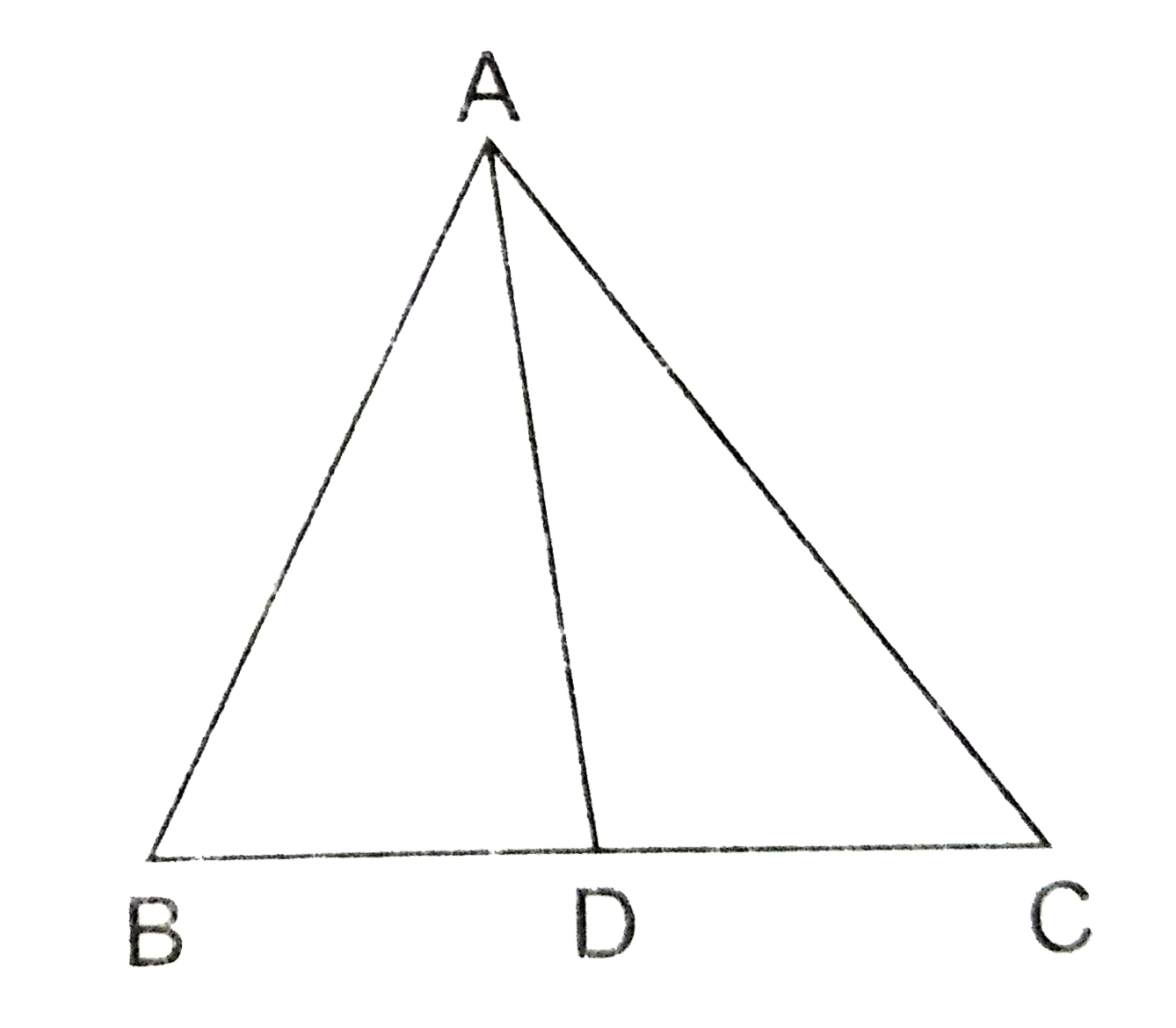
View SolutionIn `DeltaABC,AD` is the bisector of `angleA` . Then , `(ar(DeltaABD))/(ar(DeltaACD))=`
View Solution`Delta ABC~Delta DEF` and the perimeters of `Delta ABCd and Delta DEF` are 30 cm and 18 cm respectively. If `BC=9 cm`, then EF=?
View Solution`DeltaABC ~ DeltaDEF` such that AB = 9.1 cm and DE =6.5 cm. If the perimeter of `DeltaDEF` is 25 cm, what is the perimeter of `DeltaABC`?
View SolutionA vertical stick 20 m long casts a shadow 10m long on the ground. At the same time, a tower casts a shadow 50m long on the ground. The height of the tower is (a) 100m (b) 120m (c) 25m (d) 200m
View SolutionTwo isosceles triangles have equal angles and their areas are in the ratio `36 : 81` .The ratio of their corrersponding heights is
View SolutionIf ` A B C` and ` D E F` are similar such that `2\ A B=D E` and `B C=8c m` , then `E F=` (a) 16cm (b) 12cm (c) 8cm (d) 4cm.
View SolutionIn ` A B C` , a line `X Y` parallel to `B C` cuts `A B` at `X` and `A C` at `Y` . If `B Y` bisects `/_X Y C` , then `B C=C Y` (b) `B C=B Y` (c) `B C!=C Y` (d) `B C!=B Y`
View SolutionLet ABC be an equilateral triangel. Let `BE_|_CA` meeting CA at E, then `(AB^(2)+BC^(2)+CA^(2))` is equal to :
View SolutionA right triangle has hypotenuse of length `p\ c m` and one side of length `q\ c m` . If `p-q=1` , find the length of the third side of the triangle.
View SolutionABC is an isosceles triangle with AC = BC. If `A B^2=2A C^2`, prove that ABC is a right triangle.
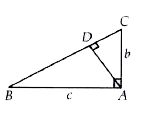
View Solution`DeltaABC` is a right triangle , right angled at A and `ADbotBC` . If AB= c and AC = b, then AD is equal to
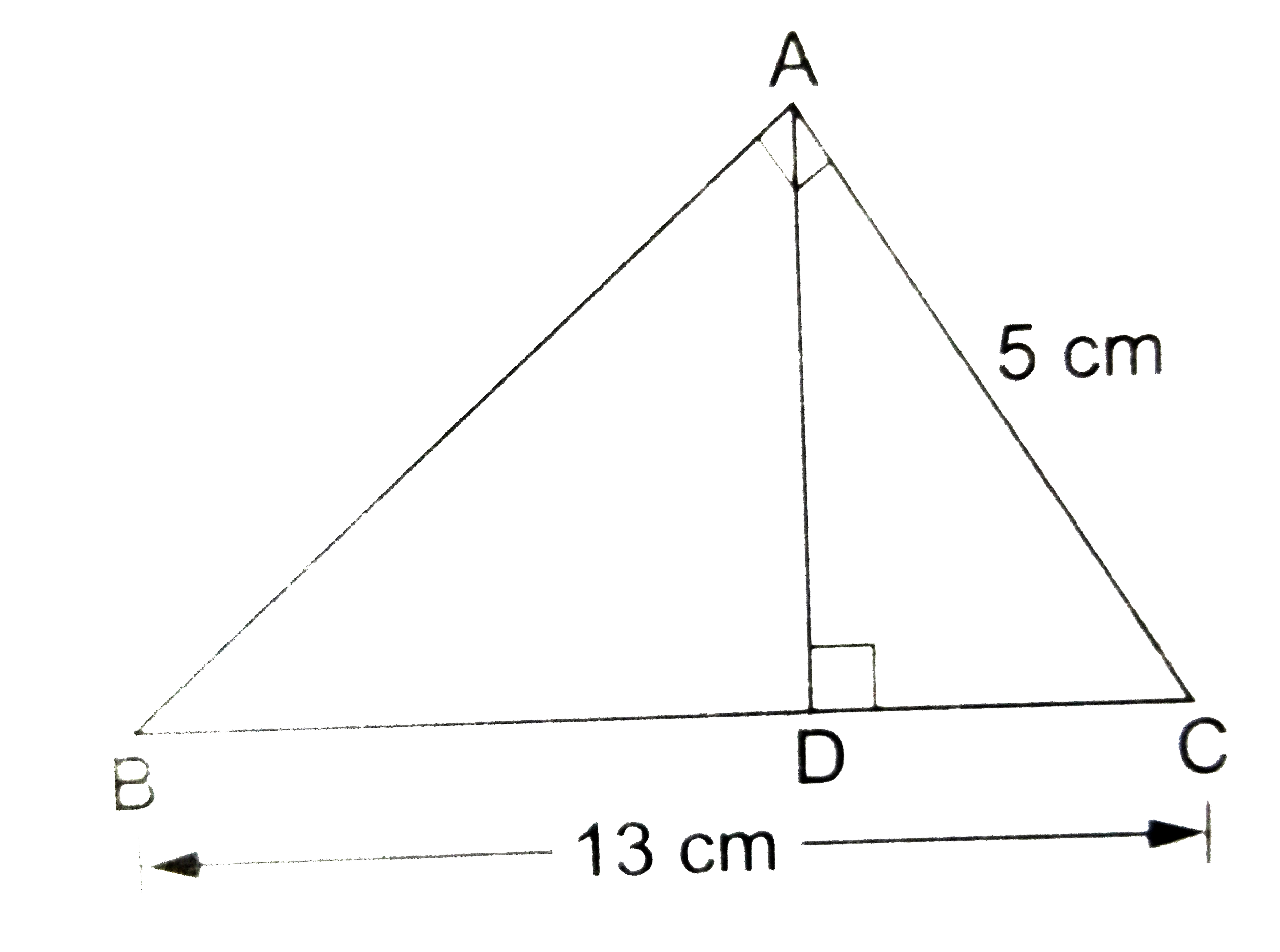
View Solution`Delta ABC` is right-angled at A and `AD bot BC`. If `BC=13 cm and AC=5 cm`, find the ratio of areas of `Delta ABC and Delta ADC`.
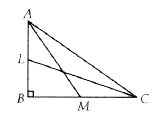
View SolutionL and M are the mid points of AB and BC respectively of `DeltaABC` , right angled at B . `4LC^(2)=`
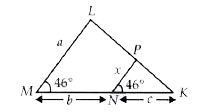
View SolutionIn the given figure , x in terms of a , b and c is
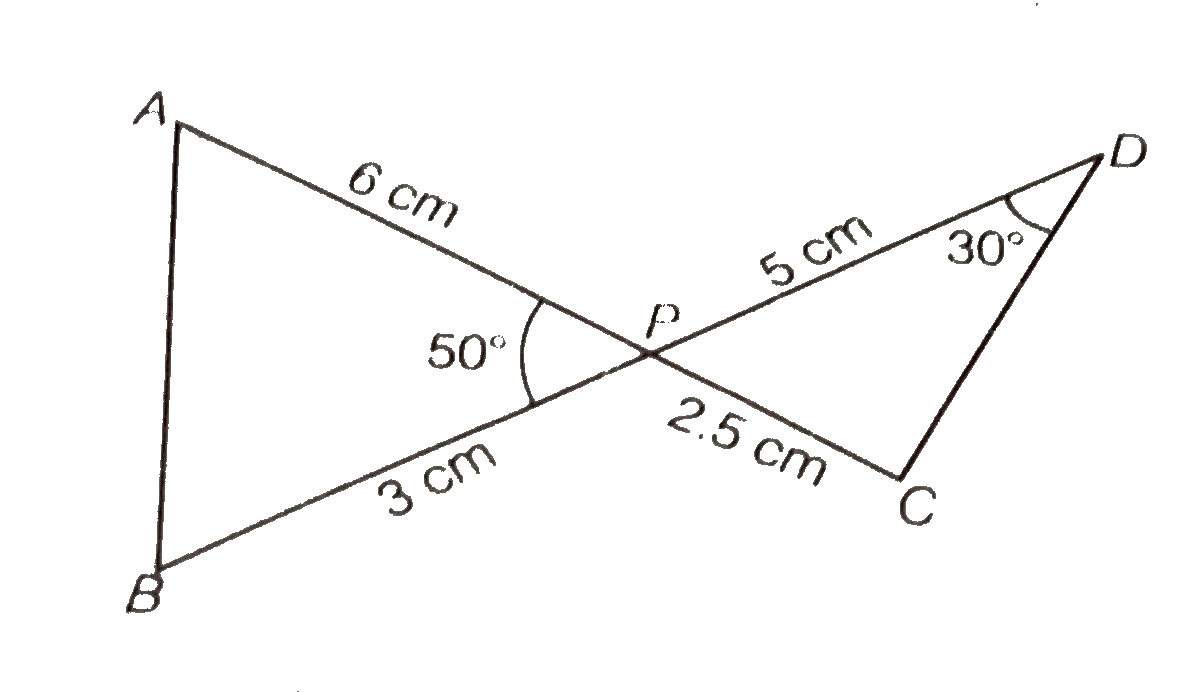
View SolutionIn figure, two line segments `AC` and `BD` intersects each other at the point `P` such that `PA` = 6 cm, `PB` = 3 cm, `PC` = 2.5 cm, `PD`=5 cm, `angleAPB=50^(@)` and `angleCDP=30^(@)`. Then, `anglePBA` is equal to
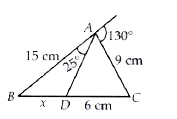
View SolutionIn the given figure , value of x is
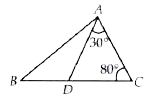
View SolutionIn the given figure , if `(AB)/(AC)=(BD)/(CD)` , then `angleABD=`
View SolutionThe point in the plane of a triangle which is at equal perpendicular distance from the sides of the triangle is :
View SolutionPSR is a triangle right angled at s . D is the mid -point of SR .If the bisector of `anglePSR` and perpendicular bisector of SR meet at O , then `DeltaOSD` is
View SolutionAn isosceles triangle has a 10 inches base and two 13 inches sides .What other value can the base have and still yields a triangle with the same areas ?
View SolutionIf the ratio of the perimeter of two similar triangles is 4:25, then find the ratio of the areas of the similar triangles.
View SolutionIn `DeltaABC,angleB=90^(@)andBDbotAC`. If DC=7 cm and AD = 3 cm , then the length of BD is
View SolutionIn which of the following options ,DE ||AB where D and E lie on the sides AC and BC of `DeltaABC` respectively ?
View SolutionIn the given figure , `DeltaABC~DeltaPQR` ,PM is median of `DeltaPQR` . If ar `(DeltaABC)=289cm^(2),BC=17cm,MR=6.5cm` , then the area of `DeltaPQM` is
View SolutionIn a rectangle ABCD , E is the mid -point of AB . If AB= 16 m and AD = 6 m , then find ED .
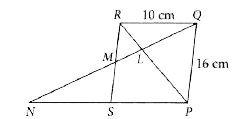
View SolutionIn the given figure , PQRS is a parallelogram with PQ = 16 cm and QR = 10 cm . L is a point on PR such that RL:LP=2:3QL is produced to meet RS at M and PS produced at N . Find the length of PN and RM (in cm ) respectively .
View SolutionThe perimeters of two similar triangles are 30 cm and 20 cm . If one altitude of the former triangle is 12 cm , then the length of the corresponding altitude of the latter triangle is
View SolutionThe length of the diagonal of a square is `7sqrt(2)` cm . Then , the area of the square in `cm^(2)` is
View SolutionA man goes 15 m due east and then 20 m due north .find his distance from the starting point .
View SolutionIn `DeltaABC `and `DeltaDEF`, if `/_A = 50^@,/_B = 70^@, /_C = 60^@, /_D = 60^@, /_E = 70^@ and /_F = 50^@`, then
View Solution
Exercise (Multiple Choice Questions) (LEVEL -1 ) questions and complete solutions for chapter TRIANGLES of MTG IIT JEE FOUNDATION of Class 10
All Exercises from MTG IIT JEE FOUNDATION Class 10 MATHS TRIANGLES
- Exercise (Assertion & Reason Type)
- Exercise (Comprehension Type)
- Exercise (Integer Numerical Value Type)
- Exercise (Match the following )
- Exercise (Multiple Choice Questions) (LEVEL -1 )
- Exercise (Multiple Choice Questions) (LEVEL -2)
- Exercise (Subjective Problems) (Long Answer Type)
- Exercise (Subjective Problems) (Short Answer Type)
- Exercise (Subjective Problems) (Very Short Answer Type)
- ILLUSTRATIONS
- NCERT Section Exercise 6.1
- NCERT Section Exercise 6.2
- NCERT Section Exercise 6.3
- NCERT Section Exercise 6.4
- NCERT Section Exercise 6.5
- NCERT Section Exercise 6 .6
- Olympiad /HOTS Corner
- SOLVED EXAMPLES