Complete Solutions to Exercise of chapter SOME APPLICATIONS OF TRIGONOMETRY of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | SOME APPLICATIONS OF TRIGONOMETRY |
| NAGEEN PRAKASHAN | Exercise |
| Number Of Questions | 32 |
| Academic Year | 2023-24 |
The length of the shadow of a pole at a time is `sqrt3`times its height.Find the sun's altitude
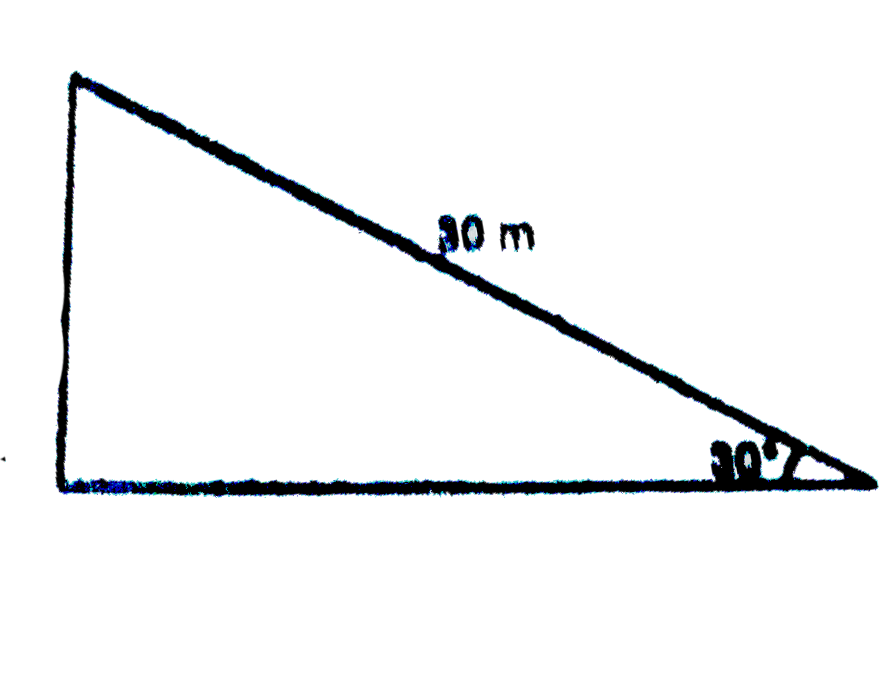
View SolutionA circus artist is climbing a 30 m rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole to the ground. Find the height of the angle made by the rope with the ground level is `30^@)`, as shown in the figure.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is `30^(@)`. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 25 m away from the foot of between the ship and tower.
View SolutionThe angle of depression of a ship from the top a tower of height 50 m is `30^(@)`. Find the horizontal distance between the ship and tower.
View SolutionThe angle of depression between a flying kite and 'a' point on the ground is 60 m. If the string makes `30^(@)`. Find the horizontal distance between the ship and tower.
View SolutionThe angle if depression of a ship from the top a tower of height 50 m is `30^(@)`. Find the horizontal distance between the boat and the bridge.
View SolutionThe upper part of a tree broken over by wind, makes an angle of `30^(@)` with the ground. If the length of the upper part is `8sqrt3` metresthen what was the height of the tree before broken ?
View SolutionIn a violent storm, a tree got bent by the wind. The top of the tree meets the ground at an angle of `30^(@)`, at a distance of 30 metres from the root. At what height from the bottom did the tree bent? What was the original height of the tree? (Use `sqrt3=1.73`)
View SolutionThe angle of elevation of the top of a tower from a point on the ground is `30^(@)`. After walking 45 m towards the tower, the angle of elevation becomes `45^(@)`. Find the height of the tower.
View SolutionThere are two points on the horizontal line passing through the foot of a tower in the same side of the tower. The angle of depression of these point from the top of the tower are `45^(@)" and "60^(@)` respectively. Find the distance between the two point if the height if the tower is 150 metres.
View SolutionFrom the top of a light house, the angles of depression of two ships on the opposite sides of its are observed to be `alphaa n dbeta` . If the height of the light house be h metres and the line joining the ships passes through the foot of the light house, show that the distance between the ship is `(h(tanalpha+tanbeta)/(tanalphat a nbeta)`
View SolutionFrom the top of a light-hours, the angles of depression of two ships on the opposite sides are observed to be `30^(@)" and "45^(@)`. If the height of the light-house, is 90 m and the line joining the two ships passes through the light- house, then find the distance between the ships.
View SolutionAn aeroplane, when 3000 m high, passes vertically above anthoer aeroplane at an instant when the angles of elevation of the two planes from the same point on the ground are `45^(@)" and "60^(@)` respectively. Find the vertical distance between the tow planes.
View SolutionThe angle of elevation of the top of am incomplete temple, at a point 30 m away from its foot, is `30^(@)`. How much more high the temple must ne constructed so that the angle of elevation of its top at the same point be `45^(@)`.
View SolutionThe angle of elevation of the top of an incomplete tower, at a point 40m away from its foot, is `45^(@)`. How much more high the tower must be constructed so that the angle of elevation of its top at the same point be `45^(@)`?
View SolutionOn a straight line passing through the foot of a tower, two points C and D are at distances of 4 m and 16 m from the foot respectively. If the angles of elevation from C and D of the top of the tower are complementary, then find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from the foot of a house, situated at a distance of 20 m from the tower is `60^(@)`. From the top of the top of the house the angle of elevation of the top of the tower os `45^(@)`. Find the height of house and tower.
View SolutionThere is a 7m high statue standing on a cliff. At a point P on the ground, the angle of elevation of the foot of the statue is `alpha`. After walking 34 metres towords the cliff from that point, the angle of elevation of the top of the statue is `(90^(@)-alpha)`, find the height of the cliff if `tanalpha=1/2`.
View SolutionThe distance between two towers is 140 m while seeing from the top if the second tower, the angle of elevation of first tower is `30^(@)`.If the height of the second tower is 60 m, then find the height of the first tower.
View SolutionA temple and a flagstaff surmounted at its top, each subtends equal angle of `30^(@)` at a point on the ground. If the height of the temple is 10 m, find the height of the flagstaff.
View SolutionA 7 m long flagstaff is fixed on the top of a tower on the horizontal plane. From a point on the ground, the angles of elevation of the top and bottom of the flagstaff are `45^(@)" and " 30^(@)` respectively. Find the height of the tower.
View SolutionAt one side of a road, there os a house and on the other side there is a tower. House and tower are on the same horizontal plane. The angle of depression of the top and foot of the house, from the top of the tower are `30^(@)" and "45^(@)` respectively. If the height of the house is 10 m, then find the distance between the house and the tower.
View SolutionA tower subtends an angle of `60^(@)` at a point on the plane passing through its foot and at a point 20 m vertically above the first point, the angle of depression of the foot of tower is `45^(@)`. Find the height of the tower.
View SolutionAn aeroplane is flying over two houses which are at a distance of 300 m from each other. If at some moment from the aeroplane the angle of depression of two houses on the same side are `45^(@)" and "60^(@)`, at what height the plane is flying ?
View SolutionFrom the top of a 96 m tower, the angles of depression of two cars, on the same side of the tower are `alpha" and "beta`respectively. If `tanalpha=1/4" and "tanbeta=1/7`, then find the distance between two cars.
View SolutionThe angle of elevation of the top of a vertical tower, from a point in the horizontal plane passing through the foot of the tower is `theta`. On moving towards the tower 192 metres, the angle of elevation becomes `phi`. If `tan theta=5/12" and "thephi=3/4`, then find the height of the tower.
View SolutionThe upper part of a tree broken over by the wind makes an angle of `60^(@)` with the ground and the horizontal distance from the foot of the tree to the point where the top of the tree meets the ground is 10 metres. Find the height of the tree before broken.
View SolutionFrom a boat, which is moving towards a bridge, the angle of elevation of bridge is `30^(@)`. After 10 minutes, the angle of elevation becomes `60^(@)`. Find how much more time will take the boat to reach at the bridge?
View SolutionThe angle of elevation of the top of a building from the foot of the tower is `60^(@)`. After a flight of 15 seconds, the angle of elevation changes to `30^(@)`. If the aeroplane is flying at a constant height of `1500sqrt3 `m find the speed of the plane in km/hr.
View SolutionThe angle of elevation of the top of a building from the foot of the tower is `30^(@)` and the angle of elevation of the top of the tower from the foot of the building is `45^(@)`. If the tower is 30 m high, find the height of the building.
View SolutionA flagstaff on the top of tower 80 m high, subtends an angle `tan^(-1)(1/9)` at point on the ground 100 m from the tower. The height of flagstaff is
View Solution
Exercise questions and complete solutions for chapter SOME APPLICATIONS OF TRIGONOMETRY of NAGEEN MATHS (HINGLISH) of Class 10