Complete Solutions to ACHIEVERS SECTION of chapter SOF INTERNATIONAL MATHEMATICS OLYMPIAD QUESTION PAPER 2020 of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | SOF INTERNATIONAL MATHEMATICS OLYMPIAD QUESTION PAPER 2020 |
| SCIENCE OLYMPIAD FOUNDATION | ACHIEVERS SECTION |
| Number Of Questions | 4 |
| Academic Year | 2023-24 |
Read the given statements carefully and state T for true and F for false.
(i) The value of k for which the equations x + 2y = 5 and 3x + ky - 15 = 0 has no solution, is 6.
(ii) The system of equations 3x - 5y = 20 and 6x - 10y = 40 has infinitely many solutions.
(iii) If the sum of the digits of a two digit number is 8 and difference between the number and the number formed by reversing the digits is 18, then the number is 34.
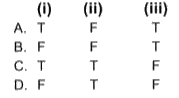
View SolutionRead the statements carefully and select the correct option
Statement-I: If there are (2n + 1) terms in an A.P., then the ratio of the sum of odd terms and the sum of even terms is (n + 1): n.
Statement-II : If `S_n` the sum of first n terms of an A.P. is given by `S_n = 5n^2 + 3n`, then its `n^(th)` term is 10n - 2.
View SolutionMatch the following and select the correct option

View SolutionSolve the following:
(i) Three consecutive natural numbers are such that the square of the middle number exceeds the difference of the squares of the other two by 60. Find the numbers.
(ii) A train travels 360 km at a uniform speed. If the speed had been 5 km/h more, it would have taken 1 hour less for the same journey . Find the speed of the train.
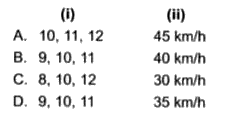
View Solution
ACHIEVERS SECTION questions and complete solutions for chapter SOF INTERNATIONAL MATHEMATICS OLYMPIAD QUESTION PAPER 2020 of HT Olympiad Previous Year Paper of Class 10