Complete Solutions to II. Answer the following of chapter S.S.L.C ANNUAL EXAMINATION QUESTION PAPER MARCH- 2019 ( WITH ANSWERS ) of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | S.S.L.C ANNUAL EXAMINATION QUESTION PAPER MARCH- 2019 ( WITH ANSWERS ) |
| SUBHASH PUBLICATION | II. Answer the following |
| Number Of Questions | 38 |
| Academic Year | 2023-24 |
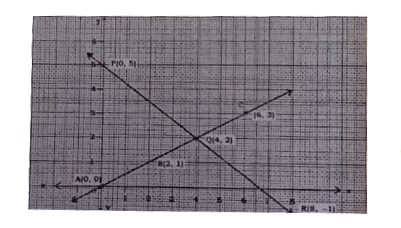
The given graph represents a pair of linear equations in two varibles . Write how many solutions these pair of equations have .
View Solution17 = `6 xx2`+5 is compared with Euclid's Division lemma a = bq +r then which number is representing the remainder
View SolutionFind the zeroes of the polynomial P (x) = `x^(2)-3`
View SolutionWrite the degree of the polynomial P (x) = `2x^(3)-x^(2)+5`
View SolutionFind the value of the discriminant of the quadratic equation `2x^(2)-4x+3=0`
View SolutionWrite the foumula to calculate the curved surface area of the frustum of a cone .
View SolutionFind the sum of first twenty terms of Arithmetic series 2 +7+12 + `cdots ` using suitable formula.
View SolutionIn `Delta` ABC , AD `bot`BC and `AD^(2)= BD xx CD`. Prove that `AB^(2) + AC^(2)`= `(BD + CD)^(2)`

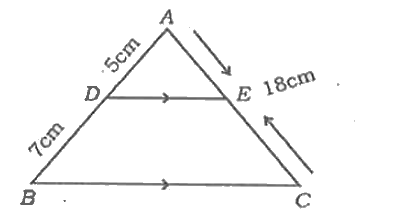
View SolutionIn `Delta` ABC , DE `||` BC . If AD= 5 cm , BD = 7 cm and AC = 18 cm , find the length of AE.
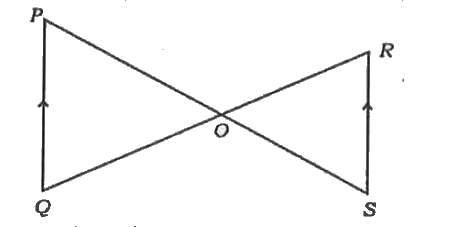
View SolutionIn the given figure PQ `||` RS , prove that `Delta POQ~Delta SOR.`
View SolutionSolve the following pair of linear equations by any suitable method.
x+y = 5 `" "` 2x-3y=5
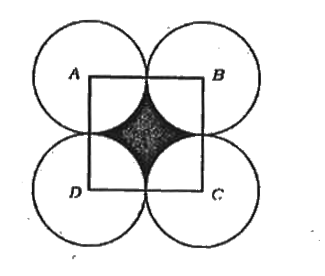
View SolutionIn the figure , ABCD is a square of side 14 cm. A, B, C and D are the centres of four congruent circle such that each circle touches externally two of the remaining three circles . Find the area of the shaded reginon.
View SolutionDraw a circle of radius 4 cm and construct a pair of tangents such that the angle between them is `60^(@)`.
View SolutionFind the co - ordinates of points which divides the line segment joining the points A `(4,-3)` and B (8,5) in the ratio `3:1` internally .
View SolutionProve that `3+2sqrt(5)` is an irrational number .
View SolutionThe sum and product of the zeroes an a quadratic polynomial P (x) = `ax^(2) + bx +c` are `-3` and `2` respectively, Show that b+c = 5a.
View SolutionFind the quotient and the remainder when P (x) = `3x^(3)+ x^(2)+2x+5` is divided by g(x) = `x^(2)` +2x+1.
View SolutionSolve `2x^(2)`-5x+3 = 0 by using formula.
View SolutionThe length of a rectangular field is 3 time its breadth . If the area of the field is 147 sq.m, find its length and breadth.
View SolutionIf sin `theta = (12)/(13)` find the values of cos `theta` and tan`theta`. `" " (2)`
View SolutionIf `sqrt(3) "tan" theta = 1 "and " theta` is acute find the value of sin `3theta "cos" 2theta` .
View SolutionProve that `((1+cos theta)/(1-cos theta))=("cosec"theta + "cot"theta)^(2)`
View SolutionA cubical die numbered from 1 to 6 are rolled twice . Find the probability of getting the sum of numbers on its faces is 10 .
View SolutionThe radii of two circular ends of a frustum of a cone shaped dustbin are 15 cm and 18 cm . If its depth is 63 cm find the volume of the dustbin
View SolutionProve that the "Length of tangents drawn from an external point a circle are equal".
View SolutionProve that "the lengths of tangents drawn from an external point to a circle are equal".
OR
In the given figure PQ and RS are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersecting PQ at A and RS at B. Prove that `angleAOB = 90^(@)`.

View SolutionCalculate the median of the following frequency distribution tabel :
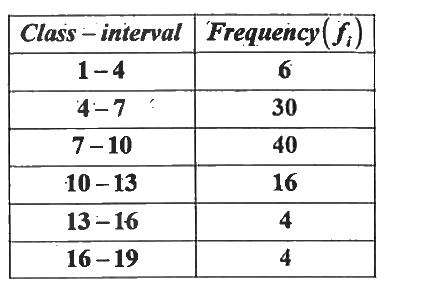
View SolutionCalculate the mode for the following frequency distribution table
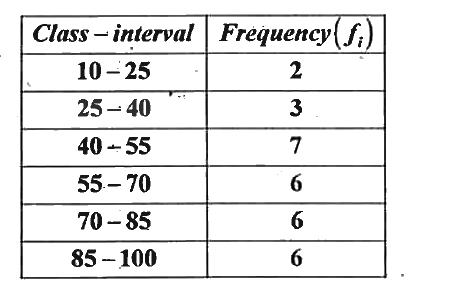
View SolutionDuring the medical check-up of 35 students of a class, their weights were recorded as follows:
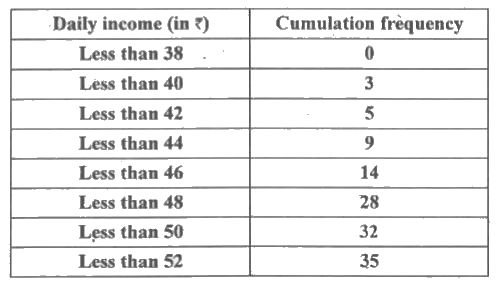
Draw a less than type ogive for the given data. Hence obtain the median weight from the graph and varify the result by using the formula.
View SolutionThe seventh term of an arithmetic progression is four times itss second term and twelth term is 2 more than three times of its fourth term. Find the progression.
View SolutionA line segment is divided into four parts forming an arithmetic progression . The sum of the lengths of 3 rd and 4 th parts is three times the sum of the lengths of first two part. If the length of fourth part is s14 cm, find the total length of the line segment.
View SolutionThe vertices of a `Delta ` ABC are A (-3,2) , B (-1,-4) and C (5,2) . If M and N are the mid - points of AB and AC respectively show that 2 MN = BC .
View SolutionThe vertices of a `Delta ` ABC are A (-5,-1), B (3,-5) , C (5,2). Show that the area of the `Delta ` ABC is four times the area of the triangle formed by joining the mid-points of the sides of the triangle ABC.
View SolutionConstruct a triangle with sides 5 cm, 6 cm and 7 cm and then another triangle whose sides are `(7)/(5)` of the corresponding sides of the first triangle.
View SolutionIf the angles of elevation of the top of a tower from two points at a distance of 4m and 9m from the base of the tower and in the same straight line with it are complementary, find the height of the tower.
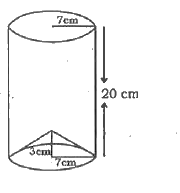
View SolutionThe bottom of a right cylindrical shaped vessel made from metallic sheet is closed by a cone shaped vessel as shown in the figure . The radius of the circular base of the cylinder and radius of the circular base of the cone each os equal to 7 cm . If the height of the cylinder is 20 cm and height of cone is 3 cm calculate the cost of milk to fill completely this vessel at the rate of Rs. 20 per litre.
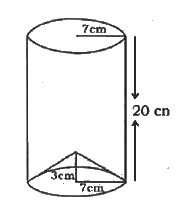
View SolutionA hemispherical vessel of radius 14 cm is fully filled with sand . This sand is poured on a level ground. The heap of sand forms a cone shape of height 7 cm . Calculate the area of ground occupied by the circular base of the heap of the sand.
View SolutionProve that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View Solution
II. Answer the following questions and complete solutions for chapter S.S.L.C ANNUAL EXAMINATION QUESTION PAPER MARCH- 2019 ( WITH ANSWERS ) of SUPER COMPANION MADE EASY of Class 10