Complete Solutions to EXERCISE 2.5 of chapter TRIANGLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | TRIANGLES |
| SUBHASH PUBLICATION | EXERCISE 2.5 |
| Number Of Questions | 23 |
| Academic Year | 2023-24 |
Sides of triangles are given below. Determine which of them are right triangles.
In case of a right triangle , write the length of its hypotenuse.
7cm, 24 cm, 25 cm
View SolutionSides of triangles are given below. Determine which of them are right triangles.
In case of a right triangle , write the length of its hypotenuse.
3cm, 24 cm, 25 cm
View SolutionSides of triangles are given below. Determine which of them are right triangles.
In case of a right triangle , write the length of its hypotenuse.
50cm, 80cm, 100 cm
View SolutionSides of triangles are given below. Determine which of them are right triangles.
In case of a right triangle , write the length of its hypotenuse.
13cm, 12 cm, 5 cm
View SolutionPQR is a triangle right angled at P and M is a point on QR such that `PM bot QR` . Show that `PM^(2)=QM .MR.`
View SolutionIn Fig , ABD is a triangle right angled at A and `AC bot BD.` show that
`AB^(2)=BC.BD`
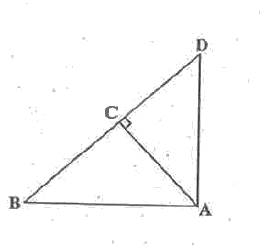
View SolutionIn Fig , ABD is a triangle right angled at A and `AC bot BD.` show that
`AC^(2)=BC . DC`
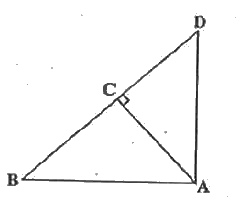
View SolutionIn Fig , ABD is a triangle right angled at A and `AC bot BD.` show that
`AD^(2)=BD.CD`
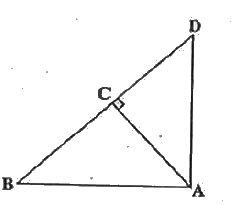
View SolutionABC is an isosceles triangle right angled at C . Prove that `AB^(2)=2AC^(2)` .
View SolutionABC is an isosceles triangle with AC=BC . If `AB^(2)=2AC^(2)` , prove that ABC is a right triangle.
View SolutionABC is an equilateral triangle of side 2a. Find each of its altitudes.
View SolutionProve that sum of the squares of the side of a rhombus is equal to the to the sum of the squares of its diagonals.
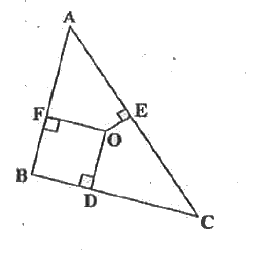
View SolutionIn Fig. 2.54, o is a point in the interior of a triangle `ABC,ODbotBC,OEbotACandofbotAB.` Show that
`OA^(2)+OB^(2)+OC^(2)-OD^(2)-OE^(2)-OF^(2)=AF^(2)+BD^(2)+CE^(2),`
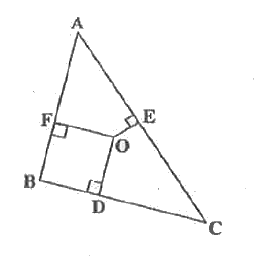
View SolutionIn Fig. 2.54, o is a point in the interior of a triangle `ABC,ODbotBC,OEbotACandofbotAB.` Show that
`AF^(2)+BD^(2)+CE^(2)=AE^(2)+CD^(2)+BF^(2).`
View SolutionA ladder 10 m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from base of the wall.
View SolutionA guy wire attached to a vertical pole of height 18 m is 24 m long and has a stake attached to the other end . How far from the base of the pole should the stake be driven so that the wire will be taut ?
View SolutionAn aeroplane leaves an airport and files due north at a speed of 1000 km per hour. At the same time, another aeroplane leaves the same airpot and flies due west at a speed of 1200 km per hour . How far apart will be the two planes after `1(1)/(2)` hours ?
View SolutionTwo poles of heights 6 m and 11 m stand on a plane ground. If the distance between the feet of the poles is 12m , find the distance between their tops .
View SolutionD and E are points on the sides CA and CB respectively of a triangle ABC right angale at C. prove that `AE^(2)+BD^(2)=AB^(2)+DE^(2).`
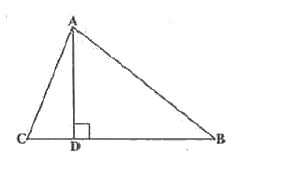
View SolutionThe perpendicular from A on side BC of a `DeltaABC` intersects BC at D such the DB = 3 CD . Prove that 2 `AB^(2)=2AC^(2)+BC^(2)`
View SolutionIn an equilateral triangle ABC , D is a point on side BC such that BD = `1/3` BC . Prove that `9 AD^(2)=7AB^(2)` .
View SolutionIn an equilateral triangle , prove that three times the square pf one side is equal to four times the square of one of its altitudes.
View SolutionTick the correct answer and justify : In `DeltaABC,AB=6sqrt3cm, AC=12 cm and BC=6cm`
The angle B is :
View Solution
EXERCISE 2.5 questions and complete solutions for chapter TRIANGLES of SUPER COMPANION MADE EASY of Class 10