Complete Solutions to EXERCISE of chapter APPLICATIONS OF TRIGONOMETRY of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | APPLICATIONS OF TRIGONOMETRY |
| VGS PUBLICATION-BRILLIANT | EXERCISE |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Draw diagram for the following situtions :
A person is flying a kite at an angle of elevation `alpha` and the length of thread from his hand to kite is 'l'.
View SolutionDraw diagram for the following situtions :
A person observes two banks of a river at angles of depression `theta_1" and "theta_2(theta_1lt theta_2)` from the top of a tree of height 'h' which is at a side of the river. The width of the river is 'd'.
View SolutionThe top of a clock tower is observed at angle of elevation of `alpha^(@)` and the foot of the tower is at the distance of d meters from the observer. Draw the diagram for this data.
View SolutionRinky observes a flower on the ground from the balcony of the first floor of a building at an angle of depression `beta^(@)`. The height of the first floor of the building is x meters. Draw the diagram for this data.
View SolutionA ladder of length x meter is leaning against a wall making angle `theta` with the ground. Which trigonometric ratio would you like to consider to find the height of the point on the wall at which the ladder is touching?
View SolutionA large balloon has been tied with a rope and it is floating in the air. A person has observed the balloon from the top of a building at angle of elevation of `theta_1` and foot of the rope at an angle of depression of `theta_2`. The height of the building is h feet. Draw the diagram for this data.
View SolutionA boy observed the top of an electric pole at an angle of elevation of `60^(@)` when the observation point is 8 meters away from the foot of the pole. Find the height of the pole.
View SolutionRajender observes a person standing on the ground from a helicopter at an angle of depression `45^(@)`. If the helicopter flies at a height of 500 meters from the ground, what is the distance of the person from Rajender?
View SolutionA tower stands vertically on the ground. From a point which is 15 meter away from the foot of the tower, the angle of elevation of the top of the tower is `45^(@)`. What is the height of the tower?
View SolutionA tree breaks due to storm and the broken part bends so that the top of the tree touches the ground by making `30^@` angle with the ground. The distance between the foot of the tree and the top of the tree on the ground is 6 m. Find the height of the tree before falling down.
View SolutionA contractor wants to set up a slide for the children to play in the park. Hed wants to set it up at the height of 2m and by making an angle of `30^(@)` with the ground. What should be the length of the slide?
View SolutionLength of the shadow of a 15 meter high pole is `5sqrt(3)` meters at 7 o'clock in the morning. Then, what is the angle of elevation of the Sun rays with the ground at the time?
View SolutionYou want to erect a pole of height 10 m with the support of three ropes. Each rope has to make an angle `30^(@)` with the pole. What should be the length of the rope?
View SolutionSuppose you are shooting an arrow from the top of a building at a height of 6 m to a target on the ground at an angle of depression of `60^(@)`. What is the distance between you and the object ?
View SolutionAn electrician wants to repair an electric connection on a pole of height 9m. He needs to reach 1.8 m below the top of the pole to do repair work. What should be the length of the ladder which he should use, when he climbs it at an angle of `60^(@)` with the ground? What will be the distance between foot of the ladder and foot of the pole?
View SolutionA boat had to cross a river. It crosses the river by making an angle of `60^(@)` with the bank of the river due to the stream of the river and travel a distance of 600 m to reach the another side of the river. What is the width of the river ?
View SolutionAn observer of height 1.8 m is 13.2 m away from a palm tree. The angle of elevation of the top of the tree from his eyes is `45^(@)`. What is the height of the palm tree ?
View SolutionIn the given figure, AC= 6 cm, AB= 5 cm and `/_BAC=30^(@)`. Find the area of the triangle.
View SolutionTwo men on either side of a temple of 30 meter height observe its top at the angles of elevation `30^(@)" and "60^(@)` respectively. Find the distance between the two men.
View SolutionA straight highway leads to the foot of a tower. Ramaiah standing at the top of the tower observe a car at an angle of depression `30^(@)`. The car is approaching the foot of the tower with a uniform speed. Six seconds later, the angle of depression of the car is found to be `60^(@)`. Find the time taken by the car to reach the foot of the tower from this point.
View SolutionA TV tower stands vertically on the side of a road. From a point on the other side directly opposite to the tower, the angle of elevation of the top of tower is `60^(@)`. From another point 10 m away from this point, on the line joining this point to the foot of the tower, the angle of elevation of the top of the tower is `30^(@)`. Find the height of the tower and the width of the road.
View SolutionA 1.5 m tall boy is looking at the top of a temple which is 30 m in height from a poin at certain distance. The angle of elevation from his eye to the top of the crown of the temple increases from `30^(@)" to "60^(@)` as he walks towards the temple. Find the distance he walked towards the temple.
View SolutionA statue stands on the top of a 2m tall pedestal. From a point on the ground, the angle of elevation of the top of the statue is `60^(@)` and from the same point the angle of elevation of the top of the pedstal is `45^(@)`. Find the height of the statue.
View SolutionFrom the top of a building, the angle of elevation of the top of a cell tower is `60^(@)` and the angle of depression to its foot is `45^(@)`. If distance of the building from the tower is 7m, then find the height of the tower.
View SolutionA wire of length 18m had been tied with electric pole at an angle of elevation `30^(@)` with the ground. As it is covering a long distance, it was cut and tied at an angle of elevation `60^(@)` with the ground. How much length of the wire was cut?
View SolutionThe angle of elevation of the top of a building from the foot of the tower is `30^(@)` and the angle of elevation of the top of the tower from the foot of the building is `60^(@)`. If the tower is 30m high, find the height of the building.
View SolutionTwo poles of equal height are standing opposite to each other on either sidef of the road, which is 120 feet wide. From a point between then on the road, the angles of elevation of the top of the poles are `60^(@)" and "30^(@)` respectively. Find the height of the poles and the distances of the point from the poles.
View SolutionThe angle of the elevation of the top of a tower from two points at a distance of 4m and 9m from the base of the tower and in the same straight line with it, are complementary. Prove that the height of the tower is 6m.
View SolutionThe angle of elevation of a jet plane from a point A on the ground is `60^(@)`. After a flight of 15 seconds, the angle of elevation changes to `30^(@)`. If the jet plane is flying at a constant height of `1500sqrt(3)` m, find the speed ot the jet plane.
View SolutionThe angle of elevation of the top of a tower from the foot of the building is `30^(@)` and the angle of elevation of fhe top of the building from the foot of the tower is `60^(@)`. What is the ratio of heights of tower and building?
View SolutionA 1.2m tall girl spots a balloon moving with the wind in a horizontal line at a height of 88.2 m from the ground. The angle of elevation of the balloon from the eyes of the girl at any instant is `60^(@)`. After sometimes, the angle of elevation reduces to `30^(@)`. Find the distance travalled by the balloon during the interval.
View SolutionThe angles of elevation of the top of a lighthouse from 3 boats A,B and C in a straight line of same side of the lighthouse are a,2a,3a respectively. If the distance between the boats A and B is x meters. Find the height of lighthouse.
View SolutionInner part of a cupboard is in the cuboidical shape with its length, breadth and height in the ratio `1: sqrt(2):1`. What is the angle made by the longest stick which can be inserted cupboard with its base inside?
View SolutionAn iron spherical ball of volume 232848 `cm^3` has been melted and converted into a cone wih the vertical angle of `120^(@)`. What are its height and base?
View SolutionShow that the area of an Isosceles triangles is `A=a^2SinthetaCostheta` where a is the length of one of the two equal sides and `theta` is the measure of one of two equal angles.
View SolutionA right circular cylindrical tower, height 'h' and radius 'r', stands on the ground. Let 'P' be a point in the horizontal plane ground and ABC be the semi-circular edge of the top of the tower such that B is the point in it nearest to P. The angles of elevation of the points A and B are `45^(@)" and "60^(@)` respectively. Show that `(h)/(r)=(sqrt(3)(1+sqrt(3)))/(2)`.
View SolutionA person from the top of a building of height 25 m has observed another building top and bottm at an angle of elevation `45^(@)` and at an angle of depression `60^@` respectively. Draw the diagram for this data.
View SolutionA person observed the top of a tree at an angle of elevation of `60^(@)` when the observation point was 5m away from the foot of the tree. Draw a diagram for this data.
View SolutionIf the angle of elevation of sun increases from '0' to 90 then the length of shadow of a tower decreases. Is this true? Justify your answer.
View SolutionIf a tower of height 'h' is observed from a point with a distance 'd' and angle '`theta`' , then express the relation among h,d, `theta`.
View SolutionA pole and its shadow have same length, find the angle of the sun ray made with the earth at that time.
View SolutionA ladder of 3.9 m length is laid against a wall. The distance between the foot of the wall and the ladder is 1.5 m. Find the height at which the ladder touches the wall.
View SolutionA boat has to cross a river. It crosses river by making an angle of `60^(@)` with bank, due to the stream of river it travels a distance of 450m to reach another side of river. Draw a diagram to this data.
View SolutionA person 25 m away from a cell tower observes the top of cell tower at an angle of elevation `30^(@)`. Draw the suitable diagram for this situation.
View SolutionA straight highway leads to foot of the tower. A man standing at the top of the tower observes a car at an angle of depression of `theta`, which is approaching the foot of the tower with a uniform speed. Six seconds later the angle of depression is `phi`. Draw a diagram for this data and analyze.
View SolutionFrom the top of a tower of h m height, Anusha observes the anges of depression of two points X and Y on the same side of tower on the ground to be `alpha" and "beta`. Draw the suitable figure for the given information.
View SolutionFrom the top of a building, the angle of elevation of the top of a cell tower is `60^(@)` and the angle of depression to its foot is `45^(@)`. If distance of the building from the tower is 7m, then find the height of the tower.
View SolutionFrom the top of the building, the angle of elevation of the top of a. T.V tower is `alpha^@` and the angle of depression to its (T.V tower) foot is `beta^@`. If distance of the building from the tower is ‘d’ meters, draw the suitable diagram to the given data.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 50 m away from the foot of the tower is `45^@`. Draw the diagram for the situation.
View SolutionAn observer flying in an aeroplane at an altitude of 900 m observes two ships in front of him, which are in the same direction at an angles of depression of `60^(@)" and "30^(@)`respectively. Find the distance between the two ships.
View SolutionA person from the top of a building height 15 m observes the top and the bottem of a cell tower with the angle of elevation as `60^(@)` and the angle of depression as `45^(@)` respectively. Then find the height of the that cell tower.
View SolutionTwo poles of equal height are standing opposite to each other on either side of the road, which is 80 m wide. From a point between then on the road, the angles of elevation of top of the poles are `60^(@)" and "30^(@)`. Find the height of poles.
View SolutionA tree is breaken without separating from the stem by the wind. The top touches the ground making an angle `30^(@)` at a distance of 12m from the foot of the tree. Find the height of the tree before breaking.
View SolutionTwo poles are standing opposite to each other on either sidef of the road which is 90 feet wide.The angle of elevation from bottom of first pole to top of second pole is `45^(@)`, the angle of elevation from bottom of second pole to top of first pole is `30^(@)`. Find the heights of poles.
View SolutionThe angle of the elevation of the top of a tower from two points at a distance of 4m and 9m from the base of the tower and in the same straight line with it, are complementary. Prove that the height of the tower is 6m.
View SolutionFrom the top of a tower of 50m high, Neha observes the angles of depression of the top and foot of another building to be `45^(@)" and "60^(@)` respectively. Find the height of the building.
View SolutionThe angle of elevation of the top of a hill from the foot of a tower is `60^@` and the angle of elevation of the top of the tower from the foot of the hill is `30^@`. If the tower is 50 m high. Find the height of the hill.
View SolutionTwo men on either side of a temple of 30 meter height observe its top at the angles of elevation `30^(@)" and "60^(@)` respectively. Find the distance between the two men.
View SolutionA man observes top of a tower at an angle of elevation of `30^@`. When he walked 40 m towards the tower, the angle of elevation is changed to `60^@` Find the height of the tower and distance from the first observation point to the tower.
View SolutionA flag pole 4 cm tall casts a 6m, shadow. At the same time , a nearby building casts a shadow of 24 m. How tall is the building?
View SolutionA pole is arranged from a height of 30m from the ground, making `60^(@)` angle with earth. Then what is its length?
View SolutionA long pole is broken in a storm. Top end of the broken pole touched the head of a man at a distance of 'd'. Then find the angle between the man and the pole that before storm.
View SolutionA kite is flown from a building with a height (h) m with a long rope. Now the kite, and the person having it are observed with angles of elevation `alpha" and "beta` respectively by a boy. The distance between boy and building is 'x' m. So draw a diagram for this data.
View SolutionA person observes tops of two buildings with an angle of elevations `35^(@)" and "46^(@)` from the mid point in between them. So which building is higher? Why?
View SolutionA 15 m long pole forms `5sqrt(3)` m long shadow at 8 AM in the morning. Then find the angle made by sun rays with earth.
View SolutionLadder 'x' meters long is laid against a well making an angle `'theta'` with the ground. If we want to directly find the distance between the foot of ladder and foot of the wall, which trigonometrical ratio should be considered?
View SolutionTwo persons A and B observe the top of a pole at an angle of elevation `alpha` and `beta` respectively. If `alpha gt beta`, then ........
View SolutionTop of a building was observed at an angle of elevation `alpha` from a point. Which is at distance 'd' meters from the foot of the building. Which trigonometrical ratio should be considered for finding height of buildings?
View SolutionIf the angle of elevation of sun increases from `0^(@)" to "90^(@)`, then the length of shadow of the tower………..
View SolutionThe angle of depression from the top of a tower 12m height, at a point on the ground is `30^(@)`. The distance of the point from the top is…………….
View SolutionA ladder touches a wall at a height of 5m. The angle made by the ladder with the ground, if its length is 10m, will be………….
View SolutionA 20 m long ladder is placed on a pole of 10 m height making '`alpha`' angle with the ground, then, `alpha` =
View SolutionA ladder 15m long just reaches the top of vertical wall. If the ladder makes an angle of `60^@` with the wall. Then the height of the wall is
View SolutionThe length of the shadow of a tree is 8 m long when the sun's angle of elevation is `45^(@)` is………….m.
View SolutionIf a pole 6 m high casts a shadow `2sqrt(3)`m long on the ground. Then the sun's angle of elevation is
View SolutionIf the angle of elevation of a tower from a distance of 100 m from its foot is `60^(@)`. Then the height of the tower is ……….m.
View SolutionThe height of a tower is 10m. The length of its shadow when sun's altitude is `45^(@)` is……….m.
View SolutionThe length of the shadow of a tower on the plane ground is `sqrt(3)` times the height of the tower. The angle of elevation of sun is
View SolutionIn the given figure, the position of the observer and the object are marked, the angle of depression is
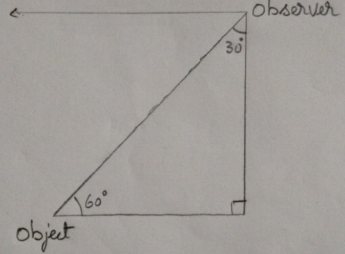
View SolutionThe ratio of the length of a rod and its shadow is `1:sqrt(3)`, then the angle of elevation of the sun is
View SolutionIf two towers of height X and Y subtend angles of `30^(@)" and "60^(@)` respectively at the centre of the line joining their feet, then X:Y is equal to
View SolutionA wall of 8m long casts a shadow 5m long. At the same time, a tower casts a shadow 50m long. Then the height of tower is
View SolutionIf the sun's angle of elevation is `60^(@)`. Then a pole of height 6m will cast a shadow of length……….m.
View SolutionA pole of 12m high casts a shadow `4sqrt(3)`m on the ground. Then the sun's angle of elevation is
View SolutionIf the height and length of the shadow of a man are the same, then the angle of elevation of the sun is
View SolutionIf the length of the shadow of a tower is `(1)/(sqrt(3))` times the height of the tower, then the angle of elevation of the sun is………
View SolutionA tower is 50m high. Its shadow is x m shorter when the sun's altitude is `45^(@)` then when it is `30^(@)`, then x=……….m.
View SolutionThe length of the string of a kitef flying at 100m above the ground with the elevation of `60^(@)` is…………..
View SolutionA player sitting on the top of a tower of height 40m observes the angle of depression of a ball lying on the ground is `60^(@)`. The distance between the foot of the tower and ball is…………m.
View SolutionIf the ratio of height of a tower and the length of its shadow on the ground is `sqrt(3):1`, then the angle of elevation of the sun is…………
View SolutionThe angle of depression from the top of a tower 100m height, at a point on the ground is `45^(@)`,. The distance of the point from the tower is…………….
View SolutionAn object is placed above the observer's horizontal, we call the angle between the line of sight and observer's horizontal is………
View SolutionAngle of elevation of the top of a building from a point on the ground is `30^(@)`, Then the angle of depression of this point from the top of the building is…………..
View SolutionWhat change will be observed in the angle of elevation as we move away from the object?
View SolutionAn objcet is placed below the observer's horizontal, then what is the angle between line of sight and observer's horizontal ?
View SolutionWhat change will be observed in the angle of elevation as we approach the foot of the tower?
View SolutionIn the figure given below, the imaginary line through the object and eye of the observer is called………..

View SolutionIf a pole height 6m casts a shadow `2sqrt(3)`m long on the ground, then the sun's elevation is
View SolutionThe length of the shadow of a tree is 7m high, when the sun's elevation is………….
View SolutionIf two tangents inclined at an angle of `60^(@)` are drawn to a circle of radius 3 cm, then length of tangents is equal to…………m.
View Solution
EXERCISE questions and complete solutions for chapter APPLICATIONS OF TRIGONOMETRY of VGS BRILLIANT MATHS (TELUGU ENGLISH) of Class 10