Complete Solutions to Taking it together of chapter CIRCULAR MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | CIRCULAR MOTION |
| DC PANDEY NEET | Taking it together |
| Number Of Questions | 67 |
| Academic Year | 2023-24 |
A particle moves in a uniform circular motion. Choose the wrong statement.
04:17View SolutionA stone is tied with a string and is rotated in a circle horizontally. When the string suddenly breaks, the stone will move
02:15View SolutionA particle moving along a circular path due to a centripetal force having constant magnitude is an example of motion with :
07:16View SolutionA particle moving on a circular path makes 600 rpm. In how much time it will complete one revolution?
02:05View SolutionA wheel having moment of interia `2 kgm^(-2)` about its axis, rotates at 50 rpm about this axis. The angular retardation that can stop the wheel in one minute is
03:20View SolutionThe speed of a particle moving in a circle is increasing. The dot product of its acceleration and velocity is
04:06View SolutionA particle moves with constant angular velocity in a circle. During the motion its
02:32View SolutionAn object is moving in a circle of radius 100 m with a constant speed of `31.4 m//s` . What is its average speed for one complete revolution
01:30View SolutionA bucket full of water is rotated in a vertical circle of radius R. If the water does not split out, the speed of the bucket at topmost point will be
05:40View SolutionA car when passes through a convex bridge exerts a force on it which is equal to
02:43View SolutionIn `1.0 s`, a particle goes from point `A` to point `B` , moving in a semicircle of radius `1.0 m ` (see figure ). The magnitude of the average velocity
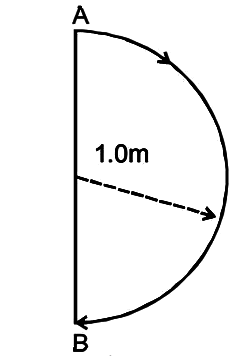 01:26View Solution
01:26View SolutionA fan makes 2400 rpm. If after it is switched off, it comes to rest in 10 s, then find the number of times it will rotate before it comes to rest after it is switched off.
04:14View SolutionThe distance between the rails of the track is `1.67` m. How much the outer rail be elecated for curve of `0.5`km radius, so that a train moving with speed `54kmh^(-1)` can take safe turn on track.
View SolutionA car is moving on a circular path and takes a turn. If `R_(1)` and `R_(2)` be the reactions on the inner and outer wheels, respectively, then
03:08View SolutionAn unbanked curve has a radius of 60m. The maximum speed at which a car can make a turn if the coefficient of static friction is `0.75` , is
03:22View SolutionA particle is moving in a circle with uniform speed v . In moving from a point to another diametrically opposite point
02:20View SolutionA motor cyclist riding at `36kmh^(-1)` has turn a corner. Find the least radius of the curve, he should follow for safe travelling, if the coefficient of friction between the tyres and the road is `0.2`.
03:06View SolutionA circular curve of a highway is designed for traffic moving at 72 km/h. if the radius of the curved path is 100 m, the correct angle of banking of the road should be given by:
02:19View SolutionA particle of mass `m` is moving in a plane along a circular path of radius `r`. Its angular momentum about the axis of rotation is `L`. The centripetal force acting on the particle is.
01:19View SolutionA conical pendulum of length L makes an angle `theta` with the vertical. The time period will be
 04:30View Solution
04:30View SolutionA body of mass 1 kg is moving in a vertical circular path of radius 1 m. The difference between the kinetic energies at its highest and lowest position is
02:21View SolutionA particle is moving along a circular along a circular path of radius 5 m with a uniform speed `5ms^(-1)`. What will be the average acceleration when the particle completes half revolution?
04:08View SolutionIf the banking angle of curved road is given by `tan^(-1)((3)/(5))` and the radius of curvature of the road is 6 m, then the safe driving speed should not exceed (take, `g=10ms^(-2)`)
04:02View SolutionA moter-cyclist moving with a velocity of 144 `kmh^(-1)` on a flat road takes a turn on the road at a point where the radius of curvature of the road is 40 m. The acceleration due to gravity is 10 `ms^(-2)`. In order to avoid sliding, he must bend with respect to the vertical plane by an angle
04:13View SolutionA train has to negotiate a curve of radius 800 m. By how much height should the outer rail be raised with respect to inner rail for a speed of `96kmh^(-1)`? The distance between the rails is 1 m
05:12View SolutionA car wheel is rotated to uniform angular acceleration about its axis. Initially its angular velocity is zero. It rotates through an angle `theta_(1)` in the first 2 s. In the next 2 s, it rotates through an additional angle `theta_(2)`, the ratio of `(theta_(2))/(theta_(1))` is
04:11View SolutionA pendulum bob on a 2 m string is displaced `60^(@)` from the vertical and then released. What is the speed of the bob as it passes through the lowest point in its path
03:30View SolutionThe bob of a `0.2` m pendulum describes an arc of circle in a vertical plane. If the tension in the cord is `sqrt3` times the weight of the bob when the cord makes an angle `30^(@)` with the vertical, the acceleration of the bob in that position is
05:45View SolutionIn a typical projectile motion, tangential acceleration at the topmost point P of the trajectory is
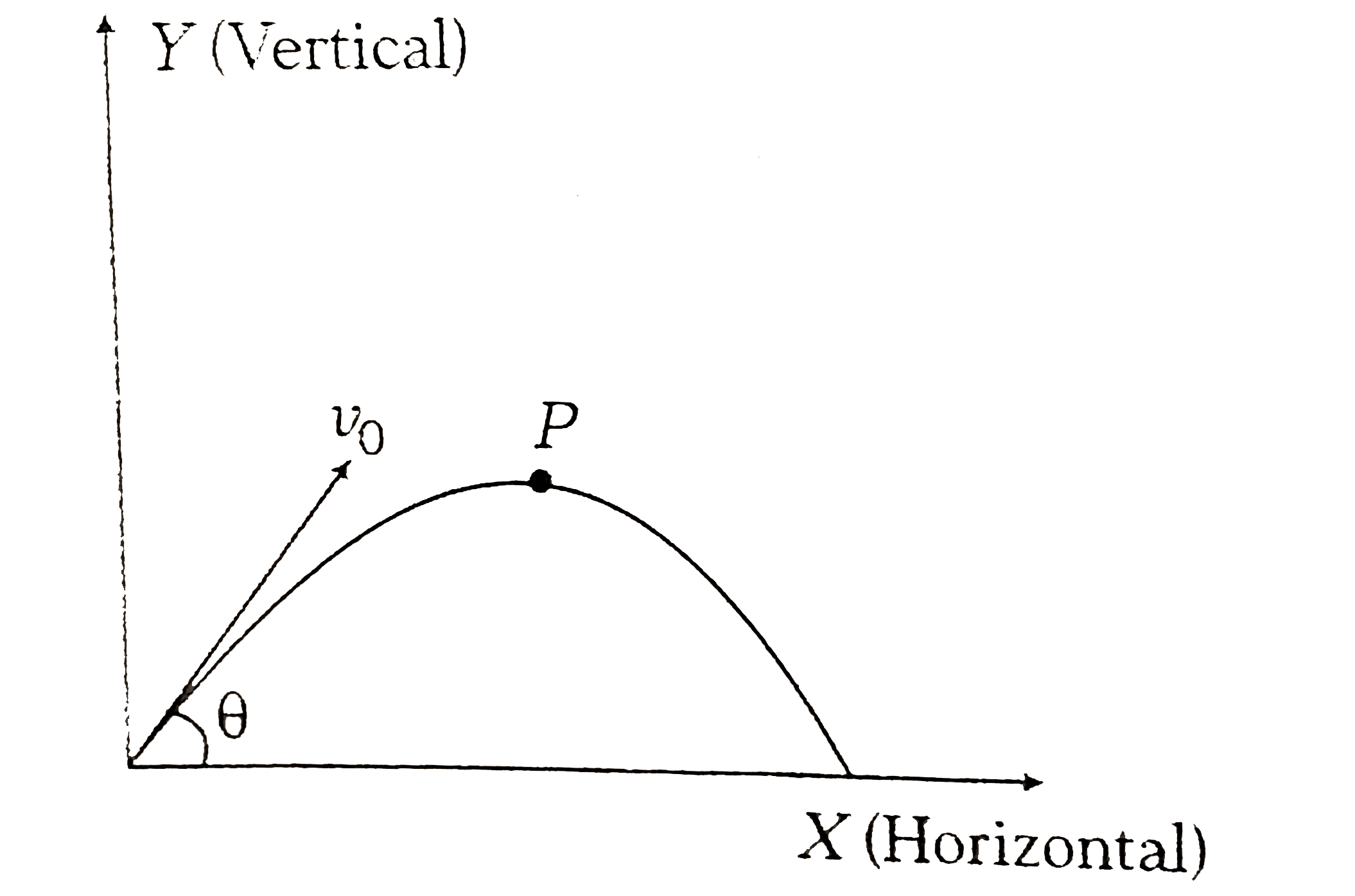 02:32View Solution
02:32View SolutionA jeep runs around a curve of radius `0.3` km at a constant speed of `60ms^(-1)`. The jeep covers a curve of `60^(@)` arc
06:38View SolutionA coin, placed on a rotating turntable slips, when it is placed at a distance of `9cm` from the center. If the angular velocity of the turnable is tripled, it will just slip, If its distance from the center is
04:44View SolutionA circular disc of radius R is rotating about its axis O with a uniform angular velocity `omega"rad s"^(-1)` as shown in the figure. The magnitude of the relative velocity of point A relative to point B on the disc is
 02:07View Solution
02:07View SolutionWhen the angular velocity of a uniformly rotating body has increased thrice, the resultant of forces applied to it increases by 60 N. Find the accelerations of the body in the two cases. The mass of the body,m = 3 kg.
02:12View SolutionAn automobile enters a turn of radius `R`. If the road is banked at an angle of `45^(@)` and the coefficient of friction is 1, the minimum and maximum speed with which the automobile can negotiate the turn without skidding is `:`
10:16View SolutionA mass is attached to the end of a string of length l which is tied to a fixed point O. The mass is released from the initial horizontal position of the string. Below the point O at what minimum distance a peg P should be fixed so that the mass turns about P and can describe a complete circle in the vertical plane?
03:20View SolutionA stone is rotated in a vertical circle. Speed at bottommost point is `sqrt(8gR)`, where R is the radius of circle. The ratio of tension at the top and the bottom is
02:52View SolutionThe string of a pendulum is horizontal. The mass of the bob is m. Now the string is released. The tension in the string in the lowest position is -
02:58View SolutionA body is moving in a vertical circle of radius r such that the string is just taut at its highest point. The speed of the particle when the string is horizontal is
01:58View SolutionA small ball is pushed from a height h along a smooth hemispherical bowl of rodius R. With what speed should the ball be pushed so that it just reaches the top of the opposite end of the bowl?
02:14View SolutionA 50 kg girl is swinging on a swing from rest. Then, the power delivered when moving with a velocity of `2ms^(-1)` upwards in a direction making an angle `60^(@)` with the vertical is
04:10View SolutionA simple pendulum of length l has a maximum angular displacement `theta`. The maximum kinetic energy of the bob of mass m will be
02:02View SolutionToy cart tied to the end of an unstretched string of length a, when revolved moves in a horizontal circle of radius 2a with a time period T. Now the toy cart is speeded up until it moves in a horizontal circle of radius 3a with a period T. If Hooke's law (F=kx) holds, then
03:12View SolutionA spere is suspended by a thread of length l. What minimum horizontal velocity has to be imparted to the ball for it to reach the height of the suspension?
01:26View SolutionA cyclist starts from the centre O of a circular park of radius 1km, reaches the edge P of the park, then cycles along the PQ circumference and returns to the centre along OQ as shown in fig. If the round trip taken ten minute, the net displacement and average speed of the cyclist (in kilometer and kinetic per hour) is
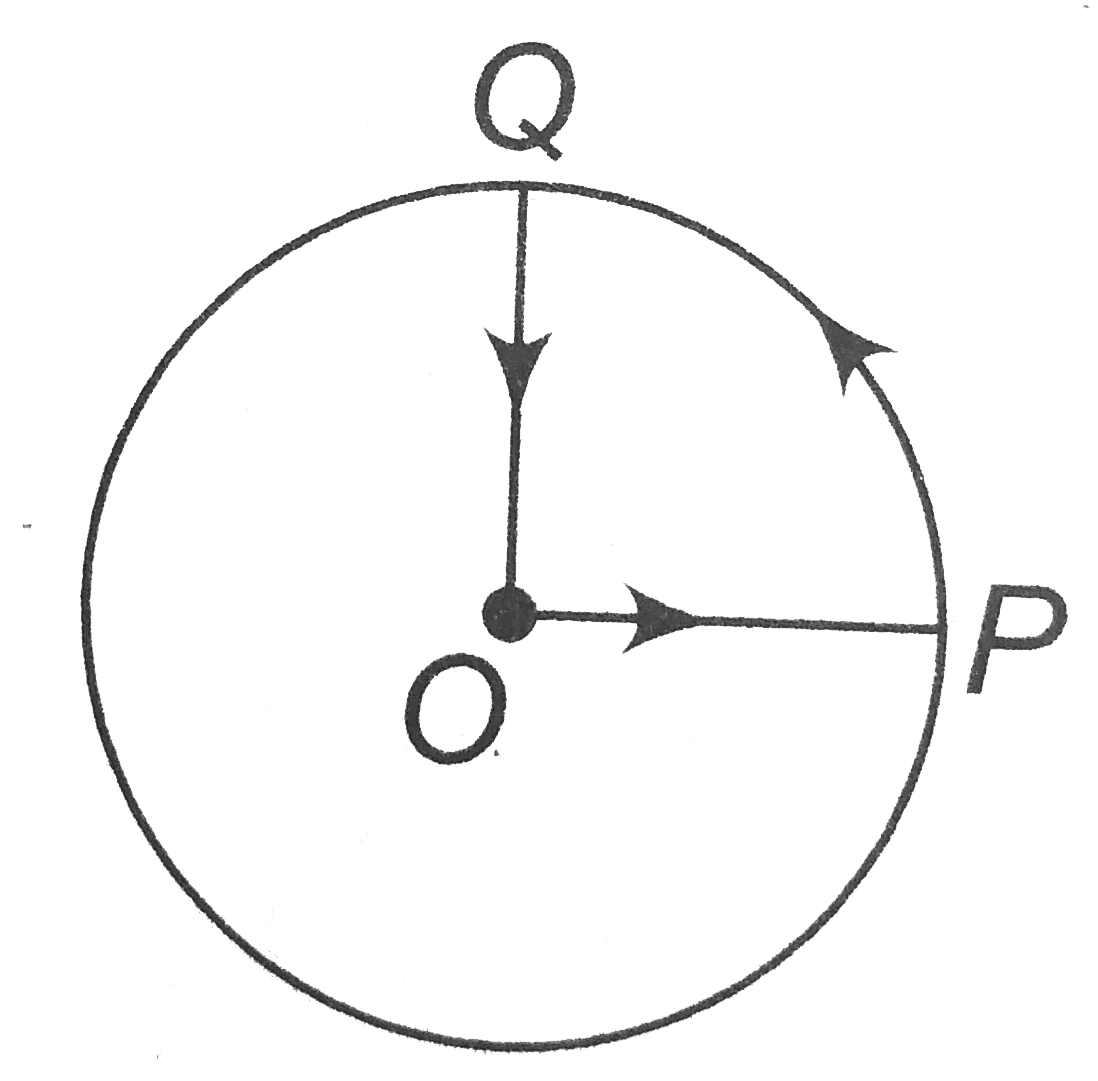 07:52View Solution
07:52View SolutionThree particles A, B and C move in a circle of radius `r=(1)/(pi)`m, in anti-clockwise direaction with speed `1ms^(-1), 2.5ms^(-1)` and `2ms^(-1)` respectively. The initial position of A, B and C are as shown in figure.
The ratio of distance travelled by B and C by the instant A, B and C meet for the first time is01:02View SolutionTwo particles A and B start at the origin O and travel in opposite directions along the circular path at constant speeds `v_(A)=1.4ms^(-1)` and `v_(B)=3ms^(-1)` respectively. The time when they collide and the magnitude of the acceleration of B just before this happens are
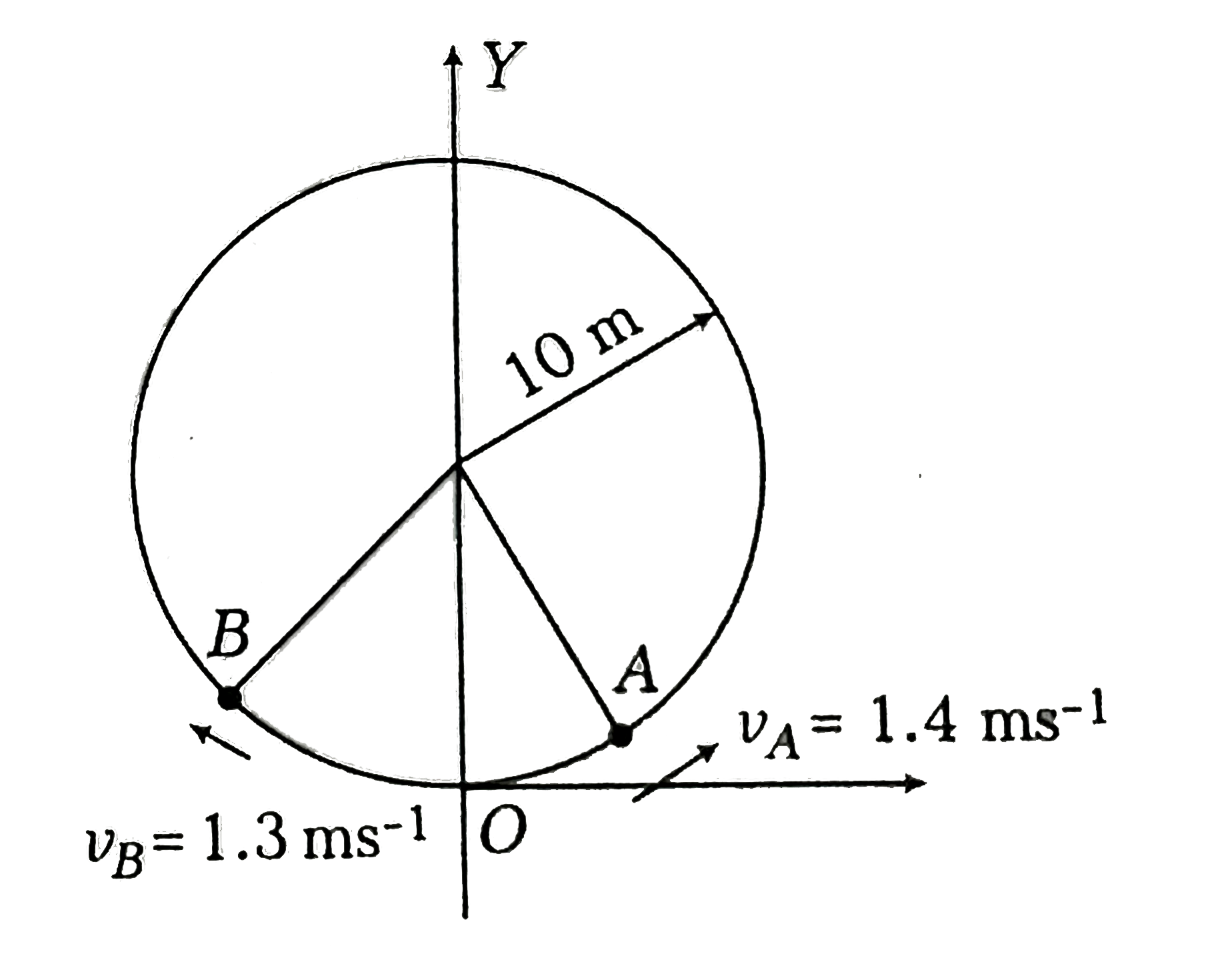 01:34View Solution
01:34View SolutionA student whirls a stone in a horizontal circle of radius 3 m and at height 8 m above level ground. The string breaks, at lowest point and the stone flies off horizontally and strikes the ground after travelling a horizontal distance of 20 m. What is the magnitude of the centripetal acceleration of the stone while breaking off.
01:27View SolutionA stone is tied to a string of length l and is whirled in a vertical circle with the other end of the string as the centre. At a certain instant of time, the stone is at its lowest position and has a speed u. The magnitude of the change in velocity as it reaches a position where the string is horizontal (g being acceleration due to gravity) is
07:20View SolutionA ball suspended by a thread swings in a vertical plane so that its acceleration in the extreme position and lowest position are equal. The angle of thread deflection in the extreme position will be - 2
02:25View SolutionA body of mass m hangs at one end of a string of lenth l, the other end of which is fixed. It is given a horizontal velocity so that the string would just reach where it makes an angle of `60^(@)` with the vertical. The tension in the string at bottommost point position is
01:25View SolutionA simple pendulum oscillates in a vertical plane. When it passes through the mean position, the tension in the string is `3` times the weight of the pendulum bob.what is the maximum displacement of the pendulum with respect to the vertical
02:03View SolutionA stone of mass 1 kg tied to a light inextensible string of lenth `L=(10)/(3)m`, whirling in a circular path in a vertical plane. The ratio of maximum tension to the minimum tension in the string is 4. If g is taken to be `10ms^(-2)`, the speed of the stone at the highest point of the circle is
05:43View SolutionA string of length l fixed at one end carries a mass `m` at the other end. The strings makes `(2)/(pi)revs^(-1)` around the axis through the fixed end as shown in the figure, the tension in the string is
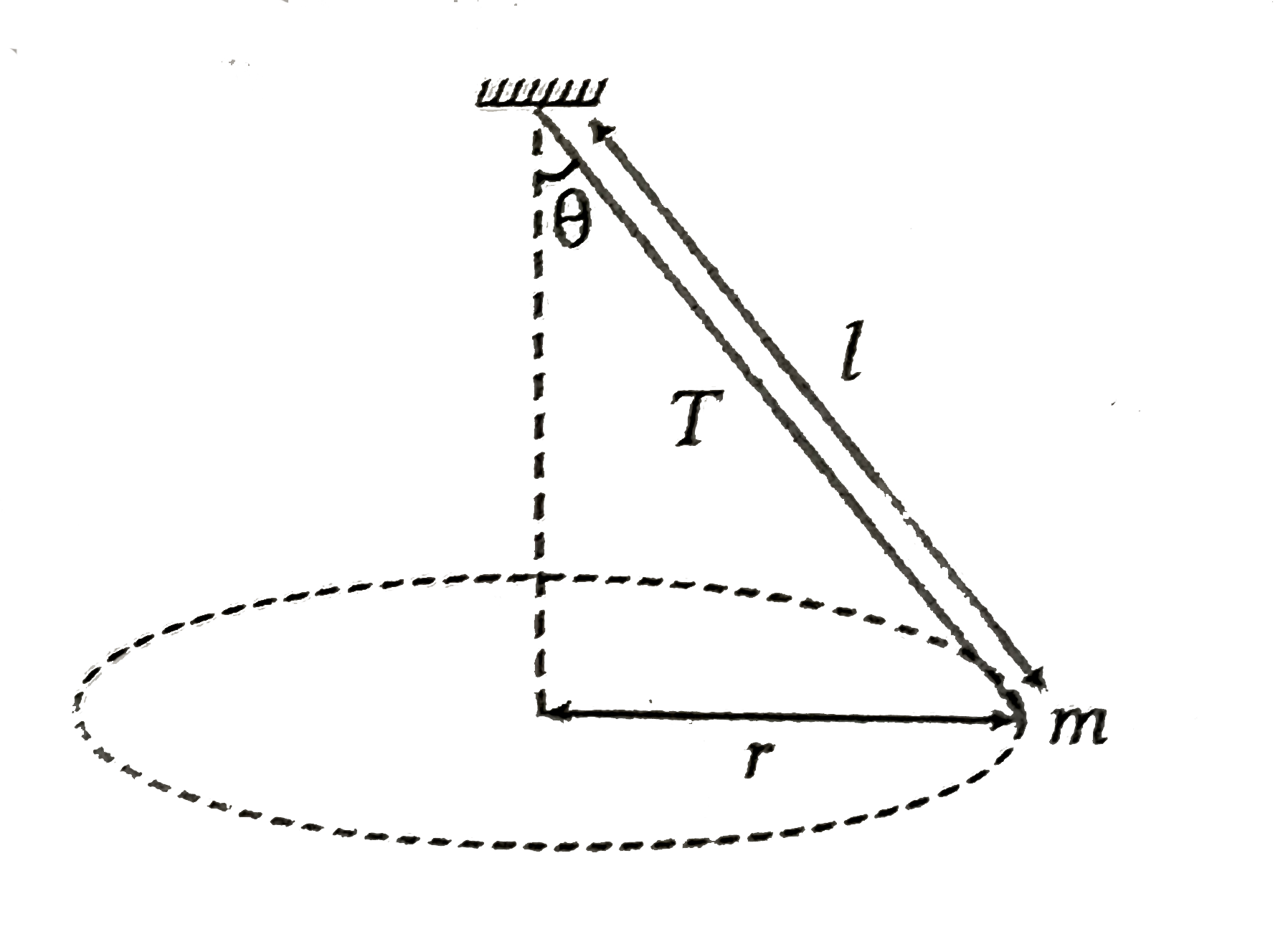 01:17View Solution
01:17View SolutionA particle starts travelling on a circle with constant tangential acceleration. The angle between velocity vector and acceleration vector, at the moment when particle completes half the circular track. Is
04:04View SolutionA wet open umbrella is held verticle and whirled about the handle at a uniform rate of 21 rev in 44 s. If the rim of the umbrella is a circle of 1 m in diameter and the hight of the rim above the floor is `4.9` m. The locus of the drop on the floor is a circle of radius
06:17View SolutionA heavy particle is tied to the end A of a string of the length`1.6` m. Its other end O is fixed. It revolves as a conical pendulum with the string making `60^(@)` with the horizontal. Then,
12:18View SolutionA pendulum bob has a speed of `3ms^(-1)` at its lowest position. The pendulum is `0.5` m long. The speed of the bob, when string makes an angle of `60^(@)` to the vertical is `("take, g"=10ms^(-1))`
02:21View SolutionA block is released from rest at the top of an inclined plane which later curves into a circular track of radius r as shown in figure. The minimum height h from where it should be released so that it is able to complete the circle, is
View SolutionA small body of mass m slides without friction from the top of a hemisphere of radius r. At what height will the body be detached from the surface of hemisphere?
 09:55View Solution
09:55View SolutionThe maximum tension that an inextensible ring of radius 1 m and mass density `0.1` kg `m^(-1)` can bear is 40 N. The maximum angular velocity with which it can be rotated in a circular path is
06:02View SolutionTwo bodies of masses `m` and `4m` are attached to a light string as shown in figure. A body of mass m hanging from string is executing oscillations with angular amplitude `60^@`, while other body is at rest on a horizontal surface. The minimum coefficient of friction between mass `4m` and the horizontal surface is (here pulley is light and smooth)
 02:50View Solution
02:50View SolutionA bullet of mass m moving with a horizontal velocity u strikes a stationary wooden block of mass M suspended by a string of length L = 50 cm. The bullet emerges out of the block with speed `(u)/(4)`. If M = 6 m, minimum value of u so that the block can complete the vertical circle (take, g = 10 `ms^(-2)`)
07:21View SolutionThree identical particles are joined together by a thread as shown in figure. All the three particles are moving in a horizontal plane. If the velocity of the outermost particle is `v_(0)`, then the ratio of tensions in the three sections of the string is `(T_(BC):T_(AB):T_(DA))`
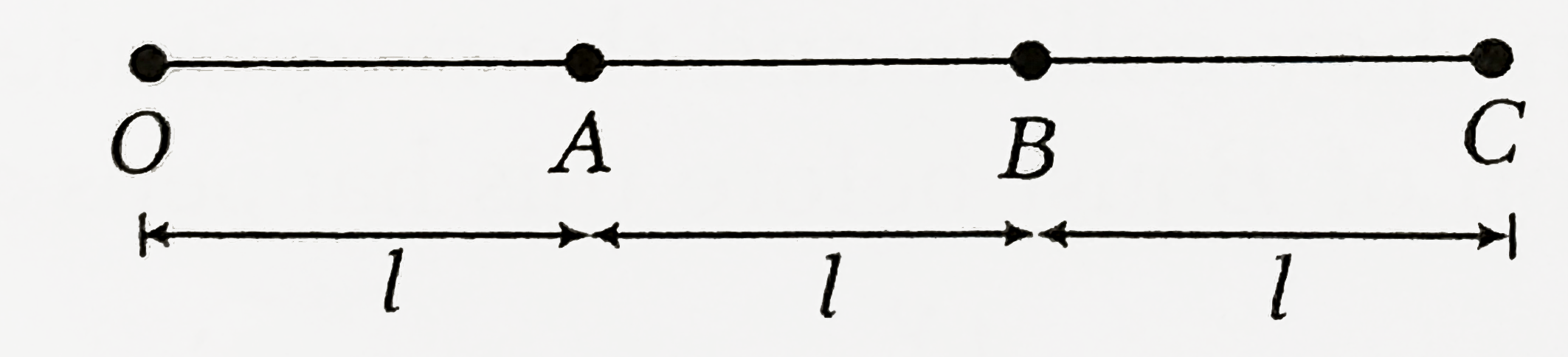 03:39View Solution
03:39View SolutionA partical moves from rest at A on the surface of a smooth circule cylinder of radius `r` as shown . At `B` it leavels the cylinder. The equation releaseder . The equation relating `alpha and beta` is
 02:54View Solution
02:54View SolutionA ball is placed on a smooth inclined plane of inclination `theta=30^(@)` to the horizontal, which is rotating at frequency `0.5` Hz about a vertical axiz passing through its lower end. At what distance from the lower end does the ball remain at rest?
02:37View SolutionA particle suspended by a light inextensible thread of length l is projected horizontally from its lowest position with velocity `sqrt(7gl//2)`. The string will slack after swinging through an angle equal to
07:45View SolutionThe kinetic energy of a particle moving along a circle of radius R depends on the distance covered s as T = KS2 where K is a constant. Find the force acting on the particle as a function of S -
02:52View Solution
Taking it together questions and complete solutions for chapter CIRCULAR MOTION of DC PANDEY PHYSICS (HINGLISH) of Class 11
All Exercises from DC PANDEY NEET Class 11 PHYSICS CIRCULAR MOTION
- Assertion And Reason
- Check point
- Comprehension
- Example
- Exercise 10.1
- Exercise 10.2
- Exercise 10.3
- Integer
- JEE Advanced
- JEE Main
- Level 1 Objective
- Level 1 Subjective
- Level 2 Comprehension Based
- Level 2 More Than One Correct
- Level 2 Single Correct
- Level 2 Subjective
- Match the columns
- Matrix Matching
- Medical entrances s gallery
- Miscellaneous Examples
- More than One Option is Correct
- Solved Examples
- Subjective Questions
- Taking it together
