Complete Solutions to TEXTUAL QUESTIONS SOLVED (NUMERICAL QUESTIONS) of chapter KINEMATICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | KINEMATICS |
| FULL MARKS | TEXTUAL QUESTIONS SOLVED (NUMERICAL QUESTIONS) |
| Number Of Questions | 44 |
| Academic Year | 2023-24 |
The position vectors particle has length 1m and makes `30^(@)` with the x-axis. What are the lengths of the x and y components of the position vector?
View SolutionA particle has its position moved from `vec(r_(1))=3hati+4hatj` to `vec(r_(2))=hati+2hatj`. Calculate the displacment vector `(Delta vecr)` and draw the `vec(r_(1)), vec(r_(2)) and Delta vecr` vector in a two dimensional Cartesian coordinate system.
View SolutionCalculate the average velocity of the particle whose position vector changes from `vec(r_(1))=5hati+6hatj" to "vec(r_(2))=2hati+3hatj` in a tine 5 second.
View SolutionConvert the vector `vecr=3hati+2hatj` into a unit vector.
View SolutionWhat are the resultants of the vector product of two given vectors. Given by
`vecA=4hati-2hatj+hatk and vecB=5hati+3hatj-4hatk`
View SolutionAn object at an angle such that the horizontal range is 4 time of the maximum height. What is the angle of projection of the object?
View SolutionThe following graphs represent velocity- time graph. Identify what kind of motion a particle undergoes in each graph
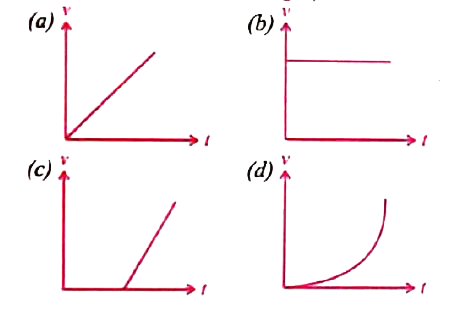
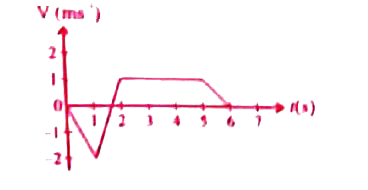
View SolutionThe following velocity-time graph represents a particle moving in the positive x-direction . Analyse its motion from 0 to 7s . Calculate the displacement covered and distance travelled by the particle from 0 to 2s
View SolutionA particle is projected at an angle of `theta` with respect to the horizontal direction. Match the following for the above motion.
(a) `V_(x)" "-" decrease and increases"`
(b) `V_(y)" "-" remains constant"`
(c) `"Acceleration - varies"`
(d) `"Position vector "-" remains downward"`
View SolutionA water fountain on the ground sprinkles water all around it. If the speed of the water coming out of the fountain is v. Calculate the total area around the fountain that gets wet.
View SolutionComplete the table.

View SolutionThe resultant of two vectors A and B is perpendicular to vector A and its magnitude is equal to half of the magnitude of vector B . Then the angle between A and B is :
(a) `30^(@)` (b) ` 45^(@)`
(c) `150^(@)` (d) `120^(@)`
View SolutionCompare the components for the following vector equations
(a) `Thatj-mghatj=mahatj` (b) `vecT+vecF=vecA+vecB` © `vecT-vecF=vecA-vecB` (d) `Thatj+mghatj=mahatj`
View SolutionCalculate the area of the triangle for which two of its sides are given by the vectors `vecA=5hati-3hatj, vecB=4hati+6hatj`.
View SolutionIf Earth completes one revolution in 24 hours, what is the angular displacement made by Earth in one hour? Express your answer in both radian and degree.
View SolutionAn object is thrown with initial speed `5ms^(-1)` with an angle of projection `30^(@)`. What is the maximum height and range reached by the particle?
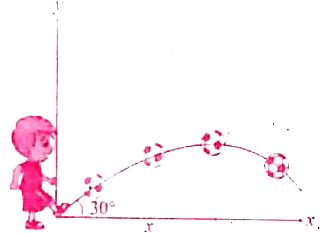
View SolutionA foot - ball player hits tha ball with speed `20ms^(-1)` with angle `30^(@)` with respect to horizontal direction as shown in the figure. The goal post is at distance of 40 m from him. Find out whether ball reaches the goal post
View SolutionIf an object is thrown horizontally with an initial speed `"10 ms"^(-1)` from the top of a building of height 100 m. What is the horizontal distance covered by the particle.
View SolutionAn object is executing uniform circular motion with an angular speed of `(pi)/(12)` radian per second. At `t=0`, the object starts at an angle` theta=0`. What is the angular displacement of the particle after 4s?
View SolutionConsider the x-axis as representing east, the y-axis as north and z-axis as vertically upwards. Give the vector representing each of the following points .
5 m north east and 2 m up,
View SolutionThe moon is orbiting the Earth approximately in 27 days, what is the angle transversed by the Moon per day?
View SolutionAn object of mass m has angular acceleration `alpha =0.2" rad s"^(-2)`. What is the angular displacement covered by the object after 3 second ? (Assume that the object started with angle zero with angular velocity).
View SolutionThe position vector of the particle has length 1 m and makes `30^(@)` with the x-axis. What are the lengths of the x and y-components of the position vector?

View SolutionA particle has its position moved from `vecr_(1)=3hati+4hatj` to `vecr_(2)=hati+2hatj`. Calculate the displacement vector `(Deltavecr)` and draw the `vecr_(1).vecr_(2)andDeltavecr` vector in a two dimensional Cartesian coordinate system.

View SolutionCalculate the average velocity of the particle whose position vector changes from `vec(r_(1))=5hati+6hatj" to "vec(r_(2))=2hati+3hatj` in a tine 5 second.
View SolutionConvert the vector `vecr=3hati+2hatj` into a unit vector.
View SolutionWhat are the resultants of the vector product of two given vectors. Given by
`vecA=4hati-2hatj+hatk and vecB=5hati+3hatj-4hatk`
View SolutionAn object at an angle such that the horizontal range is 4 time of the maximum height. What is the angle of projection of the object?
View SolutionThe following graphs represent velocity – time graph. Identify what kind of motion a particle undergoes in each graph

View SolutionThe following velocity-time graph represents a particle moving in the positive x-direction. Analyse its motion from 0 to 7 s. Calculate the displacement covered and distance travelled by the particle from 0 to 2 s.

View SolutionA particle is projected at an angle of `theta` with respect to the horizontal direction. Match the following for the above motion.
(a) `V_(x)" "-" decrease and increases"`
(b) `V_(y)" "-" remains constant"`
(c) `"Acceleration - varies"`
(d) `"Position vector "-" remains downward"`
View SolutionA water fountain on the ground sprinkles water all around it. If the speed of the water coming out of the fountain is v. Calculate the total area around the fountain that gets wet.
View SolutionThe following table gives the range of a particle when thrown on different planets. All the particles are thrown at the same angle with the horizontal and with the same initial speed. Arrange the planets in ascending order according to their acceleration due to gravity, (g value).

View SolutionThe resultant of two vectors A and B is perpendicular to vector A and its magnitude is equal to half of the magnitude of vector B . Then the angle between A and B is :
(a) `30^(@)` (b) ` 45^(@)`
(c) `150^(@)` (d) `120^(@)`
View SolutionCompare the components for the following vector equations
(a) `Thatj-mghatj=mahatj` (b) `vecT+vecF=vecA+vecB` © `vecT-vecF=vecA-vecB` (d) `Thatj+mghatj=mahatj`
View SolutionCalculate the area of the triangle for which two of its sides are given by the vectors `vecA=5hati-3hatj, vecB=4hati+6hatj`.
View SolutionIf Earth completes one revolution in 24 hours, what is the angular displacement made by Earth in one hour? Express your answer in both radian and degree.
View SolutionAn object is thrown with initial speed `5ms^(-1)` with an angle of projection `30^(@)`. What is the maximum height and range reached by the particle?
View SolutionA foot-ball player hits the ball with speed `20ms^(-1)` with angle `30^(@)` with respect to horizontal direction as shown in the figure. The goal post is at a distance of 40 m from him. Find out whether ball reaches the goal post?

View SolutionIf an object is thrown horizontally with an initial speed `"10 ms"^(-1)` from the top of a building of height 100 m. What is the horizontal distance covered by the particle.
View SolutionAn object is executing uniform circular motion with an angular speed of `(pi)/(12)` radian per second. At `t=0`, the object starts at an angle` theta=0`. What is the angular displacement of the particle after 4s?
View SolutionConsider the x - axis as representing east, the y - axis as north and z - axis as vertically upwards. Give the vector representing each of the following points and the direction is of 45°.
a) 5 m north east and 2 m up
b) 4 m south east and 3 m up
c) 2 m north west and 4 m up
View SolutionThe moon is orbiting the Earth approximately in 27 days, what is the angle transversed by the Moon per day?
View SolutionAn object of mass m has angular acceleration `alpha =0.2" rad s"^(-2)`. What is the angular displacement covered by the object after 3 second ? (Assume that the object started with angle zero with angular velocity).
View Solution
TEXTUAL QUESTIONS SOLVED (NUMERICAL QUESTIONS) questions and complete solutions for chapter KINEMATICS of FULL MARKS PHYSICS (TAMIL ENGLISH) of Class 11
All Exercises from FULL MARKS Class 11 PHYSICS KINEMATICS
- ADDITIONAL QUESTIONS SOLVED (I.MULTIPLE CHOICE)
- ADDITIONAL QUESTIONS SOLVED (LONG ANSWER QUESTIONS)
- ADDITIONAL QUESTIONS SOLVED (NUMERICAL QUESTIONS)
- ADDITIONAL QUESTIONS SOLVED (SHORT ANSWER QUESTIONS (1 MARK))
- ADDITIONAL QUESTIONS SOLVED (SHORT ANSWER QUESTIONS - 1 (2 MARKS))
- ADDITIONAL QUESTIONS SOLVED (SHORT ANSWER QUESTIONS - 1 (3 MARKS))
- IN-TEXT SOLVED EXAMPLES
- TEXTUAL QUESTIONS SOLVED (LONG ANSWER QUESTIONS)
- TEXTUAL QUESTIONS SOLVED (MULTIPLE CHOICE QUESTIONS:)
- TEXTUAL QUESTIONS SOLVED (NUMERICAL QUESTIONS)
- TEXTUAL QUESTIONS SOLVED (SHORT ANSWER QUESTIONS)