Complete Solutions to MULTIPLE CHOICE QUESTIONS of chapter STATISTICS of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | STATISTICS |
| ARYA PUBLISHERS | MULTIPLE CHOICE QUESTIONS |
| Number Of Questions | 31 |
| Academic Year | 2023-24 |
The measure of central tendency of a statistical data which takes into account all the data is
(i) mean
(ii) median
(iii) mode
(iv) range
View SolutionThe mean of five numbers is 30. If one number is excluded, their mean becomes 28. The excluded number is
(i) 28
(ii) 30
(iii) 35
(iv) 38
View SolutionThe mean of 100 observations is 50. If one of the observation which was 50 is replaced by 150, the resulting mean will be
(i) 50.5
(ii) 51
(iii) 51.5
(iv) 52
View SolutionIf `barx` is the mean of n observations `x_(1),x_(2),x_(3)……x_(n)`, then the value of `sum_(i=1)^(n)(x_(i)-barx)` is (i) `-1` (ii) 0 (iii) 1 (iv) n-1
View SolutionMedian of the numbers 4,4,5,7,6,7,7,3,12 is (i) 4 (ii) 5 (iii) 6 (iv) 7
View SolutionThe median of the data 78,56,22,34,45,54,39,68,54,84 is
(i) 45
(ii) 49.5
(iii) 54
(iv) 56
View SolutionThe most frequently occurring number in a set of value is called
(i) mean
(ii) median
(iii) mode
(iv) range
View SolutionMode of the data :15,14,19,20,14,15,16,14,15,18,19,15,17,15 is (i) 14 (ii) 15 (iii) 16 (iv) 17
View SolutionIf the mean of the following distribution is 2.6 Then the value of p is
Variable x: 1 2 3 4 5
frequency of x: 4 5 P 1 2
View SolutionThe time in seconds, taken by 150 athletes to run a 110 m hurdle race are tabulated below:

How many athletes completed the race in less than 14.6sec?
View SolutionConsider the following frequency distribution

The upper limit of the median class in
View SolutionConsider the data
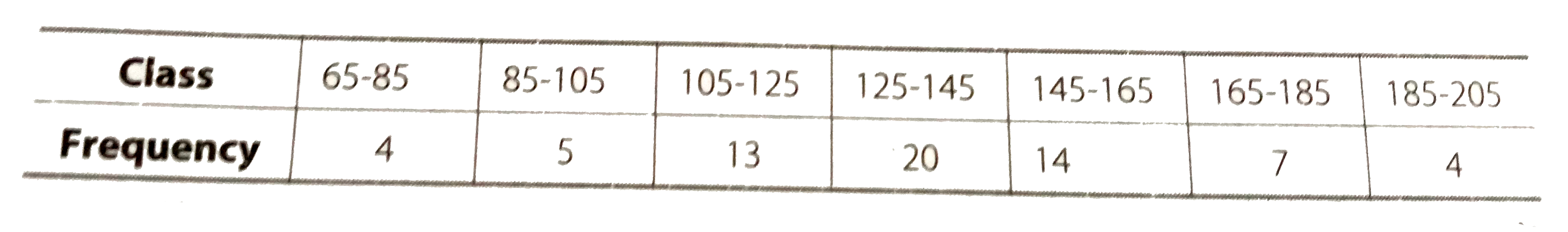
The frequency of the upper limit of the median class and the lower limit of the modal class is
View SolutionThe mean deviation for n observations `x_(1),x_(2)…….x_(n)` from their median M is given by
(i) `sum_(i=1)^(n)(x_(i)-M)`
(ii) `(1)/(n)sum_(i=1)^(n)|x_(i)-M|`
(iii) `(1)/(n)sum_(i=1)^(n)(x_(i)-M)^(2)`
(iv) `(1)/(n)sum_(i=1)^(n)(x_(i)-M)`
View SolutionThe mean deviation of the data 4,5,7,8,9,10,6 from the median is
View SolutionThe mean deviation of the data 4,5,7,8,9,10,6 from the median is
View SolutionThe variance of first 5 natural numbers is
(i) 1
(ii) 2
(iii) 3
(iv) 4
View SolutionThe standard deviation of first 11 nutural numbers is
(i) 2
(ii) `2sqrt2`
(iii) 3
(iv) `sqrt10`
View SolutionIf for a distribution `sum(x-7)=6` and `sum(x-7)^(2)=78` and the total number of items is 12 then mean and standard deviation are
View SolutionIf for a distribution `sumx_(i)^2=2400` and `sumx_(i)=250` and the total number of observations is 50, then variance is
View SolutionThe mean of 100 observations is 50 and their standard deviation is 5. the sum of squares all the observations is
(i) 50000
(ii) 250000
(iii) 252500
(iv) 255000
View SolutionThe mean of 100 observations is 40 and their standard deviation respectively is 10. if 5 is added to each observation then the new mean and new standard deviation will be
View SolutionThe mean of 5 observations is 4.4 and variance is 8.24. If three of the five observations are 1,2, and 6 then remaining two observations are
(i) 9, 16
(ii) 9, 4
(iii) 81, 16
(iv) 81, 4
View SolutionLet `x_(1),x_(2),x_(3),……..,x_(n)` be n observations with mean `barx` and standard deviation `sigma`. The mean the standard deviation of `kx_(1),kx_(2),……,kx_(n)` respectively are
(i) `barx,ksigma`
(ii) `kbarx,sigma`
(iii) `kbarx,ksigma`
(iv) `barx,sigma`
View SolutionIf `x_(1),x_(2),x_(3),.........,x_(n)` be n observations with mean `barx` and variance `sigma^(2)`. The mean and variance of `x_(1)+k,x_(2)+k,x_(3)+k,......,x_(n)+k` respectively are (i) `barx+k,sigma^(2)` (ii) `barx+k,sigma^(2)+k^(2)` (iii) `barx+k,(sigma+k)^(2)` (iv) `barx,sigma^(2)`
View SolutionConsider the numbers 1,2,3,4,5,6,7,8,9,10. If 2 is added to each number then variance of the numbers so obtained is
(i) 6.5
(ii) 2.87
(iii) 3.87
(iv) 8.25
View SolutionA set of n values `x_(1),x_(2),…….,x_(n)` has standard deviation `sigma`. The standard deviation of n values `x_(1)-k,x_(2)-k,…….,x_(n)-k` is
View SolutionA set of n value `x_(1),x_(2),……..,x_(n)` has mean `barx` and standard deviation `sigma`. The mean and standard deviation of n values `(x_(1))/(k),(x_(2))/(k),.......,(x_(n))/(k)(kne0` respectively are
(i) `kbarx,(sigma)/(k)`
(ii) `(barx)/(k),(sigma)/(k)`
(iii) `kbarx,ksigma`
(iv) `(barx)/(k),ksigma`
View SolutionCalculate the possible values of x, if the standard deviation of the numbers 2, 3, 2x and 11 is 3.5.
View SolutionThe coefficient of variation of two distributions are 70 and 75 and their standard deviations are 28 and 27 respectively. The difference of their arithmetic means is
View SolutionThe coefficient of variation of distributions are 50 and 60 and their arithmetic means are 30 and 25 respectively. The difference of their standard deviations is
View SolutionLet `x_(1),x_(2),……,x_(n)` be n observations and `barx` be their arithmetic mean. The formula for the standard deviation is
View Solution
MULTIPLE CHOICE QUESTIONS questions and complete solutions for chapter STATISTICS of S CHAND MATHS (ENGLISH) of Class 11