Complete Solutions to Example of chapter Elasticity of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | Elasticity |
| MODERN PUBLICATION | Example |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
A wire increases by `10^-3` of its length when a stress of `10^8 Nm^-2` is applied on it what is the Young's modulus of the material of the wire?
View SolutionFind the bulk modulus of water from the following data : Initial volume = 500.0 litre, pressure increase-100.0 atm, final volume-497.5 litre.
View SolutionA cube of aluminium of each side 4 cm is subjected to a tangential (shearing) force. The top face of the cube is sheared through 0.012 cm with respect to the bottom Find shearing strain Given `nu =2.08 xx 10^11` dyne `cm^2`
View SolutionA cube of aluminium of each side 4 cm is subjected to a tangential (shearing) force. The top face of the cube is sheared through 0.012 cm with respect to the bottom Find shearing stress and shearing force. Given `nu =2.08 xx 10^11` dyne `cm^2`
View SolutionA steel wire of length 3.6 m and cross section `2.5 xx 10^-5 m^2` stretches by the same amount as a copper wire of length 2.4 m and cross-section `3.2 xx 10^3 m^2` under a given load. What is the ratio of the Young's modulus of steel to that of copper?
View SolutionIf a compressive force of `3.0 xx 10^4` N is exerted on the end of a 20 cm long bone of cross-sectional area `3.6 cm^2`. will the bone break? Given, compressive strength of bone = `7.7 xx 10^8 N m^2` and Young's modulus of bone `1.5 xx 10^10 Nm^2`
View SolutionIf a compressive force of `3.0 xx 10^4` N is exerted on the end of a 20 cm long bone of cross-sectional area `3.6 cm^2`. if the bone do not break, by how much does it shorten ? Given, compressive strength of bone = `7.7 xx 10^8 N m^2` and Young's modulus of bone `1.5 xx 10^10 Nm^2`
View SolutionA hollow cylindrical column of steel support a load of 16,000 kg. The inner and outer radii of the column are 40 cm and 50 cm respectively. Assuming the load distribution to be uniform, calculate the compressional strain of the column. Given that Young's modulus of steel = `2.0 xx 10^41` Pa.
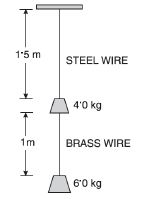
View SolutionFind the elongation of the steel and brass wire in the Fig. Unloaded length of steel wire-1.5 m, unloaded length of brass wires 1 m, diameter of each wire 0.3 cm. Young's modulus for steel-`20x10^10 Pa` and that for brass= `90x10^10Pa`
View SolutionA mass of 5.0 kg is hung from a copper wire of 5 mm diameter and 2 m in length. Calculate the extension produced. What should be the minimum. diameter of the wire, so that its elastic limit is not exceeded? Elastic limit for copper=`1.5 x 10^ 9 dyne cm^-2` and Y for copper-1.1 x10^12 dyne cm^2.
View SolutionA 45 kg traffic light is suspended with two steel wires of equal lengths and radii 0.5cm. if the wires make an angle of `15^@` with the horizontal, what is the fractional increase in their length due to the weight of the light? given youngs modulus of Steel = `2xx10^11 Nm^-2`
View SolutionA wire suspended vertically from one of its ends is stretched by attaching a weight of 200 N to the lower end. The weight stretches the wire by 1 mm. Find the elastic energy stored in the wire.
View SolutionIf S is stress and Y is Young's modulus of material of a wire, find the energy stored in the wire per unit volume in terms of S and Y.
View SolutionBy how much the pressure on one litre of water be changed to compress it by 0.1 % ? Given that bulk modulus of the water - `2.2 xx 10^9` Pa.
View SolutionThe pressure of a medium is changed from `1.01 xx 10^5`Pa to `1.165 xx 10^5` Pa and change in volume is 10% keeping temperature constant. Find the bulk modulus of the medium.
View SolutionTwo metal plates are held together by two rivets width radii of 0.2 cm. If the maximum shear stress a single rivet can withstand is `5xx10^8N m^2`, how much force must be applied parallel to the plates to shear off both the rivets?
View SolutionA square lead slab of side 50 cm and thickness 50 cm is subjected to a shearing force (on its narrow face) of magnitude `9.0 xx 10^4 N`. The lower edge is riveted to the floor. How much is the upper edge displaced, if the shear modulus of lead is `5.6xx 10^9` Pa?
View SolutionA stone of 0.5 kg mass is attached to one end of a 0.8 m long aluminium wire of 0.7 mm diameter and suspended vertically . The stone is now rotated in a horizontal plane at a rate such that the wire makes an angle of `85^@` with the vertical . find the increase in the length of the wire . The Young's modulus of aluminium = `7 xx 10^(10) Nm^(-2)`, `sin 85^@`= 0.9962 , cos `85^@` = 0.0872
View SolutionA load of 31.4 kg is suspended from a wire of radius `10^-3` m and density `9 xx 10^3 kg m^-3`, Calculate the change in temperature of the wire, if 75% of the work done is converted into heat. Given that Young's modulus and heat. capacity of the material of the wire are `9.8 xx 10^10 Nm^-2` and `490 Jkg^-1 K^-1` respectively.
View SolutionWhat is deforming force?
View Solutionwhich is a property of a body that opposes its deformation?
View Solutionunder what condition, the restoring forces are equal and opposite to the external deforming force?
View SolutionWhich of the two forces deforming or restoring is responsible for elastic behaviour of a substance?
View SolutionState Hooke's law.
View Solutionwhat is the limitation of hooke`s law?
View Solutiondefine stress and give its SI unit
View Solutionwhat are the factors on which modulus of elasticity of a material depends?
View SolutionWhy do we prefer steel to manufacture a spring?
View SolutionIn stretching a wire, work has to be performed. Why?
View SolutionWhen a wire is stretched, work has to be done. What happens to the work done during the stretching of the wire?
View SolutionWhich of the three elastic moduli possible in all the three states of matter?
View SolutionA hard wire is broken by bending it repeatedly in opposite direction. Why?
View Solutionwhat is meant by elastic fatigue?
View Solutionwhy any metallic part of a machinery is never subjected to a stress beyond the elastic limit of the material?
View SolutionWhat is an elastomer?
View SolutionWhy are electric poles given hollow structure?
View SolutionName factors which affect the property of elasticity of a solid.
View SolutionDistinguish between elasticity and plasticity.
View SolutionElasticity has different meaning in physics and in our daily life. Comment.
View SolutionWhat is the cause of elasticity?
View Solutionrepresent graphically the variation of extension with load in an elastic body. On the graph, mark: Hooke's law region
View Solutionrepresent graphically the variation of extension with load in an elastic body. On the graph, mark: Elastic limit
View Solutionrepresent graphically the variation of extension with load in an elastic body. On the graph, mark: Yield point
View Solutionrepresent graphically the variation of extension with load in an elastic body. On the graph, mark: breaking point
View SolutionDraw a schematic of a graph of the applied force versus resulting elongation of a metallic wire. Show the elastic limit on this graph. What feature of this graph is related to the value of Young's modulus of material of wire?
View SolutionThe stress-strain graph for a metal wire shown in the Fig. Upto the point E, the wire returns to its original state O along the curve EPO, when it is gradually unloaded. The point B corresponds to the fracture of the wire. Upto what point on the curve is Hooke's law obeyed? (This point is sometimes called 'proportional limit').
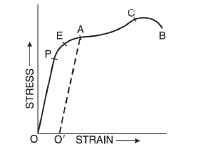
View SolutionThe stress-strain graph for a metal wire shown in the Fig. Upto the point E, the wire returns to its original state O along the curve EPO, when it is gradually unloaded. The point B corresponds to the fracture of the wire. Which point on the curve corresponds to 'elastic limit' or 'yield point of the wire ?
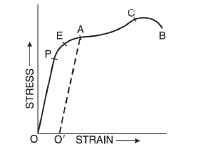
View SolutionThe stress-strain graph for a metal wire shown in the Fig. Upto the point E, the wire returns to its original state O along the curve EPO, when it is gradually unloaded. The point B corresponds to the fracture of the wire. Indicate the elastic and plastic regions of the stress-strain graph.
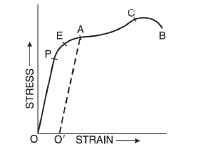
View SolutionThe stress-strain graph for a metal wire shown in the Fig. Upto the point E, the wire returns to its original state O along the curve EPO, when it is gradually unloaded. The point B corresponds to the fracture of the wire. Describe what happens, when the wire is loaded up to a stress corres-ponding to the point A on the graph and then unloaded gradually. In particular, explain the dotted curve.
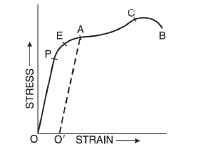
View SolutionThe stress-strain graph for a metal wire shown in the Fig. Upto the point E, the wire returns to its original state O along the curve EPO, when it is gradually unloaded. The point B corresponds to the fracture of the wire. Indicate the elastic and plastic regions of the stress-strain graph.

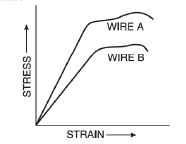
View SolutionThe stress versus strain graphs for wires of two materials A and B are as shown in Fig. Which material is more ductile ?
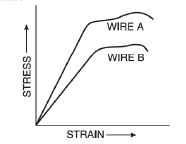
View SolutionThe stress versus strain graphs for wires of two materials A and B are as shown in Fig. Which material is more brittle?
View SolutionWhy do we prefer steel to manufacture a spring?
View SolutionThe length of a wire increases by 8mm. Then a weight of 3 kg is hung. if all conditions are the same, what will be the increase in its length when diameter is doubled?
View SolutionA wire stretches by a certain amount under a load. If the load and radius are both increased to four times, find the stretch caused in the wire.
View SolutionTwo wires of different materials are suspended from a rigid support. They have same length and diameter and carry the sameload at the free ends. Will extension, strain and stress be different?
View SolutionSteel is more elastic than rubber. Explain why?
View SolutionThe length of a metallic wire is `L_1` : when tension on the wie is `T_1` , and length is `L_2`, when the tension in the wire is `T_2` , Find original length of the wire.
View SolutionAn elastic wire is cut to half its original length. How would it affect the maximum load that the wire can support?
View SolutionThe breaking force for a wire is F. what will be the breaking force for two parallel wires of the same size?
View SolutionThe breaking force for a wire is F, what will be the breaking force for a single wire of double the thickness?
View SolutionA cable is replaced by another of the same length and material but of twice diameter.
How does this affect elongation under a given load?
View SolutionA cable is replaced by another of the same length and material of twice diameter. How many times will be the maximum load supported by the latter as compared to the former?
View SolutionWhy do spring balances show wrong readings after they have been used for a long time?
View SolutionWhy bridges are declared unsafe after long use?
View SolutionRead the following two statements below carefully and state, with reasons, if it is true or false. The Young’s modulus of rubber is greater than that of steel,
View SolutionRead the following two statements below carefully and state, with reasons, if it is true or false. The stretching of a coil is determined by its shear modulus.
View Solutionread the statement below carefully and state with reasons if it is true or false. When a material is under tensile stress the restoring forces are caused by Inter atomic attraction while under compressional stress the restoring forces are due to interatomic repulsion.
View SolutionGraphite consists of plains of carbon atoms. sach carbon atoms in the plains are held by very weak forces. what kind of elastic properties do you expect from graphite?
View SolutionWhy a solid is ductile or brittle?
View SolutionFind stress, strain and Young's modulus of elasticity in the case of a wire 1.5 m long and `1 mm^2` in cross-section, if it increases by 1.55 mm in length, when a weight of 10 kg is suspended from it.
View SolutionA structural steel rod has a radius of 10 mm and a length of 1.0 m. A 100 kN force stretches it along its length. Calculate stress. Young's modulus of structural steel is `2.0 xx 10^(11) N m^(-2)`
View SolutionA structural steel rod has a radius of 10 mm and a length of 1.0 m. A 100 kN force stretches it along its length. Calculate elongation. Young's modulus of structural steel is `2.0 xx 10^(11) N m^(-2)`
View SolutionA structural steel rod has a radius of 10 mm and a length of 1.0 m. A 100 kN force stretches it along its length. Calculate strain on the rod. Young's modulus of structural steel is `2.0 xx 10^(11) N m^(-2)`
View SolutionWhat force is required to stretch a copper wire `1 cm^2` in cross-section to double its length? Y for copper is `1.26 xx 10^12` dyne. cm
View SolutionDetermine the force required to double the length of a steel wire of area of cross section `5xx10^-5 m^2` Young's modulus of steel- `2xx 10^11 Nm^-2`
View SolutionA load of 2 kg produces an extension of 1 mm in a wire 3 mm in length and 1 mm in diameter Calculate the Young's modulus of the wire
View SolutionCalculate the percentage increase in length of a wire of diameter 2 mm stretched by force of 1 kgf. Young's modulus of the material of wire `15 xx10^10 Nm^-2`
View SolutionFind the maximum length of the steel wire that can hang without breaking. Given that breaking stress for steel `7.9 x 10^11 Nm^-2` and density of steel- `7.9xx 10^3 kgm^-3`
View SolutionA silica glass rod has a diameter of 1 cm and is 10 cm long. Estimate the largest mass that can be hung from it without breaking it. Given that Young's modulus of silica -6.5 xx10^10 Nm^-2` and breaking stress for silica -`5.0xx10^7` Nm^-2`
View SolutionA steel wire of length 5.0 m and cross-section `3.0xx10^(-5) m^(2)` stretches by the same amount as a copper wire of length 3.0 m and cross-section `4.0xx10^(-5)m^(2)` under a given load. What is the ratio of Young's modulus of steel to that of copper?
View SolutionA mass of 5.0 kg is hung from a copper wire of 5 mm diameter and 2 m in length. Calculate the extension produced. What should be the minimum. diameter of the wire, so that its elastic limit is not exceeded? Elastic limit for copper=`1.5 x 10^ 9 dyne cm^-2` and Y for copper-1.1 x10^12 dyne cm^2.
View SolutionThe average depth of Indian ocean is 3 km. Find the fractional compression of water at the bottom of the ocean, given that bulk modulus of water id `2.2 xx 10^9 Nm^-2`.
View SolutionA solid ball 3 cm in diameter is submerged in a lake to a depth, where the pressure is `10^4 kgf m^-2` Find the change in volume of the ball, if bulk modulus of the material of the ball is `10^6Nm^-2`
View SolutionWhen a metallic cube is subjected to a stress of `6xx10^9 Nm^-2`, each side of the cube gets shortened by 1%. Find volumetric strain and bulk modulus of the metal.
View SolutionA solid ball 300 cm in diameter is submerged in a lake a such a depth that the pressure exerted by water is `1.0 kgf cm^(-2)`. Find the change in volume of the ball at this depth k for material of the ball =`1.0xx10^(13) dyne cm^(-2)`
View SolutionA sphere contracts to 0.01% volume, when taken to the bottom of sea 1 km deep. Find the bulk modulus of the material of the sphere. Density of sea water = `1.0xx10^3 kg//m^3`.
View SolutionIf the normal density of sea water is `1.00 g//cm^(3)`, what will be its density at a depth of 3km? Given compressibility of water = `5xx10^-5` per atmosphere. 1 atmospheric pressure =`10^(6) dyne//cm^(2)`, g= `980 cm//s^(2)`.
View SolutionWhat is the density of lead under a pressure of `2xx10^(8)Nm^(-2)` , if the bulk modulus of lead is `8xx10^(9)Nm^(-2)` and normal density of lead is `11.4 g cm^(-3)`?
View SolutionA metallic cube whose each side is 10 cm is subjected to a shearing force of 100 kgf. The top face is displaced through 0.25 cm with respect to the bottom ? Calculate the shearing stress, strain and shear modulus.
View SolutionAn Indian rubber cube of side 7 cm has one side fixed, while a tangential force equal to the weight of 200 kilogram is applied to the opposite face. Find the shearing strain produced and distance through which th strained side moves. Modulus of rigidity for rubber is `2xx10^(7) dyne cm^(-2)`
View SolutionThe Young's modulus for Steel is `2.0 xx 10^11 N m^-2`. If the interatomic spacing for the metal is `2.8 overset@A`, find the increase in the interatomic spacing for a force of `10^9N m^-2`
View SolutionA stress of `10^(6) N//m^(2)` is required for breaking a material. If the density of the material is `3 xx 10^(3) Kg//m^(3)`, then what should be the minimum length of the wire made of the same material so that it breaks by its own weight `(g = 10m//s^(2))`
View SolutionAn Indian rubber cord 10 m long is suspended vertically How much does it stretch under its own weight Density of rubber=`1.5xx10^3 kg m^-3` and Young's modulus of rubber - `5xx10^8 Nm^-2`
View SolutionA mass of 100 g is attached to the end of a rubber string 49 cm long and of cross-sectional area 20 `mm^2` When the mass is whirled round at a constant angular speed of 40 rps, it moves along a circular path of radius 51 cm. Find young's modulus of rubber.
View SolutionA rubber cord catapult has a cross sectional area `1mm^2` and total unstretched length of 10cm. It is stretched to 12cm and then released to project a missile of mass 5g. Find the velocity of projection of missile and tension in the cord. Young's modulus = `5xx10^8 N m^-2`
View SolutionTwo parallel steel wires A and B are fixed to rigid support at the upper ends and subjected to the same load at the lower ends The lengths of the wires are in the ratio 4:5 and their radii are in the ratio 4:3. The increase in the length of the wire A is 1 mm. Calculate the increase in the length of the wire B.
View SolutionA steel wire and copper wire of the same diameter and of length 1m and 2m respectively are connected end to end and a force is applied. The increase in length of the combination is 0.01m.Calculate the elongation produced in the individual wires. Given that Y of copper is 12 x 10^(10) Nm^(2) and that of steel is 20 x 10^(10) N m^(-2).
View SolutionA composite wire of uniform diameter 3 mm consists of a copper wire of length 2.2 m and a steel wire of length 1.6m stretches under a load by 0.7 mm Calculate the load, given that the Young's modulus for copper in `1.1xx10^11` Pa and that for steel is `20xx10^11` Pa.
View SolutionA wire loaded by a weight of density `7.8g cm^-3` is found to be of length 95cm. On immersing the weight in water, the length decreased by 0.19cm. Find the original length of the wire.
View Solution
Example questions and complete solutions for chapter Elasticity of MODERN PUBLICATION of Class 11