Complete Solutions to Solved Example of chapter CONIC SECTION of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | CONIC SECTION |
| NAGEEN PRAKASHAN | Solved Example |
| Number Of Questions | 59 |
| Academic Year | 2023-24 |
Find the equation of a circle whose centre is (2, 3) and radius is 5.
View SolutionFind the centre and radius of the circle `(x-2)^(2)+(y+5)^(2)=49`
View SolutionFind the centre and radius of the circle `(x+2)^(2)+(y+3)^(2)=5`
View SolutionFind the equation of a circle which touches the `X-axis` and whose centre is `(1,2)`.
View SolutionFind the radius and centre of the circle of the circle `x^(2) + y^(2) + 2x + 4y -1=0`.
View SolutionFind the centre and radius of the circle `3x^(2)+ 3y^(2) - 6x +9y - 8 =0`.
View SolutionFind the equation of a circle which passes through the origin and whose centre is `(a, b).`
View SolutionProve that the radii of the circles `x^(2)+y^(2)=1,x^(2)+y^(2)-2x-6y=6and x^(2)+y^(2)-4x-12y-9=0` are in arithmetic progression.
View SolutionProve that the circle `x^(2)+y^(2)+2x+2y+1=0` and circle `x^(2)+y^(2)-4x-6y-3=0` touch each other.
View SolutionFind the equation of a circle which passes through the point `(3, 2)` and concentric with the circle `x^(2)+y^(2)-4x +2y - 1=0`.
View SolutionFind the equation of a circle which passes the centre of the circle `x^(2)+y^(2)-6x =1` and concentric with the circle `2x^(2)+2y^(2)-8x +12y -1=0`.
View SolutionFind the equation of a circle, the co-ordinates of the ends of whose diameter are `(-1,-3) and (2,5)`
Now the equation of circle
`(x-x_(1))(x-x_(2))+(y-y_(1))(y-y_(2))=0`
`rArr(x+1)(x-2)+(y+3)(y-5)=0`
`rArr x^(2)-x-2+ y^(2)-2y-15=0`
`rArr x^(2)+y^(2)-x-2y-17=0`
We is the required equation.
View SolutionIn `DeltaABC,angleA=90^(@)` and the co-ordinates of points B and C are `(2,-4) and (1,5)`. Find the equation of the circumcircle of `Delta ABC`.
View SolutionPQRS is a square of side 'b'. Prove that the equation of circumcircle of square PQRS, where PQ and PS are the axes, is `x^(2)+y^(2)=b(x+y)`.
View Solution`y=2x` be a chord of the circle `x^(2) +y^(2)=10x`. Find the equation of a circle whose diameter is this chord.
View SolutionFind the equation of circle of a circle which passes through the points `(0,-6), (1,1) and (7,-7)`.
View SolutionFind the equation of the circle which passes through the points `(1,-2),(4,-3)` and whose center lies on the line `3x+4y=7.`
View SolutionFind the equation of circumcircle of the triangle whose sides are `2x +y = 4,x + y = 6 and x +2y=5`.
View SolutionFind the parametric equations of the circles `x^(2)+y^(2)=16`.
View SolutionFind the position of the point `(-2,3)` with respect to the circle `x^(2) +y^(2)=25`.
View SolutionFind the equation of a circle, the equation of whose two diameters are `x + y = 6 and 3x +4y =16` and radius is 10.
View SolutionIf the circles `x^(2)+y^(2)+2gx+2fy=0and x^(2)+y^(2)+2g'x+2f'=0` touch each other then prove that `f' f = fg`.
View SolutionFind the length of chord cut on x-axis from the circle `x^(2)+y^(2)-5x-2y+6=0`.
View SolutionFind the vertex, focus, equation of axis, equation of axis, equation of directrix, length of latus rectum and the co-ordinates of the ends of latus rectum for the following parabolas :
(i) `y^(2) =8x`
(ii) `y^(2) = -12x`
(iii) `x^(2)=6y`
(iv) `x^(2)=-2y`.
View SolutionFind the equation of parabola whose focus is (2,0) and equation of directrix is x=-2.
View SolutionFind the equation of a parabola whose focus and vertex are (0, 0) and (0, 2) respectively.
View SolutionFind the vertex, focus, axis , latus rectum and directrix of the parabola
`y^(2)+4x+6y+17=0`
View SolutionThe focal distance of a point of a parabola `y^(2)=8x` is 5. Find the abscissa of that point.
View SolutionFind the equation of a line joining the vertex of parabola `y^(2)=8x` to its upper end of latus rectum.
View SolutionFind the equation of a parabola whose vertex is `(-2,0)` and focus is `(0,0)`.
View SolutionFind the equation of the parabola whose is (2,-3) and directrix is x+4=0.
View SolutionFind the equation of a parabola whose focus is `(-8,-2)` and equation of directrix is `y = 2x-9`.
View SolutionIf the co-ordinates of one end of a focal chord of a parabola `y^(2)`=4ax are `(at^(2),2at)`, find the co-ordinates of its other end.
View SolutionShow that the parametric point `(2+t^(2),2t+1)` represents a parabola. Show that its vertex is (2,1).
View SolutionIf the parabola `y^(2)=4ax` passes through the (4,-8) then find the length of latus rectum and the co-ordinates of focus.
View SolutionShow that the locus of the mid-points of all chords passing through the vertices of the parabola `y^(2)`=4ax is the parabola `y^(2)=2ax`.
View SolutionFind the equation of that focal chord of the parabola `y^(2)=8x` whose mid-point is (2,0).
View SolutionFind the equation of the ellipse whose major axis is `8` and minor axis is `4`.
View SolutionFind the co-ordinates of vertices, length of major and minor axes, eccentricity, co-ordinates of foci, equation of directrices and length of latus rectum for each of the following ellipse :
(i) `(x^(2))/(49)+y^(2)/(25)=1`
(ii) `(x^(2))/(4)+(y^(2))/(9)=1`
View SolutionFind the equation of ellipse whole foci are `(pm1,0)` and eccentricity is `(1)/(3)`.
View SolutionFind the equation of the ellipse whose vertices are `(+-13 ,0)`and foci are `(+-5,0)`.
View SolutionFind the equation of ellipse whose vertices are `(0,pm13)` and foci `(0,pm5)`.
View SolutionFind the equation of the ellipse whose major axis is 8 and co-ordinates of foci are `(pm2,0)`
View SolutionFind the equation of the ellipse whose is (5,6), equation of directrix x+y+2=0 and eccentricity is `(1)/(2)`.
View SolutionFind the equation of the ellipse whose centre is at origin, focus is (0,4) and major axis is 10.
View SolutionFind the equation of an ellipse the distance between the foci is 8 units and the distance between the directrices is 18 units.
View SolutionFind the equation of the ellipse whose centre is at origin, the distance between foci is `2` and eccentricity is `(1)/(sqrt(2))`.
View SolutionProve that the curves x=4 `(costheta+sintheta)` and y=3 `(costheta-sintheta)` represents an ellipse.
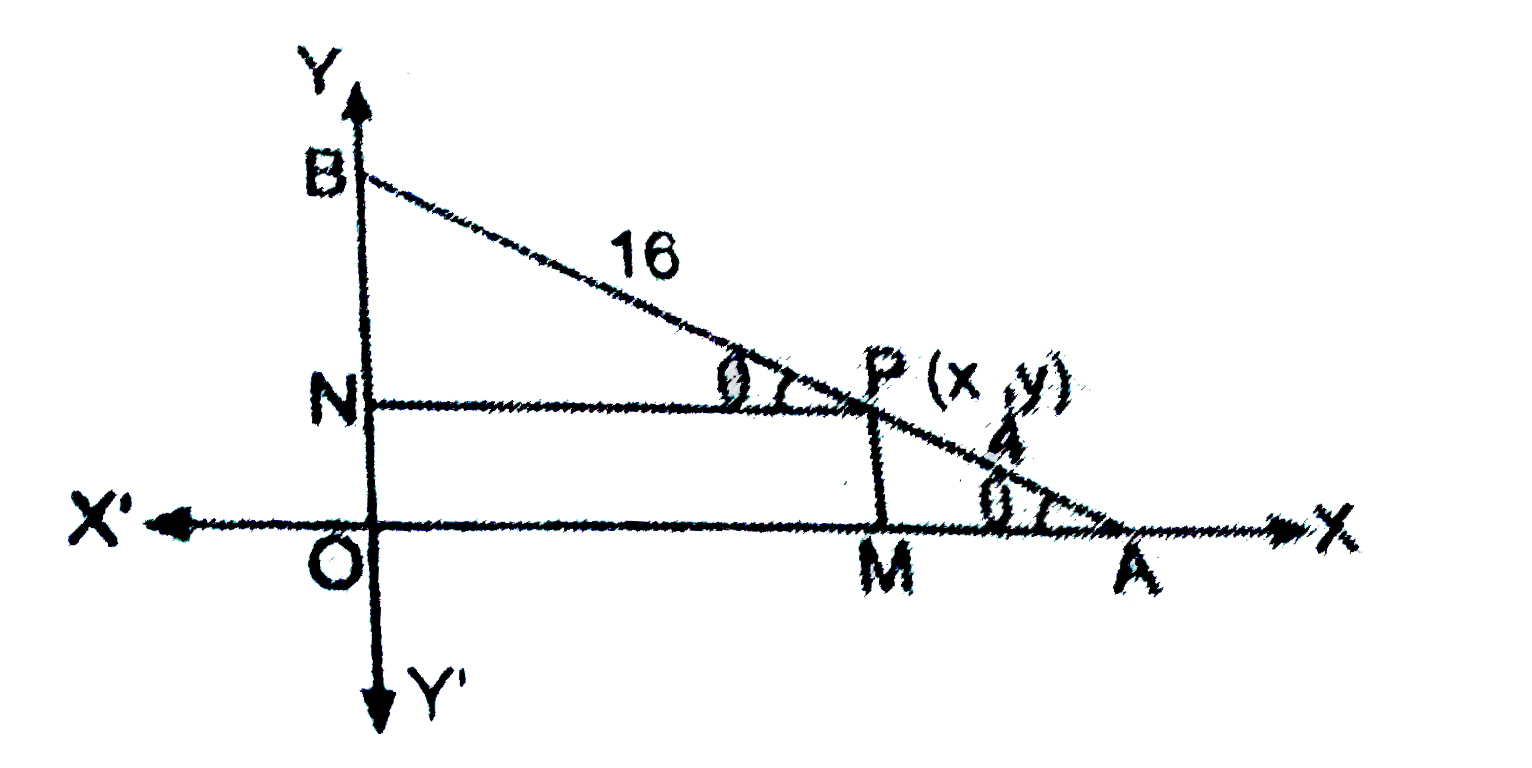
View SolutionThe ends of 20 cm long rod moves on two mutually perpendicular lines. If a point on this rod at 4 cm distance from end then from the eccentricity of the ellipse formed by moving that point.
View SolutionIf `e_(1)ande_(2)` be the eccentricities of the ellipses `(x^(2))/(a^(2))+(4y^(2))/(b^(2))=1and(x^(2))/(a^(2))+(4y^(2))/(b^(2))=1` respectively then prove that `3=4e_(2)^(2)-e_(1)^(2)`.
View SolutionFind the equation of the hyperbola whole transverse and conjugate axes are 8 and 6 respectively.
View SolutionFind the vertices, eccentricity foci, rquation of directrices and length of latus rectum of the hyperbola `9x^(2)-25y^(2)=225`.
View SolutionFind the vertices, eccentricity, foci, equation of directrices length of latus rectum for the hyperbola `3y^(2)-x^(2)=27`.
View SolutionFind the equation of hyperbola whose vertices are `(0,pm5)` and are `(0,pm10)`
View SolutionFind the equation of the hyperbola where foci are `(0,+-12)`and the length of the latus rectum is 36.
View SolutionFind th equation of the hyperbola eccentricity is 2 and the distance between foci 16.
View SolutionFind the equation of the hyperbola whose focus is (1,1), eccentricity is 2 and equation of directrix is x+y+1=0.
View SolutionThe difference of the distances of variable point from two given points (3,0) and (-3,0) is 4. Find the locus of the point.
View SolutionIn a rectangular hyperbola `x^(2)-y^(2)=a` , prove that `SP*S'P=CP^(2)` where P is any point on the hyperbola, C is origin and S and S' are foci.
View Solution
Solved Example questions and complete solutions for chapter CONIC SECTION of NAGEEN MATHS (HINGLISH) of Class 11