Complete Solutions to LEVEL-I (H.W) of chapter MECHANICAL PROPERTIES OF SOLIDS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MECHANICAL PROPERTIES OF SOLIDS |
| NARAYANA | LEVEL-I (H.W) |
| Number Of Questions | 38 |
| Academic Year | 2023-24 |
A steel wire of `2mm` in diameter is stretched by applying a force of `72N`. Stress in the wire is
View SolutionThe length of a wire under stress changes by `0.01%`.The strain produced is
View SolutionAn air filled balloon is at a depth of `1 km` below the water level in an ocean . The normal stress of the balloon (in Pa) is
(Given,` rho_("water") = 10^(3) kgm^(-3), g = 9.8 ms^(-2)` and `P_(atm) = 10^(5) Pa)`
View SolutionA wire of `10m` long and `1mm^(2)` area of cross section is strechted by a force of `20N`. If the elongation is `2mm` the young's modulus of the material of the wire (in `Pa`) is
View SolutionThe area of cross-section of a wire is `10^(-5) m^(2)` when its length is increased by `0.1%` a tension of `1000N` is produced. The Young's modulus of the wire will be (in `Nm^(-2)`)
View SolutionThere are two wires of same material. Their radii and lengths are both in the ratio `1:2` If the extensions produced are equal, the ratio of the loads is
View SolutionIf stress is numbercially equal to young's modulus, the elongation will be
View SolutionTwo wires of the same length and same material but radii in the ratio of `1:2` are stretched by unequal forces to produce equal elongation. The ratio of the two forces is
View SolutionA steel wire fo length `5m` and cross-sectional area `2xx10^(-6)m^(2)` streches by the same amount as a copper wire of length `4m` and cross sectional area of `3xx10^(-6) m^(2)` under a given load. The ratio of young's mouduls of steel to that of copper is
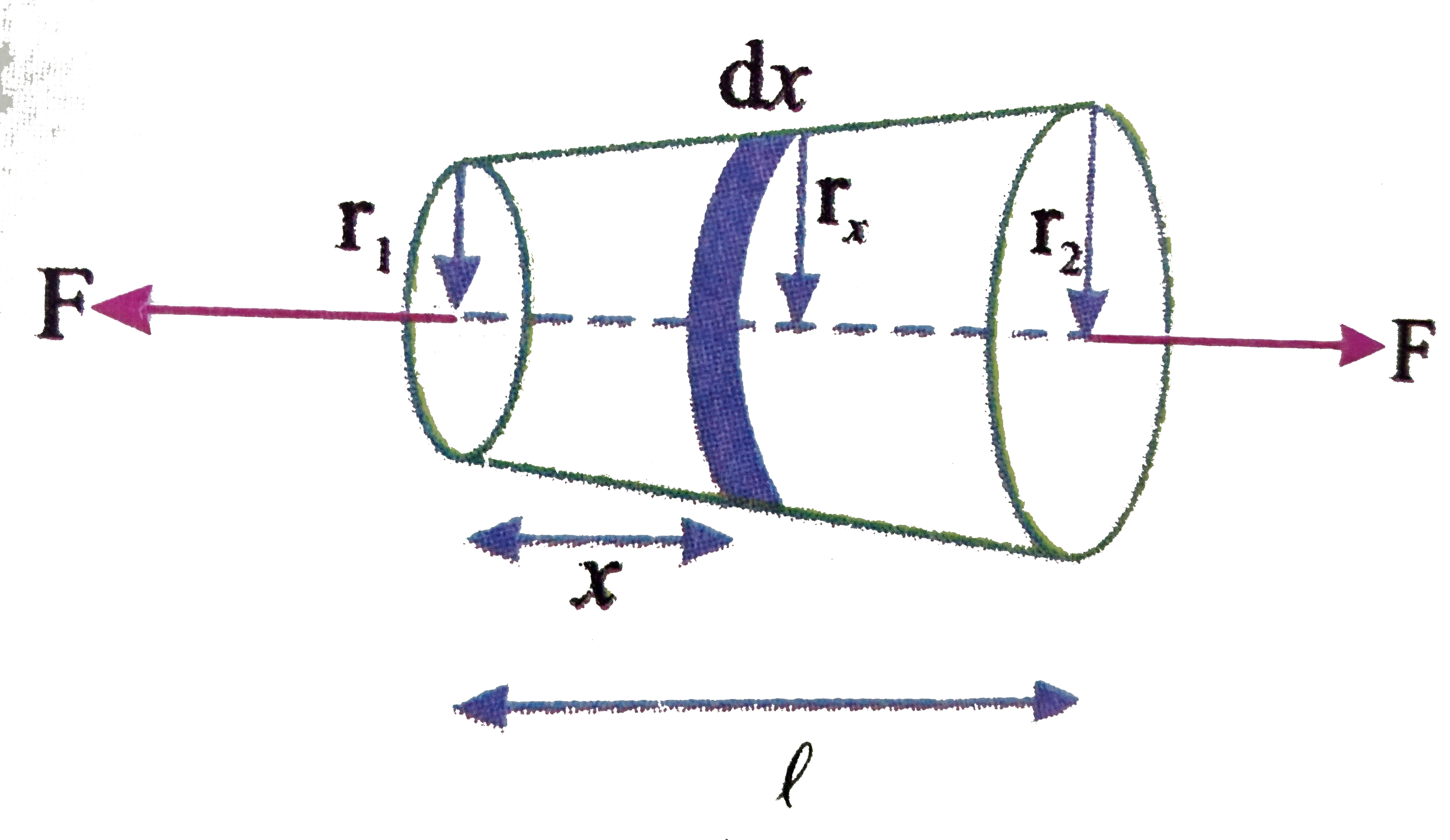
View SolutionA slightly conical wire of length `l` and radii, `r_(1)` and `r_(2)` is strecthced by two forces each of magnitude `F` applied parallel to length in opposide directions and normal to end faces. If `Y` denotes the Young's modulus then find the elogation of the wire `(r_(2) gt r_(1))`.
View SolutionWhen the tension on a wire is `4N` its length is `l_(1)`. When the tension on the wire is `4N` length is `l_(1)`. When the tension on the wire is `5N` its length is `l_(2)`. Find its natural length.
View SolutionA wire whose cross-sectional area is `4mm^(2)` is streched by `0.1mm` by a certain load. If a similar wire of double the area of cross-section is under the same load, then the elongation would be
View SolutionTwo wires `A` and `B` have Young moduli in the ratio `1:2` and ratio of lenghts is `1:1`. Under the application of same stress the ratio of elonagations is
View SolutionA wire is strected by `0.1mm` by a certain force `"F'` another wire of same material whose diameter and lengths are double to original wire is streched by the same force then its elongation will be
View SolutionA brass wire of length `300cm` when subjected to a force `F` produces an elongation `"a"`. Another wire of twice the diameter and of same length and material, when subjeceted to the force `F` produces and elongation `b`. Then the value of `a//b` is
View SolutionTwo bars `A` and `B` of circular cross section, same volume and made of the same material, are subjected to tension. If the diameter of `A` is half that of `B` and if the force applied to both the rod is the same and it is in the elastic limit, the ratio of extension of `A` to that of `B` will be
View SolutionTwo wires of the same material have masses in the ratio of their extenisons under the same load if their lenghts are in the ratio `9:10` is
View SolutionTwo rods of different materials are clamped at their ends rigidy. When they are heated for the same rise in temperature, same thermal stresses are prouded in them. If their Young's modulll are in the ratio `x:y` then ratio fo coefficients of their linear expanison is
View SolutionA tungsten wire, `0.5mm` in diameter, is just strechted between two fixed points at a temperture of `40^(@)C`. Determine the tension in the wire when the temperature falls to `20^(@)C`. (coefficant of linear expansion of tungsten `= 4.5xx10^(-6)//.^(0)C,` Young's modulus of tungsten `= 3.4xx10^(10) Nm^(-2))`
View SolutionA unifrom steel rod of length `1m` and area of cross-section `20 cm^(2)` is hanging from a fixed support. Find the increases in the length of the rod.
View SolutionA rope 1 cm in diameter breaks if the tension in it exceeds 500 N. The maximum tension that any be given to a similar rope of diameter 2 cm is
View SolutionA cabe breaks if strethced by more than `2mm`. It is cut into two equal parts. By how much eitther part can be streched without breaking
View SolutionA metal cube of side `10 cm` is subjected to a shearing stress of `10^(6)N//m^(2)`. Calculate the modulus of rigidity if the edge of the cube is displaced by `0.05 cm` with respect to its bottom.
View SolutionThe upper end of a wire of radius `4mm` and lengh `100cm` is clamped and its other end if twiced through an angle of `60^(@)` the angle of shear si
View SolutionA thin cylindrical rod of length `2.5m` and radius `5mm` is firmly fixed at upper end when lower end is twiced, the shear angle is found to be `0.06^(@)` then angle of twising is
View SolutionA spherical ball of volume `1000 cm^(3)` is subjected to a pressure of `10` atomosphere. The change in volume is `10^(-2)cm^(-3)`. IF the ball is made of iron find its bulk modulus.
(Atmospheric pressure `= 1xx10^(5) Nm^(-2)`)
View SolutionOn taking a solid rubber ball from the surface to the bottom of a lake `100m` deep, the reduction in volume is found to eb `0.5%` if the density of water si `10^(3) kgm^(-3)` and `g = 10 ms^(-1)`, find the bulk modulus of rubber.
View SolutionEstimate the change in the density of water in ocean at a depth fo `500m` below the surface. The density of water at the surface `= 1030 kgm^(-3)` and the bulk modulus of water `= 2.2xx10^(9) Nm^(-2)`
View SolutionA material has Poisson's ratio `0.3`, If a uniform rod of it suffers a longtiudinal strain of `25xx10^(-3)` then the percentage increases in its volume is
View SolutionA metal rod fo Young's modulus `2xx10^(10) Nm^(2)` undergoes an elastic strain of `0.02%` the energy per unit volume stored in the rod in `"joule"//m^(3)` is
View SolutionA wire suspended vertically from one of itsends is strached by attached a weight of `200 N` to the lower end . The weight streches the wire by `1 mm` . Then the elastic energy stored in the wire is
View SolutionTwo wire of same radius and lengh the are subjected to the same load. One wire is of steel and the other is of copper. If the Young's modulus of steel is twice that of copper, the ratio the energy stored per unit volume in steel to that of copper wire is
View SolutionThe potential energy of a spring when stretched by `2cm is U` if the spring is stretched by `8cm` the potential energy in it is
View SolutionIf bulk modulus of the metal is `2xx10^(12)Pa` and Poisson's ratio is `0.4` then young's modulus of the metal is
View Solution`Y,K` and `eta` respectively the young's Bulk and rigidly modulus of a body, if `eta = (K)/(2)` then correct relation is
View SolutionFind poisson's ratio of a metal if young's modulus is `2.8` times rigidly modulus.
View SolutionFor a metal `Y = 1.1xx10^(10) N//m^(2)` and Bulk modulus is `K = 11xx10^(10) N//m^(2)` then Poisson's ratio is (nearly)
View SolutionIf the Possion's ratio of a solid is `1//4`. Then the ratio of its Rigidity Modulus to the Young's modulus is
View Solution
LEVEL-I (H.W) questions and complete solutions for chapter MECHANICAL PROPERTIES OF SOLIDS of NARAYANA PHYSICS (HINGLISH) of Class 11