Complete Solutions to level 2 of chapter ATOMIC STUCTURE of Class 11 book with complete answers and questions
| Class 11 | CHEMISTRY |
| Chapter | ATOMIC STUCTURE |
| NARENDRA AWASTHI | level 2 |
| Number Of Questions | 30 |
| Academic Year | 2023-24 |
Potential energy of electron present in `He^(+)` is :
View SolutionA single electron in an ion has ionization energy equal to `217.6eV`. What is the total number of neutrons present in one ion of it?
View SolutionFor a hypothetical hydrogen like atom, the potential energy of the system is given by `U(r)=(-Ke^(2))/(r^(3))`, where r is the distance between the two particles. If Bohr's model of quantization of angular momentum is applicable then velocity of particle is given by:
View SolutionA small piece of mass m moves in such a way the P.E. =`-(1)/(2)mkr^(2)`. Where k is a constant and r is the distance of the particle from origin. Assuming Bohr's model of quantization of angular momentum and circular orbit, r is directly proportional to :
(a)`n^(2)`
(b)n
(c)`sqrt(n)`
(d)none of these
View SolutionA beam of specific kind of particles of velocity `2.1 xx 10^7 m//s` is scattered by a gold `(Z = 79)` nuclei. Find out specific charge (charge/mass) of this particle if the distance of closest approach is `2.5 xx 10^-14 m`.
View SolutionWhat is the angular velocity `(omega)` of an electron occupying second orbit of `Li^(2+)` ion?
(a)`(8pi^(3)me^(4))/(h^(3))K^(2)`
(b)`(8pi^(3)me^(4))/(9h^(3))K^(2)`
(c)`(64)/(9)xx(pi^(3)me^(4))/(h^(3))K^(2)`
(d)`(9pi^(3)me^(4))/(h^(3))K^(2)`
View SolutionThe ratio of the radius difference between `4^(th)` and `3^(rd)` orbit of H-atom and that of `Li^(2+)` ion is :
View SolutionThe velocity of an e in excited state of H-atom is `1.093 xx 10^(6)m//s`, what is the circumference of this orbit?
View SolutionThe angular momentum of an electron in a Bohr's orbit of `He^(+)` is `3.1652xx10^(-34)` kg-`m^(2)`/sec. What is the wave number in terms of Rydberg constant (R ) of the spectral line emitted when an electron falls from this level to the first excited state.l [ Use h`=6.626xx10^(-34)` Js]
View SolutionIf radiation corresponding to second line of "Balmer series" of `Li^(+2)` ion, knocked out electron from first excited state of H-atom, then kinetic energy of ejected electron would be:
View SolutionWhen an electron makes a transition from `(n+1)` state of nth state, the frequency of emitted radiations is related to n according to `(n gtgt 1)`:
View SolutionIn a collection of H-atoms, all the electrons jump from n=5 to ground level finally ( directly of indirectly) ,without emitting any line in Balmer series. The number of possible different radiations is :
View SolutionAn electron is allowed to move freely in a closed cubic box of length of side 10 cm. The uncertainty in its velocity will be :
View SolutionAn element undergoes a reaction as shown `sx+2e^(-)tox^(-2)`
Energy released `=30.87` ev/atom. If the energy released is used to dissociated `4g` to `H_(2)` molecules equally into `H^(+)` and `H^(+)` is excited state of `H` atoms where the electron travels in orbit whose circumference equal to four times its de -roglie's wavelength. Determine the minimum number of moles of `x` that would be required.
Given IE of `H=13.6` ev/atom, bond energy of `H_(2)=4.526`v/molecule
(a)1
(b)2
(c)3
(d)4
View SolutionGround state energy of H-atom is `(-E_(1))`,t he velocity of photoelectrons emitted when photon of energy `E_(2)` strikes stationary `Li^(2+)` ion in ground state will be:
View SolutionAt which temperature will the translational kinetic energy of H-atom equal to that for H-atom of first line Lyman transition? (Given `N_(A)=6xx10^(23)`)
View SolutionFor a 3s - orbital, value of `Phi` is given by following realation:
`Psi(3s)=(1)/(9sqrt(3))((1)/(a_(0)))^(3//2)(6-6sigma+sigma^(2))e^(-sigma//2)," where " sigma=(2r.Z)/(3a_(0))`
What is the maximum radial distance of node from nucleus?
View SolutionMonochromatic radiation of specific wavelength is incident on H-atoms in ground state. H-atoms absorb energy and emit subsequently radiations of six different wavelength. Find wavelength of incident radiations:
View SolutionThe energy of a I,II and III energy levels of a certain atom are `E,(4E)/(3)` and 2E respectively. A photon of wavelength `lambda` is emitted during a transition from III to I. what will be the wavelength of emission for II to I?
View SolutionCalculate the minimum and maximum number of electrons which may have magnetic quantum number `m=+1` and spin quantum number `s=+(1)/(2)` in chromium (Cr)
(a)0,1
(b)1,2
(c)4,6
(d)2,3
View SolutionAn electron in a hydrogen atom in its ground state absorbs 1.5 times as much energy as the minimum required for it to escape from the atom. What is the velocity of the emitted electron?
View SolutionIn a measurement of quantum efficiency of photosynthesis in green plants, it was found that 10 quanta of red light of wavelength 6850 Å were needed to release one molecule of `O_(2)`. The average energy storage in this process for 1 mol `O_(2)` evolved is 112 Kcal.
What is the energy conversion efficieny in this experiment?
Given: 1 cal =4.18 J, `N_(A)=6xx10^(23),h=6.63xx10^(-34)` J.s
View SolutionA hydrogen like species (atomic number Z) is present in a higher excited state of quantum number n. This excited atom can make a transitionn to the first excited state by successive emission of two photons of energies 10.20 eV and 17.0 eV respectively. Altetnatively, the atom from the same excited state can make a transition to the second excited state by successive of two photons of energy 4.25 eV and 5.95 eVv respectively. Determine the value of Z.
View SolutionH-atom is exposed to electromagnetic radiation of `lambda=1025.6` Å and excited atom gives out induced radiation. What is the minimum wavelength of the induced radiation?
(a)`102.6 nm`
(b)`12.09` nm
(c)`121.6` nm
(d)`810.8` nm
View SolutionIf the lowest energy X-rays have `lambda=3.055xx10^(-8)` m, estimate the minimum difference in energy between two Bohr's orbits such that an electronic transition would correspond to the emission of an X-ray. Assuming that the electrons in other shells exert no influence, at what Z (minimum) would a transition form the second level to the first result in the emission of an X-ray?
View SolutionAn `alpha`-particle having kinetic energy 5 MeV falls on a Cu-foil. The shortest distance from the nucleus of Cu to which `alpha`- particle reaches is (Atomic no. of Cu=29,`K=9xx10^(9)Nm^(2)//C^(2)`)
(a)`2.35xx10^(-13)` m
(b)`1.67xx10^(-14)` m
(c)`5.98xx10^(-15)` m
(d)none of these
View SolutionIn the graph between `sqrt(v)` and Z for the Mosley's equation `sqrt(v)`=a(Z-b), the intercept OX is -1 on `sqrt(v)` axis.
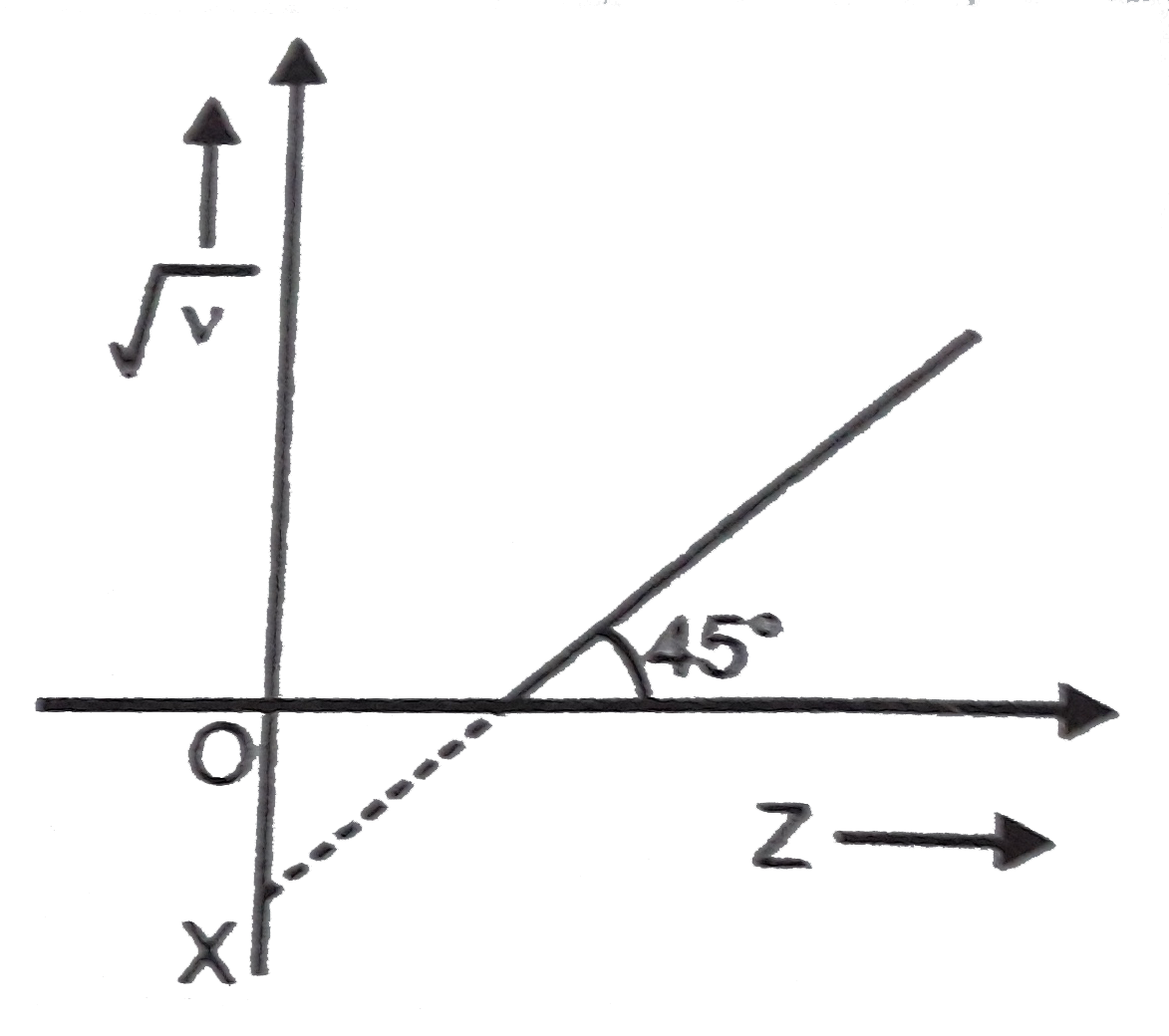
What is the frequency when atomic number (Z) is 51?
View SolutionBalmer gave an equation for wavelength of visible region of H-spectrum as `lambda=(Kn^(2))/(n^(2)-4)`.
Where n= principal quantum number of energy level, K=constant in terms of R (Rydberg constant).
The value of K in term of R is :
View SolutionThe energy of separation of an electron in a hydrogen like atom in excited state is 3.4eV. The de-Broglie wave length (in Å) associated with the electron is :
View SolutionIf I exciation energy for the H-like (hypothetical) sample is 24 eV, then binding energy in III excited state is :
(a)2eV
(b)3eV
(c)4eV
(d)5eV
View Solution
level 2 questions and complete solutions for chapter ATOMIC STUCTURE of NARENDER AVASTHI CHEMISTRY (ENGLISH) of Class 11