Complete Solutions to Simple Harmonic Motion of chapter OSCILLATIONS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | OSCILLATIONS |
| NCERT FINGERTIPS | Simple Harmonic Motion |
| Number Of Questions | 17 |
| Academic Year | 2023-24 |
Out of the following functions representing motion of a particle which represents SHM?
1. `x=sin^(3)omegat`
2. `x=1+omegat+omega^(2)t^(2)`
3. `x=cosomegat+cos3omegat+cos5omegat`
4. `x=sinomegat+cosomegat`
View SolutionWhich of the following is not characteristics of simple harmonic motion?
View SolutionThe equation of motion of a simple harmonic motion is
View SolutionWhich of the following expression does not represent simple harmonic motion?
View SolutionIf a simple harmonic motion is represented by `(d^(2)x)/(dt^(2)) + alphax = 0`, its time period is :
View SolutionThe time period of simple harmonic motion depends upon
View SolutionWhich of the following motions is not simple harmonic?
View SolutionIf x, v and a denote the displacement, the velocity and the acceleration of a particle executing simple harmonic motion of time period T, then, which of the following does not change with time?
View SolutionWhich of the following functions of time represent (a) simple harmonic motion and (b) periodic but not simple harmonic motion? Give the period for each case.
(i)` sinomegat-cosomegat` (ii) `sin^(2)omegat` (iii) `cosomegat+2sin^(2)omegat`
View SolutionA particle executing simple harmonic motion with an amplitude A. the distance travelled by the particle in one time period is
View SolutionDisplacement versus time curve for a particle executing SHM is shown in figure. Choose the correct statements.
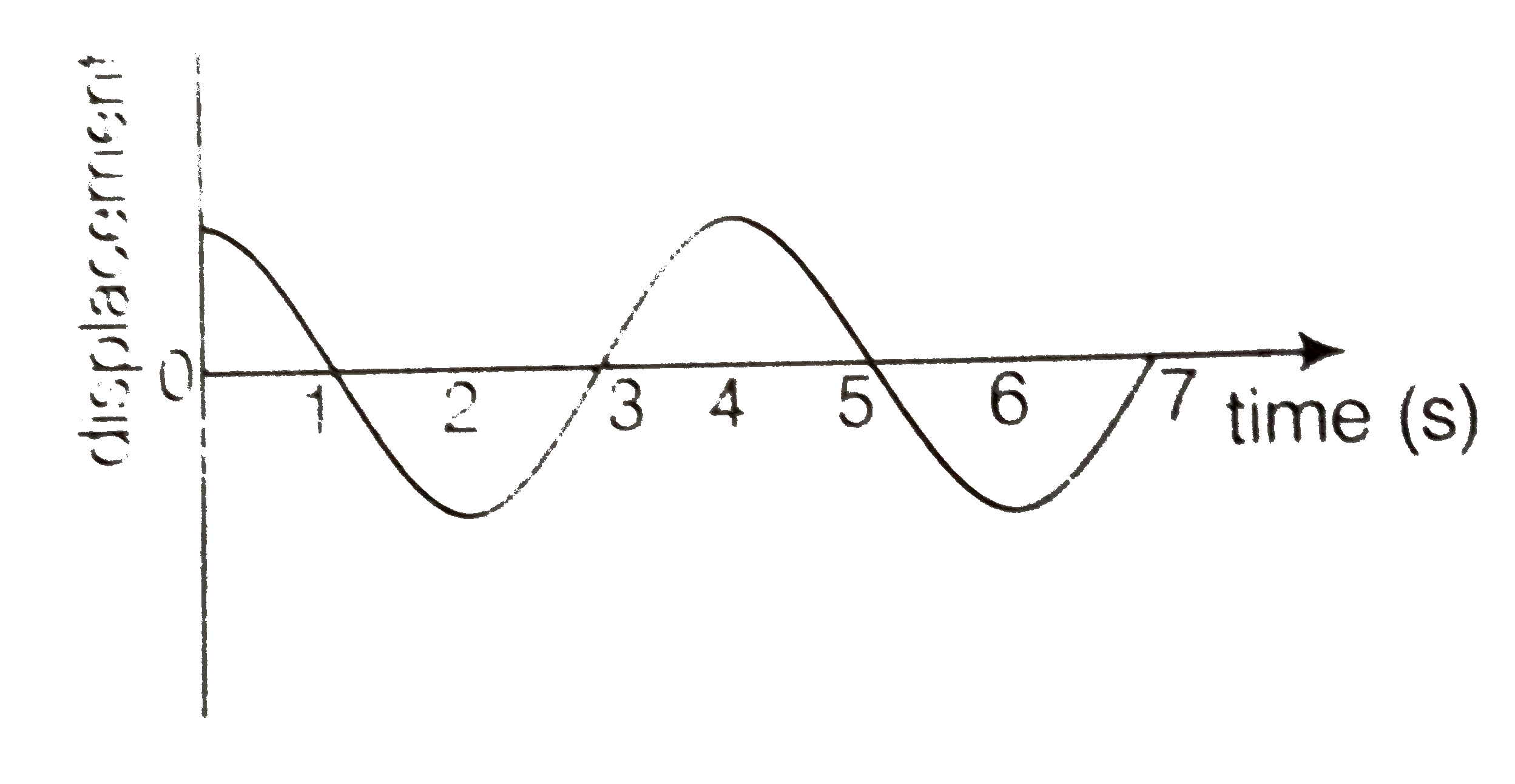
View SolutionTwo simple harmonic motions are represented by the equations.
`y_(1)=10"sin"(pi)/(4)(12t+1),y_(2)=5(sin3pt+sqrt(3)cos3pt)` the ratio of their amplitudes is
View SolutionA vibratory motion is represented by `x=2Acosomegat+Acos(omegat+(pi)/(2))+Acos(omegat+pi)+(A)/(2)cos(omegat+(3pi)/(2))`.
the resultant amplitude of the motion is
View SolutionA particle executing SHM is described by the displacement function `x(t)=Acos(omegat+phi)`, if the initial (t=0) position of the particle is 1 cm, its initial velocity is `pii" cm "s^(-1)` and its angular frequency is `pis^(-1)`, then the amplitude of its motion is
View SolutionTwo particles execute SHMs of the same amplitude and frequency along the same straight line. They cross one another when going in opposite direction. What is the phase difference between them when their displacements are half of their amplitudes ?
View SolutionTwo particles execute SHM of same amplitude and frequency on parallel lines. They pass one another when moving in opposite directions each time their displacement is one third their amplitude. What is the phase difference between them?
View SolutionA mass of 2kg is attached to the spring of spring constant `50Nm^(-1)`. The block is pulled to a distance of 5 cm from its equilibrium position at `x=0` on a horizontal frictionless surface from rest at t=0. Write the expression for its displacement at anytime t.
View Solution
Simple Harmonic Motion questions and complete solutions for chapter OSCILLATIONS of NCERT FINGERTIPS PHYSICS (HINGLISH) of Class 11
All Exercises from NCERT FINGERTIPS Class 11 PHYSICS OSCILLATIONS
- Assertion And Reason
- Damped Simple Harmonic Motion
- Energy In Simple Harmonic Motion
- Exemplar Problems
- Force Law For Simple Harmonic Motion
- Forced Oscillation And Resonance
- Higher Order Thinking Skills
- MCQs
- NCERT Exemplar
- Periodic And Oscillatory Motions
- Simple Harmonic Motion
- Simple Harmonic Motion And Uniform Circular Motion
- Some Systems Executing Simple Harmonic Motion
- Velocity And Acceleration In Simple Harmonic Motion