Complete Solutions to Exercise of chapter Laws of Motion of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | Laws of Motion |
| SL ARORA | Exercise |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
A force acts for 10 s on a body of mass 10 kg after which the force ceases and the body describes 50 m in the next 5 s. Find the magnitude of the force.
View SolutionA truck starts from rest and rolls down a hill with a constant acceleration. It travels a distance of `400m` in `20 s`. Find its acceleration. Find the force acting on it if its mass is `7` metric tonnes (Hint.1 metric tonne=1000kg)
View SolutionA force of `5N` gives a mass `m_(1)`, an acceleration of `8 m//s^(2)`, and a mass `m_(2)`, an acceleration of `24 m//s^(2)`. What acceleration would it give if both the masses are tied together?
View SolutionIn an `X-ray` machine an electron is subjected to a force of `10^(-23) N` In how much time will the electron a distance of `0.1m` ? Take mass of electron `=10^(-30)kg` .
View SolutionA stone of mass `5kg` falls from the top of a cliff `50m` high and buries `1m` deep in sand . Find the average resistance offered by the sand and the time it takes to penetrate .
View SolutionA bullet of mass `100 gram` moving with `20 m//s` Strikes a wooden plank and penetrates upto 20 cm. Calculate the resistance offered by the wooden plank .
View SolutionA motor car running at the rate of `7ms^(1)` can be stooped by applying brakes in `10m` Show that total resistance to the motion when brakes are on is one fourth of the weight of the car .
View SolutionA force of `50N` is inclined to the vertical at an angle of `30^(@)` Find the acceleration it produes in a body of mass `2kg` which moves in the horizontal direction .
View SolutionA ship of mass `3xx10^7kg` initially at rest, is pulled by a force of `5xx10^5N` through a distance of 3m. Assuming that the resistance due to water is negligible, the speed of the ship is
View SolutionA ballon has a mass of 5 g in air. The air escapes from the ballon at a uniform rate with a velocity of `5 cms^(-1)` . If the ballon shrinks completely in 2.5 s, find the average foce acting on the ballon
View SolutionA cricket ball of mass 150 kg is moving with a velocity of `12 m//s` and is hit by a bat so that ball is turned back with a velocity of `20 m//s` . The force of the blow acts for 0.01 s on the ball . Find the average force exerted by the bat on the ball.
View SolutionA hammer weighing 1 kg moving with the speed of `10ms^(-1)` strikes the head of a nail driving it 10 cm into a wall. Neglecting the mass of the nail, calculate (i) the acceleration during impact (ii) the time interval of the impact and (iii) the impulse.
View SolutionA hammer weighing 1 kg moving with the speed of `10ms^(-1)` strikes the head of a nail driving it 10 cm into a wall. Neglecting the mass of the nail, calculate (i) the acceleration during impact (ii) the time interval of the impact and (iii) the impulse.
View SolutionA hammer weighing 1 kg moving with the speed of `10ms^(-1)` strikes the head of a nail driving it 10 cm into a wall. Neglecting the mass of the nail, calculate (i) the acceleration during impact (ii) the time interval of the impact and (iii) the impulse.
View SolutionTwo billiard balls of mass 50 g moving in oppositedirections with speed of `16 ms^(-1)` collide and rebound with the same speed. What is the impulse imparted by ech ball to the other?
View SolutionA machine gun has a mass of `20 kg` . It fires` 35 g` bullets at the rate of` 400` bullets per minute with a speed of `400 m//s` What force must be applied to the gun to keep it in position ?
View SolutionA ball of mass `20` gram hits a wall at an angle of `45^(@)` with a velocity of `15m//s` If ball rebounds at `90^(@)` to the direction of incidence, calculate the impulse received by the ball.
View SolutionA glass ball whose mass is 10 g falls from a height of 40 m and rebounds to a height of 10 m. Find the impulse and the average force between the glass ball and the floor if the time during which they are in contact is `0.1` s.
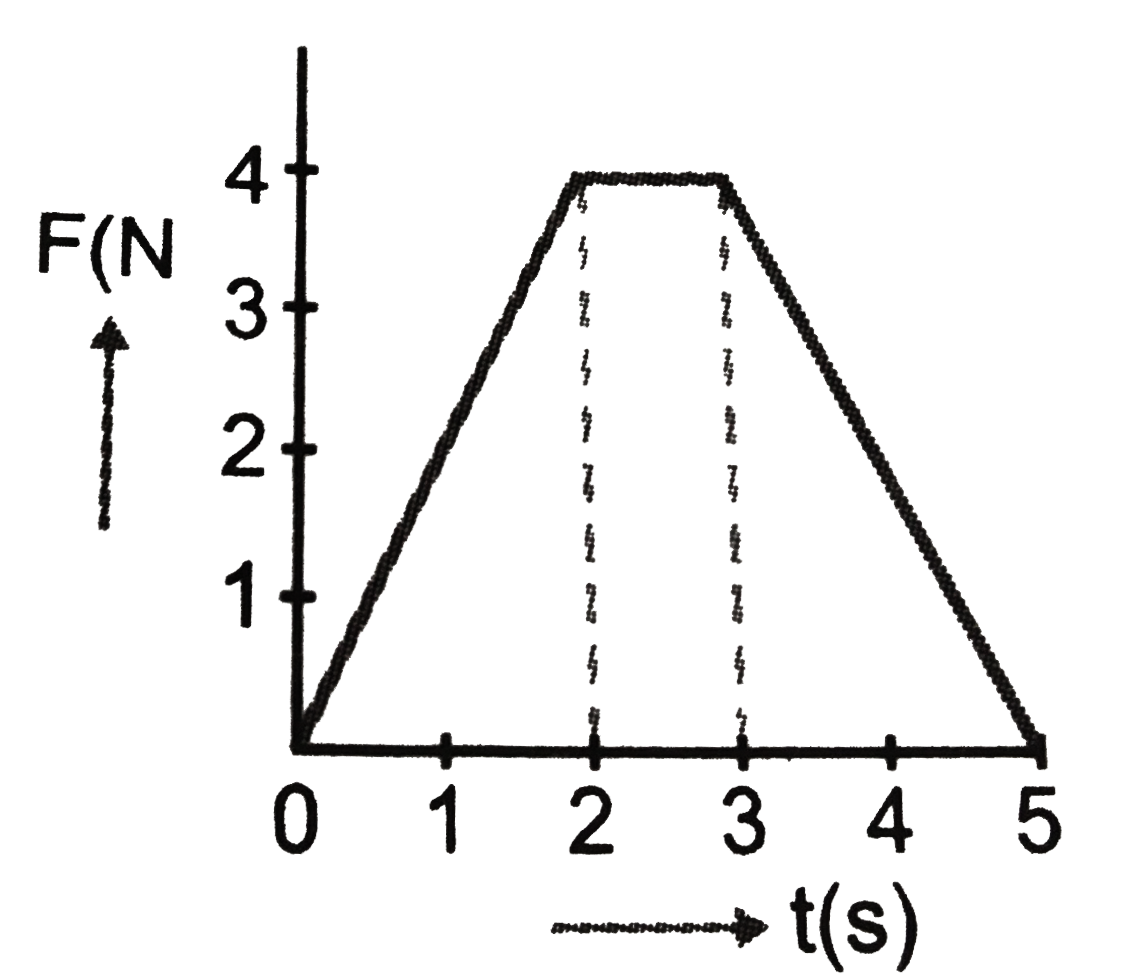
View SolutionA force acting on a body of mass 2 kg varies with time as shown in fig.5.11. Find impulse of the force.
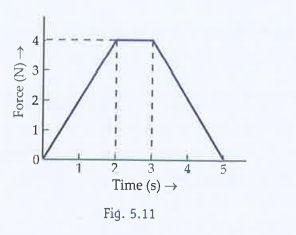
View SolutionA force acting on a body of mass 2 kg varies with time as shown in fig . 20 find impulse of the force and final velocity of the body.
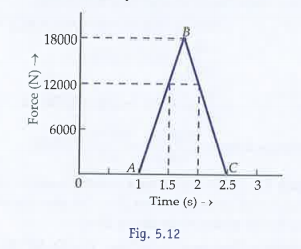
View SolutionFig.5.12 shows an estimated force-time graph for a base ball struck by a bat. From the curve, determine: impulse delivered to the ball.
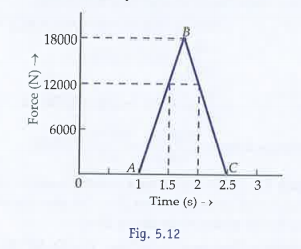
View SolutionFig.5.12 shows an estimated force-time graph for a base ball struck by a bat. From the curve, determine: Force exerted on the ball.
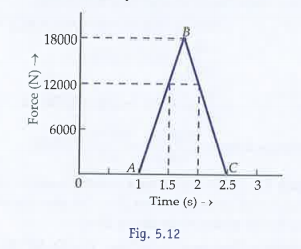
View SolutionFig.5.12 shows an estimated force-time graph for a base ball struck by a bat. From the curve, determine: The maximum force on the ball.
View SolutionA ball moving with a momen - tum of `15 kg ms^(-1) ` strickes against the wall at an angle of `30 ^(@)` and is reflected back with the same momentum at the same angle . Calculte impulse.
View SolutionCalculate the impulse necessary to stop a 1500 kg car travelling at 90 `km//h`.
View SolutionA body of mass 0.25 kg moving with velocity 12`m//s` is stopped by applying a force of 0.6 N. Calculate the time taken to stop the body. Also calculate the impulse of this force.
View SolutionAn elevator weighing 5000 kg is moving upward and tension in the supporting cable is 5000 N . Find upward acceleration . Starting from rest , how far does it rise in 10 secound ?
View SolutionA woman weighing 50 kgf stands on a weighing machine placed in lift. What will be the reading of the machine, when the lift is (i) moving upwards with a uniform velocity of `5ms^(-1)` and (ii) moving downwards with a uniform acceleration of `1ms^(-2)` ? Take g = 10 `ms^(-2)`.
View SolutionA woman weighing 50 kgf stands on a weighing machine placed in lift. What will be the reading of the machine, when the lift is (i) moving upwards with a uniform velocity of `5ms^(-1)` and (ii) moving downwards with a uniform acceleration of `1ms^(-2)` ? Take g = 10 `ms^(-2)`.
View SolutionA 75 kg man stands in a lift. What force does the floor does the floor exert on him when the elevator starts moving upwards with an acceleration of `2.0ms^(-2)`. ? Take g = 10`ms^(-2)`.
View SolutionFind the appared weight of a man weighing 49 kg on earth, when he is standing in a lift which is (i) rising with an acceleration of `1.2 m//s^(2)` (ii) going down with same acceleration (iii) falling freely under gravity (iv) going up or down with unifrom velocity . Take `g= 9.8 m//s^(2) `. \
View SolutionFind the appared weight of a man weighing 49 kg on earth, when he is standing in a lift which is (i) rising with an acceleration of `1.2 m//s^(2)` (ii) going down with same acceleration (iii) falling freely under gravity (iv) going up or down with unifrom velocity . Take `g= 9.8 m//s^(2) `. \
View SolutionFind the apparent weight of a man weighing 49 kg on earth when he is standing in a lift which is falling freely under the action of gravity.
View SolutionFind the appared weight of a man weighing 49 kg on earth, when he is standing in a lift which is (i) rising with an acceleration of `1.2 m//s^(2)` (ii) going down with same acceleration (iii) falling freely under gravity (iv) going up or down with unifrom velocity . Take `g= 9.8 m//s^(2) `. \
View SolutionA body of mass 15 kg is hung by a spring balance in a lift. What would be the reading of the balance when (i) the lift is ascending with an acceleration of `2ms^(-2)` (ii) descending with the same acceleration (iii) descending with a constant velocity of `2ms^(-1)`.? Take g = 10 `ms^(-2)`.
View SolutionA body of mass 15 kg is hung by a spring balance in a lift. What would be the reading of the balance when (i) the lift is ascending with an acceleration of `2ms^(-2)` (ii) descending with the same acceleration (iii) descending with a constant velocity of `2ms^(-1)`.? Take g = 10 `ms^(-2)`.
View SolutionA body of mass 15 kg is hung by a spring balance in a lift. What would be the reading of the balance when (i) the lift is ascending with an acceleration of `2ms^(-2)` (ii) descending with the same acceleration (iii) descending with a constant velocity of `2ms^(-1)`.? Take g = 10 `ms^(-2)`.
View SolutionA mass of 10 kg is suspended from a string, the other end of which is held in hand Find the tension in string when the hand is moved up with a uniform acceleration of `2ms^(-1)`. Given `g = 10 ms^(-1)`.
View SolutionThe strings of a parachute can bear a maximum tension of 72 kg wt. By what minimum acceleration can a person of 96 kg descend by means of this parachute?
View SolutionA 70 kg man in sea is being lifted by a helicopter with the help of a rope , which can bear a maximum tension of 100 kg wt . With what maximum acceleration the helicopter should rise so that the rope does not break ? Take `g = 9.8 m//s^(2)` .
View SolutionAn elevator and its load weigh a total of `800kg` Find the tension `T` in the supporting cable when the elevator originally moving downwards at `20m//s` is brought to rest with constant retardation in a distnce of `50m`.
View SolutionA 30 g bullet leaves a rifle with a velocity of 300 `ms^(-1)` and the rifle recoils with a velocity of `0.60ms^(-1)`. Find the mass of the rifle.
View SolutionA `40kg` shell is flying at a speed of `72km//h` It explodes into two pieces One piece of mass `15kg` just stops . What is the speed of the other ?
View SolutionA gun weighing 4 kg fires a bullet of 80 g with a velocity of `120 ms^(-1)` . With what velocity does the gin recoil? What is the combined momentum of the gun and bullet before firing and after firing?
View SolutionA car of mass 1000 kg moving with a speed of `30 m//s` collides with the back of a stationery lorry of mass 9000 kg. Calculate the speed of the vehichles immediately after the collision if they remain jammed together.
View SolutionA bullet of mass `7kg` is fired into a block of metal weighing `7kg` The block is free to move Caluculte initial velocity of the bullet if the velocity of the block with the bullet in is `0.7m//s` .
View SolutionA truck of mass `2xx10^(4)` kg travelling at `0.5ms^(-1)` collides with another truck of half its mass moving in the opposite direction with a velocity of `0.4ms^(-1)`. If the trucks couple automatically on collision, calculate the common velocity with which they move.
View SolutionA neutron of mass `1.67xx10^(27)` kg moving with a speed of `3xx10^(6)ms^(-1)` collides with a deutron of mass `3.34xx10^(-27)` kg which is at rest. After collision, the neutron sticks to the deutron and forms a triton. What is the speed of the triton?
View SolutionA bomb at rest explodes into three fragments of equal massses Two fragments fly off at right angles to each other with velocities of `9m//s` and `12//s` Calculate the speed of the third fragment .
View SolutionA man weighing 60 kg runs along the rails with a velocity of `18 kmh^(-1)` and jumps into a car of mass 1 quintal standing on the rails. Calculate the velocity which the car will start travelling along the rails.
View SolutionA machine gun of mass 10 kg fires 20 g bullets at the rate of 10 bullets per second with a speed of 500 `ms^(-1)`. What force is required to hold the gun in position ?
View SolutionA rocket with a lift mass of 10,000 kg is blasted upwards with an acceleration of `10ms^(-2)`. Calculate the initial thrust of the blast
View SolutionFuel is consumend at the rate of 50 g per second in a rocket. The exhaust gases are rejected at the rate of `5xx10^(5)cms^(-1)`. What is the thrust experienced by the rocket?
View SolutionCalculate the ratio `m_(0)//m` for a rocket to attain the escape velocity of `11.2km s^(-1)` after starting from rest, when maximum exhaust velocity of gases is `1.6km//s` .
View SolutionIn the first second of its flight a rocket ejects `(1)/(60)` of its mass with a reative velocity `2073ms^(-1)` What is the initial acceleration of the rocket ? .
View SolutionA rocket motor consumes 100 kg of fuel per second exhausting it with a speed of `6 xx 10^(3) ms^(-1)` What thrust is exerted on the rocket ? What will be the velocity of the rocket at the instant its mass is reduced to `(1 // 40)` of its initial mass ? Take initial velocity of rocket as zero . Neglect gravity .
View SolutionA rocket motor consumes 100 kg of fuel per second exhausting it with a speed of `6 xx 10^(3) ms^(-1)` What thrust is exerted on the rocket ? What will be the velocity of the rocket at the instant its mass is reduced to `(1 // 40)` of its initial mass ? Take initial velocity of rocket as zero . Neglect gravity .
View SolutionA rocket fired from the earth's surface ejects `1%` of its mass at a speed of 2000 `ms^(-1)` in the first second. Find the average acceleration of the rocket in the first second.
View SolutionA rocket is going upward with acceleration motion. A man string in it feels his weight increased 5 time his own weight. If the mass of the rocket including that of the man is `1.0xx10^(4)kg` , how much force is being applied by rocket engine? `(Take g=10ms^(-2))` .
View SolutionA ballon of mass m is rising up with an acceleration a show that the fraction og weight of balloon that must be detached in order to double its acceleration , assuming the upthrust of air to remain the same
View SolutionA mass of 6 kg is suspended by a rope of length 2 m from the ceilling A force of 50 N in the horizontal direction is applied at the mid - point P of the rope as shown . What is the angle the rope makes with the vertical in equilibrium ? (Take = `10 ms^(-2)`) . Neglect mass of the rope .
View SolutionA mass of 20 kg is suspended by a rope of length 2 m from a ceiling. A force of `173.2` N in the horizontal direction is applied at the midpoint of the rope as shown in Fig. What is the angle the rope makes with the horizontal in equilibrium ? Take g = 10`ms^(-2)`. Neglect mass of the rope.
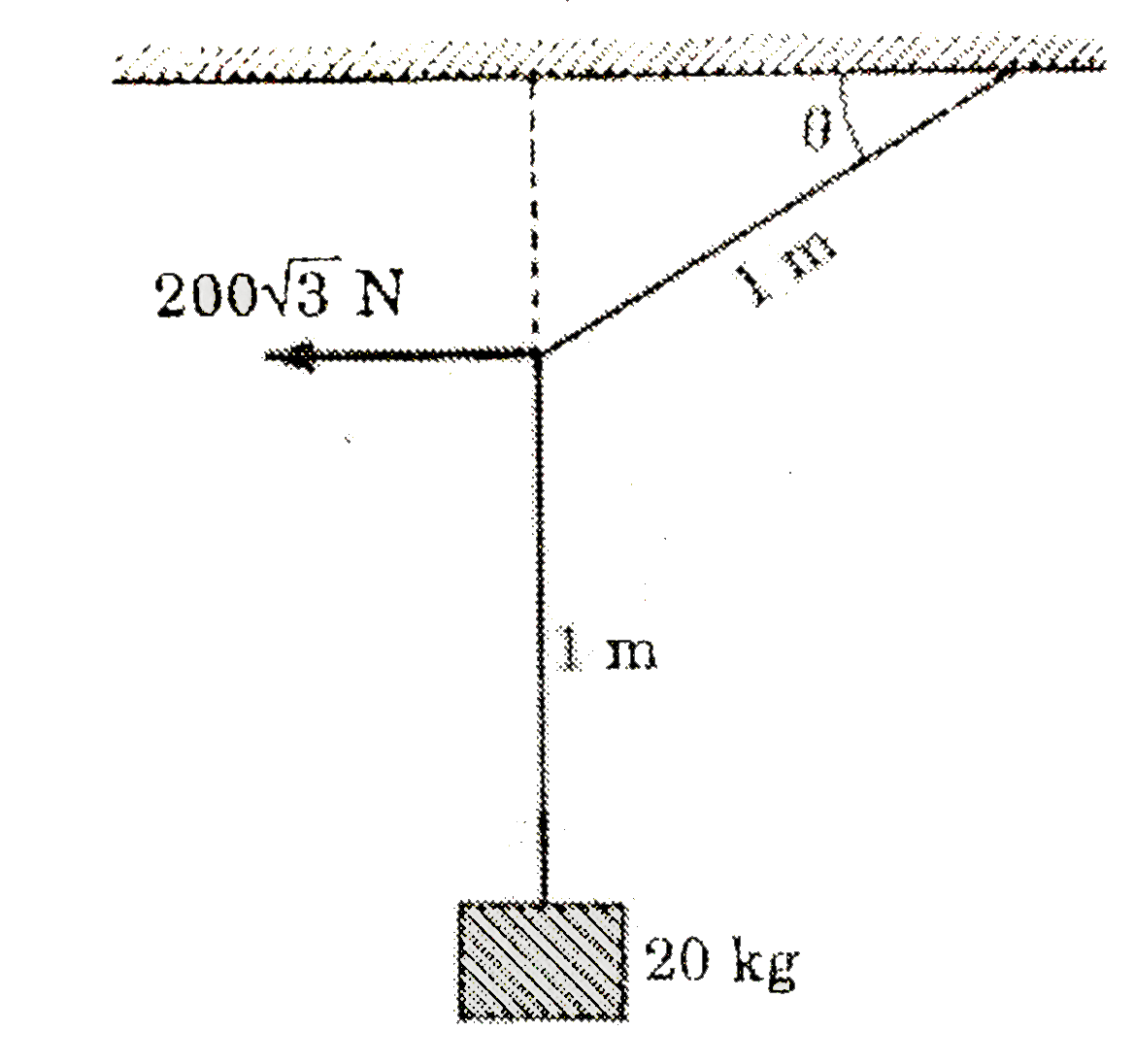
View SolutionA mass of 20 kg is suspended by a rope of length 2 m from a ceiling. A force of `173.2` N in the horizontal direction is applied at the midpoint of the rope as shown in Fig. What is the angle the rope makes with the horizontal in equilibrium ? Take g = 10`ms^(-2)`. Neglect mass of the rope.

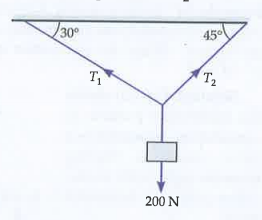
View SolutionA body of weight 200 N is supended with the help of strings as shown in Fig.5.38.Find the tensions `T_1` and `T_2`.
View SolutionTwo masses 7 kg and 12 kg are connected at the two ends of a light inetensible string that goes over a frictionless pulley. Find the acceleration of the masses and the tension in the string when the masses are released.
View SolutionTwo masses `m_(1)` and `m_(2)` are connected by a massless string as shown in Fig. Find the value of tension in the string if a force of 200 N is applied on (i) `m_(1)` and `m_(2)`.
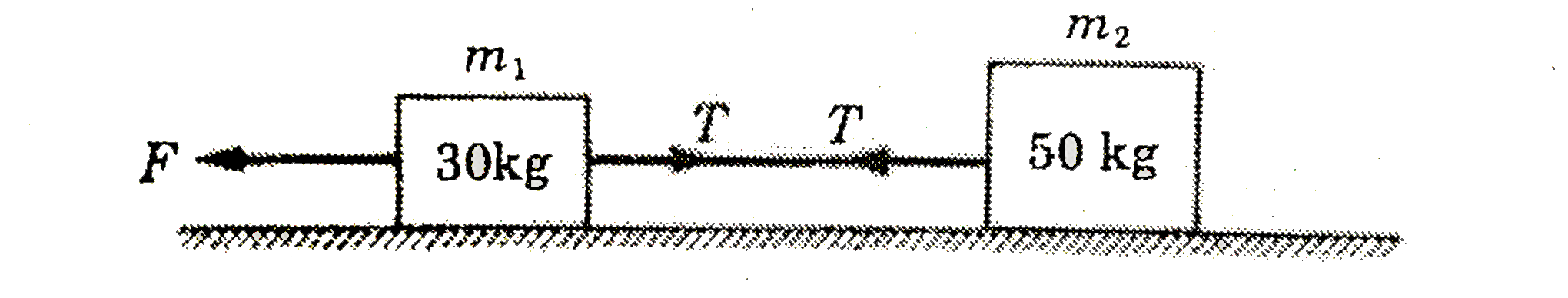
View SolutionTwo masses `m_(1)` and `m_(2)` are connected by a massless string as shown in Fig. Find the value of tension in the string if a force of 200 N is applied on (i) `m_(1)` and `m_(2)`.

View SolutionTwo bodies whose masses are `m_(1) =50kg` and `m_(2) =150kg` are tied by a light string and are placed on a frictionless horizontal surface When `m_(1)` is pulled by force `F` an acceleration of `5ms^(-2)` is produced in both the bodies Calculate the value of `F` What is the tension in the string `1` ?
View SolutionAs shown in Fig. three masses m, 3m and 5m connected together lie on a frictionless horizontal surface and pulled to the left by a force F. The tension `T_(1)` in the first string is 24 N. Find (i) acceleration of the system, (ii) tension in the second string, and (iii) force F.
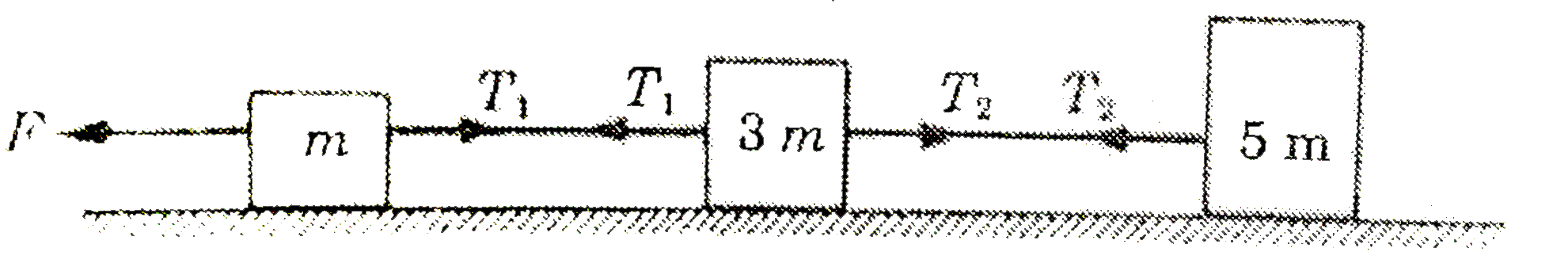
View SolutionAs shown in Fig. three masses m, 3m and 5m connected together lie on a frictionless horizontal surface and pulled to the left by a force F. The tension `T_(1)` in the first string is 24 N. Find (i) acceleration of the system, (ii) tension in the second string, and (iii) force F.
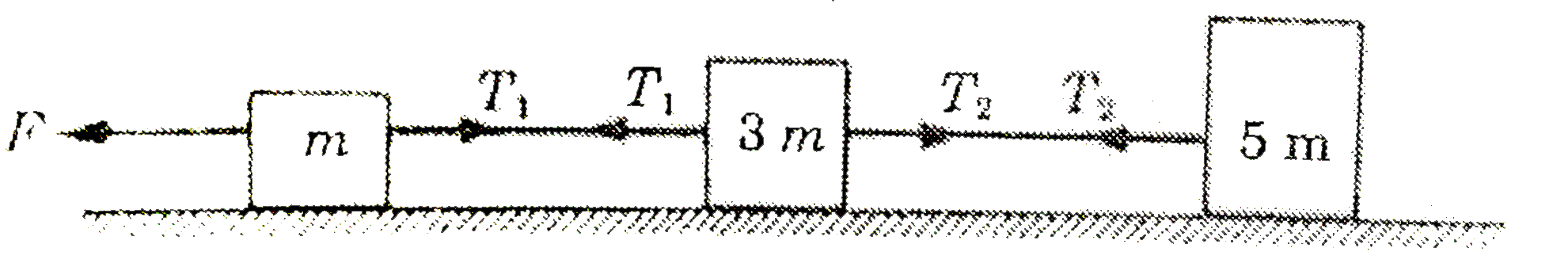
View SolutionAs shown in Fig. three masses m, 3m and 5m connected together lie on a frictionless horizontal surface and pulled to the left by a force F. The tension `T_(1)` in the first string is 24 N. Find (i) acceleration of the system, (ii) tension in the second string, and (iii) force F.
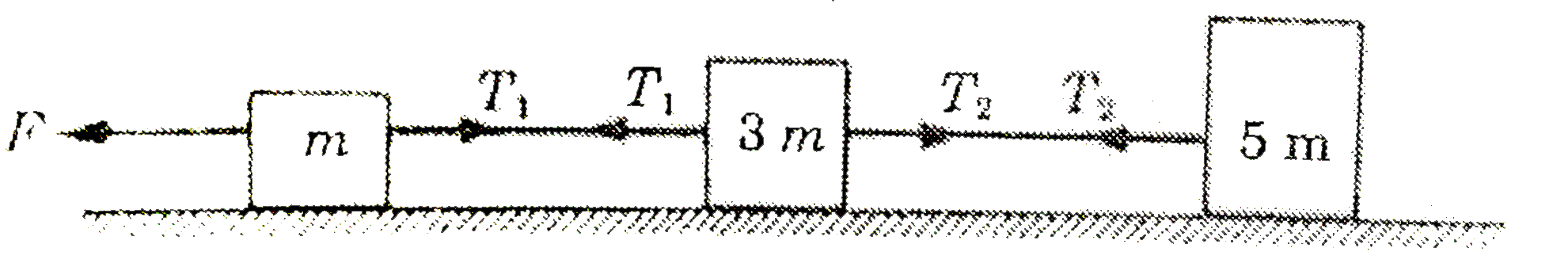
View SolutionThree identical blocks, each having a mass `M`, are pushed by a force `F` on a frictionless table as shown in (figure) What is the acceleration of the blocks? What is net force on block `A`? What force does `A` apply on `B`? What force does `B` apply on `C`? show action reaction pairs on the contact surface of the blocks.
View SolutionFour blocks of the same mass m connected by cords are pulled by a force F on a smooth horizontal surface as shown in Determine the tensions `T_1 ,T_2` and `T_3` in the cords.
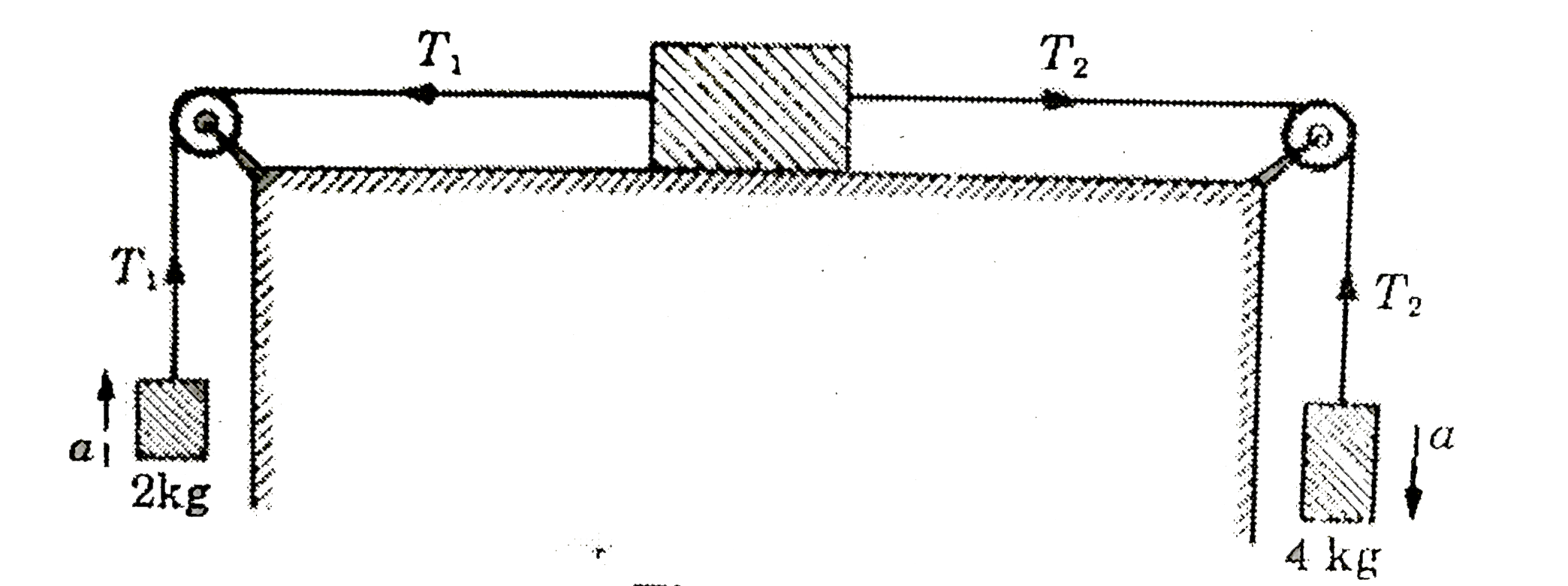
View SolutionIn Fig. find the acceleration a of the system and the tesnions `T_(1)` and `T_(2)` in the strings. Assume that the table and the pulleys are frictionless and the strings are massless. Take g = `9.8ms^(2)`
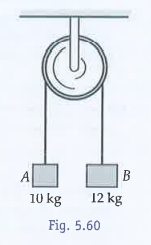
View SolutionIn the Atwood's machine [Fig. 5.60], the system starts from rest. What is the speed and distance moved by each mass at t=3s?
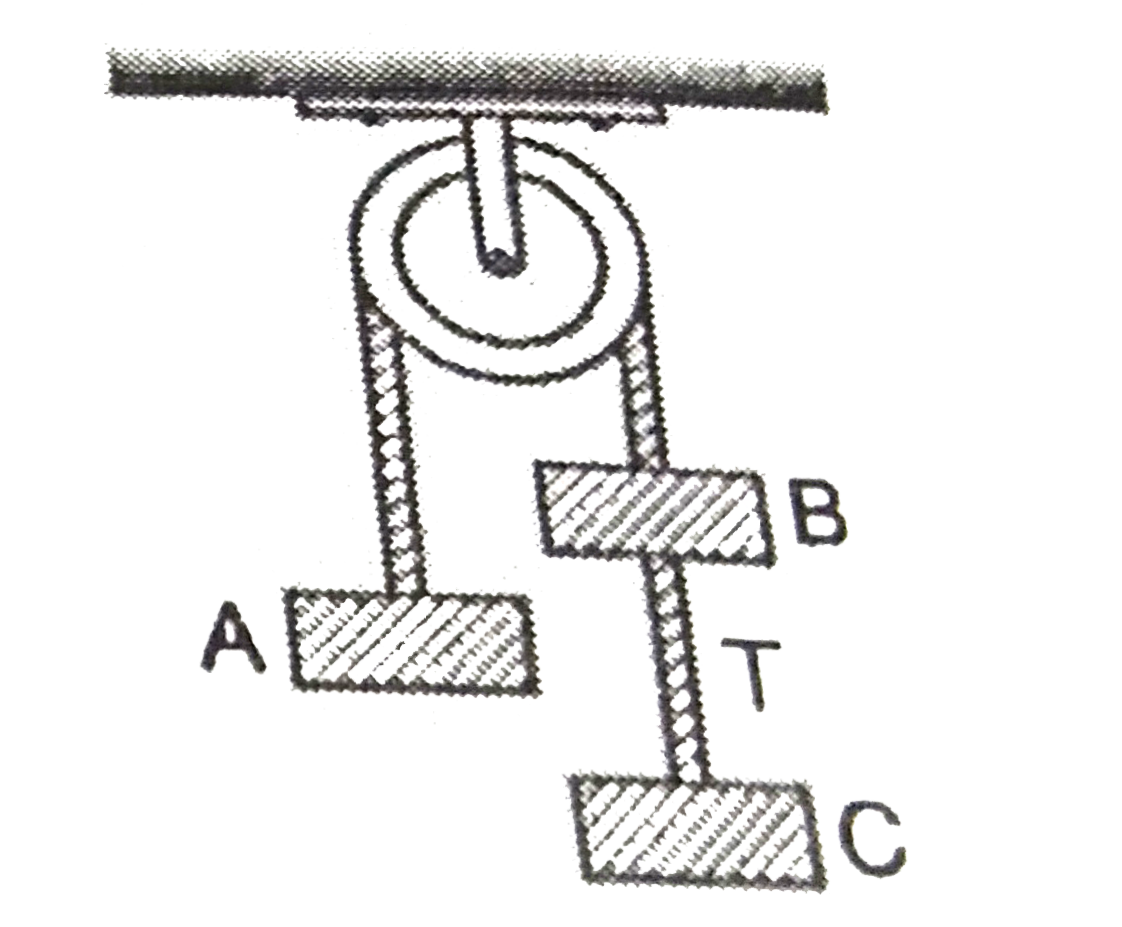
View SolutionThree equal weights of mass 2 kg each are hanging on a string passing over a fixed pulley as shown in fig What is the tension in the string connecting weights B and C?
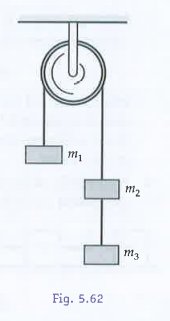
View SolutionIn the arrangement shown in Fig.5.62, show that the tension in the string between masses `m_2` and `m_3` is `T=frac(2m_1 m_3 g)(m_1 +m_2+m_3)`
View SolutionA block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is `5m//s^2`, the frictional force acting on the block is…………newtons.
View SolutionA body weighing 20 kg just slides down a rough inclined plane that rises 5 m in every 13 m. What is the coefficient of friction?
View SolutionA scooter weighs 120 kg f. Brakes are applied so that wheels stop rolling and start skidding. Find the force of friction if the coefficient of friction is `0.4`.
View SolutionAn automobile is moving on a horizontal road with a speed `upsilon` If the coefficient of friction between the tyres and the road is `mu` show that the shortest distance in which the automobile can be stooped is `upsilon^(2)//2 mu g`.
View SolutionFind the distance travelled by a body before coming rest, if it is moving with a speed of `10ms^(-1)` and the coefficient of friction between the ground and the body is `0.4`.
View SolutionA motor car running at the rate of `7ms^(1)` can be stooped by applying brakes in `10m` Show that total resistance to the motion when brakes are on is one fourth of the weight of the car .
View SolutionFind the power of an engine which can maintain speed of `50ms^(-1)` for a train of mass `3xx10^(5)`kg on a rough line. The coefficient of friction is `0.05`. Take `g=10ms^(-2)`
View SolutionA train weighing `1000` quintals is running on a level road with a unifrom speed of `72km//h` If the frictional resistance amounts to 50g wt. per quintal find power in watt, take `g = 9.8ms^(2)`.
View SolutionAn automobile of mass m starts from rest and accelerates at a maximum rate possible without slipping on a road with `mu_(s)=0.5`. If only the rear wheels are driven and half the weight of the automobile is supported on these wheels, how much time is required to reach a speed of 100 `kmh^(-1)`.
View SolutionA suitcase is gently dropped on a conveyor belt moving at `3ms^(-1)` If the coefficient of friction between the belt and suitcase is `0.5` how far will the suitcase move on the belt before coming to rest ?
View SolutionA truck moving at `72 kmh ^(-1)` carries a steel girder which rests on its wooden floor. What is the minimum time in which the truck can come to stop without the girder moving forward ? Coefficient of static friction between steel and wood is `0.5`.
View SolutionA bullet of mass 10 gram is fired horizontally into a 5 kg wooden block at rest on a horizontal surface The coefficient of kinetic friction between the block and the surface is 0.1 Calculate the speed of the bullet striking the block if the combination moves 20 m before coming rest .
View SolutionIn the masses of A and B are 10 kg and 5 kg . Calculate the minimum mass of C which may stop A from slipping Coefficient of static friction between block A and table is 0.2
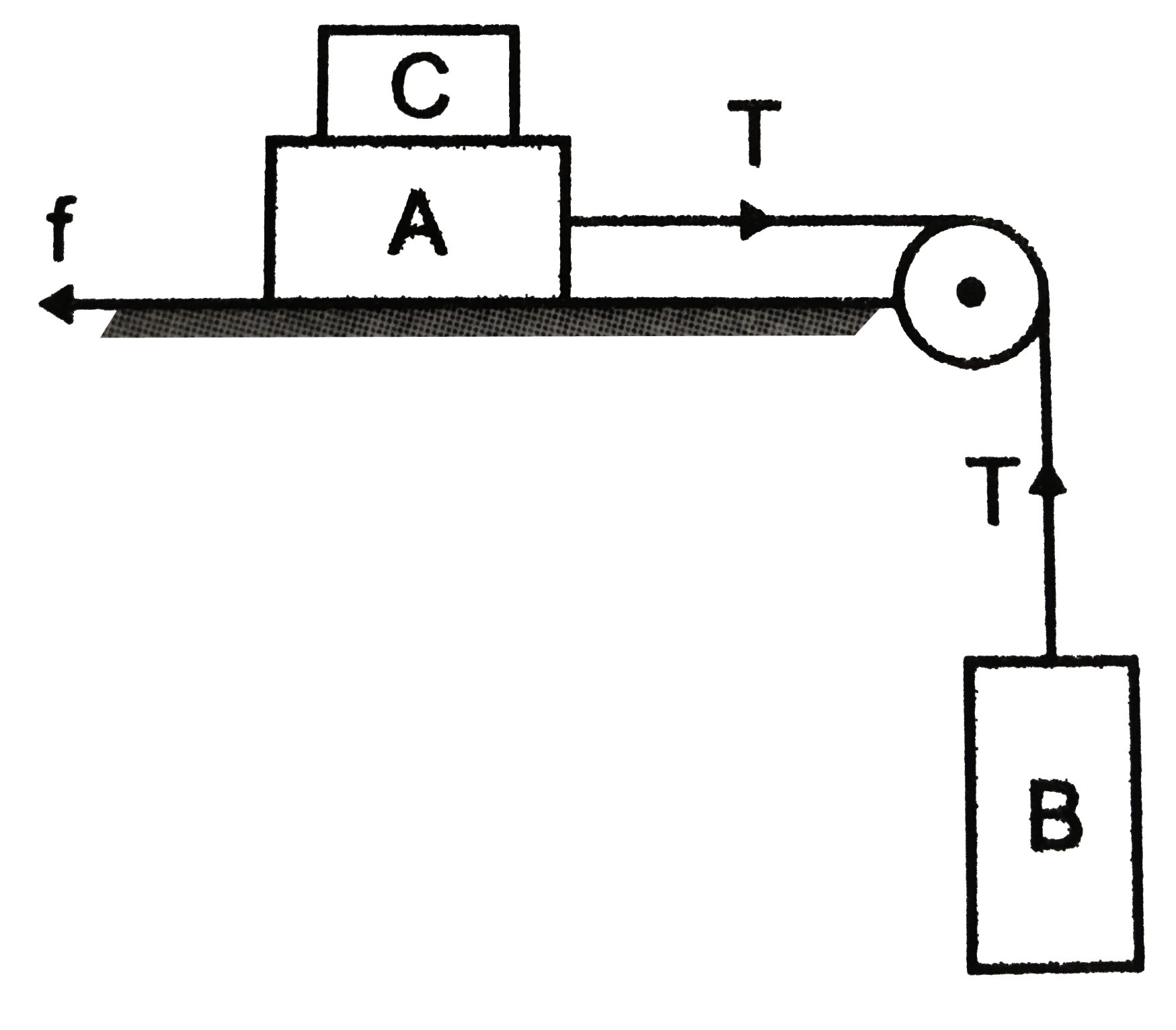 .
.
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of `30^@` with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View SolutionA block of mass 10 kg is sliding on a surface inclined at `30^(@)` with horizontal . If coefficient of friction between the block and the surface is 0.5 , find acceleration produced in the block . Take ` g = 9.8 m //^(2)` .
View SolutionFind the force required to move a train of mass `10^(5)` kg up an incline of 1 in 50 with an acceleration of `2 ms^(-2)` . Coefficient of friction between the train and rails is 0.005 . Take `g = 10^(2)` .
View SolutionA block slides down an incline of angle `30^(@)` with an acceleration of `g//4` Find the coefficient of kinetic friction .
View SolutionA 10 kg block slides without acceleration down a rough inclined plane making an angle of `20^(@)` with the horizontal. Calculate the acceleration when the inclination of the plane is increased to `30^(@)` and the work done over a distance of `1.2m`. Take `g=9.8ms^(-2)`.
View SolutionA railway engine weighing 40 metic ton is travelling along a level track at a speed of `54 km H^(-1)` What additional power is required to maintain the same speed up an incline of 1 in 49 Take `g = 9.8 m//s^(2)` and `u = 0.1` .
View SolutionA metal block of mass `0.5` kg is placed on a plane inclined to the horizontal at an angle of `30^(@)`. If the coefficient of friction is `0.2`, what force must be applied (i) to just prevent the block from sliding down the inclined plane (ii) to just move the block up the inclined plane and (iii) to move it up the inclined plane with an acceleration of `20cms^(-2)`?
View SolutionA metal block of mass `0.5` kg is placed on a plane inclined to the horizontal at an angle of `30^(@)`. If the coefficient of friction is `0.2`, what force must be applied (i) to just prevent the block from sliding down the inclined plane (ii) to just move the block up the inclined plane and (iii) to move it up the inclined plane with an acceleration of `20cms^(-2)`?
View SolutionA metal block of mass `0.5` kg is placed on a plane inclined to the horizontal at an angle of `30^(@)`. If the coefficient of friction is `0.2`, what force must be applied (i) to just prevent the block from sliding down the inclined plane (ii) to just move the block up the inclined plane and (iii) to move it up the inclined plane with an acceleration of `20cms^(-2)`?
View SolutionA block A of mass 14 kg moves alongg an inclined plance that makes an angle of `30^(@)` with the horizontal . This block is connected to another block B of mass 14 kg by a taut massless string that runs around a massless frictionless pulley . The block B moves down with constant velocity . Calculate force of friction and coefficient of kinetic friction .
View Solution
Exercise questions and complete solutions for chapter Laws of Motion of DHANPAT RAI & CO PHYSICS (HINGLISH) of Class 11