Complete Solutions to JEE Main And Advanced of chapter MOMENTUM & IMPULSE of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MOMENTUM & IMPULSE |
| SUNIL BATRA | JEE Main And Advanced |
| Number Of Questions | 30 |
| Academic Year | 2023-24 |
A particle of mass 4 m which is at rest explodes into three fragments. Two of the fragments each of mass m are found to move with a speed v each in mutually perpendicular directions. The total energy released in the process of explosion is ............
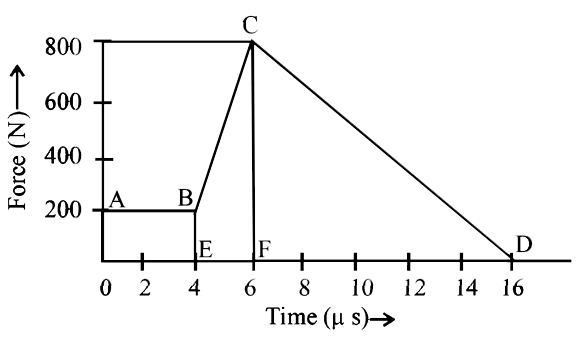
View SolutionThe magnitude of the force (in newtons) acting on a body varies with time t (in micro seconds) as shown in the fig AB, BC and CD are straight line segments. The magnitude of the total impulse of the force on the body from `t= 4 mus` to `t=16 mus` is ….Ns
View SolutionTwo particles of masses `m_1 and m_2` in projectile motion have velocities `vecv_1 and vecv_2` respectively at time `t=0.` They collide at time `t_0.` Their velocities become `vecv_1' and vecv_2'` at time `2t_0` while still moving in air. The value of `|(m_1vecv_1' + m_2vecv_2') - (m_1vecv_1 + m_2vecv_2)|` is
View SolutionTwo blocks of masses 10 kg and 4 kg are connected by a spring of negligible mass and placed on a frictionless horizontal surface. An impulse gives a velocity of `14 m//s` to the heavier block in the direction of the lighter block. The velocity of the centre of mass is
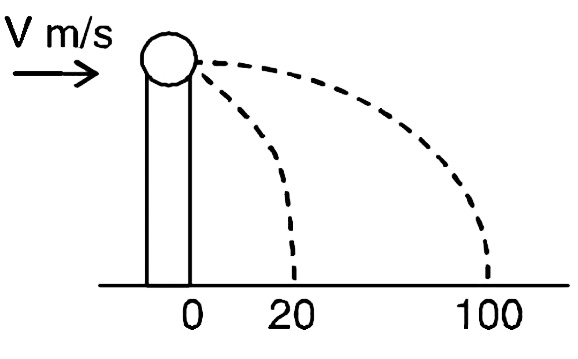
View SolutionA ball of mass 0.2 kg rests on a vertical post of height 5 m. A bullet of mass 0.01 kg, travelling with a velocity `V m//s` in a horizontal direction, hits the centre of the ball. After the collision, the ball and bullet travel independently. The ball hits the ground at a distance of 20 m and the bullet at a distance of 100 m from the foot of the post. The velocity V of the bullet is
View SolutionA particle of mass m is projected from the ground with an initial speed `u_0` at an angle `alpha` with the horizontal. At the highest point of its trajectory, it makes a completely inelastic collision with another identical particle, which was thrown vertically upward from the ground with the same initial speed `u_0.` The angle that the composite system makes with the horizontal immediately after the collision is
View SolutionA ball hits the floor and rebounds after an inelastic collision. In this case
View SolutionA shell is fired from a cannon with a velocity `v (m//sec.)` at an angle `theta` with the horizontal direction. At the highest point in its path it explodes into two pieces of equal mass. One of the pieces retraces its path to the cannon and the speed (in `m//sec.`) of the other piece immediately after the explosion is
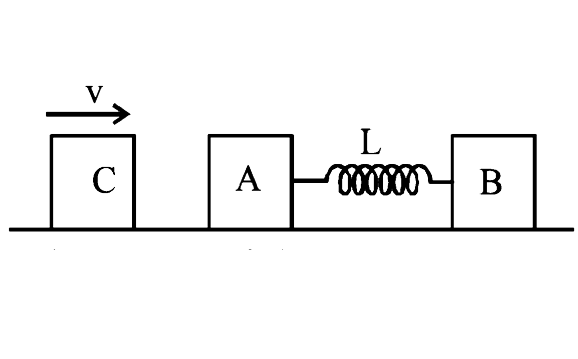
View SolutionTwo blocks A and B, each of mass m, are connected by a masslesss spring of natural length L and spring constant K. The blocks are initially resting on a smooth horizontal floor with the spring at its natural length, as shown in fig. A third identical block C, also of mass m, moves on the floor with a speed v along the line joining A and B, and collides elastically with A. Then
View SolutionThe balls, having linear momenta `vecp_1=vecpi and vecp_2_2=-vecpi`, undergo a collision in free space. There is no external force acting on the balls. Let `vecp'_1 and vec p'_2` be their final momenta.The following option (s) is (are) NOT ALLOWED for any non-zero value of `p, a_1, a_2, b_1, b_2, c_1 and c_2`.
View SolutionA point mass of 1kg collides elastically with a stationary point mass of 5 kg. After their collision, the 1kg mass reverses its direction and moves with a speed of `2ms^-1`. Which of the following statements (s) is (are) correct for the system of these two masses?
View SolutionA particle of mass m is attached to one end of a mass-less spring of force constant k, lying on a frictionless horizontal plane. The other end of the spring is fixed. The particle starts moving horizontally from its equilibrium position at time `t=0` with an initial velocity `u_0`. when the speed of the particle is `0.5u_0`, it collides elastically with a rigid wall. After this collision
View SolutionA body of mass m moving with velocity V in the X-direction collides with another body of mass M moving in Y-direction with velocity v. They coalesce into one body during collision. Calculate :
(i) the direction and magnitude of the momentum of the final body.
(ii) the fraction of initial kinetic energy transformed into heat during the collision in terms of the two masses.
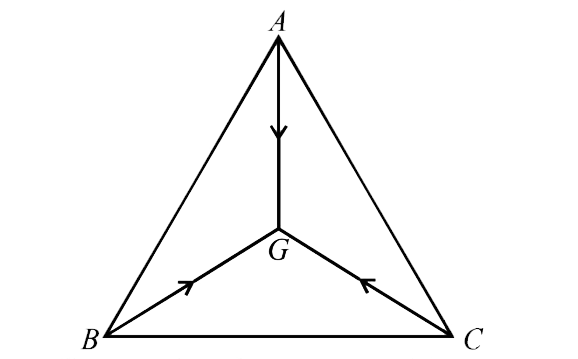
View SolutionThree particles A, B and C of equal mass move with equal speed V along the medians of an equilateral triangle as shown in hgure. They collide at the centroid G of the triangle. After the collision, A comes to test, B retraces its path with the speed V. What is the velocity of C ?
View SolutionTwo bodies A and B of masses m and 2 m respectively are placed on a smooth floor. They are connected by a spring. A third body C of mass m moves with velocity `v_0` along the line joining A and B and collides elastically with A as shown in Fig.
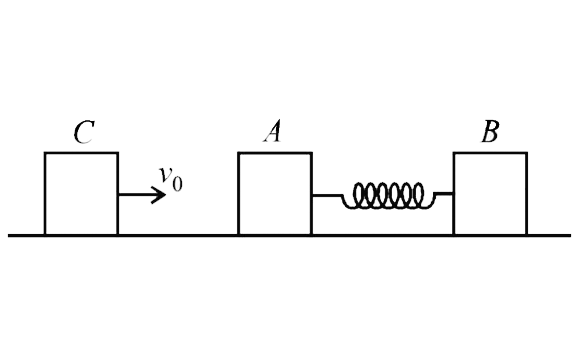
At a certain instant of time `t_0` after collision, it is found that the instantaneous velocities of A and B are the same. Further at this instant the compression of the spring is found to be `x_0`. Determine (i) the common velocity of A and B at time `t_0`, and (ii) the spring constant.
View SolutionA ball of mass 100 gm is projected vertically upwards from the ground with a velocity of `49m//sec`. At the same time another identical ball is dropped from a height of 98 m to fall freely along the same path as that followed by the first ball. After some time the two balls collide and stick together and finally fall to the ground. Find the time of flight of the masses.
View SolutionA bullet of mass M is fired with a velocity `50m//s` at an angle with the horizontal. At the highest point of its trajectory, it collides head-on with a bob of mass 3M suspended by a massless string of length `10//3` metres and gets embeded in the bob. After the collision, the string moves through an angle of `120^@`. Find
(i) the angle `theta`,
(ii) the vertical and horizontal coordinates of the initial position of the bob with respect to the point of firing of the bullet. Take `g=10 m//s^2`
View SolutionA block 'A' of mass 2m is placed on another block 'B' of mass 4m which in turn is placed on a fixed table. The two blocks have a same length 4d and they are placed as shown in fig The coefficient of friction (both static and kinetic) between the block 'B' and table is `mu`. There is no friction between the two blocks. A small object of mass m moving horizontally along a line passing through the centre of mass (cm.) of the block B and perpendicular to its face with a speed v collides elastically with the block B at a height d above the table.
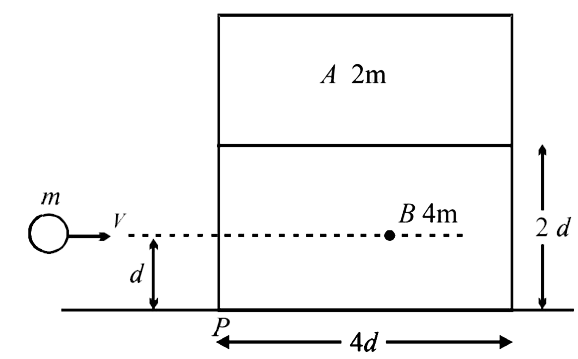
(a) What is the minimum value of v(call it `v_0`) required to make the block A topple?
(b) If `v=2v_0`,find the distance (from the point P in the figure ) at which the mass m falls on the table after collision. (Ignore the role of friction during the collision).
View SolutionA cart is moving along +x direction with a velocityof `4 m//s`. A person on the cart throws a stone with a velocity of `6 m//s` relative to himself. In the frame of reference of the cart the stone is thrown in y-z plane making an angle of `30^@` with vertical z-axis. At the highest point of its trajectory, the stone hits an object of equal mass hung vertically from the branch of a tree by means of a string of length L. A completely inelastic collision occurs, in which the stone gets embedded in the object. Determine :
(i) The speed of the combined mass immediately after the collision with respect to an observer on the ground,
(ii) The length L of the string such that the tension in the string becomes zero when the string becomes horizontal during the subsequent motion of the combined mass.
View SolutionA car P is moving with a uniform speed `5sqrt3 m//s` towards a carriage of mass 9 kg at rest kept on the rails at a point B as shown in figure. The height AC is 120 m. Cannon balls of 1 kg are fired from the car with an initial velocity `100m//s` at an angle `30^@` with the horizontal. The first cannon hall hits the stationary carriage after a time`t_0` and sticks to it. Determine `t_0`.
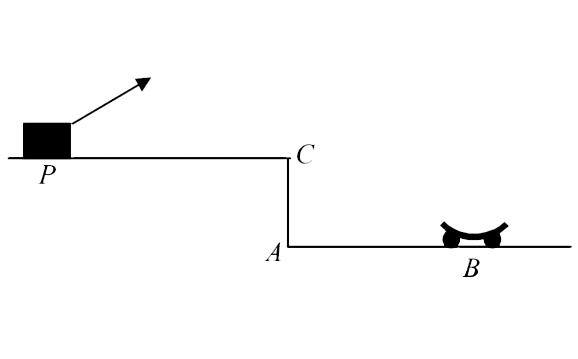
At `t_0`, the second cannon ball is fired. Assume that the resistive force between the rails and the carriage is constant and ignore the vertical motion of the carriage throughout. If the second ball also hits and sticks to the carriage, what will be the horizontal velocity of the carriage just after the second impact?
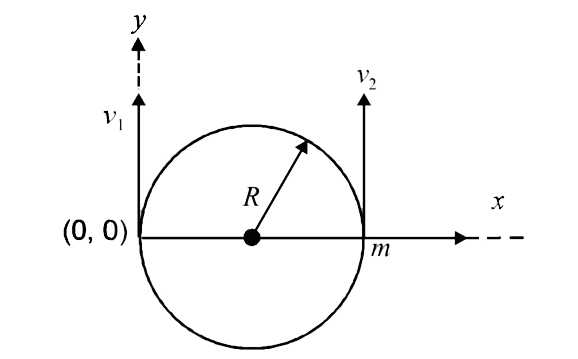
View SolutionA particle of mass m, moving in a cicular path of radius R with a constant speed `v_2` is located at point (2R,0) at time `t=0` and a man starts moving with a velocity `v_1` along the +ve y-axis from origin at time `t=0`. Calculate the linear momentum of the particle w.r.t. the man as a function of time.
View SolutionSTATEMENT-l : In an elastic collision between two bodies, the relative speed of the bodies after collision is equal to the relative speed before the collision.
STATEMENT-2 : In an elastic collision, the linear momentum of the system is conserved.
View SolutionA bob of mass m, suspended by a string of length `l_1` is given a minimum velocity required to complete a full circle in the vertical plane. At the highest point, it collides elastically with another bob of mass m suspended by a string of length `l_2`, which is initially at rest. Both the strings are mass-less and inextensible. If the second bob, after collision acquires the minimum speed required to complete a full circle in the vertical plane, the ratio `(l_1)/(l_2)` is
View SolutionA machine gun fires a bullet of mass 40 g with a velocity `1200 ms^-1`. The man holding it can exert a maximum force of 144 N on the gun. How many bullets can be fire per second at the most?
View SolutionTwo sphere `A and B` of masses `m_(1) and m_(2)` respectivelly colides. A is at rest initally and `B` is moving with velocity `v` along x-axis. After collision `B` has a velocity `(v)/(2)` in a direction perpendicular to the original direction. The mass `A` moves after collision in the direction.
View SolutionA bomb of mass 16kg at rest explodes into two pieces of masses 4 kg and 12 kg. The velolcity of the 12 kg mass is `4 ms^-1`. The kinetic energy of the other mass is
View SolutionStatement 1 : Two particles moving in the same direction do not lose all their energy in a completely inelastic collision.
Statement 2 : The principle of conservation of momentum holds true for all kinds of collisions.
View SolutionThe figure shows the position-time (x-t) graph of one-dimensional motion of a body of mass 0.4kg. The magnitude of each impulse is
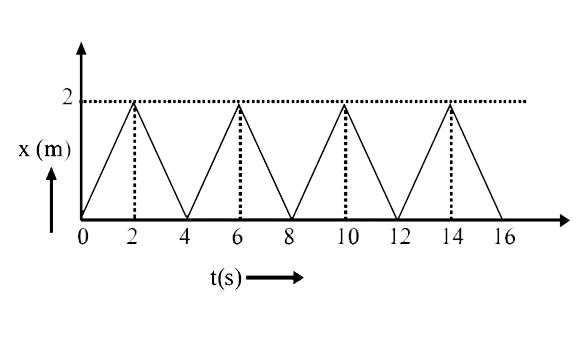
View SolutionThis question has statement I and statement II. Of the four choices given after the statements, choose the one that best describes the two statements.
Statement I: A point particle of mass m moving with speed v collides with stationary point particle of mass M. If the maximum energy loss possible glven as `f(1/2mv^2)` then `f=(m/(M+m))`
Statement II: Maximum energy loss occurs when the particles get stuck together as a result of the collision.
View SolutionA particle of mass m moving in the x direction with speed 2v is hit by another particle of mass 2m moving in they y direction with speed v. If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to :
View Solution
JEE Main And Advanced questions and complete solutions for chapter MOMENTUM & IMPULSE of SUNIL BATRA 41 YEARS IITJEE PHYSICS (HINGLISH) of Class 11