Complete Solutions to Example of chapter TRIGONOMETRIC FUNCTIONS AND IDENTITIES of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | TRIGONOMETRIC FUNCTIONS AND IDENTITIES |
| ARIHANT MATHS | Example |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Convert `40^o2 0^(prime)`into radian measure.
View SolutionExpress the following angle in degrees.
(i) `((5pi)/(12))^(c)` `" "` (ii) `-((7pi)/(12))^(c)`
(iii) `(1^(c))/(3)` `" "` (iv) `-(2pi^(c))/(9)`
View SolutionExpress the following angle in degrees, minutes and seconds form
`(321.9)^(@)`
View SolutionIn `DeltaABC , angleA=(2pi^(c))/(3)` and `angleB=45^(@)`. Find ` angleC` in both the systems.
View SolutionThe sum of two angles is `5 pi^(c)` and their difference is `60^(@)`. Find the angles in degrees.
View SolutionOne angle of a quadrilateral has measure `(2pi^(c))/(5)` and the measures of other three angles are in the ratio `2:3:4` . Find their measures in radians and in degrees.
View SolutionExpress the following angles in radians.
(i) `120^(@) " " (ii) -600^(@)`
(iii) `-144^(@)`
View SolutionThe angles of a quadrilateral are `x^(@),60^(@),60^(@) "and" (5pi^(c))/(6)`, Find x.
View SolutionIn the circle of 5cm.radius, what is the length of the are which subtends and angle of `33^(@)15'` at the centre.
View SolutionThe minute hand a watch is 35cm long. How for does its tip move in 18minutes? `(use pi=22/7)`
View SolutionThe wheel of a railway carriage is 40cm in diameter and makes 6 revolutions in a second, how fast is the train going?
View SolutionAssuming that a person of normal sight can red print to such distance that the letters subtend and angle of 5 at his eye, find is the height of the letters that he can read at a distance of 12 metres.
View SolutionShow That `2(sin^6x+cos^6x)-3(sin^4x+cos^4x)+1=0`
View SolutionShow that
(i) `sin^(8)A-cos^(8)A=(sin^(2)A-cos^(2)A)(1-2sin^(2)A.cos^(2)A)`
(ii) `(1)/(sec A-tan A)-(1)/(cos A)=(1)/(cos A)-(1)/(sec A + tan A)`
View SolutionIf `tantheta+sectheta=1. 5 ,` find `sintheta,tanthetaa n dsecthetadot`
View SolutionIf `(cos^4A)/(cos^2B)+(sin^4A)/(sin^2B)=1` then prove that `(cos^4B)/(cos^2A)+(sin^4B)/(sin^2A)=1`
View SolutionIf `tan^(2)theta=1-e^(2)`, then prove that `sectheta+tan^(3)theta"cosec"theta=(2-e^(2))^((3)/(2)).`
View SolutionShow that the equation `sec^2 theta=(4xy)/(x+y)^2` is only possible when x=y
View SolutionShow that the equation `sintheta=x+1/x` is not possible if `x` is real.
View SolutionIf `cos e ctheta-sintheta=ma n dsectheta-costheta=n ,e l i m in a t ethetadot`
View SolutionIf `3 sin theta + 4 cos theta=5`, then find the value of `4 sin theta-3 cos theta`.
View SolutionIf `a sec alpha-c tan alpha=d` and `b sec alpha + d tan alpha=c`, then eliminate `alpha` from above equations.
View SolutionEliminate `theta` from the equations `asectheta+btantheta+c=0` and `psectheta+qtantheta+r=0`
View SolutionIf `x=sectheta-tanthetaa n dy=cos e ctheta+cottheta,` then prove that `x y+1y-xdot`
View SolutionIf `x=rsinthetacosphi,y=rsinthetasinphi and z=rcostheta, then x^2+y^2+z^2` is independent of `(a)theta,phi` (b) `r ,theta` (c) `r ,phi` (d) r
View SolutionIf `0 lt theta lt pi/2, x= sum_(n=0)^(oo) cos^(2n) theta, y= sum_(n=0)^(oo) sin^(2n) theta` and `z=sum_(n=0)^(oo) cos^(2n) theta* Sin^(2n) theta`, then show `xyz=xy+z`.
(a) xyz = xz + y
(b) xyz = xy + z
(c) xyz = x + y + z
(d) xyz = yz + x
View SolutionIf in given fig, `tan ( angle BAO)=3`, then find the ratio `BC:CA`
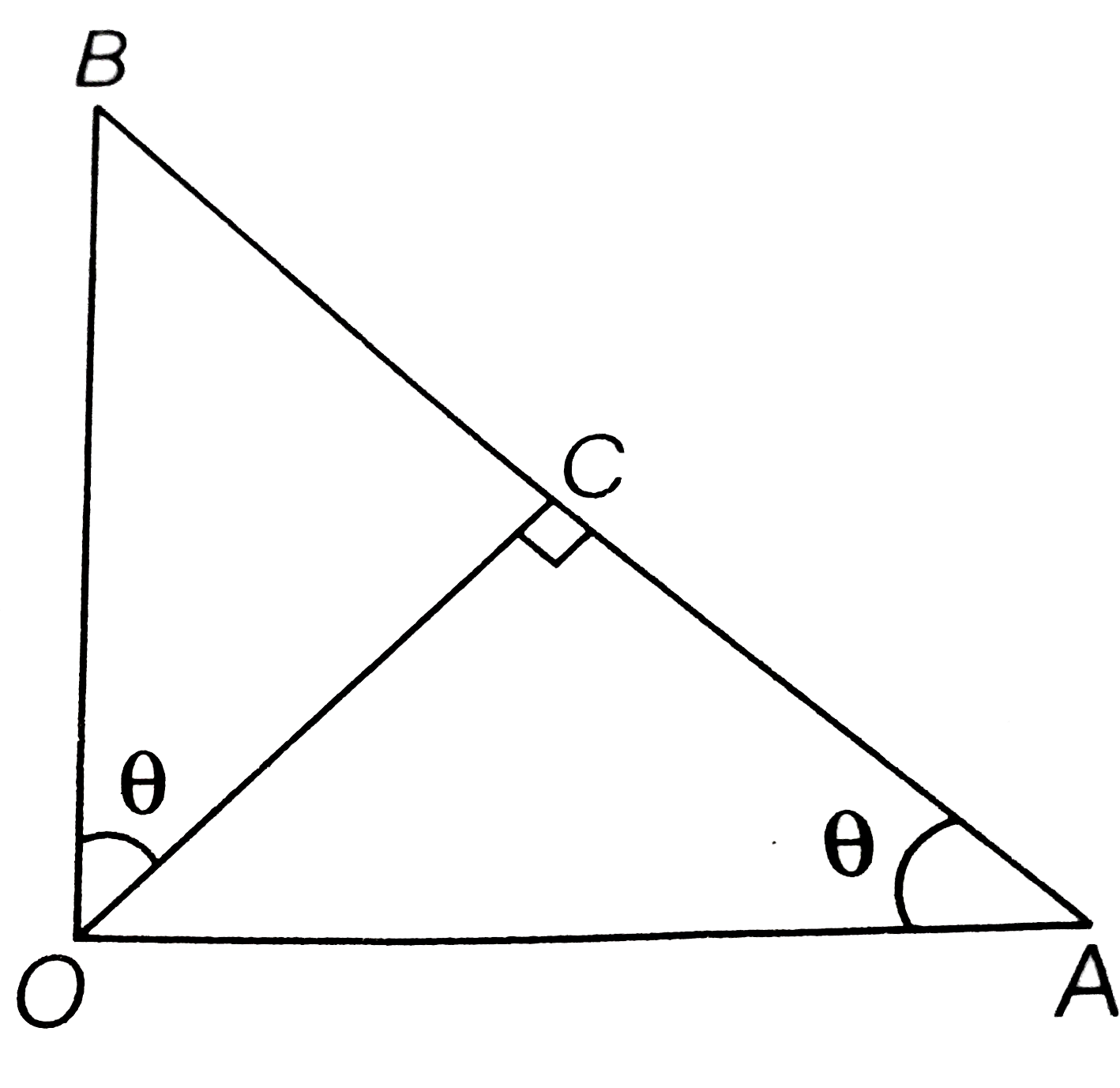
View SolutionIf angle C of triangle ABC is `90^0,` then prove that `tanA+tanB=(c^2)/(a b)` (where, `a , b , c ,` are sides opposite to angles `A , B , C ,` respectively).
View SolutionIn Triangle `A B C ,B C=8,C A=6a n dA B=10.` A line dividing the triangle `A B C` into regions of equal area is perpendicular to `A B` at point `Xdot` Find the value of `B X Isqrt(2)dot`
View SolutionLet `P Qa n dR S` be tangent at the extremities of the diameter `P R` of a circle of radius `rdot` If `P Sa n dR Q` intersect at a point `X` on the circumference of the circle, then prove that `2r=sqrt(P Q x R S)` .
View SolutionFind the values of the other five trigonometric functions in each of the following questions
(i) `tan theta = 5/12` , where `theta` is in third quadrant.
(ii) `sin theta = 3/5`, where `theta` is in second quadrant.
View SolutionIf `sintheta=(12)/(13)a n d\ theta` lies in the second quadrant, find the values of `s e ctheta+t a nthetadot`
View SolutionDraw the graph of `y=3 sin 2x`
View SolutionSketch the graph of `y=cos(x-pi/4)dot`
View SolutionWhich of the following is the least? sin 3 (b) sin 2 (c) sin 1 (d) sin 7
View SolutionFind the value of `x` for which `f(x)=sqrt(sinx-cosx)` is defined, `x in [0,2pi)dot`
View SolutionSolve `tanx >cotx ,` where `x in [0,2pi]dot`
View SolutionProve that: `sin^2(pi/6)+cos^2(pi/3)-t a n^2pi/4=-1/2`
View Solution`2sin^(2)""(pi)/(6)+cosec^(2)""(7pi)/(6) cos^(2)""(pi)/(3)=(3)/(2)`
View Solution`cot^2` `pi/6` `+cose c` `(5pi)/6` `+3tan^2` `pi/6=6`
View SolutionProve that: `2s in^2(3pi)/4+2cos^2pi/4+s e c^2pi/3=10`
View SolutionProve that `(cos(90^0+theta)sec(-theta)"tan"(180^0-theta))/(sec(360^0-theta)sin(180^0+theta)cot(90^0-theta))=-1`
View SolutionThe value of `(tan1^(@) tan2^(@) tan3^(@)……tan89^(@))` is
View SolutionShow that `sin^2 5^0+sin^2 10^0+sin^2 15^0++sin^2 90^0=9 1/2dot`
View SolutionFind the value of `cos^2pi/(16)+cos^2(3pi)/(16)+cos^2(5pi)/(16)+cos^2(7pi)/(16)dot`
View SolutionFind the values of the following: `tan105^0` ii. `tan(13pi)/(12)`
View SolutionProve that: `t a n 70^0=t a n 20^0+2t a n 50^0dot`
View SolutionIf `A+B=45^(@)` , show that `(1+tanA)(1+tan B)=2`.
View SolutionFind the value of `(tan 495^(@))/(cot 855^(@))`
View SolutionEvaluate `sin{ n pi+(-1)^(n) (pi)/(4)`, where n is an integer.
View SolutionProve that: `cos18^0-sin 18^0=sqrt(2)sin27^0`
View SolutionThe value of `cot((pi)/(4)+theta)cot((pi)/(4)-theta)` is
View SolutionIf `sinalphasinbeta-cosalphacosbeta+1=0,` then prove that `1+cotalphatanbeta=0`
View SolutionProve that `(sin(B-C))/(cos B cos C)+(sin(C-A))/(cos C cos A)+(sin(A-B))/(cos A cos B)=0`
View SolutionValue of tan `75^(@) + cot 75^(@) = ? `
View SolutionIf `t a nbeta=(ns inalphacosalpha)/(1-ns in^2alpha)` , show that `tan(alpha-beta)=(1-n)t a nalphadot`
View SolutionShow that `cos^(2)theta+cos^(2)(alpha+theta)-2cos alpha cos theta cos (alpha+beta)` is independent of `theta`.
View SolutionIf `3 tan theta tan phi=1`, then prove that 2 `cos (theta+varphi)=cos (theta-varphi)`.
View SolutionLet `A , B , C` be the three angles such that `A+B+C=pi` . If `tanA.tanB=2,` then find the value of `(cosAcosB)/(cosC)`
View Solution`(cos 10^0+s in 10^0)/(cos 10^0-s in 10^0)` is equal to `tan55^0` b. `cos 55^0` c.`-tan35^0` d. `-cot35^0`
View SolutionIf `sin(A-B)=1/(sqrt(10 ,))cos(A+B)=2/(sqrt(29)),fin dt h ev a l u eof2Aw h e r eAa n dBl i eb e t w e e n0a n dpi/4dot`
View SolutionProve that `(1+ tan 1^@ ) (1+ tan 2^@ )...(1+ tan 45^@ )= 2^23`
View SolutionIf `cos(alpha-beta)+cos(beta-gamma)+cos(gamma-alpha)=-3/2` , prove that `cosalpha+cosbeta+cosgamma=s inalpha+s inbeta+s ingamma=0`
View SolutionFind the value of `(cot 25^@+cot55^@)/(tan 25^@ + tan 55^@)+(cot 55^@ + cot100^@)/(tan 55^@ + tan 100^@)+(cot100^@ + cot 25^@)/(tan 100^@+tan 25^@)`
View SolutionProve that: `sum_(k=1)^(100)sin(k x)cos(101-k)x=50"sin"(101 x)`
View SolutionIf `A=pi/5,` then find the value of `sum_(r=1)^8tan(r A)tan((r+1)A)dot`
View Solutionprove that `sinthetadotsec3theta+sin3theta,sec3^2theta+sin3^2thetadotsec3^3theta+ u p ton` terms `=1/2[tan3^ntheta-tantheta]`
View SolutionIn a triangle ABC, if `sinAsin(B-C)=s9nCsin(A-B),` then prove that `cotA ,cotB ,cotCa r einAdotPdot`
View SolutionIf `0 lt beta lt alpha lt pi//4, cos(alpha+beta)=3//5` and ` cos(alpha-beta)=4//5`, then evaluate `sin 2alpha`.
View Solutionif `cosalpha=1/2(x+1/x)andcosbeta=1/2(y+1/y),(xygt0),x,y,alpha,beta, inR`then
View SolutionIf `2 sin alphacos beta sin gamma=sinbeta sin(alpha+gamma),then tan alpha,tan beta and gamma` are in
View SolutionProve that `cos 55^(@)+cos65^(@)+cos175^(@)=0`
View SolutionProve that `(sinA+sin2A+sin4A+sin5A)/(cos A+cos2A+cos 4A+cos5A)=tan3A`.
View SolutionProve that: `(cosalpha+cosbeta)^2+(sinalpha+sinbeta)^2=4cos^2((alpha-beta)/2)`
View SolutionIf `sinA=sinBa n dcosA=cosB ,` then prove that `sin(A-B)/2=0`
View SolutionProve that: `sin20^0sin40^0sin80^0=(sqrt(3))/8`
View SolutionProve that: `"s i n A s i n"(60-"A")sin(60+"A")=1/4sin3"A"dot`
View SolutionIf `sin A=3/5,w h e r e0^0ltAlt90^0` , then find the values of `sin2A , cos2A ,tan2Aa n dsin4A`
View SolutionProve that `(1+sin2theta)/(1-sin2theta)=((1+tantheta)/(1-tan theta))^(2)`
View SolutionProve that `(1-tan^2(pi/4-A))/(1+tan^2(pi/4-A))=sin2Adot`
View SolutionProve that:`(sec8theta-1)/(sec4theta-1)=(tan8theta)/(tan2theta)`
View SolutionShow that: `sqrt(2+sqrt(2+sqrt(2+2cos8theta)))=2costheta,0
View SolutionShow that `sqrt(3)\ cos e c\ 20^0-sec20^0=4.`
View SolutionThe value of `(1+cospi/8)(1+cos(3pi)/8)(1+cos(5pi)/8)(1+cos(7pi)/8)i s` 1/4 (b) 3/4 (c) 1/8 (d) 3/8
View SolutionIf `t a n^2theta=2t a n^2varphi+1` , prove that `cos2theta+s in^2varphi=0.`
View Solutionprove that : `tan(alpha)+2 tan(2alpha) +4(tan4alpha)+8cot(8alpha) = cot(alpha)`
View SolutionThe smallest positive value of x (in degrees) for which `tan(x+100^@)=tan(x+50^@).tanx.tan(x-50^@)` is
View SolutionIf `s in A=(12)/(13)a n dsinB=4/5,\ w h e r e\ pi/2
View SolutionShow that `(1+tan .theta/2)/(1-tan. theta/2)=(1+sintheta)/(cos theta)=tan(pi/4+theta/2)`
View SolutionProve that `(cos A + cos B ) ^(2) +(sin A + sin B) ^(2) = 4 cos ^(2) ((A -B)/(2)).`
View SolutionProve that: `cos^4pi/8+cos^4(3pi)/8+cos^4(5pi)/8+cos^4(7pi)/8=3/2`
View SolutionFind the value of tan `pi/8`.
View SolutionIf `tan x = -(4)/(3), (pi)/(2) lt x lt pi`, then find the value of `sin(x/2), cos(x/2)` and `tan(x/2)`.
View SolutionFind the value of `sin. (23pi)/(24)` .
View SolutionIf `alpha = 112^(@) 30'`, find the value of `sin alpha ` and `cos alpha`
View SolutionIf `t a ntheta/2=sqrt((a-b)/(a+b))tanvarphi/2` , prove that `costheta=(a cosvarphi+b)/(a+b cosvarphi)` .
View SolutionIf `cos theta=(cos alpha-cos beta)/(1-cos alpha cos beta),`prove that `tan theta/2=+-tan alpha/2 cot beta/2.`
View SolutionProve that `4 cos 6^(@) cos 42 ^(@) cos 66^(@) cos 78^(@) = (1)/(4).`
View SolutionProve that: `sin(pi/5)sin( (2pi)/5)sin(3pi/5)sin(4pi/5)=5/(16)`
View SolutionFind the value of
`(i) sin 22^(@) 30'` (ii) cos `22^(@) 30'` (iii) tan `22^(@) 30'`
View Solution
Example questions and complete solutions for chapter TRIGONOMETRIC FUNCTIONS AND IDENTITIES of ARIHANT MATHS (ENGLISH) of Class 12
All Exercises from ARIHANT MATHS Class 12 MATHS TRIGONOMETRIC FUNCTIONS AND IDENTITIES
- Example
- Exercise (Matching Type Questions)
- Exercise (More Than One Correct Option Type Questions)
- Exercise (Passage Based Questions)
- Exercise (Questions Asked In Previous 13 Years Exam)
- Exercise (Single Integer Answer Type Questions)
- Exercise (Single Option Correct Type Questions)
- Exercise (Statement I And Ii Type Questions)
- Exercise (Subjective Type Questions)
- Exercise For Session 1
- Exercise For Session 10
- Exercise For Session 11
- Exercise For Session 2
- Exercise For Session 3
- Exercise For Session 4
- Exercise For Session 5
- Exercise For Session 6
- Exercise For Session 7
- Exercise For Session 8
- Exercise For Session 9
- Solved Examples : Single Option Correct Type Questions