Complete Solutions to Examples of chapter TRIGONOMETRIC EQUATIONS AND INEQUATIONS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | TRIGONOMETRIC EQUATIONS AND INEQUATIONS |
| ARIHANT MATHS | Examples |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
If ` sin alpha , 1 , cos 2 alpha ` are in GP, then find the general solution for ` alpha `
View SolutionIf `1/6 sin theta, cos theta` and `tan theta` are in G.P. then the general solution for `theta` is
View SolutionSolve `sin^(2) theta-cos theta=1/4, 0 le theta le 2pi`.
View SolutionSolve `cos theta+cos 7 theta+cos 3theta+cos 5 theta=0`,
View SolutionFind the number of solution for , `sin 5 theta * cos 3 theta = sin 9 theta * cos 7 theta ` in `[0,(pi)/2]`
View SolutionSolve `( sin x + "i" cos x )/( 1 +i),i =sqrt(-1)` when it is purely imaginary .
View SolutionFind the most general solution of `2^1|cosx|+cos^2x+|cosx|^(3+oo)=4`
View SolutionIf `x!=(npi)/2,\ n\ in I` and `( cosx )^(sin ^2x-3sinx+2)=1,` then find the general solution of `xdot`
View SolutionSolve `(tan 3x - tan 2x)/(1+tan 3x tan 2x)=1`.
View SolutionSolve ` sin x=0 and ( sin x )/(cos ""(x)/2 cos ""(3x)/2)=0` and show their solutions are different .
View SolutionFind the number of solution of ` tan x + sec x =2 cos x ` in `[0,2pi]`
View SolutionSolve `secx-1=(sqrt(2)-1)tanx`
View SolutionSolve ` tan theta + tan 2 theta + tan theta * tan 2 theta * tan 3 theta=1 `
View SolutionFind the number of solutions of `|cos x|=sin x , 0 le x le 4 pi`
View SolutionSolve ` cot theta = sin 2 theta ` by substituting ` sin 2 theta =( 2 tan theta )/( 1+tan^(2) theta )` and again by substituting ` sin 2 theta = 2 sin theta * cos theta ` and check whether the two answer are same or not .
View SolutionProve that the equation ` p cos x - q sin x =r ` admits solution for x only if `-sqrt(p^(2)+q^(2)) lt r lt sqrt(p^(2)+q^(2))`
View SolutionSolve the equation `sqrt3cos x + sin x = sqrt2 `.
View SolutionFind the number of distinct solution of ` sec x + tan x =sqrt(3)` , where ` 0 le x le 3pi`
View SolutionProt that the equation `k cos x-3sin x=k+1` possess a solution if `k in (-oo,4]`.
View SolutionLet [x] = the greatest integer less than or equal to x and let `f (x) = sin x + cosx.` Then the most general solution of `f(x)=[f(pi/10)]` is
View SolutionFind the number of solutions of ` cos x =|1+ sin x |, 0 le x le 3 pi`
View SolutionSolve the following equation: `\ 3cos^2theta-2\ sqrt(3)sinthetacostheta-3sin^2theta=0`
View SolutionSolve the equation ` 5 sin^(2)x-7 sinx cos x + 16 cos^(2)x=4 `
View SolutionSolve the equation `( cos x - sin x )(2 tan x +(1)/( cos x ))+2 =0`
View SolutionSolve : `sin^(10)x+cos^(10)x=29/16cos^4 2x`
View SolutionSolve the equation ` sin x + cos x -2sqrt2 sin x cos x =0`
View SolutionFind the most general value of ` theta ` which satisfies the equation ` sin theta =(1)/(2) and tan theta =(1)/(sqrt(3))`
View SolutionIf `tan(A-B)=1, and sec(A+B)=2/sqrt3,` find the smallest positive values of A and B and also their most general values.
View SolutionIf `x+y=2pi//3` and `sin x//sin y=2`, then the general solution for x and y
View SolutionIf ` r gt 0, - pi le theta le pi and r, theta ` satisfy ` r sin theta =3 and r=4(1+ sin theta )` , then find the possible solutions of the pair `(r , theta )`
View SolutionThe equation `2 "cos"^(2)((x)/(2))"sin"^(2) x = x^(2) + (1)/(x^(2)), 0 le x le (pi)/(2)` has
View SolutionGeneral solution of equation `sin^6x=1+cos^4 3x`
View Solutionsolve `sin^4x=1+tan^8x`
View SolutionSolve `sin^2 x+ cos^2 y = 2 sec^2 z `
View SolutionThe number of real solution of the equation `sqrt(1 + cos 2x) = sqrt2 sin^(-1) (sin x), -pi le x le pi`, is
View SolutionFind the most general solution for ` 2^(sin x )+2^( cos x )=2^((1-(1/sqrt2)))`
View SolutionSolve ` |sqrt(3) cos x - sin x |ge 2` for ` x in [0,4pi]` .
View SolutionShow that the equation , ` sin x =[1+ sin x]+[1- cos x]` has no solution for ` x in R `. ( where [.] represents greatest integers functions ).
View SolutionFind the solution set of the inequality `sinx > 1/2.`
View SolutionFind the solution set of the inequality `cosx>= (-1)/2.`
View SolutionNumber of solutions of the equation `sin^(4) x-cos^(2)x sin x + 2 sin^(2) x+sin x=0` in `0 le x le 3pi` is ________.
View SolutionSolve : `2 cos^(2)theta + sin theta le 2`, where `pi//2 le theta le 3pi//2.`
View SolutionSolve `sin^(2) theta gt cos^(2) theta`.
View SolutionFind the solution set for , `| tan x | le 1` when ` x in [-pi, pi]`
View SolutionSolve the inequality `sin2x gtsqrt(2)sin^2x+(2-sqrt(2))cos^2x`
View SolutionSolve `tan^(3) x+3 gt 3tan x+tan^(2) x`.
View SolutionThe number of solution of equation ` 8[x^(2)-x]+4[x]=13+12[sinx],[.]` denotes GIF is
View SolutionThe total number of ordered pairs `(x , y)` satisfying `|x|+|y|=2,sin((pix^2)/3)=1,` is equal to 2 (b) 3 (c) 4 (d) 6
View SolutionSolve the equation: `cos^2[pi/4(sinx+sqrt(2)cos^2x)]-tan^2[x+pi/4tan^2x]=1`
View Solutionthe general solution of `sin^2thetasectheta+sqrt3 tantheta=0` is
View SolutionThe number of solutions of the equation `sin((pix)/(2sqrt3))=x^2-2sqrt3x+4`
View Solution` x_(1) and x_(2)` are two solutions of the equation ` e^(x) cos x=1 ` , The minimum number of the solution of the equation ` e^(x) sin x =1`, lying between ` x_(1) and x_(2)` can be
View SolutionThe product of common differences of all possible AP which are made from values of 'x' satisfying `cos^(2)((1)/(2)lambda x )+ cos^(2)((1)/(2) mu x )=1 `
View SolutionNumber of solutions of the equation `cos^(4) 2x+2 sin^(2) 2x`
`=17 (cos x + sin x)^(8), 0 lt x lt 2 pi` is
View SolutionThe number of values of `theta` in the interval `(-pi/2,pi/2)` satisfying the equation `(sqrt(3))^sec^(2theta)=tan^4theta+2tan^2theta` is 2 (b) 4 (c) 0 (d) 1
View SolutionNumber of solutions of the equation ` cot( theta ) + cot( theta +(pi)/(3))+ cos ( theta -(pi)/(3))+ cot( 3 theta ) =0` , where ` theta in ( 0,(pi)/(2))`
View Solution`0 lt a lt 2 pi , sin^(-1)(sin a ) lt x^(2)-2x ` for all ` x in I` then ` a in `
View SolutionIf `(cos^(2)x + 1/(cos^(2) x)) (1+tan^(2) 2y) (3+ sin 3z)=4`, then
View SolutionSolve `(sqrt(5)-1)/(sinx)+(sqrt(10+2sqrt(5)))/(cosx)=8,x in (0,pi/2)`
View Solutionfind the range of values of t for which `2sint=(1-2x+5x^2)/(3x^2-2x-1)`
View SolutionThe system of equations `tan x=a cot x, tan 2x=b cos y`
View SolutionIf `(y+3)/(2y+5)=sin^2x+2cosx+1,` then the value of `y` lies in the interval `(-oo,-8/3)` (b) `(-(12)/5,oo)` `(-8/3,-(12)/5)` (d) `(-8/3,oo)`
View SolutionWhich of the following set of values of x satisfies the equation `2^((2 sin^(2) x-3sinx +1))+2^((2-2 sin^(2)x+3 sin x))=9` ?
View SolutionFor `0 lt theta lt pi/2` , the solution (s) of `sum_(m=1)^6cos e c(theta+((m-1)pi/4)) cos e c(theta+(mpi)/4) = 4sqrt(2) is(a r e)` (a) `pi/4` (b) `pi/6` (c) `pi/(12)` (d) `(5pi)/(12)`
View SolutionIf ` (1+ sin6^(@))/( cos 6^(@))=tan A =sqrt((1+ sinB)/(1- sin B)),` where A and `B in (0,90^(@))` , then
View SolutionIf ` sqrt(1+ sin A )-sqrt(1-sin A )=2 cos""(A)/(2)`, then value of A can be
(a)`110^(@)` (b)`260^(@)` (c)`300^(@)` (d)`190^(@)`
View SolutionConsider a circle , in which a point P is lying inside the circle such that `(PA)(PB)=(PC)(PD)` ( as shown in figure ) .
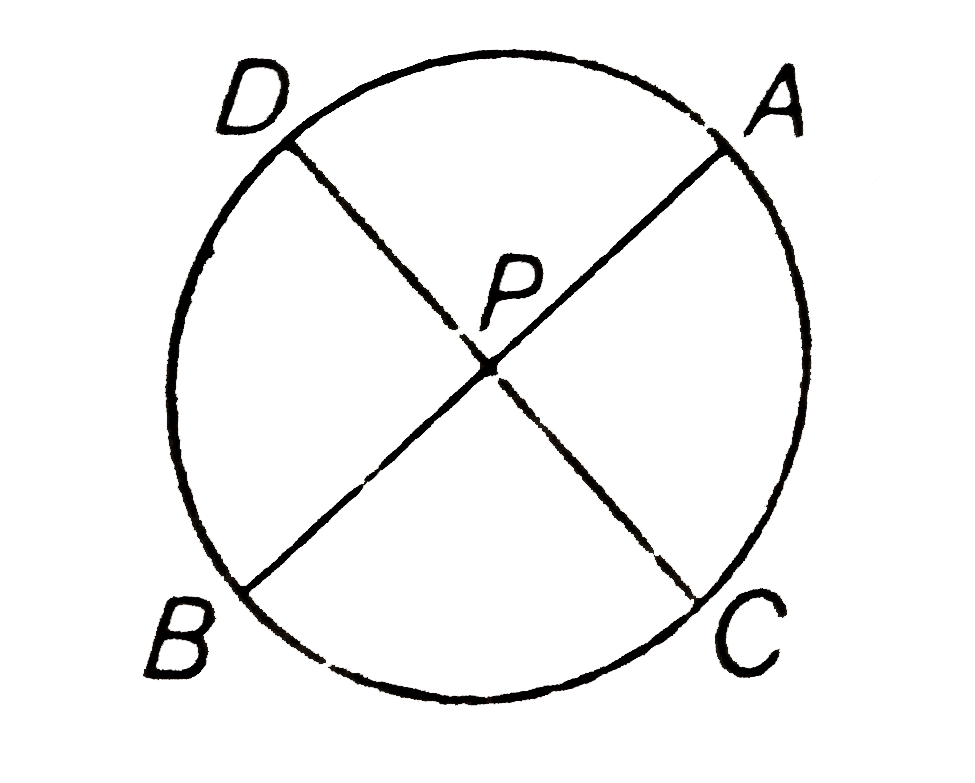
On the basis of above information , answer the question:
Let PA=4 , PB=3 cm and CD is diameter of the circle having the length 8 cm. If `PC gt PD` , then `(PC)/(PD) ` is equal to
View SolutionConsider a circle , in which a point P is lying inside the circle such that `(PA)(PB)=(PC)(PD)` ( as shown in figure ) .
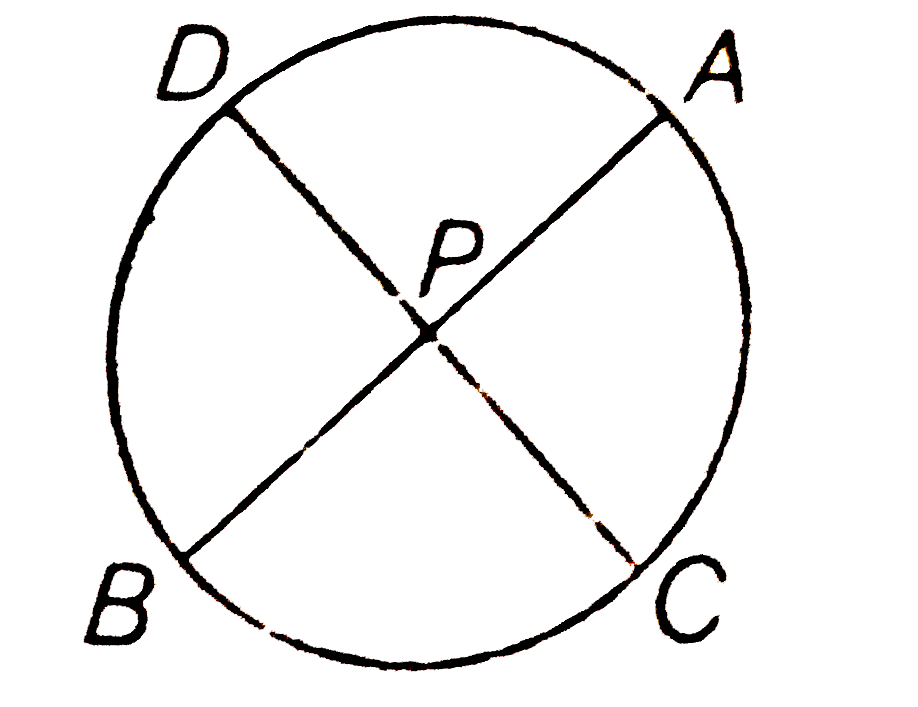
On the basis of above information , answer the questions
If `PA=| cos theta + sin theta | and PB=| cos theta - sin theta |`, then maximum value of (PC)(PD) , is equal to
View SolutionConsider a circle , in which a point P is lying inside the circle such that `(PA)(PB)=(PC)(PD)` ( as shown in figure ) .
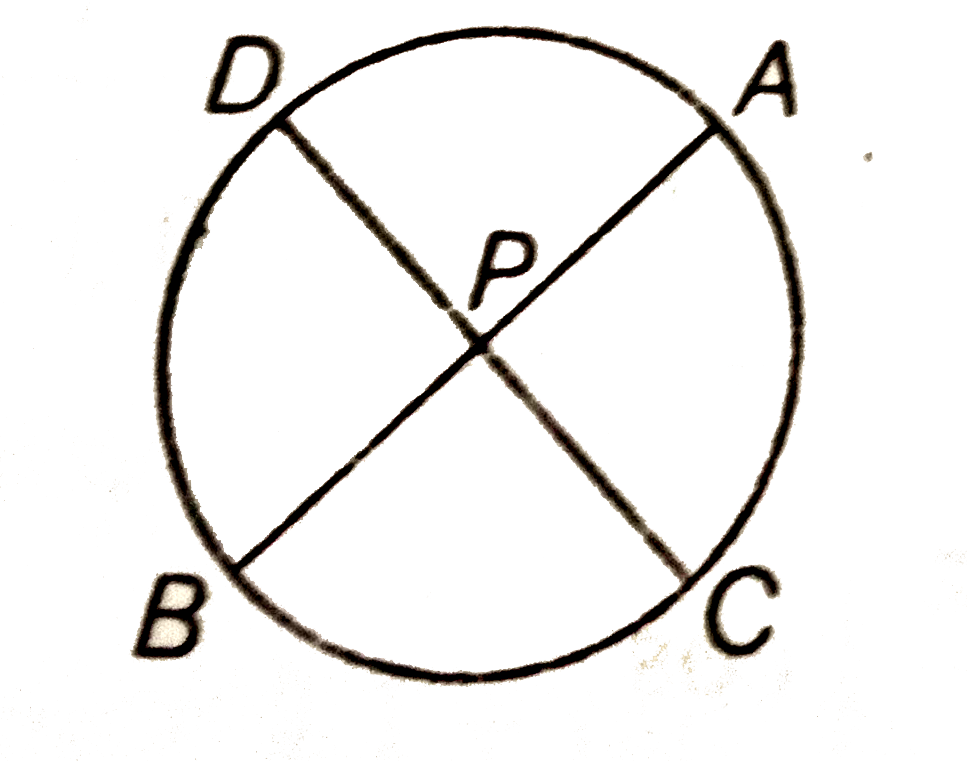
On the basis of above information , answer the questions
If ` log_(PA) x=2 , log_(PB)x=3, log_(x) PC=4 , ` then ` log_(PD)` x is equal to
View SolutionPA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ` angleAPB= theta `.
On the basis of above information answer the questions .
Area of `DeltaAPB` is
View SolutionPA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ` angleAPB= theta `.
On the basis of above information answer the questions .
value of sin2theta + cos4theta +sin5theta+tan7theta +cos 8theta
View SolutionPA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ` angleAPB= theta `.
On the basis of above information answer the questions .
Number of solution(s) of the equation `log_(cos theta )(x+2)=2+3 log_((x+2)) sin""((5 theta )/(2))` is
View Solution` 3sin^(2)x-7sin x +2=0, x in [0,(pi)/(2)] and f_(n)(theta)=sin^(n) theta + cos^(n) theta ` .
On the basis of above information, the value of `f_(4)(x) ` is:
View Solution` 3sin^(2)x-7sin x +2=0, x in [0,(pi)/(2)] and f_(n)(theta)=sin^(n) theta + cos^(n) theta ` .
On the basis of above information, the value of ` ( sin 5 x + sin 4 x)/( 1+ 2 cos 3 x)` is:
View SolutionNumber of integral solution of the equation ` log_(sin x) sqrt(sin^(2)x)+ log_(cos x)sqrt( cos^(2)x)=`, where ` x in [0,6pi]` is
View SolutionIf `x_k = (sectheta)^(1/2^k)+ (tan theta)^(1/(2^k) ` `and y_k = (sectheta)^(1/(2^k))-(tan theta)^(1/2^k)` , then value of `3y_n prod_(k=0)^n (x_k)` is equal to
View SolutionThe number of ordered pairs `(alpha , beta )` , where ` alpha , beta in [0,2pi]` satisfying ` log_(2 sec x )(beta^(2)-6beta+10)=log_(3)| cos alpha |` is
View SolutionIf ` (cos^(3) theta )/( (1- sin theta ))+( sin^(3) theta )/( (1+ cos theta ))=1+ cos theta `, then number of possible values of ` theta ` is ( where ` theta in [0,2pi]`)
View SolutionIf the sum of all value of x satisfying the system of equations
`tan x + tan y+ tan x* tan y=5 `
`sin (x +y)=4 cos x * cos y`
is `(k pi )/2` , where ` x in (0, (pi)/(2))` then find the values of k .
View SolutionStatement I ` x =(kpi)/(13), k in I` does not represent the general solution of trigonometric equation.
` sin 13 x - sin 13 x cos 2x=0`
Statement II Both ` x=r pi , r in I and x=(k pi )/(13) , k in I ` satisfies the trigonometric equation.
`sin 13 x - sin 13 x cos 2x =0`
View SolutionFind `dy/dx if 2x-3y=sinx-cosx`
View SolutionIf ` 0 le x le 3 pi , 0 le y le 3 pi and cos x * sin y=1 `, then find the possible number of values of the orederd pair (x,y) .
View SolutionIf ` theta in [0,3 pi] and r in R ` . Then , find the pair of `( r , theta )` satisfying` 2 sin theta = r^(4)- 2r^(2)+3 ` .
View SolutionFind all the value of ` theta ` satisfying the equation , ` sin 7 theta = sin theta + sin 3 theta ` such that ` 0 le theta le pi`
View SolutionSolve ` sin 3x + cos 2 x =-2 `
View Solution`sin(3theta+alpha)+sin(3theta-alpha)+sin(alpha-theta)-sin(alpha+theta)=cosalpha`
View SolutionSolve `sin 2x+cos 4x=2`.
View SolutionFind all the solution of ` 4 cos^(2)x sinx -2 sin^(2) x=3 sinx `
View SolutionSolve the equation: `1 + 2 cosecx = -(sec^2 (x/2))/2`
View SolutionFind all values of `theta ` lying between `0` and `2 pi` satisfying the equation `r sin theta = sqrt 3` and `r + 4 sin theta =2(sqrt 3+1)`
View SolutionSolve the following system of equations. ` sin x + cos y=1, cos 2x-cos 2y=1 `
View SolutionFind the coordinates of the points of intersection of the curves `y=cosx , y=sin3x if-pi/2lt=xlt=pi/2`
View SolutionFind the range of y such that the equation in x , ` y + cos x = sin x ` has a real solutions . For `y=1` , find x such that ` 0 lt x lt 2 pi`
View SolutionA triangle `ABC` is such that `sin(2A+B) =1/2` and If `A, B` and `C` are in `A.P.,` then find the value of `A` and `C`
View SolutionFind the values of `theta` in the interval `(-pi/2,pi/2)` satisfying the equation `(1-tantheta)(1+tantheta)sec^2theta+2^tan2θ =0`
View SolutionSolve `sin x("cos"x/4-2 sin x)+(1+"sin"x/4-2 cos x)cos x =0`.
View SolutionFind all possible triplets (x,y,z) such that `(x+y)+(y+2z) cos 2 theta +(z-x) sin^(2) theta =0` , for all ` theta `.
View SolutionFor every real number find all the real solutions to equation ` sin x + cos(a+x)+ cos (a-x)=2 `
View SolutionSolve the equation ` (tan x )^( cos^(2) x)= ( cot x )^( sin x )`
View SolutionSolve the equation ` a cos x + cot x +1="cosec " x `
View Solution
Examples questions and complete solutions for chapter TRIGONOMETRIC EQUATIONS AND INEQUATIONS of ARIHANT MATHS (ENGLISH) of Class 12
All Exercises from ARIHANT MATHS Class 12 MATHS TRIGONOMETRIC EQUATIONS AND INEQUATIONS
- Examples
- EXAMPLES ( Matching Type Questions )
- EXAMPLES ( Subjective Type Examples )
- Exercise (Matching Type Questions)
- Exercise (More Than One Correct Option Type Questions)
- Exercise (Passage Based Questions)
- Exercise (Questions Asked In Previous 13 Years Exam)
- Exercise (Single Integer Answer Type Questions)
- Exercise (Single Option Correct Type Questions)
- Exercise (Statement I And Ii Type Questions)
- Exercise (Subjective Type Questions)
- Exercise For Session 1
- Exercise For Session 2
- Exercise For Session 3
- Exercise For Session 4