Complete Solutions to Exercise (Subjective Type Questions) of chapter AREA OF BOUNDED REGIONS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | AREA OF BOUNDED REGIONS |
| ARIHANT MATHS | Exercise (Subjective Type Questions) |
| Number Of Questions | 16 |
| Academic Year | 2023-24 |
Find the continuous function `f` where `(x^4-4x^2)lt=f(x)lt=(2x^2-x^3)` such that the area bounded by `y=f(x),y=x^4-4x^2dot` then y-axis, and the line `x=t ,` where `(0lt=tlt=2)` is `k` times the area bounded by `y=f(x),y=2x^2-x^3`,y-axis , and line `x=t(w h e r e0lt=tlt=2)dot`
View SolutionLet `f(t)=|t-1|-|t|+|t+1|, AA t in R`. Find `g(x) = max {f(t):x+1letlex+2}, AA x in R`. Find `g(x)` and the area bounded by the curve `y=g(x)`, the X-axis and the lines `x=-3//2 and x=5`.
View SolutionLet f(x)= minimum `{e^(x),3//2,1+e^(-x)},0lexle1`. Find the area bounded by `y=f(x)`, X-axis and the line x=1.
View SolutionFind the area bounded by `y=f(x)` and the curve `y=2/(1+x^2)` satisfying the condition `f(x),f(y)=f(xy) AA x,y in R and f'(1)=2,f(1)=1`,
View SolutionThe value of
`overset(sin^(2)x)underset(0)int sin^(-1)sqrt(t)dt+overset(cos^(2)x)underset(0)int cos^(-1)sqrt(t)dt`, is
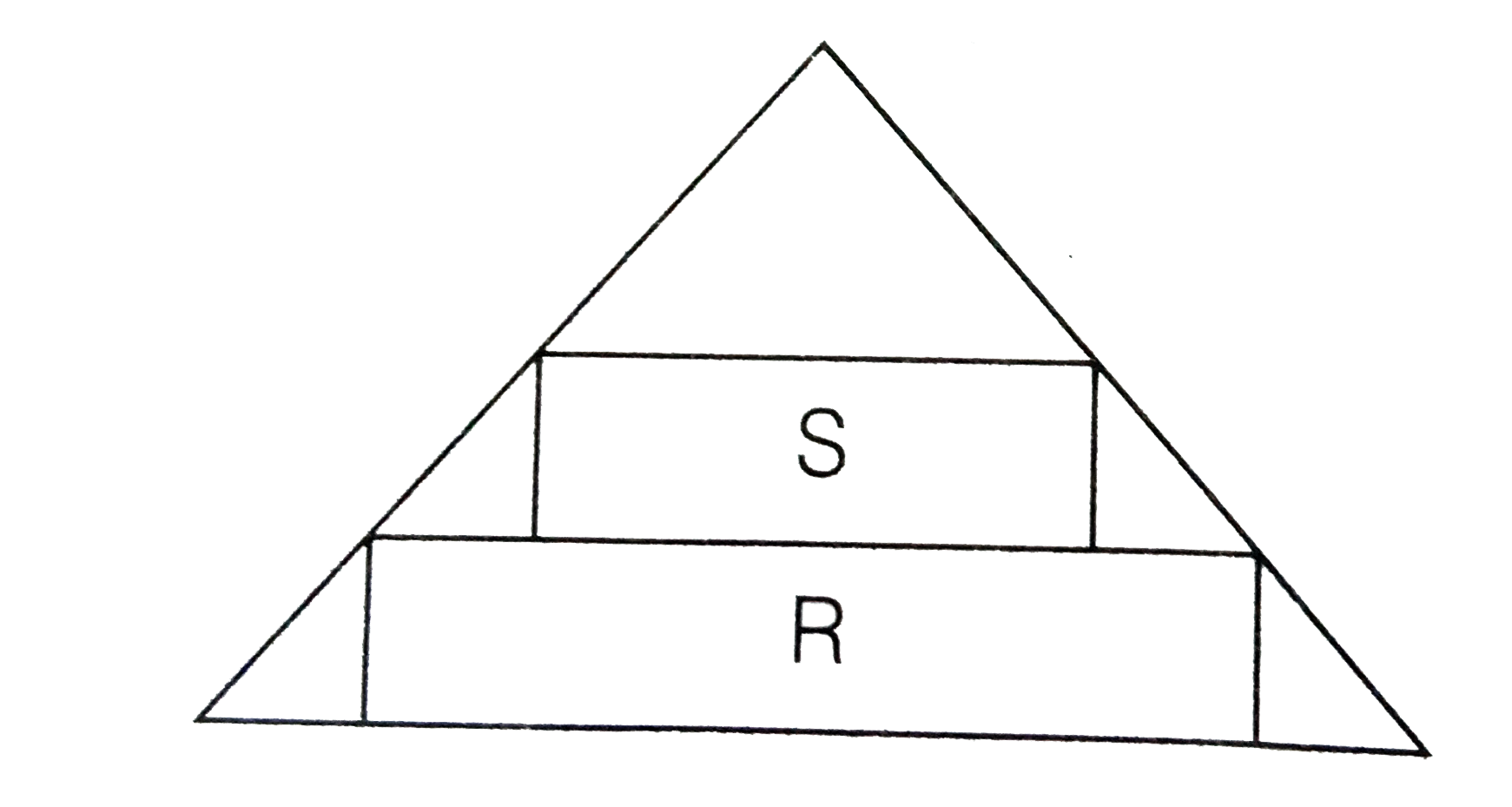
View SolutionLet T be an acute triangle Inscribe a pair R,S of rectangle in T as shown:
Let `A(x)` denote the area of polygon X find the maximum value (or show that no maximum exists), of `(A(R)+A(S))/(A(T))`, where T ranges over all triangles and R,S over all rectangle as above.
 .
.
View SolutionFind the maximum area of the ellipse that can be inscribed in an isoceles triangles of area A and having one axis lying along the perpendicular from the vertex of the triangles to its base.
View SolutionFind the area of the region bounded by curve `y=25^(x)+16` and the curve `y=b.5^(x)+4`, whose tangent at the point x=1 make an angle `tan^(-1)` (40 In 5) with the X-axis.
View SolutionIf the circles of the maximum area inscriabed in the region bounded by the curves `y=x^(2)-2x-3` and `y=3+2x-x^(2)` , then the area of region `y-x^(2)+2x+3le0,y+x^(2)-2x-3le0 and sle0`.
View SolutionFind limit of the ratio of the area of the triangle formed by the origin and intersection points of the parabola `y=4x^2` and the line `y=a^2` to the area between the parabola and the line as `a` approaches to zero.
View SolutionFind the area of curve enclosed by `|x+y|+|x-y|le4,|x|le1, y ge sqrt(x^(2)-2x+1)`.
View SolutionCalculate the area enclosed by the curve `4lex^(2)+y^(2)le2(|x|+|y|)`.
View SolutionFind the area enclosed by the curve `[x]+[y]-4` in 1st quadrant (where [.] denotes greatest integer function).
View SolutionSketch the region and find the area bounded by the curves `|y+x|le1,|y-x|le1` and `2x^(2)+2y^(2)ge1`.
View SolutionFind the area of the region bounded by the curve `2^(|x|)|y|+2^(|x|-1)le1`, with in the square formed by the lines `|x|le1//2,|y|le1//2`.
View SolutionThe value of the parameter `a(a>=1)` for which the area of the figure bounded by the pair of staight lines `y^2-3y+2=0` and the curves `y=[a]x^2, y=1/2[a]x^2` is greatest is (Here [.] denotes the greatest integer function). (A) `[0,1)` (B) `[1,2)` (C) `[2,3)` (D) `[3,4)`
View Solution
Exercise (Subjective Type Questions) questions and complete solutions for chapter AREA OF BOUNDED REGIONS of ARIHANT MATHS (ENGLISH) of Class 12
All Exercises from ARIHANT MATHS Class 12 MATHS AREA OF BOUNDED REGIONS
- Area of bounded Regions Exercise 5: Matching Type Questions
- Area of bounded Regions Exercise 7: Subjective Type Questions
- Examples
- Exercise (More Than One Correct Option Type Questions)
- Exercise (Passage Based Questions)
- Exercise (Questions Asked In Previous 13 Years Exam)
- Exercise (Single Integer Answer Type Questions)
- Exercise (Single Option Correct Type Questions)
- Exercise (Statement I And Ii Type Questions)
- Exercise (Subjective Type Questions)
- Exercise For Session 1
- Exercise For Session 2