Complete Solutions to EXAMPLE of chapter LINEAR PROGRAMMING of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | LINEAR PROGRAMMING |
| BODY BOOKS PUBLICATION | EXAMPLE |
| Number Of Questions | 62 |
| Academic Year | 2023-24 |
Food X contains 6 units of vitamins A and 7 units of vitamins B per gram and it costs 12 paise per gram.Food Y contains 8 units of vitamin A and 12 units of vitamins B per gram and it costs 20 paise per gram.Daily minimum requiremrnts of vitamin A and vitamin B are 100 units and 120 units respectively.Formulate the LPP mathematically so that the cost is to be minimized.
View SolutionManu has Rs.36000 for purchases of rice and wheat cost Rs.180 and Rs.120 respectively.He has storage capacity for 250 bags only.He earns a profit of Rs.11 and Rs.9 per bag of rice and wheat respectively.(i) Formulate an LPP to maximize the profit.
View SolutionManu has Rs.36000 for purchases of rice and wheat cost Rs.180 and Rs.120 respectively.He has storage capacity for 250 bags only.He earns a profit of Rs.11 and Rs.9 per bag of rice and wheat respectively.Slove the LPP.
View SolutionDraw the graph of 5x+10y=50,x+y=1,y=4,x,y=0.
View SolutionSolve graphically, the linear programming problem: Minimize Z=2x+y subject to `5x+10yle50,x+yge1,yle4`,`x,yge0`
View SolutionA manufacture make two types of furniture,chairs and tables.Both the products are processed on three machines `A_1,A_2 and A_3`.Machine `A_1` requires 3 hours for a chair and 3 hours for a table,machine `A_2`requires 5 hours for a chair and 2 hours for a table and machine `A_3` requires 2hours for a chair and 6 hours for a table.Maximum time available on machine `A_1,A_2 and A_3` is 36 hours,50 hours and 60 hours respectively.Profits are Rs.20 per chair and Rs.30 per table.Formulate the above as a linear programming problem to maximize the profit.
View SolutionSolve the following problem graphically :Minimize,Z=3x+3y Subject to constraints:`x+yge8, 3x+5yle15xge0,yge0`
View SolutionThe region other than the feasible region is called_______
View SolutionIf the feasible region of a linear programming is bounded,then it is always a _______
View SolutionWho invented Linear programming problem?
View SolutionA person deals only two items,Cycles and scooters.He has Rs.1,20,000 to invest and a space to store at most 38 pieces.One scooter costs him Rs.12000 and a cycle costs him Rs.800.He can sell a scooter at a profit of Rs.1500 and a cycle at a profit of Rs.200.Assuming that he can sell all the items he buys,how should he invest his money in order that he may maximum his profit.Formulate the problem mathematically.
View SolutionThe corner points of the feasible region determined by the following system of inequalities: `2 x+y le 10, x+3 y le 15, x, y ge 0` are `(0,0),(5,0),(3,4)`, and `(0,5)`. Let `Z=p x+q y`, where `p, qgt0`. Condition on `p` and `q` so that the maximum of `Z` occurs at both `(3,4)` and `(0,5)` is a)`p=q` b)`p=2q` c)`p=3q` d)`q=3p`
View SolutionA firm manufactures 3 products A,B and C.The profit are Rs.3,Rs.2 and Rs.4 respectively.The firm has 2 machines and below is the required processing time in minutes for each machine on each product:Machine `M_1 and M_2` have 2000 and 2500 machine minutes respectively.The firm must manufacture 100A's,200B's and 50C's but not more than 150A's.Set up a LPP to maximize the profit.

View SolutionDetermine graphically the minimum value of the objective function `Z=-50 x+20 y` subject to the constraints: `2 x-y ge -5`, `3 x+y ge 3`, `2 x-3 y le 12`, `x ge 0, y ge 0`
View SolutionA company products two aricles X and Y.There are two different departments through which the articles are processed namely assembly and finishing.The potential capacity of the assembly department is 60 hours,a week and that of finishing department is 48 hours a week.Production of one unit of X requires 4 hours of assembly and 2 hours of finishing.Each of the unit Y requires 2 hours in assembly and 4 hours in finishing.If profit is Rs.8 for each unit of X and Rs.6 for each unit of y,(i) formulate an LPP to maximize the profit
View SolutionA company produces two types of goods, `A` and `B`, that require gold and silver. Each unit of type `A` requires `3 gm` of silver and `1 gm` of gold while that of type `B` requires `1 gm` of silver and `2 gm` of gold. The company can use `9 gm` of silver and `8 gm` of gold. If each unit of type `A` brings a profit of Rs. `40` and that of type B Rs. `50`, find the number of units of each type that the company should produce to maximise the profit. What is the maximum profit.
View SolutionSolve the following problem graphically.
Minimise and Maximise `Z=3 x+9 y`
subject to the constraints: `x+3 y le 60`, `x+y ge 10`, `x leq y`, `x ge 0, y ge 0`
View SolutionAn aeroplane can carry a maximum of 200 passengers. A profit of Rs 1000 made on each executive class ticket and a profit of Rs 600 is made on economy class ticket. The airline reserves atleast 20 seats for executive class. However, at least 4 times as many passengers prefer to travel by economy class than by the executive class. Determine how many tickets of each type sold in order to maximise the profit for the airline. What is the maximum profit?
View SolutionChoose the correct answer from the bracket.If an LPP is consistent,then its feasible region is always
View SolutionMaximise` Z=2x+3y` subject to the
constraints x+y<4`,`x>0`,`y>0`
View SolutionA manufacturer makes two types of tea cups, say A and B. Three machines are needed for the manufacturing and the time in minutes required for each cup on the machine is given below:
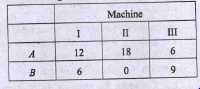
Each machine is available for a maximum of 6 hrs per day. If the profit on each cup A is 75 paise and that on each cup B is 50 paise. Show that 15 tea cups of type A and 30 tea cups of type B should be manufactured in a day to get the maximum profit.
View SolutionA diet is to contain atleast 80 units of vitamin A and 100 units of minerals.Two foods F1 and F2 are available.Food F1 costs Rs 4 per unit food and F2 costs Rs 6 per unit.One unit of food F1 contains 3 units of vitamin A and 4 units of minerals.One unit of food F2 contains 6 unit of of vitamin A and 3 units of minerals.Formulate this as a linear programming problem.Find the minimum costs for diet that consists of misture of these two foods and also meets the minimal nutritional requirements.
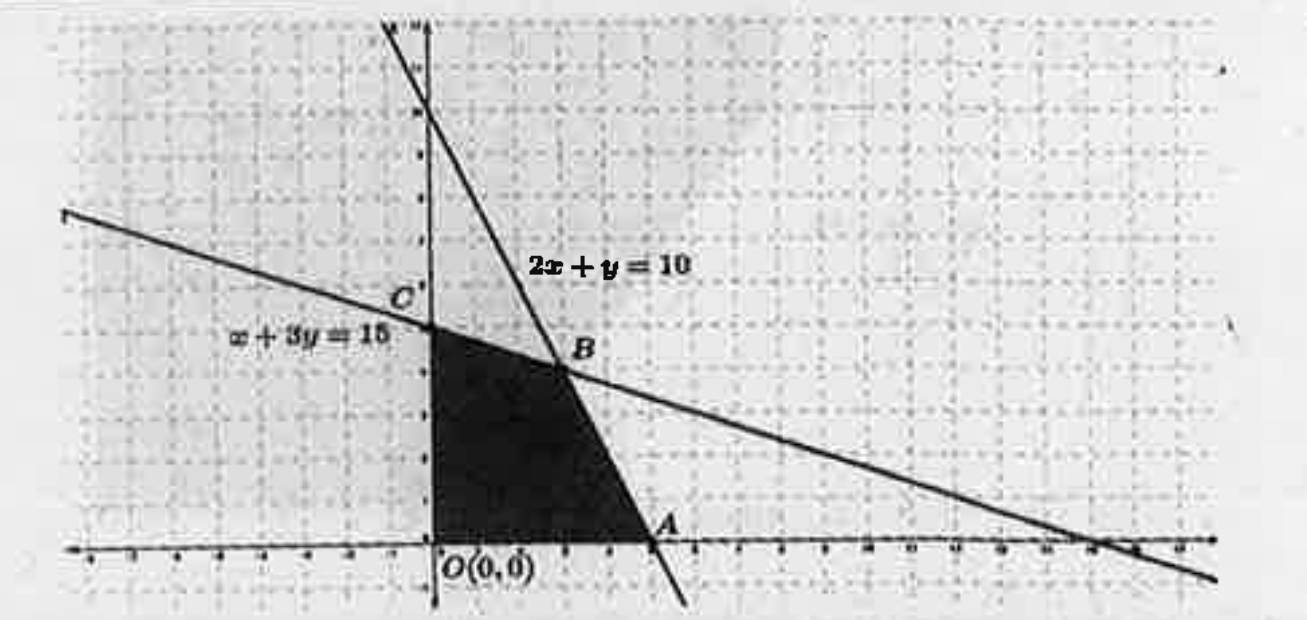
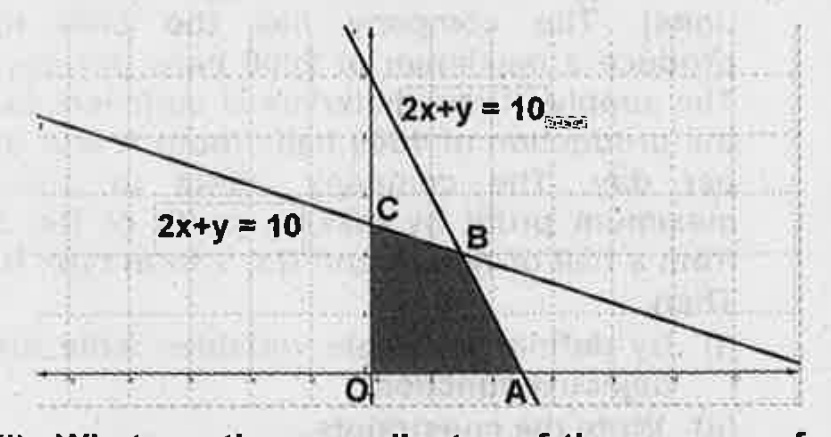
View SolutionThe graph of linear programing problem is given below.The shaded region is the feasible region.The objective function is
` Z=px+qy`
What are the co ordinates of the corners of the feasible region.
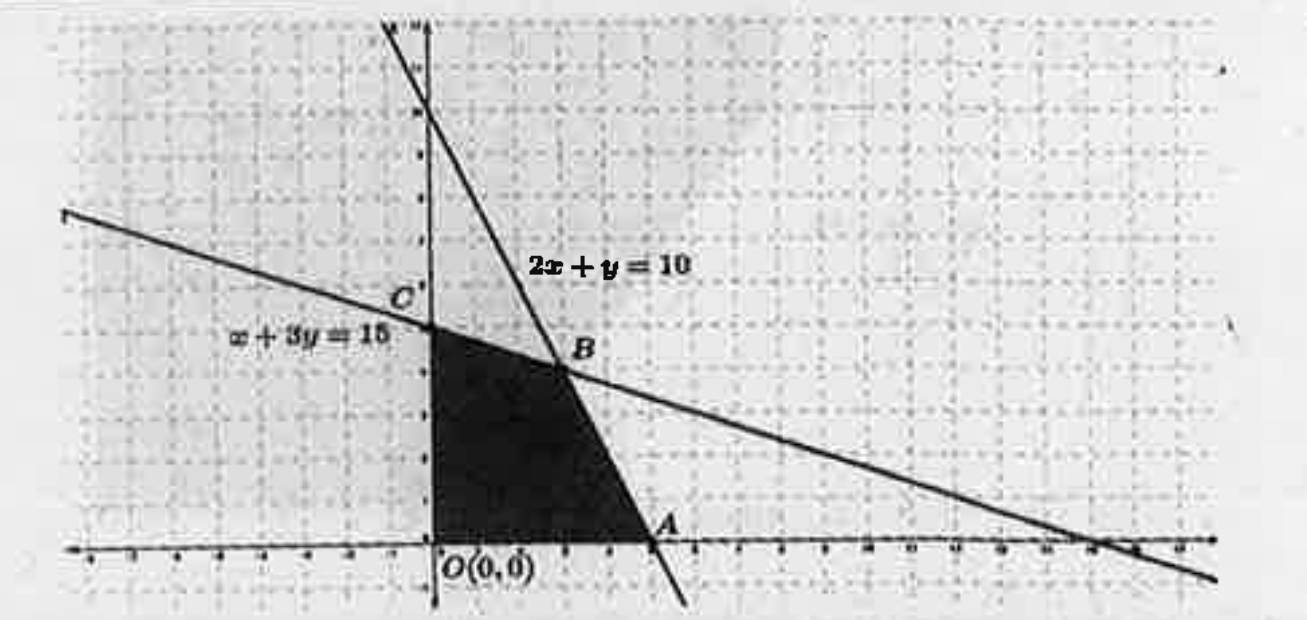
View SolutionThe graph of linear programing problem is given below.The shaded region is the feasible region.The objective function is
` Z=px+qy`
Write the constraints.
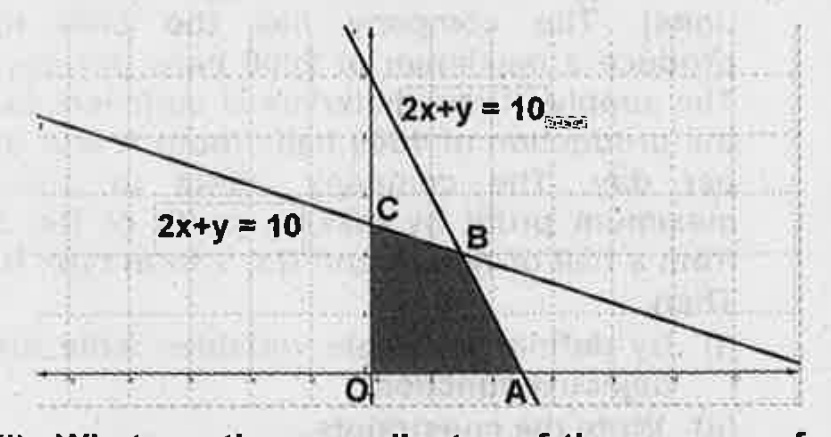
View SolutionThe graph of linear programming problem is given below.The shaded region is the feasible region.The objective function is Maximise, `Z =px+qy`
` If the Max,Z occurs at A and B,what is the relation between pand q?
View SolutionThe graph of linear programming problem is given below.The shaded region is the feasible region.The objective function is Maximise, `Z =px+qy`
If q=1, write the objective function when maximum of Z occures at A and B.`
View SolutionThe graph of linear programing problem is given below.The shaded region is the feasible region.The objective function is
`Z=3x+2y`
Find the Max Z.
View SolutionA dietician wishes to mix two type of foods in such a way that vitamin contents of the mixture contain at least 8 units of vitamin A and the 10 units of vitamin C. Food I contains 2`(unit)/(kg) ` of vitamin A and 1`(unit)/(kg)` of vitamin C. Food II contains 1 `(unit)/(kg)` of vitamin A and 2`(unit)/(kg)` of vitamin C. It costs Rs.`(50)/(kg)` to purchase food I and Rs. `(70)/(kg)` to purchase food II. Formulate this problem as a linear programming problem to minimise the cost of such a mixture ?
View SolutionReshma wishes to mix two types of food P and Q in such a way that the vitamin contents of the mixture contain atleast of 8 units of the vitamine A and 11 units of vitamine B. Food P costs Rs . `(60)/(kg)` and Food Q costs Rs. `(80)/(kg)` . Food P contains 3`(units)/(kg)` of vitamine A and 5`(units)/(kg)` of vitamine B. While food Q contains 4`(unit)/(kg)` of vitamine A and 2`(unit)/(kg)` of vitamine B. Determine the minimum cost of the mixture.
View SolutionDraw the graph of x+3y=3,x+y=2,x,y=0.
View SolutionSolve the following LPP Graphically,
Minimise, `Z=3x+5y`
Subject to constraints,
`x+3yge3, x+yge2,xge0,yge0`
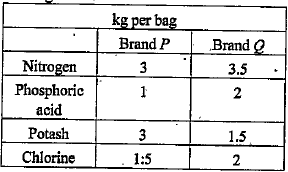
View SolutionA fruit grower can use two types of fertilizers in his garden , brand P and Q . The amounts (in kg ) of nitrogen , phosphoric acid , potash and chlorine in a bag of each brand are given in the table. Tests indicate that the garden needs atleast 240 kg of phosphoric acid , atleast 270 kg of potash and atmost 310 kg of chlorine. If the grower wants to minimise the amount of nitrogen added to the garden, how many bags of each brand should be used ? What is the minimum amount of nitrogen added in the garden ?
View SolutionSolve the linear programming problem graphically:
Max: `z=3x+2y`
Subject to:
`x+2yle10,3x+yle15,xge0,yge0`
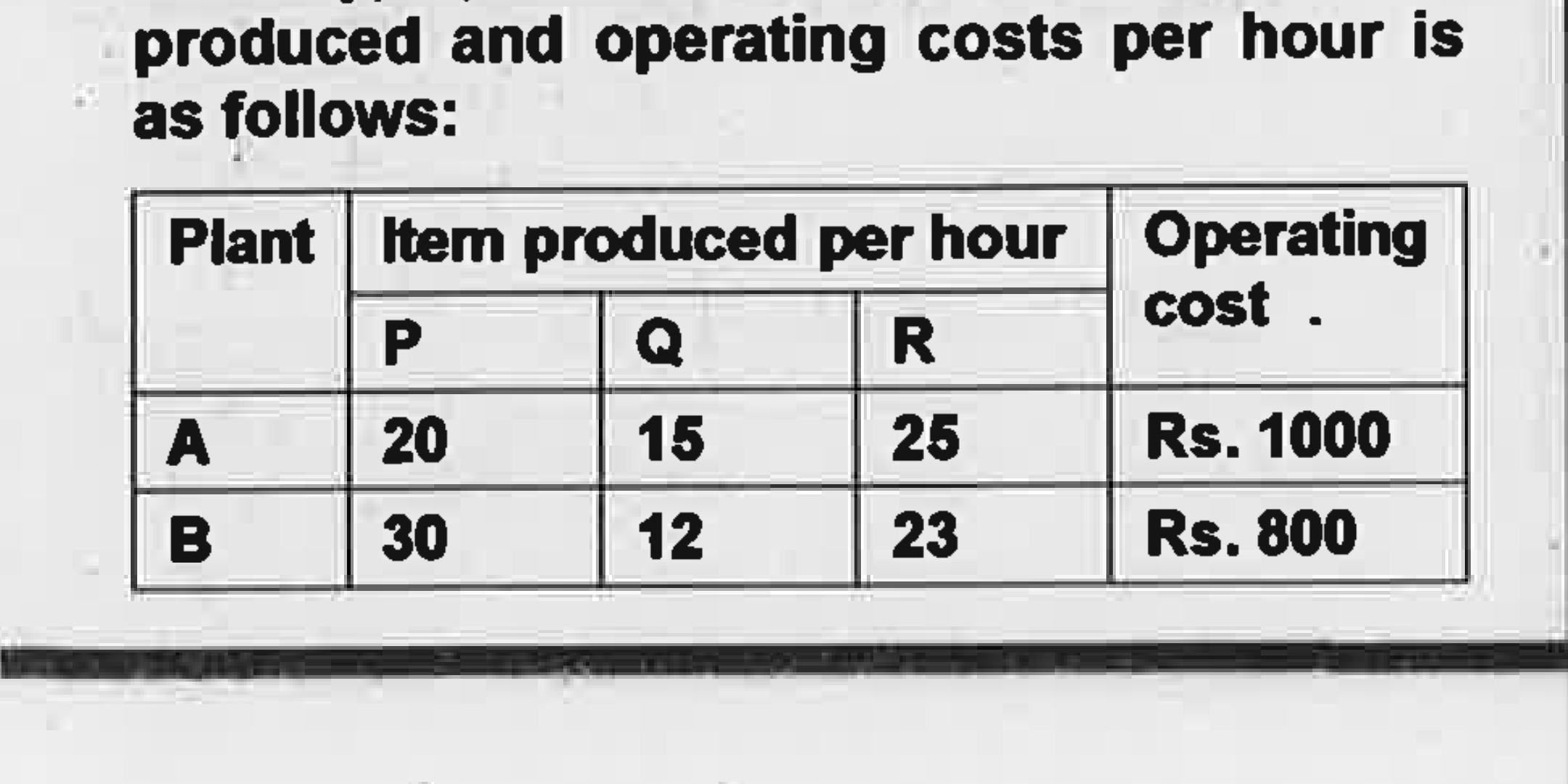
View SolutionA factory produces three items P,Q and R at two plants A and B . The number of items produced and operating costs per hour is as follows:
It is desired to produce at least 500 items of type P , at least 400 items of type Q and 300 items of type R per day.
Write the objective function and constraints.
View SolutionA manufacturer produces nuts and bolts. It takes 1 hour of work on machine A and 3 hours on machine B to produce a package of nuts. It takes 3 hours on machine A and 1 hour on machine B to produce a package of bolts. He earns profit of Rs. 17.50 per package on nuts and Rs. 7.00 per package on bolts. Formulate the above LPP if the machine operates for at most 12 hours a day
View SolutionSolve the LPP:
Maximize Z=-3x+4y
Subject to
`x+2yle8`,
`3x+2yle12`,
`xge0,yge0`.
View SolutionConsider the linear programming problem:
Maximize Z=50x+40y
Subject to the constraints
`x+2yge10`
`3x+4yle24`
`xge0,yge0`
Find the maximum value of Z.
View SolutionConsider the linear programming problem:
Maximize Z=50x+40y
Subject to the constraints
`x+2yge10`
`3x+4yle24`
`xge0,yge0`
Find the corner points of the feasible region.
View SolutionConsider the linear programming problem:
Maximize Z=50x+40y
Subject to the constraints
`x+2yge10`
`3x+4yle24`
`xge0,yge0`
Find the maximum value of Z.
View SolutionConsider the following L.P.P.Maximize Z=3x+2y subject to the constraints `x+2yle10`,`3x+yle15`,`x,yge0`.a.Draw its feasible region.
View SolutionConsider the LPP
Maximise `z=3x+2y`
Subject to the constraints
`x+2yle10`,3x+y`le`15`,`x,y`ge`0`
Find the corner points of the feasible region.
View SolutionConsider the LPP
Maximise `z=3x+2y`
Subject to the constraints
`x+2yle10`,3x+y`le`15`,`x,y`ge`0`
Find the maximum value of Z.
View SolutionConsider the linear inequalities
`2x+3yle6`,`2x+yle4`,`x,yge0`
Mark the feasible region.
View SolutionConsider the linear inequalities
`2x+3yle6`,`2x+yle4`,`x,yge0`
Maximise the function `z=4x+5y` subject to the given constraints.
View SolutionIn factory there are two machines A and B producing toys.They respectively produce 60 and 80 units in one hour.A can run a maximum of 10 hours and B a maximum of 7 hours a day .The cost of their running per hour respectively amount to 2,000 and 2,500 rupee.The total duration of working these machines cannot exceed 12 hours a day.If the total cost cannot exceed Rs. 25,000 per day and the total daily production is atleast 800 units,then formulate the problem mathematically.
View SolutionConsider the LPP
Maximise, `Z=5x+3y`
Subject to,`3x+5yle15`,`5x+2yle10`,`x,yge0`
Draw the feasible region.
View SolutionConsider the LPP
Maximise, `Z=5x+3y`
Subject to,`3x+5yle15`,`5x+2yle10`,`x,yge0`
Find the corner points of the feasible region.
View SolutionConsider the LPP
Maximise, `Z=5x+3y`
Subject to,`3x+5yle15`,`5x+2yle10`,`x,yge0`
Find the corner at which Z attains its maximum.
View SolutionConsider the LPP
Minimise,Z=200x+500y
`x+2yge10`,`3x+4yle24`,`xge0`,`yge0`
Draw the feasible region.
View SolutionConsider the LPP
Minimise,Z=200x+500y
`x+2yge10`,`3x+4yle24`,`xge0`,`yge0`
Find the co-ordinates of the corner points of the feasible region.
View SolutionConsider the LPP
Minimize,Z=200x+500y
`x+2yge10`,`3x+4yle24`,`xge0`,`yge0`
Solve the LPP.
View SolutionConsider the linear programming problem,
Maximise, `Z=x+y`,`2x+y-3le0`,`x-2y+1le0`,`yle3`,`xge0`,`yge0`
Draw its feasible region.
View SolutionConsider the linear programming problem,
Maximize, `Z=x+y`, subject to constraints `2x+y-3le0`,`x-2y+1le0`,`yle3`,`xge0`,`yge0`
Find the corner points of the feasible region.
View SolutionConsider the linear programming problem,
Maximise, `Z=x+y`, subject to the constraints `2x+y-3le0`,`x-2y+1le0`,`yle3`,`xge0`,`yge0`
Find the corner at which Z attains its maximum.
View SolutionA manufacturur produces nuts and bolts.It takes 1 hour of work on machine A and 3 hours on machine B to produce a package of nuts. It takes 3 hours on machine A and 1 hour on machine B to produce a package of bolts.He earns a profit of Rs.17.50 per package on nuts and Rs.7 per package on bolts.How many package of each should be produced each day so as to maximise the profit,if he operates his machine for at the most 12 hours a days?
By suitable defining the variables write the objective function of the problem.
View SolutionA manufacturur produces nuts and bolts.It takes 1 hour of work on machine A and 3 hours on machine B to produce a package of nuts. It takes 3 hours on machine A and 1 hour on machine B to produce a package of bolts.He earns a profit of Rs.17.50 per package on nuts and Rs.7 per package on bolts.How many package of each should be produced each day so as to maximise the profit,if he operates his machine for at the most 12 hours a days?
Formulate the problem as a linear programming problem.
View SolutionA manufacturur produces nuts and bolts.It takes 1 hour of work on machine A and 3 hours on machine B to produce a package of nuts. It takes 3 hours on machine A and 1 hour on machine B to produce a package of bolts.He earns a profit of Rs.17.50 per package on nuts and Rs.7 per package on bolts.How many package of each should be produced each day so as to maximise the profit,if he operates his machine for at the most 12 hours a days?
Solve the LPP graphically and find the number of packages of nuts and bolts to be manufactured.
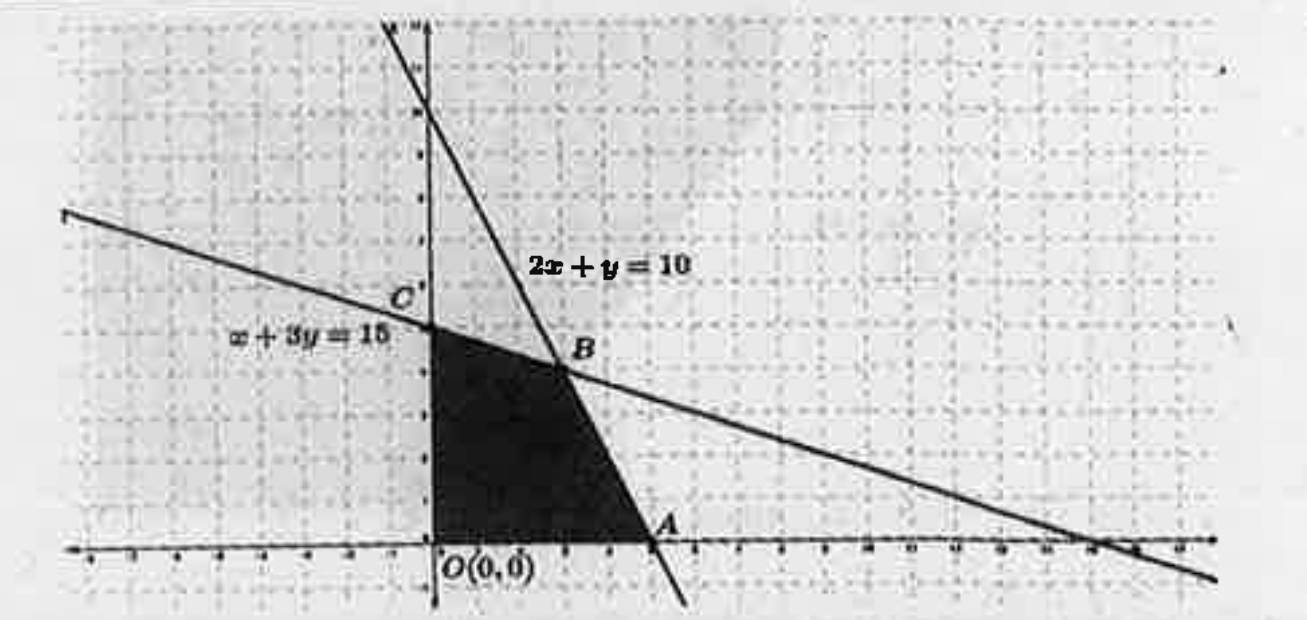
View SolutionThe graph of linear programing problem is given below.The shaded region is the feasible region.The objective function is
` Z=px+qy`
What are the co ordinates of the corners of the feasible region.

View SolutionThe graph of linear programing problem is given below.The shaded region is the feasible region.The objective function is
` Z=px+qy`
Write the constraints.

View SolutionThe graph of linear programming problem is given below.The shaded region is the feasible region.The objective function is Maximise, `Z =px+qy`
` If the Max,Z occurs at A and B,what is the relation between pand q?

View SolutionThe graph of linear programming problem is given below.The shaded region is the feasible region.The objective function is Maximise, `Z =px+qy`
If q=1, write the objective function when maximum of Z occures at A and B.`

View SolutionThe graph of linear programming problem is given below.The shaded region is the feasible region.The objective function is Maximise, `Z =px+qy`
` If the Max,Z occurs at A and B,what is the relation between pand q?

View Solution
EXAMPLE questions and complete solutions for chapter LINEAR PROGRAMMING of BODY BOOKS PUBLICATION of Class 12