Complete Solutions to For Problems of chapter CIRCLE of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | CIRCLE |
| CENGAGE NEW | For Problems |
| Number Of Questions | 43 |
| Academic Year | 2023-24 |
Tangents PA and PB are drawn to the circle `(x-4)^(2)+(y-5)^(2)=4` from the point P on the curve`y=sin x` , where A and B lie on the circle. Consider the function `y= f(x)` represented by the locus of the center of the circumcircle of triangle PAB. Then answer the following questions.
Which of the following is true ?
View SolutionTangents PA and PB are drawn to the circle `(x-4)^(2)+(y-5)^(2)=4` from the point P on the curve`y=sin x` , where A and B lie on the circle. Consider the function `y= f(x)` represented by the locus of the center of the circumcircle of triangle PAB. Then answer the following questions.
Which of the following is true ?
View SolutionTangents PA and PB are drawn to the circle `(x-4)^(2)+(y-5)^(2)=4` from the point P on the curve`y=sin x` , where A and B lie on the circle. Consider the function `y= f(x)` represented by the locus of the center of the circumcircle of triangle PAB. Then answer the following questions.
Which of the following is true ?
View Solutionabout to only mathematics
View SolutionConsider a family of circles passing through the point (3,7) and (6,5). Answer the following questions.
If each circle in the family cuts the circle `x^(2)+y^(2)-4x-6y-3=0`, then all the common chords pass through the fixed point which is
View SolutionConsider a family of circles passing through the point (3,7) and (6,5). Answer the following questions.
If the circle which belongs to the given family cuts the circle `x^(2)+y^2=29` orthogonally, then the center of that circle is (a) (1 / 2 , 3 / 2 ) (b) ( 9 / 2 , 7 / 2 ) (c) ( 7 / 2 , 9 / 2 ) (d) ( 3 , − 7 / 9 )
View SolutionConsider the relation `4l^(2)-5m^(2)+6l+1=0`, where l, m `inR`.
The line lx+my+1=0 touches a fixed circle whose equation is
View SolutionConsider the relation `4l^(2)-5m^(2)+6l+1=0` , where `l,m in R`
Tangents PA and PB are drawn to the above fixed circle from the points P on the line `x+y-1=0` . Then the chord of contact AP passes through the fixed point. (a) ( 1 / 2 , − 5 / 2 ) (b) ( 1 3 , 4 / 3 ) (c) ( − 1 / 2 , 3 / 2 ) (d) none of these
View SolutionConsider the relation `4l^(2)-5m^(2)+6l+1=0` , where `l,m in R`
The number of tangents which can be drawn from the point (2,-3) to the above fixed circle are
View SolutionA circle C whose radius is 1 unit, touches the x-axis at point A. The centre Q of C lies in first quadrant. The tangent from origin O to the circie touches it at T and a point P lies on it such that `DeltaOAP` is a right angled triangle at A and its perimeter is 8 units. The length of `QP` is
View SolutionA circle C whose radius is 1 unit touches the x-axis at point A. The center Q of C lies in the first quadrant. The tangent from the origin O to the circle touches it at T and a point P lies on its such that `Delta OAP` is a right - angles triangle at A and its perimeter is 8 units.
The length of PQ is
View SolutionA circle C whose radius is 1 unit touches the x-axis at point A. The center Q of C lies in the first quadrant. The tangent from the origin O to the circle touches it at T and a point P lies on its such that `Delta OAP` is a right - angles triangle at A and its perimeter is 8 units.
The length of PQ is
View SolutionP is a variable point of the line L = 0. Tangents are drawn to the circle `x^2 + y^2 = 4` from P to touch it at Q and R. The parallelogram PQSR is completed. If `L = 2x + y - 6 = 0`, then the locus of circumcetre of `trianglePQR` is -
View SolutionP is a variable point on the line `L=0` . Tangents are drawn to the circles `x^(2)+y^(2)=4` from P to touch it at Q and R. The parallelogram PQSR is completed.
If `P-= (6,8)`, then the area of `Delta QRS` is
View SolutionP is a variable point on the line `L=0` . Tangents are drawn to the circles `x^(2)+y^(2)=4` from P to touch it at Q and R. The parallelogram PQSR is completed.
If `P -=(3,4)`, then the coordinates of S are
View SolutionTo the circle `x^2 + y^2 = 4` two tangents are drawn from `P (-4, 0)`, which touch the circle at `T_1`, and `T_2` and a rhombus `PT_1P'T_2` is completed. Circumcentre of the triangle `PT_1T_2` is at
View SolutionTo the circle `x^(2)+y^(2)=4`, two tangents are drawn from `P(-4,0)`, which touch the circle at `T_(1)` and `T_(2)` . A rhomus `PT_(1)P'T_(2)` s completed.
The circumcenter of triangle `PT_(1)T_(2)` is at
View SolutionTo the circle `x^(2)+y^(2)=4`, two tangents are drawn from `P(-4,0)`, which touch the circle at `T_(1)` and `T_(2)` . A rhomus `PT_(1)P'T_(2)` s completed.
If P is taken to be at (h,0) such that P' lies on the circle, the area of the rhombus is
View SolutionLet `alpha` chord of a circle be that chord of the circle which subtends an angle `alpha` at the center.
If `x+y=1` is a chord of `x^(2)+y^(2)=1`, then `alpha` is equal to
View SolutionIf `alpha-` chord of a circle be that chord which subtends an angle `alpha` at the centre of the circle.
If slope of `a(pi)/(3)` chord of `x^(2)+y^(2)=4` is 1, then its equaiton is
View SolutionIf `alpha-` chord of a circle be that chord which subtends an angle `alpha` at the centre of the circle.
Distance of `(2pi)/(3)-` chord of `x^(2)=y^(2)+2x+4y+1=0` from the centre is
View SolutionTwo variable chords AB and BC of a circle `x^(2)+y^(2)=a^(2)` are such that AB=BC=a, M and N are the mid-points of AB and BC respectively such that line joining MN intersect the circle at P and Q where P is closer to AB and O is the centre of the circle.
`angleOAB` is
View SolutionTwo variable chords AB and BC of a circle `x^(2)+y^(2)=a^(2)` are such that AB=BC=a, M and N are the mid-points of AB and BC respectively such that line joining MN intersect the circle at P and Q where P is closer to AB and O is the centre of the circle.
`angleOAB` is
View SolutionTwo variable chords AB and BC of a circle `x^(2)+y^(2)=a^(2)` are such that `AB=BC=a`. M and N are the midpoints of AB and BC, respectively, such that the line joining MN intersects the circles at P and Q, where P is closer to AB and O is the center of the circle.
The locus of the points of intersection of tangents at A and C is
View SolutionGiven two circles intersecting orthogonally having the length of common chord `(24)/(5)` unit. The radius of one of the circles is 3 units.
If radius of other circle is `lamda` units then `lamda` is
View SolutionGiven two circles intersecting orthogonally having the length of common chord `(24)/(5)` unit. The radius of one of the circles is 3 units.
If radius of other circle is `lamda` units then `lamda` is
View SolutionGiven two circles intersecting orthogonally having the length of common chord `(24)/(5)` unit. The radius of one of the circles is 3 units.
If radius of other circle is `lamda` units then `lamda` is
View SolutionIn the given figure, there are two circles with centers A and B. The common tangent to the circles touches them, respectively,at P and Q. AR is 40cm and AB is divided by the point of contact of the circles in the ratio `5:3`
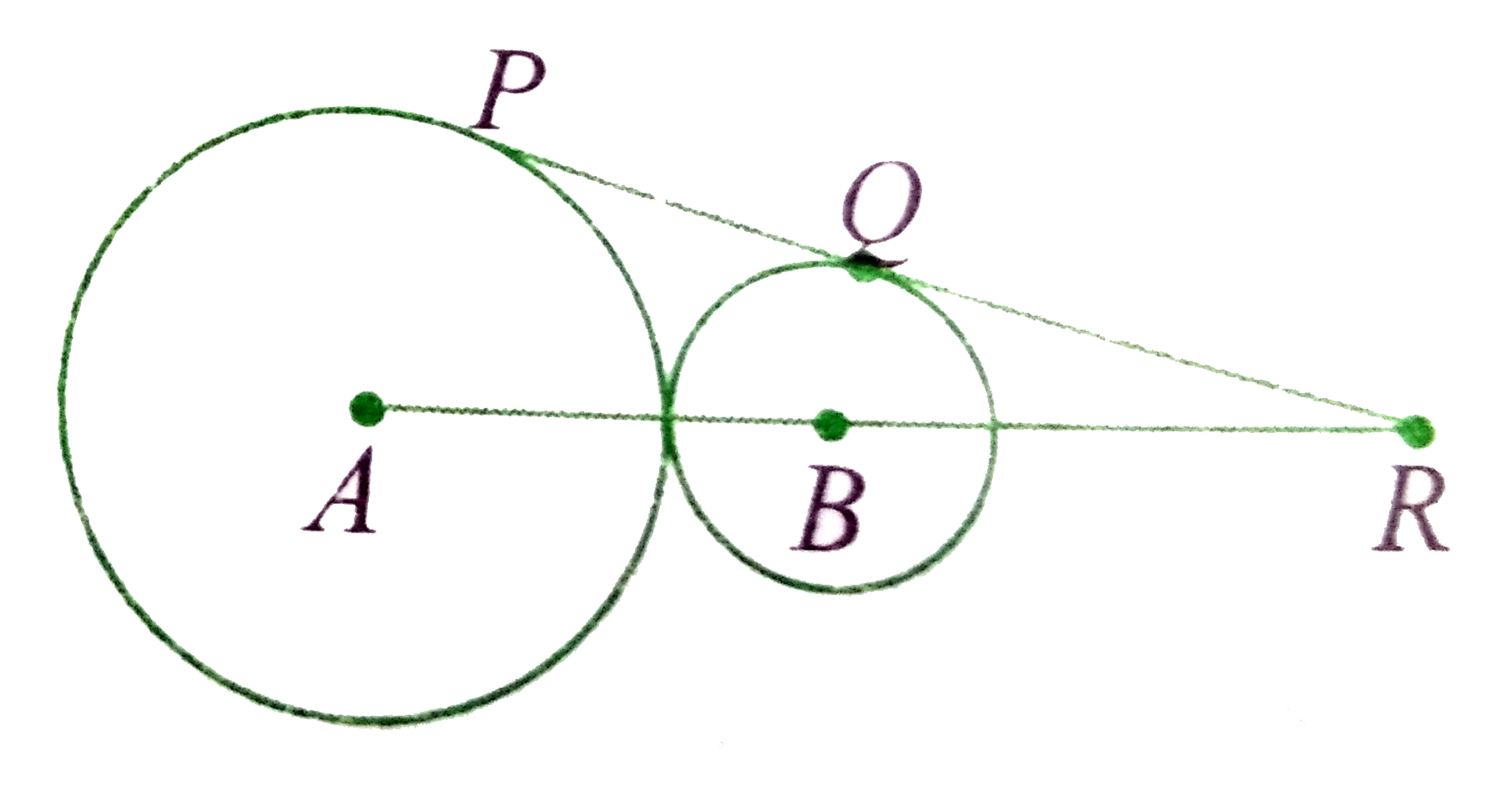
The radius of the circle with center B is
View SolutionIn the given figure, there are two circles with centers A and B. The common tangent to the circles touches them, respectively,at P and Q. AR is 40cm and AB is divided by the point of contact of the circles in the ratio `5:3`

The radius of the circle with center B is
View SolutionIn the given figure, there are two circles with centers A and B. The common tangent to the circles touches them, respectively,at P and Q. AR is 40cm and AB is divided by the point of contact of the circles in the ratio `5:3`

The radius of the circle with center B is
View SolutionLet the circles
`S_(1)-=x^(2)+y^(2)+4y-1=0`
`S_(2)-= x^(2)+y^(2)+6x+y+8=0`
touch each other . Also, let `P_(1)` be the point of contact of `S_(1)` and `S_(2) `, `C_(1)` and `C_(2)` are the centres of `S_(1)`and`S_(2)` respectively.
The coordinates of `P_(1)` are
View SolutionLet each of the circles
`S_(1)-=x^(2)+y^(2)+4y-1=0`
`S_(1)-= x^(2)+y^(2)+6x+y+8=0`
`S_(3)-=x^(2)+y^(2)-4x-4y-37=0`
touch the other two. Also, let `P_(1),P_(2)` and `P_(3)` be the points of contact of `S_(1)` and `S_(2) , S_(2)` and `S_(3)`, and `S_(3)` , respectively, `C_(1),C_(2)` and `C_(3)` are the centres of `S_(1),S_(2)` and `S_(3)` respectively.
The ratio `("area"(DeltaP_(1)P_(2)P_(3)))/("area"(DeltaC_(1)C_(2)C_(3)))` is equal to
View SolutionLet each of the circles
`S_(1)-=x^(2)+y^(2)+4y-1=0`
`S_(1)-= x^(2)+y^(2)+6x+y+8=0`
`S_(3)-=x^(2)+y^(2)-4x-4y-37=0`
touch the other two. Also, let `P_(1),P_(2)` and `P_(3)` be the points of contact of `S_(1)` and `S_(2) , S_(2)` and `S_(3)`, and `S_(3)` , respectively, `C_(1),C_(2)` and `C_(3)` are the centres of `S_(1),S_(2)` and `S_(3)` respectively.
The ratio `("area"(DeltaP_(1)P_(2)P_(3)))/("area"(DeltaC_(1)C_(2)C_(3)))` is equal to
View SolutionThe line `x+2y+a=0` intersects the circle `x^(2)+y^(2)-4=0` at two distinct points A and B. Another line `12x-6y-41=0` intersects the circle `x^(2)+y^(2)-2y+1=0` at two distinct point C and D.
The equation of the circle passing through the points A,B,D, and D is
View SolutionThe line `x + 2y = a` intersects the circle `x^2 + y^2 = 4` at two distinct points `A and B` Another line `12x - 6y - 41 = 0` intersects the circle `x^2 + y^2 - 4x - 2y + 1 = 0` at two `C and D`. The value of 'a' for which the points `A,B,C and D` are concyclic -
View SolutionLet A,B, and C be three sets such that
`A={(x,y)|(x)/(cos theta)=(y)/(sintheta)=5,"where" 'theta'"is parameter"}`
`B= {(x,y)|(x-3)/(cos phi)=(y-4)/(sin phi)=r}`
`C= { (x,y)|(x-3)^(2)+(y-4)^(2)leR^(2)}`
If `A capC =A`, then minimum value of R is
(a)5
(b)6
(c)10
(d)11
View SolutionLet A,B, and C be three sets such that
`A={(x,y)|(x)/(cos theta)=(y)/(sintheta)=5,"where" 'theta'"is parameter"}`
`B= {(x,y)|(x-3)/(cos phi)=(y-4)/(sin phi)=r}`
`C= { (x,y)|(x-3)^(2)+(y-4)^(2)leR^(2)}`
If `phi` is fixed and r varies and `(A cap B) =1`, then `sec phi` is equal to (a) `(5)/(4)` (b) `(-5)/(4)` (c) `(5)/(3)` (d) `(-5)/(3)`
View SolutionConsider the family of circles `x^(2)+y^(2)-2x-2ay-8=0` passing through two fixed points A and B . Also, `S=0` is a cricle of this family, the tangent to which at A and B intersect on the line `x+2y+5=0`.
The distance between the points A and B , is
View SolutionConsider the family of circles `x^(2)+y^(2)-2x-2ay-8=0` passing through two fixed points A and B . Also, `S=0` is a cricle of this family, the tangent to which at A and B intersect on the line `x+2y+5=0`.
The distance between the points A and B , is
View SolutionConsider the family of circles `x^(2)+y^(2)-2x-2ay-8=0` passing through two fixed points A and B . Also, `S=0` is a cricle of this family, the tangent to which at A and B intersect on the line `x+2y+5=0`.
If the circle `x^(2)+y^(2)-10x+2y=c=0` is orthogonal to `S=0`, then the value of c is
View SolutionA circle C of radius 1 is inscribed in an equilateral triangle PQR. The points of contact of C with the sides PQ, QR, RP are D, E, F, respectively. The line PQ is given by the equation `sqrt3 x+ y -6 = 0` and the point D is (3 sqrt3/2, 3/2). Further, it is given that the origin and the centre of C are on the same side of the line PQ. (1)The equation of circle C is (2)Points E and F are given by (3)Equation of the sides QR, RP are
A. `y=(2)/(sqrt3)+x+1,y=-(2)/(sqrt3)x-1`
B. `y=(1)/(sqrt3)x,y=0`
C. `y=(sqrt3)/(2)x+1,y=-(sqrt3)/(2)x-1`
D. `y=sqrt3x,y=0`
View SolutionA circle C of radius 1 is inscribed in an equilateral triangle PQR. The points of contact of C with the sides PQ, QR, RP are D, E, F, respectively. The line PQ is given by the equation `sqrt3 x+ y -6 = 0` and the point D is (3 sqrt3/2, 3/2). Further, it is given that the origin and the centre of C are on the same side of the line PQ. (1)The equation of circle C is (2)Points E and F are given by (3)Equation of the sides QR, RP are
A. `y=(2)/(sqrt3)+x+1,y=-(2)/(sqrt3)x-1`
B. `y=(1)/(sqrt3)x,y=0`
C. `y=(sqrt3)/(2)x+1,y=-(sqrt3)/(2)x-1`
D. `y=sqrt3x,y=0`
View SolutionA circle C of radius 1 is inscribed in an equilateral triangle PQR. The points of contact of C with the sides PQ, QR, RP are D, E, F, respectively. The line PQ is given by the equation `sqrt3 x+ y -6 = 0` and the point D is (3 sqrt3/2, 3/2). Further, it is given that the origin and the centre of C are on the same side of the line PQ. (1)The equation of circle C is (2)Points E and F are given by (3)Equation of the sides QR, RP are
A. `y=(2)/(sqrt3)+x+1,y=-(2)/(sqrt3)x-1`
B. `y=(1)/(sqrt3)x,y=0`
C. `y=(sqrt3)/(2)x+1,y=-(sqrt3)/(2)x-1`
D. `y=sqrt3x,y=0`
View Solution
For Problems questions and complete solutions for chapter CIRCLE of CENGAGE MATHS (ENGLISH) of Class 12
All Exercises from CENGAGE NEW Class 12 MATHS CIRCLE
- ARCHIVES (JEE MAIN)
- CONCEPT APPLICATION EXERCISE 4.1
- CONCEPT APPLICATION EXERCISE 4.10
- CONCEPT APPLICATION EXERCISE 4.11
- CONCEPT APPLICATION EXERCISE 4.12
- CONCEPT APPLICATION EXERCISE 4.13
- CONCEPT APPLICATION EXERCISE 4.14
- CONCEPT APPLICATION EXERCISE 4.15
- CONCEPT APPLICATION EXERCISE 4.16
- CONCEPT APPLICATION EXERCISE 4.17
- CONCEPT APPLICATION EXERCISE 4.18
- CONCEPT APPLICATION EXERCISE 4.19
- CONCEPT APPLICATION EXERCISE 4.2
- CONCEPT APPLICATION EXERCISE 4.20
- CONCEPT APPLICATION EXERCISE 4.3
- CONCEPT APPLICATION EXERCISE 4.4
- CONCEPT APPLICATION EXERCISE 4.5
- CONCEPT APPLICATION EXERCISE 4.6
- CONCEPT APPLICATION EXERCISE 4.7
- CONCEPT APPLICATION EXERCISE 4.8
- CONCEPT APPLICATION EXERCISE 4.9
- Examples
- Excercises (Single Correct Answer Type)
- For Problems
- For problems 3 and 4
- ILLUSTRATION
- JEE ADVANCED (Single Correct Answer Type)
- LINKED COMPREHENSION TYPE (For problem 1 and 2)
- Linked Comprehension Type (For Problem 1-3)
- MATRIX MATCH TYPE
- Multiple Correct Anser Type
- NUMERICAL VALUE TYPE
- Single Correct Answer Type