Complete Solutions to Illustration of chapter PROBABILITY I of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PROBABILITY I |
| CENGAGE NEW | Illustration |
| Number Of Questions | 42 |
| Academic Year | 2023-24 |
A coin is tossed three times, consider the following events. A : No head appears, B: Exactly one head appears and C: Atleast two appear. Do they form a set of mutually exclusive and exhaustive events?
View SolutionFind the probability of getting more than 7 when two dice are rolled.
View SolutionA die is loaded so that the probability of a face `i` is proportional to `i ,i=1,2, 6.` Then find the probability of an even number occurring when the die in rolled.
View SolutionConsider the experiment of tossing a coin. If the coin shows head, toss it again but if it shows tail then throw a die. Find the conditional probability of the event that the die shows a number greater than 4 given that 'there is at least one tail'.
View SolutionFour candidates A, B, C, D have applied for the assignment ot coach a school cricket team. If A is twice as likely to be selected as B, and B and C are given about the same chance of being selected, while C is twice as likely to be selected as D, what are the probability that (i) C will be selected ? (ii) A will not be selected?
View SolutionIf `(1+3p)/3,(1-p)/1,(1-2p)/2` are the probabilities of 3 mutually exclusive events then find the set of all values of p.
View SolutionA determinant is chosen at random from the set of all determinant of order 2 with elements 0 or 1 only. Find the probability that the determinant chosen is nonzero.
View SolutionA die is rolled 4 times. The probability of getting a larger number than the previous number each time is `17//216` b. `5//432` c. `15//432` d. none of these
View SolutionIf a coin is tossed n times, then find the probability that the head appears odd number of times.
View SolutionA card is drawn at random from a pack of cards. What ist the probability that the drawn card is neither a heart nor a king?
View SolutionCard is drawn from a pack of 52 cards. A persons bets that it is a spade or an ace. What are the odds against him of winning this bet?
View SolutionA fair dice is thrown three times. If p, q and r are the numbers obtained on the dice, then find the probability that `i^(p) + i^(q) + i^(r) = 1`, where `I = sqrt(-1)`.
View SolutionOne mapping is selected at random from all the mappings of the set `A={1,2, 3.. n}` into itself. The probability that eh mapping selected is one to one is a. `1/(n^n)` b. `1/(n !)` c. `((n-1)!)/(n^(n-1))` d. none of these
View SolutionTwo integers `xa n dy` are chosen with replacement out of the set `{0,1,,2,3 ,10}dot` Then find the probability that `|x-y|> 5.`
View SolutionFind the probability that the 3Ns come consecutively in the arrangement of the letters of the word CONSTANTINOPLE.
View SolutionOut of `3n` consecutive integers, three are selected at random. Find the probability that their sum is divisible by 3.
View SolutionFind the probability that a randomly chosen three-digit number has exactly three factors.
View SolutionIf `pa n dq` are chosen randomly from the set `{1,2,3,4,5,6,7,8,9, 10}` with replacement, determine the probability that the roots of the equation `x^2+p x+q=0` are real.
View SolutionAn integer is chosen at random and squared. Find the probability that the last digit of the square is 1 or 5.
View SolutionFour fair dices are thrown simultaneously. Find the probability that the highest number obtained is 4.
View SolutionAn unbiased dice, with faces numbered 1, 2, 3, 4, 5, 6, is thrown n times and the list of n numbers shown up is noted. Then find the probability that among the numbers 1, 2, 3, 4, 5, 6 only three numbers appear in this list and each number appears at least once.
View SolutionSix points are there on a circle from which two triangles drawn with no vertex common. Find the probability that none of the sides of the triangles intersect.
View SolutionBalls are drawn one-by-one without replacement from a box containing 2 black, 4 white and 3 red balls till all the balls are drawn. Find the probability that the balls drawn are in the order 2 black, 4 white and 3 red.
View SolutionIn how many ways, can three girls can three girls and nine boys be seated in two vans, each having numbered seats, 3 in the and 4 at the back? How many seating arrangements are possible if 3 girls should sit together in a back row on adjacent seats? Now, if all the seating arrangements are equally likely, what is the probability of 3 girls sitting together in a back row on adjacent seats?
View SolutionFind the probability that the birth days of six different persons will fall in exactly two calendar months.
View SolutionIf 10 objects are distributed at random among 10 persons, then find the probability that at least one of them will not get anything.
View Solution`2^n` players of equal strength are playing a knock out tournament. If they are paired at randomly in all rounds, find out the probability that out of two particular players `S_1a n dS_2,` exactly one will reach in semi-final `(n in N ,ngeq2)dot`
View SolutionFourteen numbered balls (1, 2, 3, …, 14) are divided in 3 groups randomly. Find the probability that the sum of the numbers on the balls, in each group, is odd.
View SolutionFive different digits from the set of numbers {1, 2, 3, 4, 5, 6, 7} are written in random order. Find the probability that five-digit number thus formed is divisible by 9.
View SolutionThree married couples sit in a row. Find the probability that no husband sits with his wife.
View SolutionA box contains 6 nails and 10 nuts. Half of the nails and half of the nuts are rusted. If one item is chosen at random, then find the probability that it is rusted or is a nail.
View SolutionThe probability that at least one of the events `Aa n dB` occurs is 0.6. If `Aa n dB` occur simultaneously with probability 0.2, then find `P( A )+P( B )dot`
View SolutionIf `P(AuuB)=3//4a n dP( A )=2//3` , then find the value of `P( A nnB)dot`
View SolutionLet `A ,B ,C` be three events. If the probability of occurring exactly one event out of `Aa n dBi s1-x ,` out of`Ba n dCi s1-2x ,` out of`Ca n dAi s1-x ,` and that of occuring three events simultaneously is `x^2` , then prove that the probability that atleast one out of A, B, C will occur is greaer than 1/2 .
View SolutionLet A and B be any two events such that P(A) = `(1)/(2)` and P(B) = `(1)/(3)`. Then find the value of `P(A' nn B')' + P(A' uu B')'`.
View SolutionIf A and B are events such that `P(A'uuB') = (3)/(4), P(A'nnB') = (1)/(4)` and `P(A) = (1)/(3)`, then find the value of `P(A' nn B)`
View SolutionA sample space consists of 9 elementary event `E_1, E_2, E_3 ..... E_8, E_9` whose probabilities are `P(E_1) = P(E_2) = 0. 08` ,`P(E_3) = P(E_4)=P(E_5) = 0. 1`, `P(E_6) = P(E_7) = 0. 2` ,`P(E_8) = P(E_9) = 0. 07`. Suppose `A = {E_1,E_5,E_8}`, `B = {E_2, E_5, E_8, E_9}`. Compute `P(A)`, `P(B)` and `P(AnnB)`. Using the addition law of probability, find `P(AuuB)`. List the composition of the event `AuuB`, and calculate, `P(AuuB)` by adding the probabilities of the elementary events. Calculate `P(barB)` from `P(B)`, also calculate `P(barB)` directly from the elementary events of `barB`.
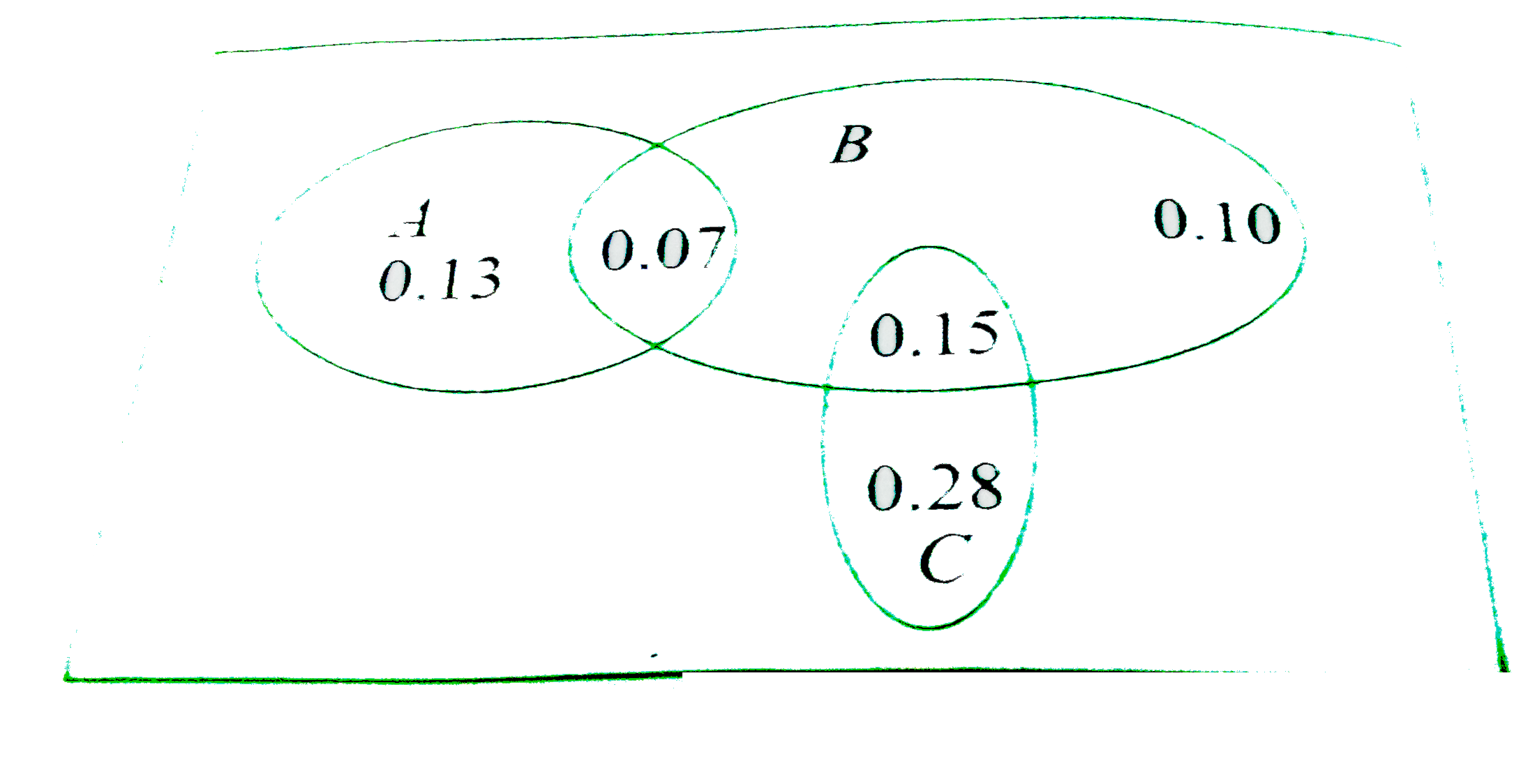
View SolutionThe following Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections.
Determine
(a) P(A)
(b) `P(B nn barC)`
(c ) `P(A uu B)`
(d) `P(A nn barB)`
(e) `P(B nn C)`
(f) Probability of the event that exactly one of A, B, and C occurs.
View SolutionThree numbers are chosen at random without replacement from {1,2,3,....10}. The probability that the minimum of the chosen number is 3 or their maximum is 7 , is:
View SolutionIf A and B are two events such that P(A) = `(1)/(2)` and P(B) = `(2)/(3)`, then show that
(a) `P(Auu B) ge (2)/(3) (b) (1)/(6) le P(A nn B) le (1)/(2)`
(c ) `P(A nn barB) le (1)/(3) (d) (1)/(6) le P(barAnn B) le (1)/(2)`
View SolutionGiven two events `Aa n dB` . If odds against `A` are as 2:1 and those in favour of `AuuB` are 3:1, then find the range of `P(B)dot`
View SolutionThe probabilities of three events `A ,B ,a n dC` are `P(A)=0. 6 ,P(B)=0. 4 ,a n dP(C)`If `P(AnnB)=0. 8 ,P(AnnC)=0. 3 ,P(AnnBnnC)=0.2P(AuuBuuC)>0.85 ,` then find the range of `P(B nn C)dot`
View Solution
Illustration questions and complete solutions for chapter PROBABILITY I of CENGAGE MATHS (ENGLISH) of Class 12